Deck 4: Basic Estimation Techniques
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/51
العب
ملء الشاشة (f)
Deck 4: Basic Estimation Techniques
1
In a linear regression equation of the form Y = a + bX, the intercept parameter a shows
A) the value of X when Y is zero.
B) the value of Y when X is zero.
C) the amount that Y changes when X changes by one unit.
D) the amount that X changes when Y changes by one unit.
A) the value of X when Y is zero.
B) the value of Y when X is zero.
C) the amount that Y changes when X changes by one unit.
D) the amount that X changes when Y changes by one unit.
the value of Y when X is zero.
2
The linear regression equation, Y = a + bX, was estimated. The following computer printout was
obtained:
-Which of the following statements is correct at the 1% level of significance?
A) Both and
are statistically significant.
B) Neither nor
is statistically significant.
C) is statistically significant, but
is not.
D) is statistically significant, but
is not.
is statistically significant, but
is not.
is not.
3
In a linear regression equation Y = a + bX, the fitted or predicted value of Y is
A) the value of Y obtained by substituting specific values of X into the sample regression equation.
B) the value of X associated with a particular value of Y.
C) the value of X that the regression equation predicts.
D) the values of the parameters predicted by the estimators.
E) the value of Y associated with a particular value of X in the sample.
A) the value of Y obtained by substituting specific values of X into the sample regression equation.
B) the value of X associated with a particular value of Y.
C) the value of X that the regression equation predicts.
D) the values of the parameters predicted by the estimators.
E) the value of Y associated with a particular value of X in the sample.
the value of Y obtained by substituting specific values of X into the sample regression equation.
4
The sample regression line
A) shows the actual (or true) relation between the dependent and independent variables.
B) is used to estimate the population regression line.
C) connects the data points in a sample.
D) is estimated by the population regression line.
E) maximizes the sum of the squared differences between the data points in a sample and the sample regression line.
A) shows the actual (or true) relation between the dependent and independent variables.
B) is used to estimate the population regression line.
C) connects the data points in a sample.
D) is estimated by the population regression line.
E) maximizes the sum of the squared differences between the data points in a sample and the sample regression line.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
5
The linear regression equation, Y = a + bX, was estimated. The following computer printout was
obtained:
-If X equals 20, what is the predicted value of Y?
A) 186.42
B) 165.69
C) -186.42
D) -411.72

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
6
The critical value of t is the value that a t-statistic must exceed in order to
A) reject the hypothesis that the true value of a parameter equals zero.
B) accept the hypothesis that the estimated value of parameter equals the true value.
C) reject the hypothesis that the estimated value of the parameter equals the true value.
D) reject the hypothesis that the estimated value of the parameter exceeds the true value.
A) reject the hypothesis that the true value of a parameter equals zero.
B) accept the hypothesis that the estimated value of parameter equals the true value.
C) reject the hypothesis that the estimated value of the parameter equals the true value.
D) reject the hypothesis that the estimated value of the parameter exceeds the true value.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
7
The linear regression equation, Y = a + bX, was estimated. The following computer printout was
obtained:
-The exact level of significance of is
A) 0.171 percent.
B) 1 percent.
C) 1.71 percent.
D) 2.66 percent.
E) 2.921 percent.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
8
The linear regression equation, Y = a + bX, was estimated. The following computer printout was
obtained:
-What is the critical value of t at the 1% level of significance?
A) 1.746
B) 2.120
C) 2.878
D) 2.921

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
9
A parameter estimate is said to be statistically significant if there is sufficient evidence that the
A) sample regression equals the population regression.
B) parameter estimated from the sample equals the true value of the parameter.
C) value of the t-ratio equals the critical value.
D) true value of the parameter does not equal zero.
A) sample regression equals the population regression.
B) parameter estimated from the sample equals the true value of the parameter.
C) value of the t-ratio equals the critical value.
D) true value of the parameter does not equal zero.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
10
The method of least squares
A) can be used to estimate the explanatory variables in a linear regression equation.
B) can be used to estimate the slope parameters of a linear equation.
C) minimizes the distance between the population regression line and the sample regression line.
D) all of the above
A) can be used to estimate the explanatory variables in a linear regression equation.
B) can be used to estimate the slope parameters of a linear equation.
C) minimizes the distance between the population regression line and the sample regression line.
D) all of the above

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
11
The linear regression equation, Y = a + bX, was estimated. The following computer printout was
obtained:
-The value of the R2 statistic indicates that
A) 0.3066% of the total variation in Y is explained by the regression equation.
B) 0.3066% of the total variation in X is explained by the regression equation.
C) 30.66% of the total variation in Y is explained by the regression equation.
D) 30.66% of the total variation in X is explained by the regression equation.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
12
In the linear model , a test of the hypothesis that parameter c equals zero is
A) an F-test.
B) an R2-test.
C) a zero-statistic.
D) a t-test.
E) a Z-test.
A) an F-test.
B) an R2-test.
C) a zero-statistic.
D) a t-test.
E) a Z-test.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
13
In a regression equation, the ______ captures the effects of factors that might influence the dependent variable but aren't used as explanatory variables.
A) intercept
B) slope parameter
C) R-square
D) random error term
A) intercept
B) slope parameter
C) R-square
D) random error term

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
14
Which of the following is an example of a time-series data set?
A) amount of labor employed in each factory in the U.S. in 2010.
B) amount of labor employed yearly in a specific factory from 1990 through 2010.
C) average amount of labor employed at specific times of the day at a specific factory in 2010.
D) All of the above are time-series data sets.
A) amount of labor employed in each factory in the U.S. in 2010.
B) amount of labor employed yearly in a specific factory from 1990 through 2010.
C) average amount of labor employed at specific times of the day at a specific factory in 2010.
D) All of the above are time-series data sets.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
15
An estimator is unbiased if it produces
A) a parameter from the sample that equals the true parameter.
B) estimates of a parameter that are close to the true parameter.
C) estimates of a parameter that are statistically significant.
D) estimates of a parameter that are on average equal to the true parameter.
E) both b and c
A) a parameter from the sample that equals the true parameter.
B) estimates of a parameter that are close to the true parameter.
C) estimates of a parameter that are statistically significant.
D) estimates of a parameter that are on average equal to the true parameter.
E) both b and c

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
16
In a linear regression equation of the form Y = a + bX, the slope parameter b shows
A) X / Y.
B) Y / X.
C) Y / b.
D) X / b.
E) none of the above
A) X / Y.
B) Y / X.
C) Y / b.
D) X / b.
E) none of the above

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
17
The linear regression equation, Y = a + bX, was estimated. The following computer printout was
obtained:
-The parameter estimate of b indicates
A) X increases by 8.03 units when Y increases by one unit.
B) X decreases by 21.36 units when Y increases by one unit.
C) Y decreases by 2.66 units when X increases by one unit.
D) a 10-unit decrease in X results in a 213.6 unit increase in Y.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
18
To test whether the overall regression equation is statistically significant one uses
A) the t-statistic.
B) the R2-statistic.
C) the F-statistic.
D) the standard error statistic.
A) the t-statistic.
B) the R2-statistic.
C) the F-statistic.
D) the standard error statistic.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
19
If an analyst believes that more than one explanatory variable explains the variation in the dependent variable, what model should be used?
A) a simple linear regression model
B) a multiple regression model
C) a nonlinear regression model
D) a log-linear model
A) a simple linear regression model
B) a multiple regression model
C) a nonlinear regression model
D) a log-linear model

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
20
The linear regression equation, Y = a + bX, was estimated. The following computer printout was
obtained:
-The parameter estimate of a indicates
A) when X is zero, Y is 5.09.
B) when X is zero, Y is 15.48.
C) when Y is zero, X is -21.36.
D) when Y is zero, X is 8.03.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
21
Suppose you are testing the statistical significance (at the 1% significance level) of a parameter estimate from the estimated regression model: M = a + bR + cI
Which is estimated using a cross-section data set on 22 firms. The critical value of the appropriate test statistic is
A) tcritical = 2.861.
B) tcritical = -.845.
C) tcritical = 2.845.
D) Fcritical = 5.93.
E) Fcritical = 19.44.
Which is estimated using a cross-section data set on 22 firms. The critical value of the appropriate test statistic is
A) tcritical = 2.861.
B) tcritical = -.845.
C) tcritical = 2.845.
D) Fcritical = 5.93.
E) Fcritical = 19.44.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
22
A firm is experiencing theft problems at its warehouse. A consultant to the firm believes that the dollar loss from theft each week (T) depends on the number of security guards (G) and on the unemployment rate in the county where the warehouse is located (U measured as a percent). In order to test this hypothesis, the consultant estimated the regression equation T = a + bG + cU and obtained the following results:
-If the firm hires 6 guards and the unemployment rate in the county is 10% (U = 10), what is the predicted dollar loss to theft per week?
A) $4,375 per week
B) $5,150 per week
C) $8,300 per week
D) $9,955 per week

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
23
A firm is experiencing theft problems at its warehouse. A consultant to the firm believes that the dollar loss from theft each week (T) depends on the number of security guards (G) and on the unemployment rate in the county where the warehouse is located (U measured as a percent). In order to test this hypothesis, the consultant estimated the regression equation T = a + bG + cU and obtained the following results:
-Which of the following is correct at the 1% level of significance?
A) The regression equation as a whole is statistically significant because the p-value of F is smaller than 0.01.
B) The estimates of the parameters a, b, and c are all statistically significant because the absolute values of their t-ratios exceed 2.797.
C) The estimates of the parameters a, b, and c are all statistically significant because the p- values for, , and are all less than 0.01.
D) The critical value of t is 2.797.
E) all of the above

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
24
If the p-value is 10%, then the
A) level of significance is 10%.
B) level of confidence is 90%.
C) probability of a Type I error is 90%.
D) both a and b
E) all of the above
A) level of significance is 10%.
B) level of confidence is 90%.
C) probability of a Type I error is 90%.
D) both a and b
E) all of the above

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
25
Refer to the following nonlinear model which relates W to P, Q, and R:
The computer output form the regression analysis is:
-The estimated value of a is
A) 0.916
B) 12.182
C) 2.50
D) 2.66
The computer output form the regression analysis is:
-The estimated value of a is
A) 0.916
B) 12.182
C) 2.50
D) 2.66

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
26
Suppose you are testing the statistical significance (at the 5% significance level) of a parameter estimate from the estimated regression equation: Y = a + bR + cS + dW
Which is estimated using a time-series sample containing monthly observations over a 30-month time period. The critical value of the appropriate test statistic is
A) tcritical = 2.042.
B) tcritical = 2.056.
C) Fcritical = 4.22.
D) Fcritical = 7.76.
Which is estimated using a time-series sample containing monthly observations over a 30-month time period. The critical value of the appropriate test statistic is
A) tcritical = 2.042.
B) tcritical = 2.056.
C) Fcritical = 4.22.
D) Fcritical = 7.76.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
27
Refer to the following computer output from estimating the parameters of the nonlinear model
-The nonlinear relation can be transformed into the following linear regression model:
A)
B)
C)
D)
-The nonlinear relation can be transformed into the following linear regression model:
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
28
Refer to the following nonlinear model which relates W to P, Q, and R:
The computer output form the regression analysis is:
-If R decreases by 12% (all other things constant), W will
A) decrease by 72%.
B) decrease by 6%.
C) increase by 6%.
D) increase by 72%.
The computer output form the regression analysis is:
-If R decreases by 12% (all other things constant), W will
A) decrease by 72%.
B) decrease by 6%.
C) increase by 6%.
D) increase by 72%.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
29
Refer to the following computer output from estimating the parameters of the nonlinear model
-The estimated value of a is
A) -0.6931
B) 0.50
C) -3.67
D) 2.66
-The estimated value of a is
A) -0.6931
B) 0.50
C) -3.67
D) 2.66

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
30
In the nonlinear function , the parameter c measures
A) If Y / Z.
B) the percent change in Y for a 1 percent change in Z.
C) the elasticity of Y with respect to Z.
D) both a and c
E) both b and c
A) If Y / Z.
B) the percent change in Y for a 1 percent change in Z.
C) the elasticity of Y with respect to Z.
D) both a and c
E) both b and c

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
31
A firm is experiencing theft problems at its warehouse. A consultant to the firm believes that the dollar loss from theft each week (T) depends on the number of security guards (G) and on the unemployment rate in the county where the warehouse is located (U measured as a percent). In order to test this hypothesis, the consultant estimated the regression equation T = a + bG + cU and obtained the following results:
-Hiring one more guard per week will decrease the losses due to theft at the warehouse by _________ per week.
A) $5,150
B) $211
C) $130
D) $480.92

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
32
Refer to the following computer output from estimating the parameters of the nonlinear model
-Which of the parameter estimates are statistically significant at the 90% level of confidence?
A) All the parameter estimates are statistically significant.
B) All parameter estimates except and
are statistically significant.
C) is not statistically significant, but all the rest of the parameter estimates are significant.
D) is not statistically significant, but all the rest of the parameter estimates are significant.
-Which of the parameter estimates are statistically significant at the 90% level of confidence?
A) All the parameter estimates are statistically significant.
B) All parameter estimates except and
are statistically significant.
C) is not statistically significant, but all the rest of the parameter estimates are significant.
D) is not statistically significant, but all the rest of the parameter estimates are significant.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
33
Refer to the following nonlinear model which relates W to P, Q, and R:
The computer output form the regression analysis is:
-The nonlinear relation can be transformed into the following linear regression model:
A)
B)
C)
D)
The computer output form the regression analysis is:
-The nonlinear relation can be transformed into the following linear regression model:
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
34
A firm is experiencing theft problems at its warehouse. A consultant to the firm believes that the dollar loss from theft each week (T) depends on the number of security guards (G) and on the unemployment rate in the county where the warehouse is located (U measured as a percent). In order to test this hypothesis, the consultant estimated the regression equation T = a + bG + cU and obtained the following results:
-Aone percent increase in the level of unemployment in the county results in an increase in losses due to theft of __________ more losses per week.
A) $75
B) $211
C) $280
D) $460

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
35
Refer to the following computer output from estimating the parameters of the nonlinear model
-If R = 1, S = 2, and T = 3, what value do you expect Y will have?
A) 143
B) 1,345
C) 3,289
D) 6,578
E) -4,559
-If R = 1, S = 2, and T = 3, what value do you expect Y will have?
A) 143
B) 1,345
C) 3,289
D) 6,578
E) -4,559

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
36
Tests for statistical significance must be performed
A) because the TRUE values of the intercept and slope parameters are random variables.
B) because the ESTIMATED values of the intercept and slope parameters are not, in general, equal to the true values of the intercept and slope parameters.
C) because the computed t-ratios are random variables and may be too large to provide evidence that b is not equal to zero.
D) in order to determine whether or not the parameter estimates are far enough away from zero to conclude that the true parameter values are not equal to zero.
E) both b and d
A) because the TRUE values of the intercept and slope parameters are random variables.
B) because the ESTIMATED values of the intercept and slope parameters are not, in general, equal to the true values of the intercept and slope parameters.
C) because the computed t-ratios are random variables and may be too large to provide evidence that b is not equal to zero.
D) in order to determine whether or not the parameter estimates are far enough away from zero to conclude that the true parameter values are not equal to zero.
E) both b and d

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
37
Refer to the following nonlinear model which relates W to P, Q, and R:
The computer output form the regression analysis is:
-Which of the parameter estimates are statistically significant at the 5% level of significance?
A) All the parameter estimates are statistically significant.
B) All parameter estimates except and
are statistically significant.
C) is not statistically significant, but all the rest of the parameter estimates are significant.
D) is not statistically significant, but all the rest of the parameter estimates are significant.
The computer output form the regression analysis is:
-Which of the parameter estimates are statistically significant at the 5% level of significance?
A) All the parameter estimates are statistically significant.
B) All parameter estimates except and
are statistically significant.
C) is not statistically significant, but all the rest of the parameter estimates are significant.
D) is not statistically significant, but all the rest of the parameter estimates are significant.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
38
Refer to the following computer output from estimating the parameters of the nonlinear model
-If S increases by 8% (all other things constant), Y will
A) decrease by 3.52%.
B) decrease by 0.44%.
C) decrease by 4.4%.
D) increase by 0.44%.
E) decrease by 2.4%.
-If S increases by 8% (all other things constant), Y will
A) decrease by 3.52%.
B) decrease by 0.44%.
C) decrease by 4.4%.
D) increase by 0.44%.
E) decrease by 2.4%.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
39
Refer to the following nonlinear model which relates W to P, Q, and R:
The computer output form the regression analysis is:
-If P = 0.5, Q = 1.5, and R = 0.8, what value do you expect W will have?
A) 16,712
B) 243,200
C) 1,345
D) 3,289
The computer output form the regression analysis is:
-If P = 0.5, Q = 1.5, and R = 0.8, what value do you expect W will have?
A) 16,712
B) 243,200
C) 1,345
D) 3,289

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
40
Refer to the following computer output from estimating the parameters of the nonlinear model
-If R decreases by 10% (all other things constant), Y will
A) increase by 4.66%.
B) increase by 46.6%.
C) decrease by 4.66%.
D) decrease by 46.6%.
-If R decreases by 10% (all other things constant), Y will
A) increase by 4.66%.
B) increase by 46.6%.
C) decrease by 4.66%.
D) decrease by 46.6%.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
41
The linear regression equation G = a + bD is estimated using 24 observations on R and W. The least-squares estimate of b is -22.5, and the standard error of the estimate is 8.36. Perform a t-test for statistical significance of at the 1% level of significance.
a. There are _____ degrees of freedom for the t-test.
b. The value of the t-statistic is _________. The critical t-value for the test is _________.
c. The parameter estimate
_________ (is, is not) statistically significant at the 1% level.
a. There are _____ degrees of freedom for the t-test.
b. The value of the t-statistic is _________. The critical t-value for the test is _________.
c. The parameter estimate
_________ (is, is not) statistically significant at the 1% level.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
42
Refer to the following nonlinear model which relates W to P, Q, and R:
The computer output form the regression analysis is:
-If P = Q = R = 1, what value do you expect W will have?
A) 0
B) 1
C) 12.182
D) 2.50
The computer output form the regression analysis is:
-If P = Q = R = 1, what value do you expect W will have?
A) 0
B) 1
C) 12.182
D) 2.50

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
43
A simple linear regression equation relates G and D as follows:
G = a + bD
a. The explanatory variable is _______, and the dependent variable is ________.
b. The slope parameter is ______, and the intercept parameter _______.
c. When D is zero, G equals _______.
d. For each one-unit increase in D, the change in R is ______ units.
G = a + bD
a. The explanatory variable is _______, and the dependent variable is ________.
b. The slope parameter is ______, and the intercept parameter _______.
c. When D is zero, G equals _______.
d. For each one-unit increase in D, the change in R is ______ units.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
44
A manager wishes to estimate an average cost equation of the following form:
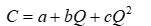
where Q is the level of output. Letting Z = Q2 and using least-squares estimation, the manager obtains the following computer output:
-When output is 40 units, what is average cost?
A) $200
B) $280
C) $360
D) $480
E) $520

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
45
In a multiple regression model, the coefficients on the independent variables measure
A) the percent of the variation in the dependent variable explained by a change in that independent variable, all other influences held constant.
B) the change in the dependent variable from a one-unit change in that independent variable, all other influences held constant.
C) the change in that independent variable from a one-unit change in the dependent variable, all other influences held constant.
D) the change in the dependent variable explained by the random error, all other influences held constant.
A) the percent of the variation in the dependent variable explained by a change in that independent variable, all other influences held constant.
B) the change in the dependent variable from a one-unit change in that independent variable, all other influences held constant.
C) the change in that independent variable from a one-unit change in the dependent variable, all other influences held constant.
D) the change in the dependent variable explained by the random error, all other influences held constant.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
46
The quadratic equation Y = a + bX +cX2 can be estimated using linear regression by estimating
A) Y = a + bX + ZX where Z = c2
B) Y = a + ZX where Z = (b + c)
C) Y = a + bZ where Z = X2
D) Y = a + ZX where Z = (b + c)2
E) none of the above will work
A) Y = a + bX + ZX where Z = c2
B) Y = a + ZX where Z = (b + c)
C) Y = a + bZ where Z = X2
D) Y = a + ZX where Z = (b + c)2
E) none of the above will work

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
47
A manager wishes to estimate an average cost equation of the following form:
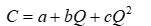
where Q is the level of output. Letting Z = Q2 and using least-squares estimation, the manager obtains the following computer output:
-The value of R2 indicates that _______ of the total variation in C is explained by the regression equation.
A) 0.7679%
B) 76.79%
C) 7.679%
D) 7679%

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
48
A manager wishes to estimate an average cost equation of the following form:
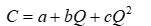
where Q is the level of output. Letting Z = Q2 and using least-squares estimation, the manager obtains the following computer output:
-Which of the parameter estimates are statistically significant at the 1% significance level?
A) All the parameter estimates are statistically significant.
B) All parameter estimates except are statistically significant.
C) is not statistically significant, but all the rest of the parameter estimates are significant.
D) is not statistically significant, but all the rest of the parameter estimates are significant.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
49
Refer to the following nonlinear model which relates W to P, Q, and R:
The computer output form the regression analysis is:
-The value of R2 tells us that
A) 0.9023% of the total variation in ln W is explained by the regression equation.
B) 90.23% of the total variation in ln W is explained by the regression equation.
C) 0.9023% of the total variation in P, W, and R is explained by the regression equation.
D) 0.9023% of the total variation in ln P, ln Q, and ln R is explained by the regression equation.
The computer output form the regression analysis is:
-The value of R2 tells us that
A) 0.9023% of the total variation in ln W is explained by the regression equation.
B) 90.23% of the total variation in ln W is explained by the regression equation.
C) 0.9023% of the total variation in P, W, and R is explained by the regression equation.
D) 0.9023% of the total variation in ln P, ln Q, and ln R is explained by the regression equation.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
50
A manager wishes to estimate an average cost equation of the following form:
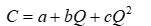
where Q is the level of output. Letting Z = Q2 and using least-squares estimation, the manager obtains the following computer output:
-When output is 20 units, what is average cost?
A) $160
B) $200
C) $280
D) $340
E) $360

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck
51
Refer to the following nonlinear model which relates W to P, Q, and R:
The computer output form the regression analysis is:
-If Q increases by 8% (all other things constant), W will
A) decrease by 99.2%.
B) decrease by 12.5%.
C) increase by 0.99%.
D) increase by 99.2%.
The computer output form the regression analysis is:
-If Q increases by 8% (all other things constant), W will
A) decrease by 99.2%.
B) decrease by 12.5%.
C) increase by 0.99%.
D) increase by 99.2%.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 51 في هذه المجموعة.
فتح الحزمة
k this deck








