Deck 15: Functions of Several Variables
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/137
العب
ملء الشاشة (f)
Deck 15: Functions of Several Variables
1
Find the volume of the tetrahedron with corners at ( 0, 0, 0 ), ( 3, 0, 0 ), ( 0, 10, 0 ) and ( 0, 0, 8 ).
A)40
B) 0
C) 80
D) 120
E) 240
A)40
B) 0
C) 80
D) 120
E) 240
40
2
Compute the integral.
A)-1,372
B) -2
C) -686
D) 172
E) -196
A)-1,372
B) -2
C) -686
D) 172
E) -196
-686
3
Compute the integral.
A)3,280.5
B) 9
C) 6,561
D) -40.5
E) 0
A)3,280.5
B) 9
C) 6,561
D) -40.5
E) 0
3,280.5
4
Compute the integral.
A)-55.5
B)
C)
D)
E)
A)-55.5
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
5
Compute the integral.
A)19
B)
C) 1
D) 7
E)
A)19
B)
C) 1
D) 7
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
6
Compute the integral.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
7
Write the integral with the order of integration reversed (changing the limits of integration as necessary).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
8
Compute the integral.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
9
Find the average value of the given function over the indicated domain. 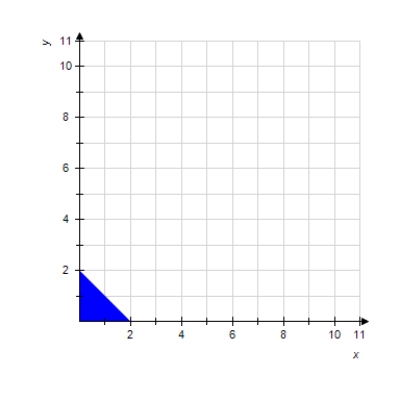
A)
B)
C)
D) 2
E)

A)
B)
C)
D) 2
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
10
Compute the integral.
A)60
B) 15
C) -52
D) 780
E) 117
A)60
B) 15
C) -52
D) 780
E) 117

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
11
Find , where and R is the indicated domain. (Remember that you often have a choice as to the order of integration.) 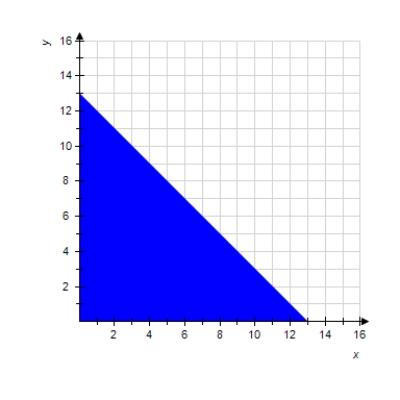
A)13
B) 2,197
C)
D) 113
E)

A)13
B) 2,197
C)
D) 113
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
12
Compute the integral.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
13
Your latest CD - ROM drive is expected to sell between and
Units if priced at p. You plan to set the price between $200 and $400. What are the maximum and minimum possible revenues you can make What is the average of all the possible revenues you can make
A)$36,800,000,000, $8,000,000,000, $140,166,667
B) $38,000,000, $16,000,000, $34,500,000
C) $36,800,000,000, $8,000,000,000, $73,500,000
D) $45,960,000, $79,840,000, $3,120,000
E) $92,000,000, $40,000,000, $774,000,000,000,000
Units if priced at p. You plan to set the price between $200 and $400. What are the maximum and minimum possible revenues you can make What is the average of all the possible revenues you can make
A)$36,800,000,000, $8,000,000,000, $140,166,667
B) $38,000,000, $16,000,000, $34,500,000
C) $36,800,000,000, $8,000,000,000, $73,500,000
D) $45,960,000, $79,840,000, $3,120,000
E) $92,000,000, $40,000,000, $774,000,000,000,000

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
14
The town of West Podunk is shaped like a rectangle 80 miles from west to east and 90 miles from north to south (see the figure). It has a population density of
hundred people per square mile x miles east and y miles north of the southwest corner of town. What is the total population of the town
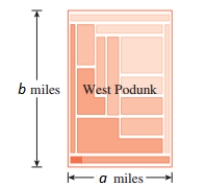
Please enter your answer as a number without the units.
hundred people per square mile x miles east and y miles north of the southwest corner of town. What is the total population of the town

Please enter your answer as a number without the units.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
15
Find the volume under the graph of over the triangle and .
A)15.75
B) 42
C) 22.5
D) 7.5
E) 29.25
A)15.75
B) 42
C) 22.5
D) 7.5
E) 29.25

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
16
Find , where and R is the indicated domain. (Remember that you often have a choice as to the order of integration.) 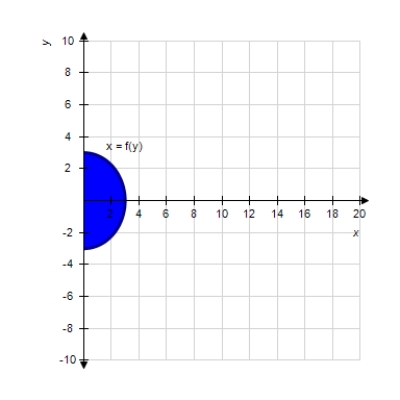
A)32.4
B) 24.3
C) 16.2
D) 27
E) 259.2

A)32.4
B) 24.3
C) 16.2
D) 27
E) 259.2

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
17
A productivity model at the Handy Gadget Company is
Where P is the number of gadgets the company turns out per month, x is the number of employees at the company, and y is the monthly operating budget in thousands of dollars. Because the company hires part - time workers, it uses anywhere between 44 and 54 workers each month, and its operating budget varies from $12,000 to $14,000 per month. What is the average of the possible numbers gadgets it can turn out per month (Round the answer to the nearest 1,000 gadgets.)
A)8,000 gadgets
B) 61,000 gadgets
C) 193,000 gadgets
D) 3,000 gadgets
Where P is the number of gadgets the company turns out per month, x is the number of employees at the company, and y is the monthly operating budget in thousands of dollars. Because the company hires part - time workers, it uses anywhere between 44 and 54 workers each month, and its operating budget varies from $12,000 to $14,000 per month. What is the average of the possible numbers gadgets it can turn out per month (Round the answer to the nearest 1,000 gadgets.)
A)8,000 gadgets
B) 61,000 gadgets
C) 193,000 gadgets
D) 3,000 gadgets

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
18
The temperature at the point on the square with vertices (0, 0), (0, 1), (1, 0) and (1, 1) is given by
Find the hottest and coldest points on the square.
A)(2, 2), (0, 0)
B) (0, 1), (0, 0)
C) (1, 1), (1, 0)
D) (1, 0), (0, 0)
E) (1, 1), (0, 0)
Find the hottest and coldest points on the square.
A)(2, 2), (0, 0)
B) (0, 1), (0, 0)
C) (1, 1), (1, 0)
D) (1, 0), (0, 0)
E) (1, 1), (0, 0)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
19
Find , where and R is the indicated domain. (Remember that you often have a choice as to the order of integration.)
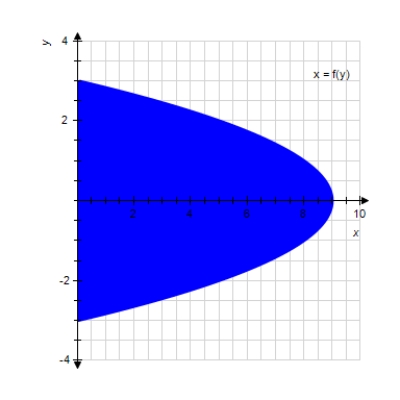
A)243
B) 729
C) 324
D) 972
E) 81

A)243
B) 729
C) 324
D) 972
E) 81

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
20
Find the average value of the given function over the indicated domain.
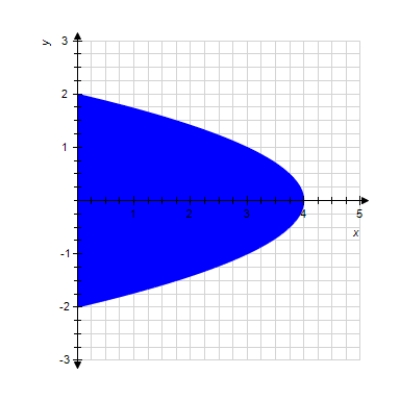
A)0
B) 2
C) 1.3
D) 10
E) 7

A)0
B) 2
C) 1.3
D) 10
E) 7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
21
Solve the given problem by using substitution.
Find the minimum value of subject to .
A)
B)
C)
D)
E)
Find the minimum value of subject to .
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
22
Use Lagrange Multipliers to solve the problem.
Find the maximum value of subject to .
A)
B)
C)
D)
E)
Find the maximum value of subject to .
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
23
Solve the given problem by using substitution.
Minimize subject to with , , .
A)
B)
C)
D)
E)
Minimize subject to with , , .
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
24
Solve the given problem by using substitution.
Find the minimum value of subject to .
A)
B)
C)
D)
E)
Find the minimum value of subject to .
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
25
What point on the surface z is closest to the origin
Hint : Minimize the square of the distance from to the origin.
A)
B) and
C)
D)
E) and
Hint : Minimize the square of the distance from to the origin.
A)
B) and
C)
D)
E) and

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
26
Classify the highlighted value in the table. 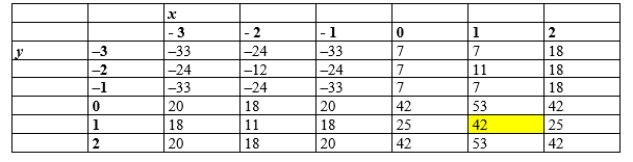
A)a relative maximum
B) a saddle point
C) a relative minimum
D) none of these

A)a relative maximum
B) a saddle point
C) a relative minimum
D) none of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
27
Solve the given problem by using substitution.
Find the maximum value of subject to .
A)
B)
C)
D)
E)
Find the maximum value of subject to .
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
28
Locate the critical point of the function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
29
Use Lagrange Multipliers to solve the problem.
Find the maximum value of subject to .
A)
B)
C)
D)
E)
Find the maximum value of subject to .
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
30
At what point on the given surface is the quantity a minimum (The method of Lagrange multipliers can be used here.)
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
31
Your latest CD - ROM drive is expected to sell between
and
units if priced at p. You plan to set the price between $200 and $300. What are the maximum and minimum possible revenues you can make What is the average of all the possible revenues you can make
Please enter your answer as three numbers, separated by commas.
and
units if priced at p. You plan to set the price between $200 and $300. What are the maximum and minimum possible revenues you can make What is the average of all the possible revenues you can make
Please enter your answer as three numbers, separated by commas.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
32
Find the point on the given plane closest to 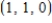 .
.
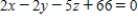
NOTE: Please enter your answer in the form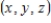 .
.
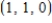 .
.
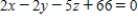
NOTE: Please enter your answer in the form
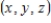 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
33
In the table below, classify each highlighted value. 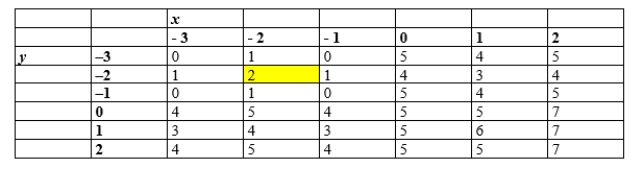
A)a saddle point
B) a relative maximum
C) a relative minimum
D) none of these

A)a saddle point
B) a relative maximum
C) a relative minimum
D) none of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
34
The temperature at the point 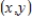 on the square with vertices (0, 0), (0, 2), (2, 0) and (2, 2) is given by
on the square with vertices (0, 0), (0, 2), (2, 0) and (2, 2) is given by 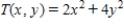
Find the hottest and coldest points on the square.
NOTE: Please enter your answers in the form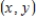 , separated by commas.
, separated by commas.
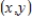 on the square with vertices (0, 0), (0, 2), (2, 0) and (2, 2) is given by
on the square with vertices (0, 0), (0, 2), (2, 0) and (2, 2) is given by 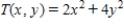
Find the hottest and coldest points on the square.
NOTE: Please enter your answers in the form
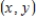 , separated by commas.
, separated by commas.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
35
Use Lagrange Multipliers to solve the given problem.
Find the maximum value of subject to .
A)
B)
C)
D)
E)
Find the maximum value of subject to .
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
36
Solve the given problem by using substitution.
Find the maximum value of subject to .
A)
B)
C)
D)
E)
Find the maximum value of subject to .
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
37
Solve the given problem by using substitution.
Minimize subject to with , , .
A)
B)
C)
D)
E)
Minimize subject to with , , .
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
38
Find the point on the given plane closest to .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
39
What point on the surface z is closest to the origin
Hint : Minimize the square of the distance from to the origin.
NOTE: Please enter your answer(s) in the form . If there is more than one answer, separate them with commas.
Hint : Minimize the square of the distance from to the origin.
NOTE: Please enter your answer(s) in the form . If there is more than one answer, separate them with commas.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
40
A productivity model at the Handy Gadget Company is
where P is the number of gadgets the company turns out per month, x is the number of employees at the company, and y is the monthly operating budget in thousands of dollars. Because the company hires part - time workers, it uses anywhere between 43 and 53 workers each month, and its operating budget varies from $13,000 to $17,000 per month. What is the average of the possible numbers gadgets it can turn out per month (Round the answer to the nearest 1,000 gadgets.)
Please enter your answer as a number without the units.
where P is the number of gadgets the company turns out per month, x is the number of employees at the company, and y is the monthly operating budget in thousands of dollars. Because the company hires part - time workers, it uses anywhere between 43 and 53 workers each month, and its operating budget varies from $13,000 to $17,000 per month. What is the average of the possible numbers gadgets it can turn out per month (Round the answer to the nearest 1,000 gadgets.)
Please enter your answer as a number without the units.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
41
Trans World Airlines (TWA) has a rule for checked baggage, "The total dimensions (length + width + height) may not exceed 57 inches for each bag". What is the volume of the largest volume bag you can check on a TWA flight
Enter your answer as a number without the units.
Enter your answer as a number without the units.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
42
The US Postal Service (USPS) will accept only packages with length plus girth no more than 120 inches. (See the figure.) 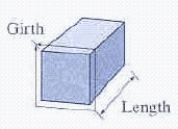
What are the dimensions of the largest volume package the USPS will accept
A) inches
B) inches
C) inches
D) inches
E) inches

What are the dimensions of the largest volume package the USPS will accept
A) inches
B) inches
C) inches
D) inches
E) inches

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
43
Locate the local minimum of the function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
44
Locate the local maximum of the function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
45
Find x and y values of the relative extrema of the function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
46
Trans World Airlines (TWA) has a rule for checked baggage, "The total dimensions (length + width + height) may not exceed 66 inches for each bag". What is the volume of the largest volume bag you can check on a TWA flight
A)10,648 in.3
B) 66 in.3
C) 287,496 in.3
D) 132 in.3
E) 22 in.3
A)10,648 in.3
B) 66 in.3
C) 287,496 in.3
D) 132 in.3
E) 22 in.3

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
47
Locate the maximum of the function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
48
How many critical points does the following function have
A)four
B) one
C) two
D) three
E) none
A)four
B) one
C) two
D) three
E) none

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
49
The cost of controlling emissions at a firm goes up rapidly as the amount of emissions reduced goes up. Here is a possible model.
Where x is the reduction in sulfur emissions, y is the reduction in lead emissions (in pounds of pollutant per day), and C is the daily cost to the firm (in dollars) of this reduction. Government clean-air subsidies amount to $600 per pound of sulfur and $50 per pound of lead removed. How many pounds of pollutant should the firm remove each day to minimize the net cost (cost minus subsidy)
A)3.5 lb of sulfur and 2 lb of lead
B) 6 lb of sulfur and 1 lb of lead
C) 3 lb of sulfur and 1 lb of lead
D) 1.9 lb of sulfur and 3.5 lb of lead
E) 1 lb of sulfur and 3 lb of lead
Where x is the reduction in sulfur emissions, y is the reduction in lead emissions (in pounds of pollutant per day), and C is the daily cost to the firm (in dollars) of this reduction. Government clean-air subsidies amount to $600 per pound of sulfur and $50 per pound of lead removed. How many pounds of pollutant should the firm remove each day to minimize the net cost (cost minus subsidy)
A)3.5 lb of sulfur and 2 lb of lead
B) 6 lb of sulfur and 1 lb of lead
C) 3 lb of sulfur and 1 lb of lead
D) 1.9 lb of sulfur and 3.5 lb of lead
E) 1 lb of sulfur and 3 lb of lead

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
50
Your company manufactures two models of stereo speakers, the Ultra Mini and the Big Stack. Demand for each depends partly on the price of the other. If one is expensive then more people will buy the other. If p1 is the price per pair of the Ultra Mini and p2 is the price of the Big Stack, demand for the Ultra Mini is given by
Where q1 represents the number of pairs of Ultra Minis that will be sold in a year. The demand for the Big Stack is given by
Find the prices for the Ultra Mini and the Big Stack that will maximize your total revenue.
Round your answer to the nearest dollar.
A)$798 for Ultra Mini and $275 for Big Stack
B) $428 for Ultra Mini and $783 for Big Stack
C) $275 for Ultra Mini and $798 for Big Stack
D) $328 for Ultra Mini and $783 for Big Stack
E) $783 for Ultra Mini and $328 for Big Stack
Where q1 represents the number of pairs of Ultra Minis that will be sold in a year. The demand for the Big Stack is given by
Find the prices for the Ultra Mini and the Big Stack that will maximize your total revenue.
Round your answer to the nearest dollar.
A)$798 for Ultra Mini and $275 for Big Stack
B) $428 for Ultra Mini and $783 for Big Stack
C) $275 for Ultra Mini and $798 for Big Stack
D) $328 for Ultra Mini and $783 for Big Stack
E) $783 for Ultra Mini and $328 for Big Stack

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
51
Locate the saddle point of the function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
52
Your company manufactures two models of stereo speakers, the Ultra Mini and the Big Stack. Demand for each depends partly on the price of the other. If one is expensive then more people will buy the other. If p1 is the price per pair of the Ultra Mini and p2 is the price of the Big Stack, demand for the Ultra Mini is given by
Where q1 represents the number of pairs of Ultra Minis that will be sold in a year. The demand for the Big Stack is given by
Find the prices for the Ultra Mini and the Big Stack that will maximize your total revenue.
Round your answer to the nearest dollar.
A)$874 for Ultra Mini and $1,033 for Big Stack
B) $1,033 for Ultra Mini and $874 for Big Stack
C) $1,186 for Ultra Mini and $859 for Big Stack
D) $1,086 for Ultra Mini and $859 for Big Stack
E) $859 for Ultra Mini and $1,086 for Big Stack
Where q1 represents the number of pairs of Ultra Minis that will be sold in a year. The demand for the Big Stack is given by
Find the prices for the Ultra Mini and the Big Stack that will maximize your total revenue.
Round your answer to the nearest dollar.
A)$874 for Ultra Mini and $1,033 for Big Stack
B) $1,033 for Ultra Mini and $874 for Big Stack
C) $1,186 for Ultra Mini and $859 for Big Stack
D) $1,086 for Ultra Mini and $859 for Big Stack
E) $859 for Ultra Mini and $1,086 for Big Stack

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
53
Locate the maximum of the function.
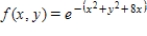
Express your answer as an ordered triple. If there is more than one answer, separate them by commas.
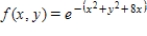
Express your answer as an ordered triple. If there is more than one answer, separate them by commas.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
54
The US Postal Service (USPS) will accept only packages with length plus girth no more than 102 inches. (See the figure.)
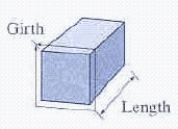
What are the dimensions of the largest volume package the USPS will accept
Express your answer as an ordered triple in the form .

What are the dimensions of the largest volume package the USPS will accept
Express your answer as an ordered triple in the form .

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
55
Locate the local minimum of the function.
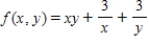
Express your answer as an ordered triple.
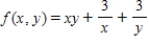
Express your answer as an ordered triple.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
56
Let
What condition on H guarantees that f has a relative extremum at the point
What condition on H guarantees that f has a relative extremum at the point

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
57
Locate the critical point of the function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
58
Locate the local maximum of the function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
59
How many critical points does the function have
A)three
B) two
C) four
D) one
E) none
A)three
B) two
C) four
D) one
E) none

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
60
The cost of controlling emissions at a firm goes up rapidly as the amount of emissions reduced goes up. Here is a possible model.
where x is the reduction in sulfur emissions, y is the reduction in lead emissions (in pounds of pollutant per day), and C is the daily cost to the firm (in dollars) of this reduction. Government clean-air subsidies amount to $300 per pound of sulfur and $50 per pound of lead removed. How many pounds of pollutant should the firm remove each day to minimize the net cost (cost minus subsidy)
Enter your answer as an ordered pair in the form . Round your answer to three decimal places if necessary.
where x is the reduction in sulfur emissions, y is the reduction in lead emissions (in pounds of pollutant per day), and C is the daily cost to the firm (in dollars) of this reduction. Government clean-air subsidies amount to $300 per pound of sulfur and $50 per pound of lead removed. How many pounds of pollutant should the firm remove each day to minimize the net cost (cost minus subsidy)
Enter your answer as an ordered pair in the form . Round your answer to three decimal places if necessary.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
61
Classify each labeled point on the graph.
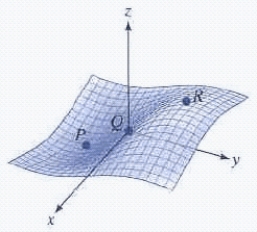
Choose the correct letter for each question.
a relative minimum
A)R
B)P
C)Q

Choose the correct letter for each question.
a relative minimum
A)R
B)P
C)Q

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
62
Calculate , and evaluate each at .
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
63
In the table below, classify each highlighted value.
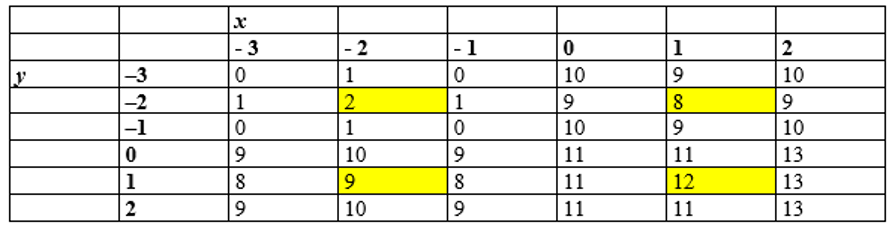
-relative maximum
A)2
B)8
C)9
D)12

-relative maximum
A)2
B)8
C)9
D)12

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
64
Classify each labeled point on the graph. 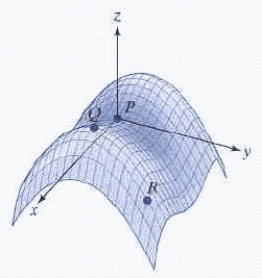 Choose the correct letter for each question.
Choose the correct letter for each question.
none
A)Q
B)P
C)R
 Choose the correct letter for each question.
Choose the correct letter for each question.none
A)Q
B)P
C)R

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
65
Calculate , and when defined.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
66
In the table below, classify each highlighted value.
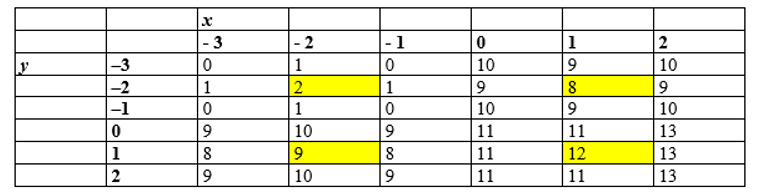
-none
A)2
B)8
C)9
D)12

-none
A)2
B)8
C)9
D)12

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
67
Classify each labeled point on the graph.  Choose the correct letter for each question.
Choose the correct letter for each question.
local maximum
A)Q
B)P
C)R
 Choose the correct letter for each question.
Choose the correct letter for each question.local maximum
A)Q
B)P
C)R

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
68
In the table below, classify each highlighted value.

-relative minimum
A)2
B)8
C)9
D)12

-relative minimum
A)2
B)8
C)9
D)12

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
69
Calculate , and when defined.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
70
Calculate , and when defined.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
71
Calculate and when defined.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
72
Classify each labeled point on the graph.

Choose the correct letter for each question.
a relative maximum
A)R
B)P
C)Q

Choose the correct letter for each question.
a relative maximum
A)R
B)P
C)Q

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
73
In the table below, classify each highlighted value.

-saddle point
A)2
B)8
C)9
D)12

-saddle point
A)2
B)8
C)9
D)12

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
74
Classify each labeled point on the graph.

Choose the correct letter for each question.
none
A)R
B)P
C)Q

Choose the correct letter for each question.
none
A)R
B)P
C)Q

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
75
Calculate , and when defined.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
76
Calculate the values of , , and at .
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
77
Calculate , and when defined.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
78
Calculate , when defined.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
79
Classify each labeled point on the graph.  Choose the correct letter for each question.
Choose the correct letter for each question.
saddle point
A)Q
B)P
C)R
 Choose the correct letter for each question.
Choose the correct letter for each question.saddle point
A)Q
B)P
C)R

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck
80
Calculate , and when defined.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 137 في هذه المجموعة.
فتح الحزمة
k this deck








