Deck 4: Elementary Number Theory and Methods of Proof
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/28
العب
ملء الشاشة (f)
Deck 4: Elementary Number Theory and Methods of Proof
1
Prove the following statement: For all real numbers x, 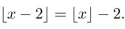
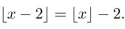

2
Prove the following statement: There is no smallest positive rational number.
3
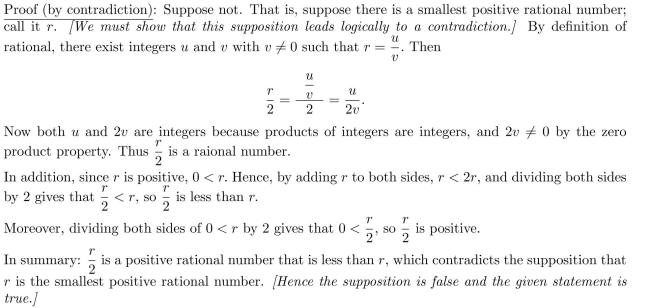
Prove the following statement by contradiction: For all real numbers r and s, if r is rational
and s is irrational, then r + 2s is irrational.
and s is irrational, then r + 2s is irrational.
4
Outline a proof of the following statement by writing the "starting point" and the "conclusion
to be shown" in a proof of the statement.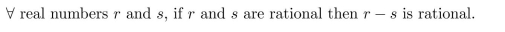
That is, complete the sentences below.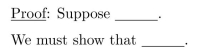
to be shown" in a proof of the statement.
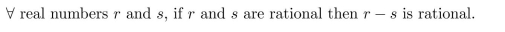
That is, complete the sentences below.
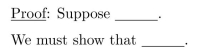

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
5
Is 605.83 a rational number? Justify your answer.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
6
Is 56.745 a rational number? Justify your answer.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
7
Consider the following statement: 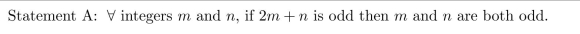
(a) Write a negation for Statement A.
(b) Disprove Statement A. That is, show that Statement A is false.
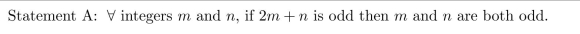
(a) Write a negation for Statement A.
(b) Disprove Statement A. That is, show that Statement A is false.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
8
Find a counterexample to show that the following statement is false: 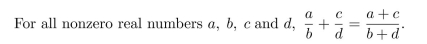
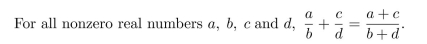

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
9
Prove the following statement: The sum of any two consecutive integers can be written in the
form 4n + 1 for some integer n.
form 4n + 1 for some integer n.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
10
Prove the statement below directly from the definitions of the terms. Do not use any other
facts previously proved in class or in the text or in the exercises.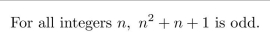
facts previously proved in class or in the text or in the exercises.
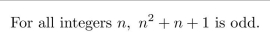

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
11
Show that the following statement is false: The product of any two irrational numbers is
irrational.
irrational.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
12
Is 0 divisible by 3? Justify your answer.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
13
State precisely (but concisely) what it means for an integer n to be odd.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
14
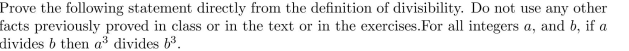

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
15
State precisely (but concisely) what it means for a number r to be rational.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
16
State precisely (but concisely) what it means for an integer n to be divisible by an integerd.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
17
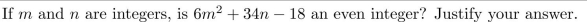

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
18
Prove the following statement directly from the definitions of the terms. Do not use any other
facts previously proved in class or in the text or in the exercises.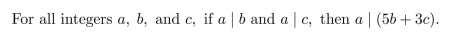
facts previously proved in class or in the text or in the exercises.
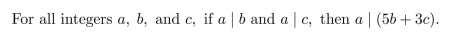

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
19
Does 12 divide 72? Justify your answer.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
20
Prove the following statement directly from the definitions of the terms. Do not use any other
facts previously proved in class or in the text or in the exercises.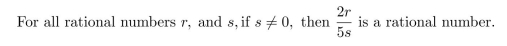
facts previously proved in class or in the text or in the exercises.
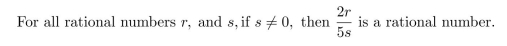

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
21
Consider the following statement: For all integers n, if n3 is odd then n is odd.
(a) Prove the statement either by contradiction or by contraposition. Clearly indicate which
method you are using.
(b) If you used proof by contradiction in part (a), write what you would "suppose" and
what you would "show" to prove the statement by contraposition. If you used proof by
contraposition in part (a), write what you would "suppose" and what you would "show"
to prove the statement by contradiction.
(a) Prove the statement either by contradiction or by contraposition. Clearly indicate which
method you are using.
(b) If you used proof by contradiction in part (a), write what you would "suppose" and
what you would "show" to prove the statement by contraposition. If you used proof by
contraposition in part (a), write what you would "suppose" and what you would "show"
to prove the statement by contradiction.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
22
True or false? For any irrational number r, r2 is irrational. Justify your answer.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
23
Consider the following statement: For all real numbers r, if r3 is irrational then r is irrational.
(a) Prove the statement either by contradiction or by contraposition. Clearly indicate which
method you are using.
(b) If you used proof by contradiction in part (a), write what you would "suppose" and
what you would "show" to prove the statement by contraposition. If you used proof by
contraposition in part (a), write what you would "suppose" and what you would "show"
to prove the statement by contradiction.
(a) Prove the statement either by contradiction or by contraposition. Clearly indicate which
method you are using.
(b) If you used proof by contradiction in part (a), write what you would "suppose" and
what you would "show" to prove the statement by contraposition. If you used proof by
contraposition in part (a), write what you would "suppose" and what you would "show"
to prove the statement by contradiction.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
24
Prove by contradiction that 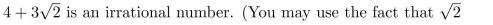
is irrational.)
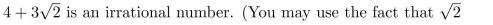
is irrational.)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
25
Use the Euclidean algorithm to find the greatest common divisor of 284 and 168. Show your
work.
work.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
26


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
27
Consider the following statement: For all integers n, if n3 is even then n is even.
(a) Prove the statement either by contradiction or by contraposition. Clearly indicate which
method you are using.
(b) If you used proof by contradiction in part (a), write what you would "suppose" and
what you would "show" to prove the statement by contraposition. If you used proof by
contraposition. in part (a), write what you would "suppose" and what you would "show"
to prove the statement by contradiction.
(a) Prove the statement either by contradiction or by contraposition. Clearly indicate which
method you are using.
(b) If you used proof by contradiction in part (a), write what you would "suppose" and
what you would "show" to prove the statement by contraposition. If you used proof by
contraposition. in part (a), write what you would "suppose" and what you would "show"
to prove the statement by contradiction.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck
28
Use the Euclidean algorithm to calculate the greatest common divisor of 10,673 and 11,284.
Show your work.
Show your work.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 28 في هذه المجموعة.
فتح الحزمة
k this deck








