تكلم مع الذكاء الاصطناعي
Deck 4: Uncertainty
ملء الشاشة (f)
سؤال
سؤال
Explain why the slope of the market line in Figures 4.4 and 4.5 shows how risk is "priced" in this market. How might the data in Application 4.4: Puts, Calls, and Black-Scholes be plotted to determine this slope? 
سؤال
سؤال
Let's examine Figure 4A.3 more closely.
1. Why do choices along the "certainty line" imply that there is no risk?
2. If the probability of state 1 is 0.6 and the probability of state 2 is 0.4, what is the actuarially fair slope for the line AE?
3. In general, what determines the slope of the indifference curve EU 3 ?
4. Given your answer to part 2, can you explain why AE and EU 3 have the same slope at point E? (This question is relatively hard.)
1. Why do choices along the "certainty line" imply that there is no risk?
2. If the probability of state 1 is 0.6 and the probability of state 2 is 0.4, what is the actuarially fair slope for the line AE?
3. In general, what determines the slope of the indifference curve EU 3 ?
4. Given your answer to part 2, can you explain why AE and EU 3 have the same slope at point E? (This question is relatively hard.)
سؤال
سؤال
Without looking at Figure 4A.3, see if you can draw your own diagram to illustrate the benefits of, say, health insurance.
2. Use a separate diagram to analyze whether a risk-neutral person would ever want to purchase health insurance and under what conditions if so.
2. Use a separate diagram to analyze whether a risk-neutral person would ever want to purchase health insurance and under what conditions if so.
سؤال
سؤال
سؤال
"Risk-averse people should only be averse to big gambles with a lot of money at stake. They should jump on any small gamble that is unfair in their favor." Explain why this statement makes sense. Use a utility-of-income graph like Figure 4.1 to illustrate the statement. 
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
Puts, Calls, and Black-Scholes
Options on financial assets are widely traded in organized markets. Not only are there options available on most company's stocks, but there are also a bewildering variety of options on such assets as bonds, foreign exchange, and commodities, or even on indexes based on groups of these assets. Probably the most common options are those related to the stock of a single company. The potential transactions underlying these options are simply promises to buy or sell the stock at a specific ("strike") price over some period in the future. Options to buy a stock at a certain strike price are termed "call" options because the buyer has the right to "call" the stock from someone else if he or she wishes to exercise the option. Options to sell a stock at a certain price are called "put" options (perhaps because you have the option to put the stock into someone else's hands).
Suppose that Microsoft stock is currently selling at $30 per share. A call option might give you the right (but, again, not the obligation) to buy Microsoft in one month at, say, $32 per share.1 Suppose you also believe there is a 50-50 chance that Microsoft will sell for either $35 or $25 in one month's time. Clearly the option to buy at $32 is valuable-the stock might end up at $35. But how much is this option worth?
An Equivalent Portfolio
One way that financial economists evaluate options is by asking whether there is another set of assets that would yield the same outcomes as would the option. If such a set exists, one can then argue that it should have the same price as the option because markets will ensure that the same good always has the same price. So, let's consider the outcomes of the Microsoft option. If Microsoft sells for $25 in a month's time, the option is worthless-why pay $32 when the stock can readily be bought for $25? If Microsoft sells for $35, however, the option will be worth $3. Could we duplicate these two payouts with some other set of assets? Suppose we borrow some funds (L) from a bank (with no interest, to make things simple) and buy a fraction (k) of a Microsoft share. After a month, we will sell the fractional share of Microsoft and pay off the loan. In this example, L and k must be chosen to yield the same outcomes as the option. That is,
These two equations can easily be solved as k = 0.3, L = 7.5. That is, buying 0.3 of a Microsoft share and taking a loan of $7.50 will yield the same outcomes as buying the option. The net cost of this strategy is $1.50?$9 to buy 0.3 of a Microsoft share at $30 less the loan of $7.50 (which in our simple case carries no interest). Hence, this also is the value of the option.
The Black-Scholes Theorem
Of course, valuing options in the real world is much more complicated than this simple example suggests. Three specific complications that need to be addressed in developing a more general theory of valuation are as follows: (1) there are far more possibilities for Microsoft stock's price in one month than just the two we assumed; (2) most popular options can be exercised at any time during a specified period, not just on a specific date; and (3) interest rates matter for any economic transaction that occurs over time. Taking account of these factors proved to be very difficult, and it was not until 1973 that Fischer Black and Myron Scholes developed an acceptable valuation model.2 Since that time, the Black-Scholes model has been widely applied to options and other markets. In one of its more innovative applications, the model is now used in reverse to calculate an "implied volatility" expected for stocks in the future. The Chicago Board Options Exchange Volatility Index (VIX) is widely followed in the financial press, where it is taken as a good measure of the current uncertainties involved in stock market investing.
For every buyer of, say, a call option, there must of course also be a seller. Why would someone sell a call option on some shares he or she already owned? How would this be different than buying a put option on this stock?
Options on financial assets are widely traded in organized markets. Not only are there options available on most company's stocks, but there are also a bewildering variety of options on such assets as bonds, foreign exchange, and commodities, or even on indexes based on groups of these assets. Probably the most common options are those related to the stock of a single company. The potential transactions underlying these options are simply promises to buy or sell the stock at a specific ("strike") price over some period in the future. Options to buy a stock at a certain strike price are termed "call" options because the buyer has the right to "call" the stock from someone else if he or she wishes to exercise the option. Options to sell a stock at a certain price are called "put" options (perhaps because you have the option to put the stock into someone else's hands).
Suppose that Microsoft stock is currently selling at $30 per share. A call option might give you the right (but, again, not the obligation) to buy Microsoft in one month at, say, $32 per share.1 Suppose you also believe there is a 50-50 chance that Microsoft will sell for either $35 or $25 in one month's time. Clearly the option to buy at $32 is valuable-the stock might end up at $35. But how much is this option worth?
An Equivalent Portfolio
One way that financial economists evaluate options is by asking whether there is another set of assets that would yield the same outcomes as would the option. If such a set exists, one can then argue that it should have the same price as the option because markets will ensure that the same good always has the same price. So, let's consider the outcomes of the Microsoft option. If Microsoft sells for $25 in a month's time, the option is worthless-why pay $32 when the stock can readily be bought for $25? If Microsoft sells for $35, however, the option will be worth $3. Could we duplicate these two payouts with some other set of assets? Suppose we borrow some funds (L) from a bank (with no interest, to make things simple) and buy a fraction (k) of a Microsoft share. After a month, we will sell the fractional share of Microsoft and pay off the loan. In this example, L and k must be chosen to yield the same outcomes as the option. That is,
These two equations can easily be solved as k = 0.3, L = 7.5. That is, buying 0.3 of a Microsoft share and taking a loan of $7.50 will yield the same outcomes as buying the option. The net cost of this strategy is $1.50?$9 to buy 0.3 of a Microsoft share at $30 less the loan of $7.50 (which in our simple case carries no interest). Hence, this also is the value of the option.
The Black-Scholes Theorem
Of course, valuing options in the real world is much more complicated than this simple example suggests. Three specific complications that need to be addressed in developing a more general theory of valuation are as follows: (1) there are far more possibilities for Microsoft stock's price in one month than just the two we assumed; (2) most popular options can be exercised at any time during a specified period, not just on a specific date; and (3) interest rates matter for any economic transaction that occurs over time. Taking account of these factors proved to be very difficult, and it was not until 1973 that Fischer Black and Myron Scholes developed an acceptable valuation model.2 Since that time, the Black-Scholes model has been widely applied to options and other markets. In one of its more innovative applications, the model is now used in reverse to calculate an "implied volatility" expected for stocks in the future. The Chicago Board Options Exchange Volatility Index (VIX) is widely followed in the financial press, where it is taken as a good measure of the current uncertainties involved in stock market investing.
For every buyer of, say, a call option, there must of course also be a seller. Why would someone sell a call option on some shares he or she already owned? How would this be different than buying a put option on this stock?
سؤال
Wen, who has current wealth of $10,000, decides to take advantage of a free trip to Las Vegas to play roulette. His utility function over the wealth he ends up with after the trip Y (which equals his current wealth adjusted by any amount he ends up winning or losing) is 
He can take one of two gambles at the roulette table:
Gamble 1: Wager $1,000 on red. If red comes up, which happens with probability 18/38, he wins $1,000; otherwise he loses his $1,000 wager.
Gamble 2: Wager $500 on the single number 00. If 00 comes up, which happens with probability 1/38, he wins 35 times his wager ($17,500); otherwise he loses his $500 wager.
a. Are these fair gambles?
b. If Wen is forced to take one of the two gambles, which would he prefer?
c. If Wen isn't forced to play roulette, would he take a gamble?
He can take one of two gambles at the roulette table: Gamble 1: Wager $1,000 on red. If red comes up, which happens with probability 18/38, he wins $1,000; otherwise he loses his $1,000 wager.
Gamble 2: Wager $500 on the single number 00. If 00 comes up, which happens with probability 1/38, he wins 35 times his wager ($17,500); otherwise he loses his $500 wager.
a. Are these fair gambles?
b. If Wen is forced to take one of the two gambles, which would he prefer?
c. If Wen isn't forced to play roulette, would he take a gamble?
سؤال
سؤال
Puts, Calls, and Black-Scholes
Options on financial assets are widely traded in organized markets. Not only are there options available on most company's stocks, but there are also a bewildering variety of options on such assets as bonds, foreign exchange, and commodities, or even on indexes based on groups of these assets. Probably the most common options are those related to the stock of a single company. The potential transactions underlying these options are simply promises to buy or sell the stock at a specific ("strike") price over some period in the future. Options to buy a stock at a certain strike price are termed "call" options because the buyer has the right to "call" the stock from someone else if he or she wishes to exercise the option. Options to sell a stock at a certain price are called "put" options (perhaps because you have the option to put the stock into someone else's hands).
Suppose that Microsoft stock is currently selling at $30 per share. A call option might give you the right (but, again, not the obligation) to buy Microsoft in one month at, say, $32 per share.1 Suppose you also believe there is a 50-50 chance that Microsoft will sell for either $35 or $25 in one month's time. Clearly the option to buy at $32 is valuable-the stock might end up at $35. But how much is this option worth?
An Equivalent Portfolio
One way that financial economists evaluate options is by asking whether there is another set of assets that would yield the same outcomes as would the option. If such a set exists, one can then argue that it should have the same price as the option because markets will ensure that the same good always has the same price. So, let's consider the outcomes of the Microsoft option. If Microsoft sells for $25 in a month's time, the option is worthless-why pay $32 when the stock can readily be bought for $25? If Microsoft sells for $35, however, the option will be worth $3. Could we duplicate these two payouts with some other set of assets? Suppose we borrow some funds (L) from a bank (with no interest, to make things simple) and buy a fraction (k) of a Microsoft share. After a month, we will sell the fractional share of Microsoft and pay off the loan. In this example, L and k must be chosen to yield the same outcomes as the option. That is,
These two equations can easily be solved as k = 0.3, L = 7.5. That is, buying 0.3 of a Microsoft share and taking a loan of $7.50 will yield the same outcomes as buying the option. The net cost of this strategy is $1.50?$9 to buy 0.3 of a Microsoft share at $30 less the loan of $7.50 (which in our simple case carries no interest). Hence, this also is the value of the option.
The Black-Scholes Theorem
Of course, valuing options in the real world is much more complicated than this simple example suggests. Three specific complications that need to be addressed in developing a more general theory of valuation are as follows: (1) there are far more possibilities for Microsoft stock's price in one month than just the two we assumed; (2) most popular options can be exercised at any time during a specified period, not just on a specific date; and (3) interest rates matter for any economic transaction that occurs over time. Taking account of these factors proved to be very difficult, and it was not until 1973 that Fischer Black and Myron Scholes developed an acceptable valuation model.2 Since that time, the Black-Scholes model has been widely applied to options and other markets. In one of its more innovative applications, the model is now used in reverse to calculate an "implied volatility" expected for stocks in the future. The Chicago Board Options Exchange Volatility Index (VIX) is widely followed in the financial press, where it is taken as a good measure of the current uncertainties involved in stock market investing.
The Black-Scholes model assumes that stock returns are random and that they follow a bell-shaped (normal) distribution. Does this seem a reasonable assumption?
Options on financial assets are widely traded in organized markets. Not only are there options available on most company's stocks, but there are also a bewildering variety of options on such assets as bonds, foreign exchange, and commodities, or even on indexes based on groups of these assets. Probably the most common options are those related to the stock of a single company. The potential transactions underlying these options are simply promises to buy or sell the stock at a specific ("strike") price over some period in the future. Options to buy a stock at a certain strike price are termed "call" options because the buyer has the right to "call" the stock from someone else if he or she wishes to exercise the option. Options to sell a stock at a certain price are called "put" options (perhaps because you have the option to put the stock into someone else's hands).
Suppose that Microsoft stock is currently selling at $30 per share. A call option might give you the right (but, again, not the obligation) to buy Microsoft in one month at, say, $32 per share.1 Suppose you also believe there is a 50-50 chance that Microsoft will sell for either $35 or $25 in one month's time. Clearly the option to buy at $32 is valuable-the stock might end up at $35. But how much is this option worth?
An Equivalent Portfolio
One way that financial economists evaluate options is by asking whether there is another set of assets that would yield the same outcomes as would the option. If such a set exists, one can then argue that it should have the same price as the option because markets will ensure that the same good always has the same price. So, let's consider the outcomes of the Microsoft option. If Microsoft sells for $25 in a month's time, the option is worthless-why pay $32 when the stock can readily be bought for $25? If Microsoft sells for $35, however, the option will be worth $3. Could we duplicate these two payouts with some other set of assets? Suppose we borrow some funds (L) from a bank (with no interest, to make things simple) and buy a fraction (k) of a Microsoft share. After a month, we will sell the fractional share of Microsoft and pay off the loan. In this example, L and k must be chosen to yield the same outcomes as the option. That is,
These two equations can easily be solved as k = 0.3, L = 7.5. That is, buying 0.3 of a Microsoft share and taking a loan of $7.50 will yield the same outcomes as buying the option. The net cost of this strategy is $1.50?$9 to buy 0.3 of a Microsoft share at $30 less the loan of $7.50 (which in our simple case carries no interest). Hence, this also is the value of the option.
The Black-Scholes Theorem
Of course, valuing options in the real world is much more complicated than this simple example suggests. Three specific complications that need to be addressed in developing a more general theory of valuation are as follows: (1) there are far more possibilities for Microsoft stock's price in one month than just the two we assumed; (2) most popular options can be exercised at any time during a specified period, not just on a specific date; and (3) interest rates matter for any economic transaction that occurs over time. Taking account of these factors proved to be very difficult, and it was not until 1973 that Fischer Black and Myron Scholes developed an acceptable valuation model.2 Since that time, the Black-Scholes model has been widely applied to options and other markets. In one of its more innovative applications, the model is now used in reverse to calculate an "implied volatility" expected for stocks in the future. The Chicago Board Options Exchange Volatility Index (VIX) is widely followed in the financial press, where it is taken as a good measure of the current uncertainties involved in stock market investing.
The Black-Scholes model assumes that stock returns are random and that they follow a bell-shaped (normal) distribution. Does this seem a reasonable assumption?
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
Suppose a person must accept one of three bets:
Bet 1: Win $100 with probability V2; lose $100with probability 1/2.
Bet 2: Win $100 with probability 3/i; lose $300 with probability 1=4.
Bet 3: Win $100 with probability 9 10; lose $900with probability 1 10.
a. Show that all of these are fair bets.
b. Graph each bet on a utility of income curve similar to Figure.
FIGURE Risk Aversion
An individual characterized by the utility-of-income curve U will obtain a higher utility (U3) from a risk-free income of $35,000 than from a 50-50 chance of winning or losing $5,000 (U2). He or she will be willing to pay up to $2,000 to avoid having to take this bet. A fair bet of $15,000 provides even less utility (U1) than the $5,000 bet.
c. Explain carefully which bet will be preferred and why.
Bet 1: Win $100 with probability V2; lose $100with probability 1/2.
Bet 2: Win $100 with probability 3/i; lose $300 with probability 1=4.
Bet 3: Win $100 with probability 9 10; lose $900with probability 1 10.
a. Show that all of these are fair bets.
b. Graph each bet on a utility of income curve similar to Figure.
FIGURE Risk Aversion
An individual characterized by the utility-of-income curve U will obtain a higher utility (U3) from a risk-free income of $35,000 than from a 50-50 chance of winning or losing $5,000 (U2). He or she will be willing to pay up to $2,000 to avoid having to take this bet. A fair bet of $15,000 provides even less utility (U1) than the $5,000 bet.
c. Explain carefully which bet will be preferred and why.
سؤال
سؤال
The Equity Premium Puzzle
As shown in Figure 4.4, differences in the rates of return of financial assets reflect, in part, the differing risks associated with those assets. The historical data show that stocks also called equities have indeed had higher returns than bonds to compensate for that risk. In fact, returns on common stock have been so favorable that they pose a puzzle to economists.
Historical Rates of Return
Table 1 cites some of the most widely used rate of return data for U.S. financial markets, compiled by Ibbotson Associates. These data show that over the period 1926-2012, common stocks of large companies provided average annual rates of return which exceeded those on long-term bonds by 5 percent per year, whether the bonds of corporations or the government are considered. The equity premium is even larger if the set of common stocks is expanded to include small companies, the average return on which was a whopping 16.5 percent over the period. The equity premium is larger still if rather than long term, we look at short-term government bonds: the average return on one-year treasury bills was a measly 3.1 percent over the period, essentially no better than inflation.
One way to measure the risk associated with various assets uses the "standard deviation" of their annual returns. This measure shows the range in which roughly two-thirds of the returns fall. For the case of, say, common stocks, the average annual return was 11.8 percent, and the standard deviation shows that in two-thirds of the years the average was within± 20.2 percent of this figure. In other words, in two-thirds of the years, common stocks returned more than -8.4 percent and less than ±32.0 percent. Rates of return on stocks were much more variable than those on bonds.
The Excess Return on Common Stocks
Although the qualitative findings from data such as those in Table 1 are consistent with risk aversion, the quantitative nature of the extra returns to common stock holding are inconsistent with many other studies of risk. These other studies suggest that individuals would accept the extra risk that stocks carry for an extra return of around 1 percent per year- significantly less than the 5 percent extra actually provided.
One set of explanations focuses on the possibility that the figures in Table 1 understate the risk of stocks. The risk individuals really care about is changes in their consumption plans. If returns on stocks were highly correlated with the business cycle, then they might pose extra risks because individuals would face a double risk from economic downturns-a fall in
income and a fall in returns from investments. Behavioral economists have offered another explanation: individuals may experience extra psychological pain from losing money on investments in any given period beyond any consequences for their ultimate wealth. (For more on this type of behavioral bias, see the section entitled "Prospect Theory" in Chapter 17.) A recent explanation of the equity premium hinges on catastrophes (wars, riots, depressions, and so on) that have the potential of wiping out much of the value of stocks but so rare that they are seldom experienced, if at all, even in a long period spanning decades. The United States may have experienced an unusually tranquil period during 1926-2102 compared to other periods or countries; subtracting catastrophic losses that might have occurred in a less tranquil period would bring down the average for the return on stocks. Work remains to convince the economics profession that any of these explanations can account for the large equity premium on its own.
Holding stocks in individual companies probably involves greater risks than are reflected in the data for all stocks in Table 1. Do you think these extra risks are relevant to appraising the extra rate of return that stocks provide?
As shown in Figure 4.4, differences in the rates of return of financial assets reflect, in part, the differing risks associated with those assets. The historical data show that stocks also called equities have indeed had higher returns than bonds to compensate for that risk. In fact, returns on common stock have been so favorable that they pose a puzzle to economists.
Historical Rates of Return
Table 1 cites some of the most widely used rate of return data for U.S. financial markets, compiled by Ibbotson Associates. These data show that over the period 1926-2012, common stocks of large companies provided average annual rates of return which exceeded those on long-term bonds by 5 percent per year, whether the bonds of corporations or the government are considered. The equity premium is even larger if the set of common stocks is expanded to include small companies, the average return on which was a whopping 16.5 percent over the period. The equity premium is larger still if rather than long term, we look at short-term government bonds: the average return on one-year treasury bills was a measly 3.1 percent over the period, essentially no better than inflation.
One way to measure the risk associated with various assets uses the "standard deviation" of their annual returns. This measure shows the range in which roughly two-thirds of the returns fall. For the case of, say, common stocks, the average annual return was 11.8 percent, and the standard deviation shows that in two-thirds of the years the average was within± 20.2 percent of this figure. In other words, in two-thirds of the years, common stocks returned more than -8.4 percent and less than ±32.0 percent. Rates of return on stocks were much more variable than those on bonds.
The Excess Return on Common Stocks
Although the qualitative findings from data such as those in Table 1 are consistent with risk aversion, the quantitative nature of the extra returns to common stock holding are inconsistent with many other studies of risk. These other studies suggest that individuals would accept the extra risk that stocks carry for an extra return of around 1 percent per year- significantly less than the 5 percent extra actually provided.
One set of explanations focuses on the possibility that the figures in Table 1 understate the risk of stocks. The risk individuals really care about is changes in their consumption plans. If returns on stocks were highly correlated with the business cycle, then they might pose extra risks because individuals would face a double risk from economic downturns-a fall in
income and a fall in returns from investments. Behavioral economists have offered another explanation: individuals may experience extra psychological pain from losing money on investments in any given period beyond any consequences for their ultimate wealth. (For more on this type of behavioral bias, see the section entitled "Prospect Theory" in Chapter 17.) A recent explanation of the equity premium hinges on catastrophes (wars, riots, depressions, and so on) that have the potential of wiping out much of the value of stocks but so rare that they are seldom experienced, if at all, even in a long period spanning decades. The United States may have experienced an unusually tranquil period during 1926-2102 compared to other periods or countries; subtracting catastrophic losses that might have occurred in a less tranquil period would bring down the average for the return on stocks. Work remains to convince the economics profession that any of these explanations can account for the large equity premium on its own.
Holding stocks in individual companies probably involves greater risks than are reflected in the data for all stocks in Table 1. Do you think these extra risks are relevant to appraising the extra rate of return that stocks provide?
سؤال
سؤال
سؤال
The Equity Premium Puzzle
As shown in Figure 4.4, differences in the rates of return of financial assets reflect, in part, the differing risks associated with those assets. The historical data show that stocks also called equities have indeed had higher returns than bonds to compensate for that risk. In fact, returns on common stock have been so favorable that they pose a puzzle to economists.
Historical Rates of Return
Table 1 cites some of the most widely used rate of return data for U.S. financial markets, compiled by Ibbotson Associates. These data show that over the period 1926-2012, common stocks of large companies provided average annual rates of return which exceeded those on long-term bonds by 5 percent per year, whether the bonds of corporations or the government are considered. The equity premium is even larger if the set of common stocks is expanded to include small companies, the average return on which was a whopping 16.5 percent over the period. The equity premium is larger still if rather than long term, we look at short-term government bonds: the average return on one-year treasury bills was a measly 3.1 percent over the period, essentially no better than inflation.
One way to measure the risk associated with various assets uses the "standard deviation" of their annual returns. This measure shows the range in which roughly two-thirds of the returns fall. For the case of, say, common stocks, the average annual return was 11.8 percent, and the standard deviation shows that in two-thirds of the years the average was within± 20.2 percent of this figure. In other words, in two-thirds of the years, common stocks returned more than -8.4 percent and less than ±32.0 percent. Rates of return on stocks were much more variable than those on bonds.
The Excess Return on Common Stocks
Although the qualitative findings from data such as those in Table 1 are consistent with risk aversion, the quantitative nature of the extra returns to common stock holding are inconsistent with many other studies of risk. These other studies suggest that individuals would accept the extra risk that stocks carry for an extra return of around 1 percent per year- significantly less than the 5 percent extra actually provided.
One set of explanations focuses on the possibility that the figures in Table 1 understate the risk of stocks. The risk individuals really care about is changes in their consumption plans. If returns on stocks were highly correlated with the business cycle, then they might pose extra risks because individuals would face a double risk from economic downturns-a fall in
income and a fall in returns from investments. Behavioral economists have offered another explanation: individuals may experience extra psychological pain from losing money on investments in any given period beyond any consequences for their ultimate wealth. (For more on this type of behavioral bias, see the section entitled "Prospect Theory" in Chapter 17.) A recent explanation of the equity premium hinges on catastrophes (wars, riots, depressions, and so on) that have the potential of wiping out much of the value of stocks but so rare that they are seldom experienced, if at all, even in a long period spanning decades. The United States may have experienced an unusually tranquil period during 1926-2102 compared to other periods or countries; subtracting catastrophic losses that might have occurred in a less tranquil period would bring down the average for the return on stocks. Work remains to convince the economics profession that any of these explanations can account for the large equity premium on its own.
The real return on short-term government bonds implied by Table 1 is less than 1 percent per year. Why do people save at all if this relatively risk-free return is so low?
As shown in Figure 4.4, differences in the rates of return of financial assets reflect, in part, the differing risks associated with those assets. The historical data show that stocks also called equities have indeed had higher returns than bonds to compensate for that risk. In fact, returns on common stock have been so favorable that they pose a puzzle to economists.
Historical Rates of Return
Table 1 cites some of the most widely used rate of return data for U.S. financial markets, compiled by Ibbotson Associates. These data show that over the period 1926-2012, common stocks of large companies provided average annual rates of return which exceeded those on long-term bonds by 5 percent per year, whether the bonds of corporations or the government are considered. The equity premium is even larger if the set of common stocks is expanded to include small companies, the average return on which was a whopping 16.5 percent over the period. The equity premium is larger still if rather than long term, we look at short-term government bonds: the average return on one-year treasury bills was a measly 3.1 percent over the period, essentially no better than inflation.
One way to measure the risk associated with various assets uses the "standard deviation" of their annual returns. This measure shows the range in which roughly two-thirds of the returns fall. For the case of, say, common stocks, the average annual return was 11.8 percent, and the standard deviation shows that in two-thirds of the years the average was within± 20.2 percent of this figure. In other words, in two-thirds of the years, common stocks returned more than -8.4 percent and less than ±32.0 percent. Rates of return on stocks were much more variable than those on bonds.
The Excess Return on Common Stocks
Although the qualitative findings from data such as those in Table 1 are consistent with risk aversion, the quantitative nature of the extra returns to common stock holding are inconsistent with many other studies of risk. These other studies suggest that individuals would accept the extra risk that stocks carry for an extra return of around 1 percent per year- significantly less than the 5 percent extra actually provided.
One set of explanations focuses on the possibility that the figures in Table 1 understate the risk of stocks. The risk individuals really care about is changes in their consumption plans. If returns on stocks were highly correlated with the business cycle, then they might pose extra risks because individuals would face a double risk from economic downturns-a fall in
income and a fall in returns from investments. Behavioral economists have offered another explanation: individuals may experience extra psychological pain from losing money on investments in any given period beyond any consequences for their ultimate wealth. (For more on this type of behavioral bias, see the section entitled "Prospect Theory" in Chapter 17.) A recent explanation of the equity premium hinges on catastrophes (wars, riots, depressions, and so on) that have the potential of wiping out much of the value of stocks but so rare that they are seldom experienced, if at all, even in a long period spanning decades. The United States may have experienced an unusually tranquil period during 1926-2102 compared to other periods or countries; subtracting catastrophic losses that might have occurred in a less tranquil period would bring down the average for the return on stocks. Work remains to convince the economics profession that any of these explanations can account for the large equity premium on its own.
The real return on short-term government bonds implied by Table 1 is less than 1 percent per year. Why do people save at all if this relatively risk-free return is so low?
سؤال
سؤال
فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/38
العب
ملء الشاشة (f)
Deck 4: Uncertainty
1
Mr. Fogg is planning an around-the-world trip. The utility from the trip is a function of how much he spends on it (Y) given by
a) The utility function of around-the world trip is given as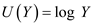 
Mr. Fogg has $10,000 to spend. The probability that he will lose $1,000 is 0.25 and hence, the probability that he will not lose anything is 0.75. Thus, the expected utility of the trip is 
Thus, the expected utility of Mr. Fogg's trip is 
.
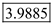b) If Mr. Fogg buys insurance by paying $250 as a premium, he will lose nothing but only the amount of premium. The expected utility in the presence of insurance can be calculated as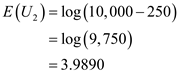
Thus, it can be seen that expected utility from insurance is greater than expected utility without insurance. That is 
.
c) Mr. Fogg would be willing to pay the amount for premium until the expected utility from insurance is same as the expected utility without insurance. That is,
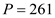Thu, the maximum amount that Mr. Fogg will pay to insure $1,000 is 
.
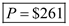d) With the probability of losing $1,000 increased to 0.3, the actuarially fair insurance premium becomes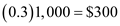
.
Since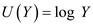
0
, Mr. Fogg will not buy the insurance despite of the fact that it is a fair insurance.
This represents moral hazard.
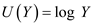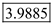Mr. Fogg has $10,000 to spend. The probability that he will lose $1,000 is 0.25 and hence, the probability that he will not lose anything is 0.75. Thus, the expected utility of the trip is Thus, the expected utility of Mr. Fogg's trip is .b) If Mr. Fogg buys insurance by paying $250 as a premium, he will lose nothing but only the amount of premium. The expected utility in the presence of insurance can be calculated as
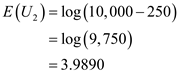Thus, it can be seen that expected utility from insurance is greater than expected utility without insurance. That is .c) Mr. Fogg would be willing to pay the amount for premium until the expected utility from insurance is same as the expected utility without insurance. That is,
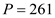Thu, the maximum amount that Mr. Fogg will pay to insure $1,000 is 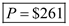.d) With the probability of losing $1,000 increased to 0.3, the actuarially fair insurance premium becomes
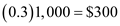.Since
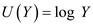0, Mr. Fogg will not buy the insurance despite of the fact that it is a fair insurance.
This represents moral hazard.
2
Explain why the slope of the market line in Figures 4.4 and 4.5 shows how risk is "priced" in this market. How might the data in Application 4.4: Puts, Calls, and Black-Scholes be plotted to determine this slope?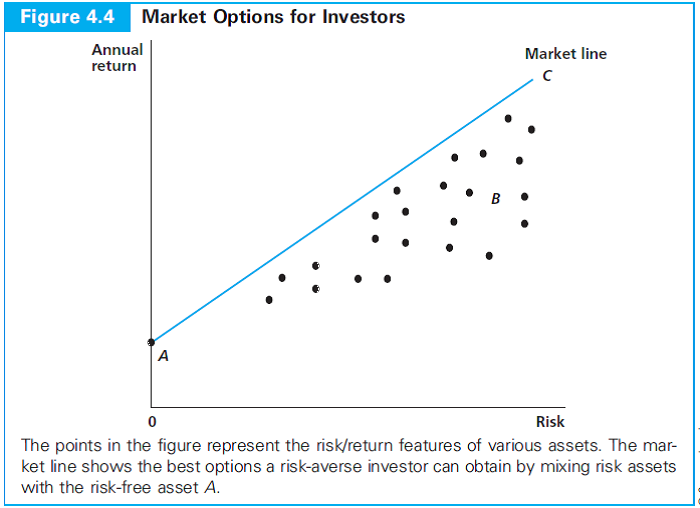
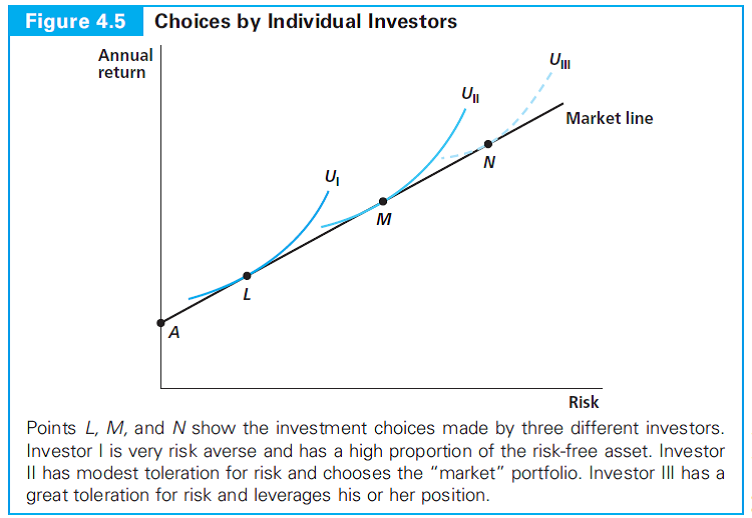
The reason why the slope of the market line in Figures 4.4 and 4.5 shows that risk is priced is required to be stated.
The slope between the expected return and risk is positive. This means as the investor reduces the portfolio risk, the expected return of his/her portfolio must also decline. This shows that the risk is priced.
The slope of these lines can be determined simply by determining the slope between any two points on the line.
The slope between the expected return and risk is positive. This means as the investor reduces the portfolio risk, the expected return of his/her portfolio must also decline. This shows that the risk is priced.
The slope of these lines can be determined simply by determining the slope between any two points on the line.
3
Gamble 1 provides you with an expected value of 50 and an expected utility of 5, while gamble 2 provides you with an expected value of 75 and an expected utility of 4:3. Is it even possible for the gambles to be ranked one way based on expected values and the other way based on expected utilities? Which gamble would you chose, and on what basis?
The first gamble gives an expected value of 50 and an expected utility of 5. The second gamble gives an expected value of 75 and an expected utility of 4.3. It is required to be stated whether it is possible for the gambles to be ranked based on expected values in one way and based on expected utilities in the other way.
If gambles are required to be ranked for preference, they must always be ranked on the basis of expected utility. These expected utilities are different for different individuals, because some individuals are risk seekers, some are risk averse, and some are risk neutral. Moreover, each type varies on degrees. For example, two people may be risk averse, and one might be a lot risk averse than the other one. So different individuals have different preferences, but all of them rank their gambles on the basis of their expected utility.
Of the two gambles given, one would choose the first gamble where the gamble has a lower expected value but higher expected utility. The reason the first gamble has higher utility than the second gamble is likely because it is very less risky than the second one.
If gambles are required to be ranked for preference, they must always be ranked on the basis of expected utility. These expected utilities are different for different individuals, because some individuals are risk seekers, some are risk averse, and some are risk neutral. Moreover, each type varies on degrees. For example, two people may be risk averse, and one might be a lot risk averse than the other one. So different individuals have different preferences, but all of them rank their gambles on the basis of their expected utility.
Of the two gambles given, one would choose the first gamble where the gamble has a lower expected value but higher expected utility. The reason the first gamble has higher utility than the second gamble is likely because it is very less risky than the second one.
4
Let's examine Figure 4A.3 more closely.
1. Why do choices along the "certainty line" imply that there is no risk?
2. If the probability of state 1 is 0.6 and the probability of state 2 is 0.4, what is the actuarially fair slope for the line AE?
3. In general, what determines the slope of the indifference curve EU 3 ?
4. Given your answer to part 2, can you explain why AE and EU 3 have the same slope at point E? (This question is relatively hard.)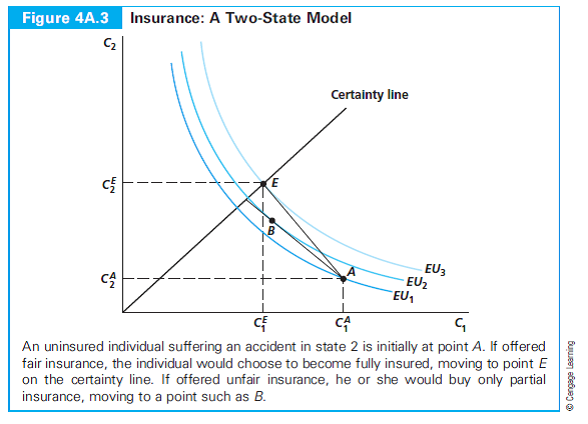
1. Why do choices along the "certainty line" imply that there is no risk?
2. If the probability of state 1 is 0.6 and the probability of state 2 is 0.4, what is the actuarially fair slope for the line AE?
3. In general, what determines the slope of the indifference curve EU 3 ?
4. Given your answer to part 2, can you explain why AE and EU 3 have the same slope at point E? (This question is relatively hard.)
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
5
George Lucas has offered to sell you the option to buy his seventh Star Wars feature for $100 million should that film ever be made.
1. Identify the underlying transaction involved in this option. How would you decide on the expected value of this transaction? How would you assess the variability attached to the value of the transaction? What is the duration of this option?
2. How would you decide how much to pay Mr. Lucas for this option?
1. Identify the underlying transaction involved in this option. How would you decide on the expected value of this transaction? How would you assess the variability attached to the value of the transaction? What is the duration of this option?
2. How would you decide how much to pay Mr. Lucas for this option?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
6
Without looking at Figure 4A.3, see if you can draw your own diagram to illustrate the benefits of, say, health insurance.
2. Use a separate diagram to analyze whether a risk-neutral person would ever want to purchase health insurance and under what conditions if so.
2. Use a separate diagram to analyze whether a risk-neutral person would ever want to purchase health insurance and under what conditions if so.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
7
Sometimes economists speak of the certainty equivalent of a risky stream of income. This problem asks you to compute the certainty equivalent of a risky bet that promises a 50-50 chance of winning or losing $5,000 for someone with a starting income of $50,000. We know that a certain income of somewhat less than $50,000 will provide the same expected utility as will taking this bet. You are asked to calculate precisely the certain income (that is, the certainty equivalent income) that provides the same utility as does this bet for three simple utility functions:
a. U(T)=y/l.
b. U(I) = ln(I) (where ln means ''natural logarithm'') 1
c. U(I)= -1/I
What do you conclude about these utility functions by comparing these three cases?
a. U(T)=y/l.
b. U(I) = ln(I) (where ln means ''natural logarithm'') 1
c. U(I)= -1/I
What do you conclude about these utility functions by comparing these three cases?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
8
If blackjack systems increase people's expected winnings, why doesn't everyone use them? Who do you expect would be most likely to learn how to use the ystems?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
9
"Risk-averse people should only be averse to big gambles with a lot of money at stake. They should jump on any small gamble that is unfair in their favor." Explain why this statement makes sense. Use a utility-of-income graph like Figure 4.1 to illustrate the statement.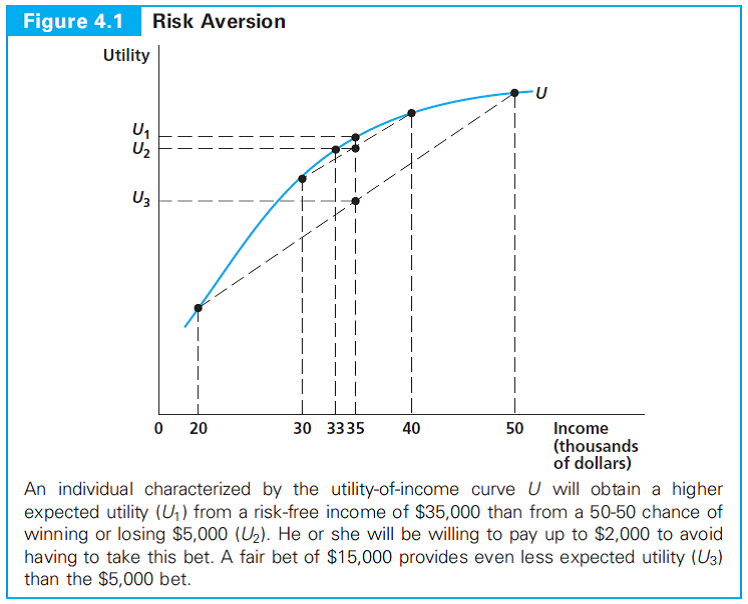
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
10
How does the fact that casinos operate many blackjack tables, slot machines, and roulette tables simultaneously reduce the risk that they will lose money? Is it more riskyto operate a small casino than a large one?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
11
Suppose Molly Jock wishes to purchase a highdefinition television to watch the Olympic wrestling competition in London. Her current income is $20,000, and she knows where she can buy the television she wants for $2,000. She had heard the rumor that the same set can be bought at Crazy Eddie's (recently out of bankruptcy) for $1,700 but is unsure if the rumor is true. Suppose this individual's utility is given by
Utility = ln(Y)where Y is her income after buying the television.
a. What is Molly's utility if she buys from the location she knows?
b. What is Molly's utility if Crazy Eddie's really does offer a lower price?
c. Suppose Molly believes there is a 50-50 chance that Crazy Eddie does offer the lower-priced television, but it will cost her $100 to drive to the discount store to find out for sure (the store is far away and has had its phone disconnected). Is it worth it to her to invest the money in the trip? (Hint: To calculate the utility associated with part c, simply average Molly's utility from the two states: [1] Eddie offers the television; [2] Eddie doesn't offer the television.)
Utility = ln(Y)where Y is her income after buying the television.
a. What is Molly's utility if she buys from the location she knows?
b. What is Molly's utility if Crazy Eddie's really does offer a lower price?
c. Suppose Molly believes there is a 50-50 chance that Crazy Eddie does offer the lower-priced television, but it will cost her $100 to drive to the discount store to find out for sure (the store is far away and has had its phone disconnected). Is it worth it to her to invest the money in the trip? (Hint: To calculate the utility associated with part c, simply average Molly's utility from the two states: [1] Eddie offers the television; [2] Eddie doesn't offer the television.)
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
12
In some cases, you can buy another insurance policy to cover a deductible in your underlying insurance. That is the case, for example, when you rent a car and for ''Medigap'' policies that cover Medicare deductibles. Does buying such a policy make sense?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
13
''The purchase of actuarily fair insurance turns an uncertain situation into a situation where you receive the expected value of income with certainty.'' Explain why this is true. Can you think of circumstances where it might not be true?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
14
Why are deductibles usually stated on an annual basis? If losses occur randomly, wouldn't a ''lifetime'' deductible be better?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
15
A person purchases a dozen eggs and must take them home. Although making trips home is costless, there is a 50 percent chance that all of the eggs carried on one trip will be broken during the trip. This person considers two strategies:
Strategy 1: Take the dozen eggs in one trip.
Strategy 2: Make two trips, taking six eggs in each trip.
a. List the possible outcomes of each strategy and the probabilities of these outcomes. Show that, on average, six eggs make it home under either strategy.
b. Develop a graph to show the utility obtainable under each strategy.
c. Could utility be improved further by taking more than two trips? How would the desirability of this possibility be affected if additional trips were costly?
Strategy 1: Take the dozen eggs in one trip.
Strategy 2: Make two trips, taking six eggs in each trip.
a. List the possible outcomes of each strategy and the probabilities of these outcomes. Show that, on average, six eggs make it home under either strategy.
b. Develop a graph to show the utility obtainable under each strategy.
c. Could utility be improved further by taking more than two trips? How would the desirability of this possibility be affected if additional trips were costly?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
16
Most studies of mutual fund performance conclude that managers cannot consistently exceed the average return in the stock market as a whole. Why might you expect
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
17
Suppose that historical data showed that returns of Japanese stocks and returns on U.S. stocks tended to move in opposite directions. Would it be better to own only one country's stocks or to hold a mixture of the two? How would your answer change if the Japanese stock market always precisely mirrored the U.S. stock market?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
18
Mutual funds compute the net asset value of each share daily. Should the fund's shares sell for this value in the open market?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
19
What is the actuarially fair price for each of the following gambles?
1. Winning $1,000 with probability 0.5 and losing $1,000 with probability 0.5
2. Winning $1,000 with probability 0.6 and losing $1,000 with probability 0.4
3. Winning $1,000 with probability 0.7, winning $2,000 with probability 0.2, and losing
$10,000 with probability 0.1
1. Winning $1,000 with probability 0.5 and losing $1,000 with probability 0.5
2. Winning $1,000 with probability 0.6 and losing $1,000 with probability 0.4
3. Winning $1,000 with probability 0.7, winning $2,000 with probability 0.2, and losing
$10,000 with probability 0.1
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
20
Sophia is a contestant on a game show and has selected the prize that lies behind door number 3. The show's host tells her that there is a 50 percent chance that there is a $15,000 diamond ring behind the door and a 50 percent chance that there is a goat behind the door (which is worth nothing to Sophia, who is allergic to goats). Before the door is opened, someone in the audience shouts, ''I will give you the option of selling me what is behind the door for $8,000 if you will pay me $4,500 for this option.''
a. If Sophia cares only about the expected dollar values of various outcomes, will she buy this option?
b. Explain why Sophia's degree of risk aversion might affect her willingness to buy this option.
a. If Sophia cares only about the expected dollar values of various outcomes, will she buy this option?
b. Explain why Sophia's degree of risk aversion might affect her willingness to buy this option.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
21
Puts, Calls, and Black-Scholes
Options on financial assets are widely traded in organized markets. Not only are there options available on most company's stocks, but there are also a bewildering variety of options on such assets as bonds, foreign exchange, and commodities, or even on indexes based on groups of these assets. Probably the most common options are those related to the stock of a single company. The potential transactions underlying these options are simply promises to buy or sell the stock at a specific ("strike") price over some period in the future. Options to buy a stock at a certain strike price are termed "call" options because the buyer has the right to "call" the stock from someone else if he or she wishes to exercise the option. Options to sell a stock at a certain price are called "put" options (perhaps because you have the option to put the stock into someone else's hands).
Suppose that Microsoft stock is currently selling at $30 per share. A call option might give you the right (but, again, not the obligation) to buy Microsoft in one month at, say, $32 per share.1 Suppose you also believe there is a 50-50 chance that Microsoft will sell for either $35 or $25 in one month's time. Clearly the option to buy at $32 is valuable-the stock might end up at $35. But how much is this option worth?
An Equivalent Portfolio
One way that financial economists evaluate options is by asking whether there is another set of assets that would yield the same outcomes as would the option. If such a set exists, one can then argue that it should have the same price as the option because markets will ensure that the same good always has the same price. So, let's consider the outcomes of the Microsoft option. If Microsoft sells for $25 in a month's time, the option is worthless-why pay $32 when the stock can readily be bought for $25? If Microsoft sells for $35, however, the option will be worth $3. Could we duplicate these two payouts with some other set of assets? Suppose we borrow some funds (L) from a bank (with no interest, to make things simple) and buy a fraction (k) of a Microsoft share. After a month, we will sell the fractional share of Microsoft and pay off the loan. In this example, L and k must be chosen to yield the same outcomes as the option. That is,
These two equations can easily be solved as k = 0.3, L = 7.5. That is, buying 0.3 of a Microsoft share and taking a loan of $7.50 will yield the same outcomes as buying the option. The net cost of this strategy is $1.50?$9 to buy 0.3 of a Microsoft share at $30 less the loan of $7.50 (which in our simple case carries no interest). Hence, this also is the value of the option.
The Black-Scholes Theorem
Of course, valuing options in the real world is much more complicated than this simple example suggests. Three specific complications that need to be addressed in developing a more general theory of valuation are as follows: (1) there are far more possibilities for Microsoft stock's price in one month than just the two we assumed; (2) most popular options can be exercised at any time during a specified period, not just on a specific date; and (3) interest rates matter for any economic transaction that occurs over time. Taking account of these factors proved to be very difficult, and it was not until 1973 that Fischer Black and Myron Scholes developed an acceptable valuation model.2 Since that time, the Black-Scholes model has been widely applied to options and other markets. In one of its more innovative applications, the model is now used in reverse to calculate an "implied volatility" expected for stocks in the future. The Chicago Board Options Exchange Volatility Index (VIX) is widely followed in the financial press, where it is taken as a good measure of the current uncertainties involved in stock market investing.
For every buyer of, say, a call option, there must of course also be a seller. Why would someone sell a call option on some shares he or she already owned? How would this be different than buying a put option on this stock?
Options on financial assets are widely traded in organized markets. Not only are there options available on most company's stocks, but there are also a bewildering variety of options on such assets as bonds, foreign exchange, and commodities, or even on indexes based on groups of these assets. Probably the most common options are those related to the stock of a single company. The potential transactions underlying these options are simply promises to buy or sell the stock at a specific ("strike") price over some period in the future. Options to buy a stock at a certain strike price are termed "call" options because the buyer has the right to "call" the stock from someone else if he or she wishes to exercise the option. Options to sell a stock at a certain price are called "put" options (perhaps because you have the option to put the stock into someone else's hands).
Suppose that Microsoft stock is currently selling at $30 per share. A call option might give you the right (but, again, not the obligation) to buy Microsoft in one month at, say, $32 per share.1 Suppose you also believe there is a 50-50 chance that Microsoft will sell for either $35 or $25 in one month's time. Clearly the option to buy at $32 is valuable-the stock might end up at $35. But how much is this option worth?
An Equivalent Portfolio
One way that financial economists evaluate options is by asking whether there is another set of assets that would yield the same outcomes as would the option. If such a set exists, one can then argue that it should have the same price as the option because markets will ensure that the same good always has the same price. So, let's consider the outcomes of the Microsoft option. If Microsoft sells for $25 in a month's time, the option is worthless-why pay $32 when the stock can readily be bought for $25? If Microsoft sells for $35, however, the option will be worth $3. Could we duplicate these two payouts with some other set of assets? Suppose we borrow some funds (L) from a bank (with no interest, to make things simple) and buy a fraction (k) of a Microsoft share. After a month, we will sell the fractional share of Microsoft and pay off the loan. In this example, L and k must be chosen to yield the same outcomes as the option. That is,
These two equations can easily be solved as k = 0.3, L = 7.5. That is, buying 0.3 of a Microsoft share and taking a loan of $7.50 will yield the same outcomes as buying the option. The net cost of this strategy is $1.50?$9 to buy 0.3 of a Microsoft share at $30 less the loan of $7.50 (which in our simple case carries no interest). Hence, this also is the value of the option.
The Black-Scholes Theorem
Of course, valuing options in the real world is much more complicated than this simple example suggests. Three specific complications that need to be addressed in developing a more general theory of valuation are as follows: (1) there are far more possibilities for Microsoft stock's price in one month than just the two we assumed; (2) most popular options can be exercised at any time during a specified period, not just on a specific date; and (3) interest rates matter for any economic transaction that occurs over time. Taking account of these factors proved to be very difficult, and it was not until 1973 that Fischer Black and Myron Scholes developed an acceptable valuation model.2 Since that time, the Black-Scholes model has been widely applied to options and other markets. In one of its more innovative applications, the model is now used in reverse to calculate an "implied volatility" expected for stocks in the future. The Chicago Board Options Exchange Volatility Index (VIX) is widely followed in the financial press, where it is taken as a good measure of the current uncertainties involved in stock market investing.
For every buyer of, say, a call option, there must of course also be a seller. Why would someone sell a call option on some shares he or she already owned? How would this be different than buying a put option on this stock?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
22
Wen, who has current wealth of $10,000, decides to take advantage of a free trip to Las Vegas to play roulette. His utility function over the wealth he ends up with after the trip Y (which equals his current wealth adjusted by any amount he ends up winning or losing) is 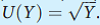He can take one of two gambles at the roulette table:
Gamble 1: Wager $1,000 on red. If red comes up, which happens with probability 18/38, he wins $1,000; otherwise he loses his $1,000 wager.
Gamble 2: Wager $500 on the single number 00. If 00 comes up, which happens with probability 1/38, he wins 35 times his wager ($17,500); otherwise he loses his $500 wager.
a. Are these fair gambles?
b. If Wen is forced to take one of the two gambles, which would he prefer?
c. If Wen isn't forced to play roulette, would he take a gamble?
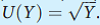He can take one of two gambles at the roulette table: Gamble 1: Wager $1,000 on red. If red comes up, which happens with probability 18/38, he wins $1,000; otherwise he loses his $1,000 wager.
Gamble 2: Wager $500 on the single number 00. If 00 comes up, which happens with probability 1/38, he wins 35 times his wager ($17,500); otherwise he loses his $500 wager.
a. Are these fair gambles?
b. If Wen is forced to take one of the two gambles, which would he prefer?
c. If Wen isn't forced to play roulette, would he take a gamble?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
23
As discussed in Application 4.4, a call option provides you with the option to buy a share of, say, Microsoft stock at a specified price of $60. Suppose that this option can only be exercised at exactly 10:00 am on June 1, 2009. What will determine the expected value of the transaction underlying this option? What will determine the variability around this expected value? Explain why the greater this expected variability, the greater is the value of this option.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
24
Puts, Calls, and Black-Scholes
Options on financial assets are widely traded in organized markets. Not only are there options available on most company's stocks, but there are also a bewildering variety of options on such assets as bonds, foreign exchange, and commodities, or even on indexes based on groups of these assets. Probably the most common options are those related to the stock of a single company. The potential transactions underlying these options are simply promises to buy or sell the stock at a specific ("strike") price over some period in the future. Options to buy a stock at a certain strike price are termed "call" options because the buyer has the right to "call" the stock from someone else if he or she wishes to exercise the option. Options to sell a stock at a certain price are called "put" options (perhaps because you have the option to put the stock into someone else's hands).
Suppose that Microsoft stock is currently selling at $30 per share. A call option might give you the right (but, again, not the obligation) to buy Microsoft in one month at, say, $32 per share.1 Suppose you also believe there is a 50-50 chance that Microsoft will sell for either $35 or $25 in one month's time. Clearly the option to buy at $32 is valuable-the stock might end up at $35. But how much is this option worth?
An Equivalent Portfolio
One way that financial economists evaluate options is by asking whether there is another set of assets that would yield the same outcomes as would the option. If such a set exists, one can then argue that it should have the same price as the option because markets will ensure that the same good always has the same price. So, let's consider the outcomes of the Microsoft option. If Microsoft sells for $25 in a month's time, the option is worthless-why pay $32 when the stock can readily be bought for $25? If Microsoft sells for $35, however, the option will be worth $3. Could we duplicate these two payouts with some other set of assets? Suppose we borrow some funds (L) from a bank (with no interest, to make things simple) and buy a fraction (k) of a Microsoft share. After a month, we will sell the fractional share of Microsoft and pay off the loan. In this example, L and k must be chosen to yield the same outcomes as the option. That is,
These two equations can easily be solved as k = 0.3, L = 7.5. That is, buying 0.3 of a Microsoft share and taking a loan of $7.50 will yield the same outcomes as buying the option. The net cost of this strategy is $1.50?$9 to buy 0.3 of a Microsoft share at $30 less the loan of $7.50 (which in our simple case carries no interest). Hence, this also is the value of the option.
The Black-Scholes Theorem
Of course, valuing options in the real world is much more complicated than this simple example suggests. Three specific complications that need to be addressed in developing a more general theory of valuation are as follows: (1) there are far more possibilities for Microsoft stock's price in one month than just the two we assumed; (2) most popular options can be exercised at any time during a specified period, not just on a specific date; and (3) interest rates matter for any economic transaction that occurs over time. Taking account of these factors proved to be very difficult, and it was not until 1973 that Fischer Black and Myron Scholes developed an acceptable valuation model.2 Since that time, the Black-Scholes model has been widely applied to options and other markets. In one of its more innovative applications, the model is now used in reverse to calculate an "implied volatility" expected for stocks in the future. The Chicago Board Options Exchange Volatility Index (VIX) is widely followed in the financial press, where it is taken as a good measure of the current uncertainties involved in stock market investing.
The Black-Scholes model assumes that stock returns are random and that they follow a bell-shaped (normal) distribution. Does this seem a reasonable assumption?
Options on financial assets are widely traded in organized markets. Not only are there options available on most company's stocks, but there are also a bewildering variety of options on such assets as bonds, foreign exchange, and commodities, or even on indexes based on groups of these assets. Probably the most common options are those related to the stock of a single company. The potential transactions underlying these options are simply promises to buy or sell the stock at a specific ("strike") price over some period in the future. Options to buy a stock at a certain strike price are termed "call" options because the buyer has the right to "call" the stock from someone else if he or she wishes to exercise the option. Options to sell a stock at a certain price are called "put" options (perhaps because you have the option to put the stock into someone else's hands).
Suppose that Microsoft stock is currently selling at $30 per share. A call option might give you the right (but, again, not the obligation) to buy Microsoft in one month at, say, $32 per share.1 Suppose you also believe there is a 50-50 chance that Microsoft will sell for either $35 or $25 in one month's time. Clearly the option to buy at $32 is valuable-the stock might end up at $35. But how much is this option worth?
An Equivalent Portfolio
One way that financial economists evaluate options is by asking whether there is another set of assets that would yield the same outcomes as would the option. If such a set exists, one can then argue that it should have the same price as the option because markets will ensure that the same good always has the same price. So, let's consider the outcomes of the Microsoft option. If Microsoft sells for $25 in a month's time, the option is worthless-why pay $32 when the stock can readily be bought for $25? If Microsoft sells for $35, however, the option will be worth $3. Could we duplicate these two payouts with some other set of assets? Suppose we borrow some funds (L) from a bank (with no interest, to make things simple) and buy a fraction (k) of a Microsoft share. After a month, we will sell the fractional share of Microsoft and pay off the loan. In this example, L and k must be chosen to yield the same outcomes as the option. That is,
These two equations can easily be solved as k = 0.3, L = 7.5. That is, buying 0.3 of a Microsoft share and taking a loan of $7.50 will yield the same outcomes as buying the option. The net cost of this strategy is $1.50?$9 to buy 0.3 of a Microsoft share at $30 less the loan of $7.50 (which in our simple case carries no interest). Hence, this also is the value of the option.
The Black-Scholes Theorem
Of course, valuing options in the real world is much more complicated than this simple example suggests. Three specific complications that need to be addressed in developing a more general theory of valuation are as follows: (1) there are far more possibilities for Microsoft stock's price in one month than just the two we assumed; (2) most popular options can be exercised at any time during a specified period, not just on a specific date; and (3) interest rates matter for any economic transaction that occurs over time. Taking account of these factors proved to be very difficult, and it was not until 1973 that Fischer Black and Myron Scholes developed an acceptable valuation model.2 Since that time, the Black-Scholes model has been widely applied to options and other markets. In one of its more innovative applications, the model is now used in reverse to calculate an "implied volatility" expected for stocks in the future. The Chicago Board Options Exchange Volatility Index (VIX) is widely followed in the financial press, where it is taken as a good measure of the current uncertainties involved in stock market investing.
The Black-Scholes model assumes that stock returns are random and that they follow a bell-shaped (normal) distribution. Does this seem a reasonable assumption?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
25
What does it mean to say we expect a fair coin to come up heads about half the time? Would you expect the fraction of heads to get closer to exactly 0.5 as more coins are flipped? Explain how this law of large numbers applies to the risks faced by casinos or insurance companies.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
26
The option on Microsoft stock described in Application 4.4: Puts, Calls, and Black-Scholes gave the owner the right to buy one share at $32 one month from now. Microsoft currently sells for $30 per share, and investors believe there is a 50-50 chance that it could become either $35 or $25 in one month. Now let's see how various features of this option affect its value:
a. How would an increase in the strike price of the option, from $32 to $33, affect the value of the option?
b. How would an increase in the current price of Microsoft stock, from $30 to $31 per share, affect the value of the original option?
c. How would an increase in the volatility of Microsoft stock, so that there was a 50-50 chance that it could sell for either $40 or $20, affect the value of the original option?
d. How would a change in the interest rate affect the value of the original option? Is this an unrealistic feature of this example? How would you make it more realistic?
a. How would an increase in the strike price of the option, from $32 to $33, affect the value of the option?
b. How would an increase in the current price of Microsoft stock, from $30 to $31 per share, affect the value of the original option?
c. How would an increase in the volatility of Microsoft stock, so that there was a 50-50 chance that it could sell for either $40 or $20, affect the value of the original option?
d. How would a change in the interest rate affect the value of the original option? Is this an unrealistic feature of this example? How would you make it more realistic?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
27
U.S. politicians have been touting the need for ''energy independence'' (reducing reliance on imported foreign oil) achieved in part by the use of alternative fuels and in part by consumer conservation. Suppose reluctance of consumers to make investments in conservation is due to lack of information or foresight.What sort of government policies might work to increase conservation?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
28
What would the utility-of-income curve U be shaped like for someone who prefers risky situations?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
29
College students are familiar with the real option of being able to drop a course before the end of the term. The text provided a list of factors affecting the value of any option (value of underlying opportunity, variation in the situation, duration, price). What is meant by each one of these factors in the context of the decision to drop a course? How do the factors affect the value of this option? Given that options are valuable, how ould explain why some colleges put certain limits on the ability of students to drop courses?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
30
Suppose instead that the energy paradox is due to consumers' sophisticated valuation of the options provided by waiting. How would this affect government conservation policy? Would there still be a reason for the government to intervene in this market?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
31
Suppose a person must accept one of three bets:
Bet 1: Win $100 with probability V2; lose $100with probability 1/2.
Bet 2: Win $100 with probability 3/i; lose $300 with probability 1=4.
Bet 3: Win $100 with probability 9 10; lose $900with probability 1 10.
a. Show that all of these are fair bets.
b. Graph each bet on a utility of income curve similar to Figure.
FIGURE Risk Aversion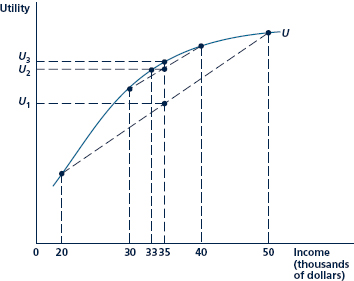
An individual characterized by the utility-of-income curve U will obtain a higher utility (U3) from a risk-free income of $35,000 than from a 50-50 chance of winning or losing $5,000 (U2). He or she will be willing to pay up to $2,000 to avoid having to take this bet. A fair bet of $15,000 provides even less utility (U1) than the $5,000 bet.
c. Explain carefully which bet will be preferred and why.
Bet 1: Win $100 with probability V2; lose $100with probability 1/2.
Bet 2: Win $100 with probability 3/i; lose $300 with probability 1=4.
Bet 3: Win $100 with probability 9 10; lose $900with probability 1 10.
a. Show that all of these are fair bets.
b. Graph each bet on a utility of income curve similar to Figure.
FIGURE Risk Aversion
An individual characterized by the utility-of-income curve U will obtain a higher utility (U3) from a risk-free income of $35,000 than from a 50-50 chance of winning or losing $5,000 (U2). He or she will be willing to pay up to $2,000 to avoid having to take this bet. A fair bet of $15,000 provides even less utility (U1) than the $5,000 bet.
c. Explain carefully which bet will be preferred and why.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
32
This problem will help you understand why Application 4.6: The Equity Premium Puzzle" really is a puzzle. Suppose that a person with $100,000 to invest believes that stocks will have a real return over the next year of 7 percent. He or she also believes that bonds will have a real return of 2 percent over the next year. This person believes (probably contrary to fact) that the real return on bonds is certain-an investment in bonds will definitely yield 2 percent. For stocks, however, he or she believes that there is a 50 percent chance that stocks will yield 16 percent, but also a 50 percent chance they will yield -2 percent. Hence stocks are viewed as being much riskier than bonds.
a. Calculate the certainty equivalent yield for stocks using the three utility functions in Problem 4.6. What do you conclude about whether this person will invest the $100,000 in stocks or bonds?
b. The most risk-averse utility function economists usually ever encounter is U(I) = -I -10. If your scientific calculator is up to the task, calculate the certainty equivalent yield for stocks with this utility function. What do you conclude?
(Hint: The calculations in this problem are most easily accomplished by using outcomes in dollars-that is, for example, those that have a 50-50 chance of producing a final wealth of $116,000 or $98,000. If this were to yield a certainty equivalent wealth of, say, $105,000, the certainty equivalent yield would be 5 percent.)
a. Calculate the certainty equivalent yield for stocks using the three utility functions in Problem 4.6. What do you conclude about whether this person will invest the $100,000 in stocks or bonds?
b. The most risk-averse utility function economists usually ever encounter is U(I) = -I -10. If your scientific calculator is up to the task, calculate the certainty equivalent yield for stocks with this utility function. What do you conclude?
(Hint: The calculations in this problem are most easily accomplished by using outcomes in dollars-that is, for example, those that have a 50-50 chance of producing a final wealth of $116,000 or $98,000. If this were to yield a certainty equivalent wealth of, say, $105,000, the certainty equivalent yield would be 5 percent.)
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
33
The Equity Premium Puzzle
As shown in Figure 4.4, differences in the rates of return of financial assets reflect, in part, the differing risks associated with those assets. The historical data show that stocks also called equities have indeed had higher returns than bonds to compensate for that risk. In fact, returns on common stock have been so favorable that they pose a puzzle to economists.
Historical Rates of Return
Table 1 cites some of the most widely used rate of return data for U.S. financial markets, compiled by Ibbotson Associates. These data show that over the period 1926-2012, common stocks of large companies provided average annual rates of return which exceeded those on long-term bonds by 5 percent per year, whether the bonds of corporations or the government are considered. The equity premium is even larger if the set of common stocks is expanded to include small companies, the average return on which was a whopping 16.5 percent over the period. The equity premium is larger still if rather than long term, we look at short-term government bonds: the average return on one-year treasury bills was a measly 3.1 percent over the period, essentially no better than inflation.
One way to measure the risk associated with various assets uses the "standard deviation" of their annual returns. This measure shows the range in which roughly two-thirds of the returns fall. For the case of, say, common stocks, the average annual return was 11.8 percent, and the standard deviation shows that in two-thirds of the years the average was within± 20.2 percent of this figure. In other words, in two-thirds of the years, common stocks returned more than -8.4 percent and less than ±32.0 percent. Rates of return on stocks were much more variable than those on bonds.
The Excess Return on Common Stocks
Although the qualitative findings from data such as those in Table 1 are consistent with risk aversion, the quantitative nature of the extra returns to common stock holding are inconsistent with many other studies of risk. These other studies suggest that individuals would accept the extra risk that stocks carry for an extra return of around 1 percent per year- significantly less than the 5 percent extra actually provided.
One set of explanations focuses on the possibility that the figures in Table 1 understate the risk of stocks. The risk individuals really care about is changes in their consumption plans. If returns on stocks were highly correlated with the business cycle, then they might pose extra risks because individuals would face a double risk from economic downturns-a fall in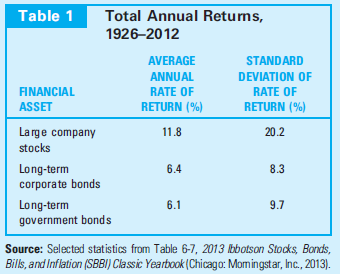
income and a fall in returns from investments. Behavioral economists have offered another explanation: individuals may experience extra psychological pain from losing money on investments in any given period beyond any consequences for their ultimate wealth. (For more on this type of behavioral bias, see the section entitled "Prospect Theory" in Chapter 17.) A recent explanation of the equity premium hinges on catastrophes (wars, riots, depressions, and so on) that have the potential of wiping out much of the value of stocks but so rare that they are seldom experienced, if at all, even in a long period spanning decades. The United States may have experienced an unusually tranquil period during 1926-2102 compared to other periods or countries; subtracting catastrophic losses that might have occurred in a less tranquil period would bring down the average for the return on stocks. Work remains to convince the economics profession that any of these explanations can account for the large equity premium on its own.
Holding stocks in individual companies probably involves greater risks than are reflected in the data for all stocks in Table 1. Do you think these extra risks are relevant to appraising the extra rate of return that stocks provide?
As shown in Figure 4.4, differences in the rates of return of financial assets reflect, in part, the differing risks associated with those assets. The historical data show that stocks also called equities have indeed had higher returns than bonds to compensate for that risk. In fact, returns on common stock have been so favorable that they pose a puzzle to economists.
Historical Rates of Return
Table 1 cites some of the most widely used rate of return data for U.S. financial markets, compiled by Ibbotson Associates. These data show that over the period 1926-2012, common stocks of large companies provided average annual rates of return which exceeded those on long-term bonds by 5 percent per year, whether the bonds of corporations or the government are considered. The equity premium is even larger if the set of common stocks is expanded to include small companies, the average return on which was a whopping 16.5 percent over the period. The equity premium is larger still if rather than long term, we look at short-term government bonds: the average return on one-year treasury bills was a measly 3.1 percent over the period, essentially no better than inflation.
One way to measure the risk associated with various assets uses the "standard deviation" of their annual returns. This measure shows the range in which roughly two-thirds of the returns fall. For the case of, say, common stocks, the average annual return was 11.8 percent, and the standard deviation shows that in two-thirds of the years the average was within± 20.2 percent of this figure. In other words, in two-thirds of the years, common stocks returned more than -8.4 percent and less than ±32.0 percent. Rates of return on stocks were much more variable than those on bonds.
The Excess Return on Common Stocks
Although the qualitative findings from data such as those in Table 1 are consistent with risk aversion, the quantitative nature of the extra returns to common stock holding are inconsistent with many other studies of risk. These other studies suggest that individuals would accept the extra risk that stocks carry for an extra return of around 1 percent per year- significantly less than the 5 percent extra actually provided.
One set of explanations focuses on the possibility that the figures in Table 1 understate the risk of stocks. The risk individuals really care about is changes in their consumption plans. If returns on stocks were highly correlated with the business cycle, then they might pose extra risks because individuals would face a double risk from economic downturns-a fall in
income and a fall in returns from investments. Behavioral economists have offered another explanation: individuals may experience extra psychological pain from losing money on investments in any given period beyond any consequences for their ultimate wealth. (For more on this type of behavioral bias, see the section entitled "Prospect Theory" in Chapter 17.) A recent explanation of the equity premium hinges on catastrophes (wars, riots, depressions, and so on) that have the potential of wiping out much of the value of stocks but so rare that they are seldom experienced, if at all, even in a long period spanning decades. The United States may have experienced an unusually tranquil period during 1926-2102 compared to other periods or countries; subtracting catastrophic losses that might have occurred in a less tranquil period would bring down the average for the return on stocks. Work remains to convince the economics profession that any of these explanations can account for the large equity premium on its own.
Holding stocks in individual companies probably involves greater risks than are reflected in the data for all stocks in Table 1. Do you think these extra risks are relevant to appraising the extra rate of return that stocks provide?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
34
Why does the assumption of diminishing marginal utility of income imply risk aversion? Can you think of other assumptions that would result in risk-averse behavior (such as the purchase of insurance) but would not require the difficult-to-verify notion of diminishing marginal utility?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
35
Our analysis in this chapter suggests that individuals have a utility-maximizing amount of information. Explain why some degree of ignorance is optimal.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
36
The Equity Premium Puzzle
As shown in Figure 4.4, differences in the rates of return of financial assets reflect, in part, the differing risks associated with those assets. The historical data show that stocks also called equities have indeed had higher returns than bonds to compensate for that risk. In fact, returns on common stock have been so favorable that they pose a puzzle to economists.
Historical Rates of Return
Table 1 cites some of the most widely used rate of return data for U.S. financial markets, compiled by Ibbotson Associates. These data show that over the period 1926-2012, common stocks of large companies provided average annual rates of return which exceeded those on long-term bonds by 5 percent per year, whether the bonds of corporations or the government are considered. The equity premium is even larger if the set of common stocks is expanded to include small companies, the average return on which was a whopping 16.5 percent over the period. The equity premium is larger still if rather than long term, we look at short-term government bonds: the average return on one-year treasury bills was a measly 3.1 percent over the period, essentially no better than inflation.
One way to measure the risk associated with various assets uses the "standard deviation" of their annual returns. This measure shows the range in which roughly two-thirds of the returns fall. For the case of, say, common stocks, the average annual return was 11.8 percent, and the standard deviation shows that in two-thirds of the years the average was within± 20.2 percent of this figure. In other words, in two-thirds of the years, common stocks returned more than -8.4 percent and less than ±32.0 percent. Rates of return on stocks were much more variable than those on bonds.
The Excess Return on Common Stocks
Although the qualitative findings from data such as those in Table 1 are consistent with risk aversion, the quantitative nature of the extra returns to common stock holding are inconsistent with many other studies of risk. These other studies suggest that individuals would accept the extra risk that stocks carry for an extra return of around 1 percent per year- significantly less than the 5 percent extra actually provided.
One set of explanations focuses on the possibility that the figures in Table 1 understate the risk of stocks. The risk individuals really care about is changes in their consumption plans. If returns on stocks were highly correlated with the business cycle, then they might pose extra risks because individuals would face a double risk from economic downturns-a fall in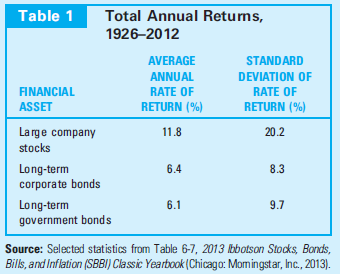
income and a fall in returns from investments. Behavioral economists have offered another explanation: individuals may experience extra psychological pain from losing money on investments in any given period beyond any consequences for their ultimate wealth. (For more on this type of behavioral bias, see the section entitled "Prospect Theory" in Chapter 17.) A recent explanation of the equity premium hinges on catastrophes (wars, riots, depressions, and so on) that have the potential of wiping out much of the value of stocks but so rare that they are seldom experienced, if at all, even in a long period spanning decades. The United States may have experienced an unusually tranquil period during 1926-2102 compared to other periods or countries; subtracting catastrophic losses that might have occurred in a less tranquil period would bring down the average for the return on stocks. Work remains to convince the economics profession that any of these explanations can account for the large equity premium on its own.
The real return on short-term government bonds implied by Table 1 is less than 1 percent per year. Why do people save at all if this relatively risk-free return is so low?
As shown in Figure 4.4, differences in the rates of return of financial assets reflect, in part, the differing risks associated with those assets. The historical data show that stocks also called equities have indeed had higher returns than bonds to compensate for that risk. In fact, returns on common stock have been so favorable that they pose a puzzle to economists.
Historical Rates of Return
Table 1 cites some of the most widely used rate of return data for U.S. financial markets, compiled by Ibbotson Associates. These data show that over the period 1926-2012, common stocks of large companies provided average annual rates of return which exceeded those on long-term bonds by 5 percent per year, whether the bonds of corporations or the government are considered. The equity premium is even larger if the set of common stocks is expanded to include small companies, the average return on which was a whopping 16.5 percent over the period. The equity premium is larger still if rather than long term, we look at short-term government bonds: the average return on one-year treasury bills was a measly 3.1 percent over the period, essentially no better than inflation.
One way to measure the risk associated with various assets uses the "standard deviation" of their annual returns. This measure shows the range in which roughly two-thirds of the returns fall. For the case of, say, common stocks, the average annual return was 11.8 percent, and the standard deviation shows that in two-thirds of the years the average was within± 20.2 percent of this figure. In other words, in two-thirds of the years, common stocks returned more than -8.4 percent and less than ±32.0 percent. Rates of return on stocks were much more variable than those on bonds.
The Excess Return on Common Stocks
Although the qualitative findings from data such as those in Table 1 are consistent with risk aversion, the quantitative nature of the extra returns to common stock holding are inconsistent with many other studies of risk. These other studies suggest that individuals would accept the extra risk that stocks carry for an extra return of around 1 percent per year- significantly less than the 5 percent extra actually provided.
One set of explanations focuses on the possibility that the figures in Table 1 understate the risk of stocks. The risk individuals really care about is changes in their consumption plans. If returns on stocks were highly correlated with the business cycle, then they might pose extra risks because individuals would face a double risk from economic downturns-a fall in
income and a fall in returns from investments. Behavioral economists have offered another explanation: individuals may experience extra psychological pain from losing money on investments in any given period beyond any consequences for their ultimate wealth. (For more on this type of behavioral bias, see the section entitled "Prospect Theory" in Chapter 17.) A recent explanation of the equity premium hinges on catastrophes (wars, riots, depressions, and so on) that have the potential of wiping out much of the value of stocks but so rare that they are seldom experienced, if at all, even in a long period spanning decades. The United States may have experienced an unusually tranquil period during 1926-2102 compared to other periods or countries; subtracting catastrophic losses that might have occurred in a less tranquil period would bring down the average for the return on stocks. Work remains to convince the economics profession that any of these explanations can account for the large equity premium on its own.
The real return on short-term government bonds implied by Table 1 is less than 1 percent per year. Why do people save at all if this relatively risk-free return is so low?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
37
Explain why the following are examples of diversification-that is, explain why each choice specified offers the same expected value, though the preferred choice is lower in risk.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
38
This problem is based on the two-state model in the appendix to this chapter. Leah, who recently graduated from college, is deciding whether to purchase renter's insurance, which insures one's personal property in an apartment against accidents or theft, from Gecko Insurance. The following questions direct you to use diagrams to analyze her decision.
a. Use a two-state diagram along the lines of Figure 4A.3 to explain why Leah could benefit from renter's insurance.
b. Suppose Leah is risk neutral. Use a diagram to determine whether she would buy fair insurance. Would she buy unfair insurance in her favor? Would she buy insurance that provides Gecko Insurance with a profit margin above what is needed to offset the expected loss (in other words, unfair insurance in Gecko's favor)?
c. Suppose again that Leah is risk averse but that now the chance of accident or theft is extremely remote. Use a diagram to explain why she would not be inclined to buy unfair insurance in Gecko's favor.
a. Use a two-state diagram along the lines of Figure 4A.3 to explain why Leah could benefit from renter's insurance.
b. Suppose Leah is risk neutral. Use a diagram to determine whether she would buy fair insurance. Would she buy unfair insurance in her favor? Would she buy insurance that provides Gecko Insurance with a profit margin above what is needed to offset the expected loss (in other words, unfair insurance in Gecko's favor)?
c. Suppose again that Leah is risk averse but that now the chance of accident or theft is extremely remote. Use a diagram to explain why she would not be inclined to buy unfair insurance in Gecko's favor.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
فتح الحزمة
k this deck
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 38 في هذه المجموعة.
