Deck 11: Partial Derivatives
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/294
العب
ملء الشاشة (f)
Deck 11: Partial Derivatives
1
Find the maximum value of the function subject to the constraint that .
A)
B)
C)1
D)
E)2
F)
G)4
H)
A)
B)
C)1
D)
E)2
F)
G)4
H)
1
2
Find the point at which the function has the maximum value subject to the constraint that .
A)
B)
C)
D)
E)
F)
G)
H)None of these
A)
B)
C)
D)
E)
F)
G)
H)None of these
3
Find the maximum value of the function subject to the constraint that .
A)
B)1
C)
D)2
E)
F)
G)
H)
A)
B)1
C)
D)2
E)
F)
G)
H)
1
4
Optimize 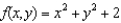 subject to
subject to 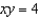 .
.
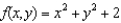 subject to
subject to 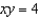 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
5
Use the method of Lagrange multipliers to find points on the surface of 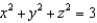 where the function
where the function 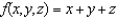 has
has
(a) a minimum
(b) a maximum.
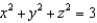 where the function
where the function 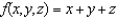 has
has(a) a minimum
(b) a maximum.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
6
Find two positive numbers who sum is eighteen and whose product is a maximum, using the method of Lagrange multipliers

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
7
Find the greatest product three numbers can have if the sum of their squares must be 48.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
8
Compute the minimum value of 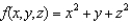 subject to the condition that
subject to the condition that 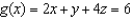 .
.
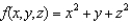 subject to the condition that
subject to the condition that 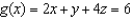 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
9
What is the shortest distance from the origin to the surface 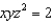 ?
?
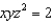 ?
?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
10
Find the point at which the function has the minimum value subject to the constraint that .
A)
B)
C)
D)
E)
F)
G)
H)None of these
A)
B)
C)
D)
E)
F)
G)
H)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
11
In using Lagrange multipliers to minimize the function subject to the constraint that , what is the value of the multiplier ?
A)
B)1
C)
D)2
E)
F)3
G)
H)4
A)
B)1
C)
D)2
E)
F)3
G)
H)4

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
12
Solve completely, using Lagrange multipliers: Find the dimension of a box with volume 1000 which minimizes the total length of the 12 edges.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
13
Find the extreme values of 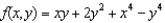 on the circle
on the circle 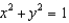 .
.
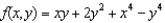 on the circle
on the circle 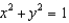 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
14
Find the maximum value of the function subject to the constraint that .
A)
B)
C)
D)
E)4
F)5
G)6
H)7
A)
B)
C)
D)
E)4
F)5
G)6
H)7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
15
Find the point on the plane 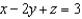 where
where 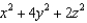 is minimum.
is minimum.
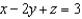 where
where 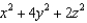 is minimum.
is minimum.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
16
In using Lagrange multipliers to minimize the function subject to the constraint that , what is the value of the multiplier ?
A)
B)1
C)
D)2
E)
F)3
G)
H)4
A)
B)1
C)
D)2
E)
F)3
G)
H)4

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
17
Find the minimum value of the function subject to the constraint that .
A)
B)1
C)
D)2
E)
F)3
G)
H)4
A)
B)1
C)
D)2
E)
F)3
G)
H)4

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
18
Find the maximum and minimum values of the function 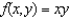 on the ellipse given by the equation
on the ellipse given by the equation 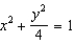 .
.
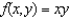 on the ellipse given by the equation
on the ellipse given by the equation 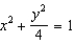 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
19
Find the minimum value of the function subject to the constraint that .
A)
B)
C)
D)
E)4
F)5
G)6
H)7
A)
B)
C)
D)
E)4
F)5
G)6
H)7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
20
Find the minimum value of the function subject to the constraint that .
A)
B)1
C)
D).2
E)
F)
G)
H)
A)
B)1
C)
D).2
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
21
The quality 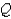 of a good produced by a company is given by
of a good produced by a company is given by 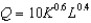 , where
, where 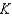 is the quantity of capital and
is the quantity of capital and 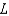 is the quantity of labor used. Capital costs are $20 per unit, labor costs are $10 per unit, and the company wants to keep costs for capital and labor combined to $150.(a) What combination of labor and capital should be used to produce maximum quantity? What is the maximum value?
is the quantity of labor used. Capital costs are $20 per unit, labor costs are $10 per unit, and the company wants to keep costs for capital and labor combined to $150.(a) What combination of labor and capital should be used to produce maximum quantity? What is the maximum value?
(b) Draw the level curves of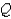 and the graph of the budget constraint on the same set of axes.(c) Complete the value of
and the graph of the budget constraint on the same set of axes.(c) Complete the value of 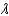 . What does
. What does 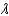 represent?
represent?
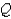 of a good produced by a company is given by
of a good produced by a company is given by 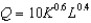 , where
, where 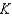 is the quantity of capital and
is the quantity of capital and 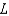 is the quantity of labor used. Capital costs are $20 per unit, labor costs are $10 per unit, and the company wants to keep costs for capital and labor combined to $150.(a) What combination of labor and capital should be used to produce maximum quantity? What is the maximum value?
is the quantity of labor used. Capital costs are $20 per unit, labor costs are $10 per unit, and the company wants to keep costs for capital and labor combined to $150.(a) What combination of labor and capital should be used to produce maximum quantity? What is the maximum value?(b) Draw the level curves of
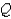 and the graph of the budget constraint on the same set of axes.(c) Complete the value of
and the graph of the budget constraint on the same set of axes.(c) Complete the value of 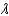 . What does
. What does 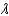 represent?
represent?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
22
Determine how many critical points the function has.
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
23
The function has one critical point. Determine its location and type.
A) , saddle point
B) , minimum point
C) , maximum point
D) , saddle point
E) , minimum point
F) , maximum point
G) , saddle point
H) , minimum point
A) , saddle point
B) , minimum point
C) , maximum point
D) , saddle point
E) , minimum point
F) , maximum point
G) , saddle point
H) , minimum point

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
24
A rancher with 300 ft of fence intends to enclose a rectangular corral, dividing it in half by a fence parallel to the short sides of the corral. What is the maximum area he can enclose? Compute the value of 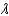 . What does
. What does 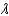 represent?
represent?
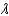 . What does
. What does 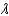 represent?
represent?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
25
Find the maximum value of the function
A)90
B)160
C)50
D)15
E)80
F)135
G)16
H)5
A)90
B)160
C)50
D)15
E)80
F)135
G)16
H)5

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
26
The function has one critical point. Determine its location and type.
A) , saddle point
B) , minimum point
C) , maximum point
D) , saddle point
E) , minimum point
F) , maximum point
G) , saddle point
H) , minimum point
A) , saddle point
B) , minimum point
C) , maximum point
D) , saddle point
E) , minimum point
F) , maximum point
G) , saddle point
H) , minimum point

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
27
The function has one critical point. Determine its location and type.
A) , saddle point
B) , minimum point
C) , maximum point
D) , saddle point
E) , minimum point
F) , maximum point
G) , saddle point
H) , minimum point
A) , saddle point
B) , minimum point
C) , maximum point
D) , saddle point
E) , minimum point
F) , maximum point
G) , saddle point
H) , minimum point

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
28
The level curves of a function 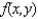 and a curve with equation
and a curve with equation 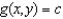 (
( 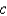 constant) are given below. Estimate the point where
constant) are given below. Estimate the point where 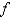 has a maximum value and the point where
has a maximum value and the point where 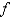 has a minimum value, subject to the constraint that
has a minimum value, subject to the constraint that 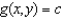 . Indicate your answer in the figure.
. Indicate your answer in the figure. 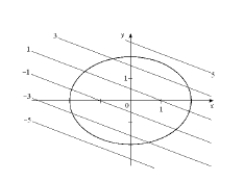
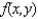 and a curve with equation
and a curve with equation 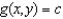 (
( 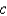 constant) are given below. Estimate the point where
constant) are given below. Estimate the point where  has a maximum value and the point where
has a maximum value and the point where  has a minimum value, subject to the constraint that
has a minimum value, subject to the constraint that 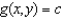 . Indicate your answer in the figure.
. Indicate your answer in the figure. 

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
29
Suppose that the quantity 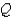 produced of a certain good depends on the number of units of labor
produced of a certain good depends on the number of units of labor 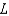 and the quantity of capital
and the quantity of capital 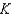 according to the function
according to the function 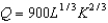 . Suppose also that labor costs $100 per unit and that capital costs $200 per unit.(a) What combination of labor and capital should be used to produce 36,000 units of the goods at minimum cost? What is that minimum cost?
. Suppose also that labor costs $100 per unit and that capital costs $200 per unit.(a) What combination of labor and capital should be used to produce 36,000 units of the goods at minimum cost? What is that minimum cost?
(b) Draw the level curves of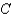 , the cost function, and the graph of the constraint on the same set of axes.(c) Complete the value of
, the cost function, and the graph of the constraint on the same set of axes.(c) Complete the value of 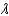 . What does
. What does 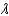 represent?
represent?
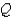 produced of a certain good depends on the number of units of labor
produced of a certain good depends on the number of units of labor 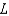 and the quantity of capital
and the quantity of capital 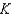 according to the function
according to the function 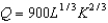 . Suppose also that labor costs $100 per unit and that capital costs $200 per unit.(a) What combination of labor and capital should be used to produce 36,000 units of the goods at minimum cost? What is that minimum cost?
. Suppose also that labor costs $100 per unit and that capital costs $200 per unit.(a) What combination of labor and capital should be used to produce 36,000 units of the goods at minimum cost? What is that minimum cost?(b) Draw the level curves of
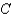 , the cost function, and the graph of the constraint on the same set of axes.(c) Complete the value of
, the cost function, and the graph of the constraint on the same set of axes.(c) Complete the value of 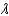 . What does
. What does 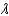 represent?
represent?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
30
What point on the surface 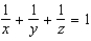 ,
, 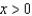 ,
, 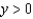 ,
, 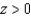 is closest to the origin?
is closest to the origin?
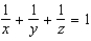 ,
, 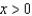 ,
, 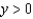 ,
, 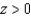 is closest to the origin?
is closest to the origin?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
31
Determine how many critical points the function has.
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
32
A package in the shape of a cylindrical box can be mailed by the U.S. Postal Service if the sum of its height and girth (the circumference of the circular base) is at most 108 in. Find the dimension of the package with largest volume that can be mailed.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
33
Find the minimum value of the function
A)8.5
B)18
C)19
D)10
E)10.5
F)9
G)9.5
H)50
A)8.5
B)18
C)19
D)10
E)10.5
F)9
G)9.5
H)50

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
34
A rectangular area of 3200 ft 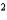 is to be fenced off. Two opposite sides will use fencing costing $1 per foot and the remaining sides will use fencing costing $2 per foot. Find the dimensions of the rectangle of least cost. Compute the value of
is to be fenced off. Two opposite sides will use fencing costing $1 per foot and the remaining sides will use fencing costing $2 per foot. Find the dimensions of the rectangle of least cost. Compute the value of 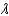 . What does
. What does 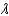 represent?
represent?
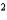 is to be fenced off. Two opposite sides will use fencing costing $1 per foot and the remaining sides will use fencing costing $2 per foot. Find the dimensions of the rectangle of least cost. Compute the value of
is to be fenced off. Two opposite sides will use fencing costing $1 per foot and the remaining sides will use fencing costing $2 per foot. Find the dimensions of the rectangle of least cost. Compute the value of 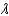 . What does
. What does 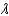 represent?
represent?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
35
The function has one critical point. Determine its location and type.
A) , saddle point
B) , minimum point
C) , maximum point
D) , saddle point
E) , minimum point
F) , maximum point
G) , saddle point
H) , minimum point
A) , saddle point
B) , minimum point
C) , maximum point
D) , saddle point
E) , minimum point
F) , maximum point
G) , saddle point
H) , minimum point

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
36
A rancher intends to fence off a rectangular region along a river (which serves as a natural boundary requiring no fence). If the enclosed area is to be 1800 square yards, what is the least amount of fence needed? Compute the value of 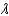 . What does
. What does 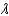 represent?
represent?
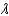 . What does
. What does 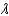 represent?
represent?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
37
Determine how many critical points the function has.
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
38
Determine how many critical points the function has.
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
39
Find the maximum and minimum values of 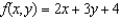 on the circle
on the circle 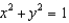 .
.
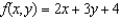 on the circle
on the circle 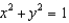 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
40
Determine how many critical points the function has.
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
41
Find the local maximum and minimum values and saddle points of the function 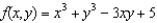 .
.
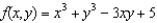 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
42
Find the local maximum and minimum values and saddle points of the function 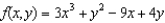 .
.
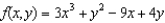 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
43
Find the point at which the function has a local maximum.
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
44
For each of the following functions, find the critical point, if there is one, and determine if it is a local maximum, local minimum, saddle point, or otherwise.(a) 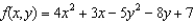 (b)
(b) 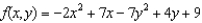 (c)
(c) 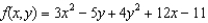 (d)
(d) 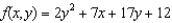
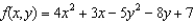 (b)
(b) 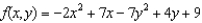 (c)
(c) 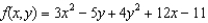 (d)
(d) 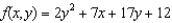

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
45
Use the level curves of 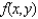 shown below to estimate the critical points of
shown below to estimate the critical points of 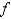 . Indicate whether
. Indicate whether 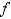 has a saddle point or a local maximum or minimum at each of those points.
has a saddle point or a local maximum or minimum at each of those points. 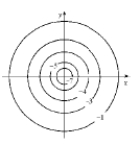
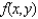 shown below to estimate the critical points of
shown below to estimate the critical points of 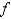 . Indicate whether
. Indicate whether 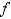 has a saddle point or a local maximum or minimum at each of those points.
has a saddle point or a local maximum or minimum at each of those points. 

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
46
A cardboard box without a lid is to have volume 100 cubic inches, with total area of cardboard as small as possible. Find its height in inches.
A)2
B)
C)4
D)5
E)
F)
G)
H)
A)2
B)
C)4
D)5
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
47
Find the local maximum and minimum values and saddle points of the function 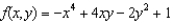 .
.
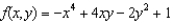 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
48
Find the local maximum and minimum values and saddle points of the function 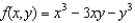 .
.
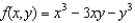 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
49
The function 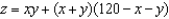 has a maximum. Find the values of
has a maximum. Find the values of 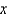 and
and 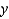 at which it occurs.
at which it occurs.
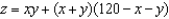 has a maximum. Find the values of
has a maximum. Find the values of 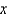 and
and 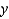 at which it occurs.
at which it occurs.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
50
Use the level curves of 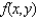 shown below to estimate the critical points of
shown below to estimate the critical points of 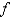 . Indicate whether
. Indicate whether  has a saddle point or a local maximum or minimum at each of those points.
has a saddle point or a local maximum or minimum at each of those points. 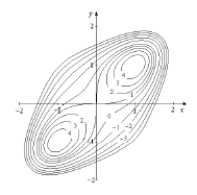
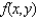 shown below to estimate the critical points of
shown below to estimate the critical points of 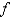 . Indicate whether
. Indicate whether  has a saddle point or a local maximum or minimum at each of those points.
has a saddle point or a local maximum or minimum at each of those points. 

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
51
Find the local maximum and minimum values and saddle points of the function 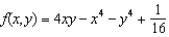 .
.
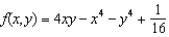 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
52
Compare the minimum value of 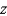 and sketch a portion of the graph of
and sketch a portion of the graph of 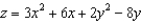 near its lowest point.
near its lowest point. 
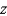 and sketch a portion of the graph of
and sketch a portion of the graph of 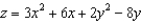 near its lowest point.
near its lowest point. 

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
53
Find the absolute maximum and minimum values of 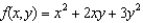 over the region
over the region 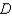 , where
, where 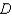 is a closed triangular region with vertices
is a closed triangular region with vertices 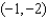 ,
,  , and
, and 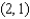 .
.
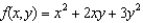 over the region
over the region 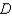 , where
, where 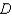 is a closed triangular region with vertices
is a closed triangular region with vertices 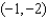 ,
,  , and
, and 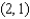 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
54
Find three positive numbers 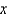 ,
, 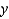 , and
, and  whose sum is 48 and product is maximum.
whose sum is 48 and product is maximum.
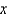 ,
, 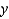 , and
, and 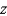 whose sum is 48 and product is maximum.
whose sum is 48 and product is maximum.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
55
Find the shortest distance from the origin to the surface .
A)
B)
C)1
D)
E)2
F)
G)4
H)
A)
B)
C)1
D)
E)2
F)
G)4
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
56
Find the critical points (if any) for 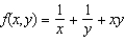 and determine if each is a local extreme value or a saddle point.
and determine if each is a local extreme value or a saddle point.
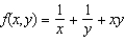 and determine if each is a local extreme value or a saddle point.
and determine if each is a local extreme value or a saddle point.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
57
Find the local maximum and minimum values and saddle points of the function 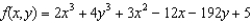 .
.
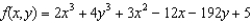 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
58
Find the absolute maximum and minimum value of 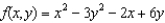 on the square region
on the square region 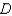 with vertices
with vertices 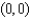 ,
, 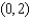 ,
, 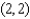 , and
, and 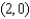 .
.
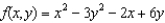 on the square region
on the square region 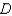 with vertices
with vertices 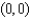 ,
, 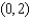 ,
, 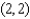 , and
, and 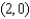 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
59
Find the absolute maximum and minimum value of  on the square region
on the square region 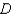 with vertices
with vertices 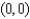 ,
, 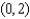 ,
, 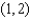 , and
, and 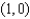 .
.
 on the square region
on the square region 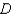 with vertices
with vertices 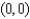 ,
, 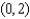 ,
, 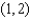 , and
, and 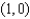 .
.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
60
A company estimates that its annual profits for next year will be 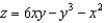 , where
, where 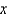 represents investment in research and
represents investment in research and 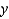 represents investment in labor. All units are in tens of thousands of dollars. Determine the amount the company should spend on research and on labor to maximize its profit. What is maximum profit?
represents investment in labor. All units are in tens of thousands of dollars. Determine the amount the company should spend on research and on labor to maximize its profit. What is maximum profit?
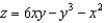 , where
, where 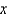 represents investment in research and
represents investment in research and 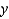 represents investment in labor. All units are in tens of thousands of dollars. Determine the amount the company should spend on research and on labor to maximize its profit. What is maximum profit?
represents investment in labor. All units are in tens of thousands of dollars. Determine the amount the company should spend on research and on labor to maximize its profit. What is maximum profit?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
61
Find an equation of the tangent plane to the surface at the point .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
62
Let . Find the gradient vector at the point .
A)i
B)j
C)k
D)
E)
F)
G)
H)0
A)i
B)j
C)k
D)
E)
F)
G)
H)0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
63
Find the directional derivative of the function at the point in the direction from toward the point ..
A)
B)
C)
D)
E)7
F)
G)
H)8
A)
B)
C)
D)
E)7
F)
G)
H)8

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
64
Find the largest value of the directional derivative of the function at the point .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
65
Let . Find the gradient vector .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
66
Find a normal vector to the surface at the point .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
67
Let . Find the gradient vector .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
68
Find the directional derivative of the function at the point in the direction .
A)
B)0
C)
D)12
E)1
F)
G)
H)
A)
B)0
C)
D)12
E)1
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
69
Find the direction of maximum increase of the function at the point .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
70
Find the second directional derivative of at the point in the direction .
A)
B)
C)2
D)6
E)
F)
G)
H)
A)
B)
C)2
D)6
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
71
Find three positive numbers 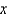 ,
, 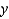 , and
, and 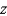 whose product is 343 and sum is minimum.
whose product is 343 and sum is minimum.
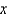 ,
, 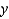 , and
, and 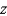 whose product is 343 and sum is minimum.
whose product is 343 and sum is minimum.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
72
Let . Find the gradient vector at the point .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
73
Find an equation of the tangent plane to the hyperboloid at the point .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
74
Find the directional derivative of the function at the point in the direction .
A)
B)
C)
D)
E)1
F)2
G)4
H)
A)
B)
C)
D)
E)1
F)2
G)4
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
75
Find the second directional derivative of at the point in the direction .
A)
B)
C)
D)14
E)
F)
G)
H)
A)
B)
C)
D)14
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
76
Find the direction in which the directional derivative of the function at the point is maximum.
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
77
Find the direction of maximum increase of the function at the point .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
78
Find an equation of the tangent plane to the surface at the point .
A)
B)
C)
D)
E)
F)
G)
H)
A)
B)
C)
D)
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
79
Find the directional derivative of the function at the point in the direction of the vector .
A)
B)
C)
D)0
E)
F)
G)
H)
A)
B)
C)
D)0
E)
F)
G)
H)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck
80
Find the directional derivative of the function at the point in the direction .
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7
A)0
B)1
C)2
D)3
E)4
F)5
G)6
H)7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 294 في هذه المجموعة.
فتح الحزمة
k this deck








