Provide the requested proof.
-In the trapezoid below, and . If is the midpoint of , prove that .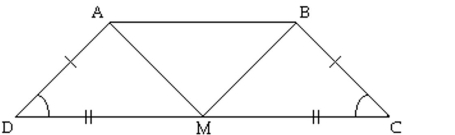
Correct Answer:
Verified
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Q3: Provide the requested proof.
-
Q4: Provide the requested proof.
-
Q5: Provide an appropriate response.
-
Q6: Provide the requested proof.
-
Q7: Provide the requested proof.
-Let
Q9: Use a ruler, protractor, and compass to
Q10: Provide the requested proof.
-
Q11: Provide the requested proof.
-
Q12: Provide the requested proof.
-Let
Q13: Provide the requested proof.
-In the figure
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents