The growth rate of the bacterium, a common bacterium found in the human intestine, is proportional to its size. Under ideal laboratory conditions, when this bacterium is grown in a nutrient broth medium, the number of cells in a culture doubles approximately every 20 min. If the initial cell population is 10, determine the function Q(t) that expresses the exponential growth of the number of cells of this bacterium as a function of time t (in minutes) . How long will it take for a colony of 10 cells to increase to a population of 1 million? If the initial cell population were 100, how would this alter the model?
A) 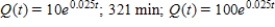
B) 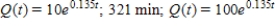
C) 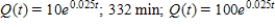
D) 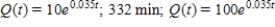
Correct Answer:
Verified
Q9: Given that a quantity Q(t) exhibiting exponential
Q10: Phosphorus 32 has a half-life of 14.2
Q11: Given that a quantity Q(t) is described
Q12: The U.S. population is approximated by the
Q13: The radioactive element polonium decays according to
Q15: During a flu epidemic, the number of
Q16: According to estimates by Paul Kroger Associates,
Q17: Wood deposits recovered from an archeological site
Q18: Strontium 90, a radioactive isotope of strontium,
Q19: On the basis of data collected during
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents