Solved

A Covariance Stationary Time Series Is Weakly Dependent If

Question 10

Multiple Choice

A covariance stationary time series is weakly dependent if:


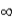A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to A covariance stationary time series is weakly dependent if: A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to as h 0. B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h . C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h . D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to as h . 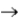as h A covariance stationary time series is weakly dependent if: A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to as h 0. B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h . C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h . D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to as h . 0.
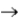
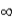B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h A covariance stationary time series is weakly dependent if: A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to as h 0. B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h . C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h . D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to as h . 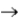A covariance stationary time series is weakly dependent if: A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to as h 0. B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h . C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h . D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to as h . .
C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h
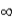A covariance stationary time series is weakly dependent if: A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to as h 0. B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h . C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h . D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to as h .
A covariance stationary time series is weakly dependent if: A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to as h 0. B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h . C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h . D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to as h . .
D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to
A covariance stationary time series is weakly dependent if: A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to as h 0. B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h . C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h . D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to as h . as h
A covariance stationary time series is weakly dependent if: A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to as h 0. B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h . C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h . D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to as h .
A covariance stationary time series is weakly dependent if: A) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to as h 0. B) the correlation between the independent variable at time 't' and the dependent variable at time 't + h' goes to 0 as h . C) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to 0 as h . D) the correlation between the independent variable at time 't' and the independent variable at time 't + h' goes to as h . .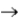

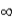Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q2: If a process is said to be

Q4: A process is stationary if:
A)any collection of

Q5: Suppose ut is the error term for

Q6: Which of the following statements is true

Q7: The model yt = et +

Q12: Weakly dependent processes are said to be

Q13: Which of the following statements is true?
A)A

Q13: Consider the model: yt =

Q14: If ut refers to the error term

Q15: Unit root processes, such as a random

Unlock this Answer For Free Now!

View this answer and more for free by performing one of the following actions

qr-code

Scan the QR code to install the App and get 2 free unlocks

upload documents

Unlock quizzes for free by uploading documents