There Is an Old Saying in Golf: "You Drive for Show

There is an old saying in golf: "You drive for show and you putt for dough." The point is that good putting is more important than long driving for shooting low scores and hence winning money.To see if this is the case,data on the top 69 money winners on the PGA tour in 1993 are examined.The average number of putts per hole for each player is used to predict the total winnings (in thousands of dollars) using the simple linear regression model (1993 winnings) _i = $\beta$ ₀ + $\beta$ ₁(average number of putts per hole) _i + $\varepsilon$ _i,
Where the deviations $\varepsilon$ _i are assumed to be independent and Normally distributed with a mean of 0 and a standard deviation of $\sigma$ .This model was fit to the data using the method of least squares.The following results were obtained from statistical software. 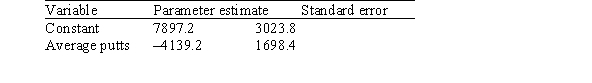Which of the following conclusions seems most justified?

A) There is no evidence of a relation between the average number of putts per round and the 1993 winnings of PGA tour pros.
B) There is distinct evidence (P-value less than 0.05) that there is a positive correlation between 1993 winnings and average number of putts per round.
C) There is some evidence that PGA tour pros who averaged fewer putts per round had higher winnings in 1993.
D) The presence of strongly influential observations in these data makes it impossible to draw any conclusions about the relationship between 1993 winnings and average number of putts per round.