A company manufactures products A, B, and C. Each product is processed in three departments: I, II, and III. The total available labor-hours per week for departments I, II, and III are 930, 1,060, and 840, respectively. The time requirements (in hours/unit) and the profit per unit for each product are as follows: 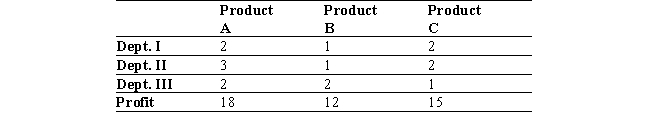 If management decides that the number of units of product B manufactured must equal or exceed the number of units of products A and C manufactured, how many units of each product should the company produce in order to maximize its profit?
If management decides that the number of units of product B manufactured must equal or exceed the number of units of products A and C manufactured, how many units of each product should the company produce in order to maximize its profit?
A) The company should produce 280 units of product A, 0 units of product B, and 280 units of product C to realize a maximum profit of $9,240.
B) The company should produce 0 units of product A, 280 units of product B, and 280 units of product C to realize a maximum profit of $7,56.0
C) The company should produce 0 units of product A, 280 units of product B, and 280 units of product C to realize a maximum profit of $7,560.
Correct Answer:
Verified
Q76: Use the simplex method for solving nonstandard
Q77: The owner of the Health JuiceBar wishes
Q78: The owner of the Health JuiceBar wishes
Q79: Solve the linear programming problem by the
Q80: Determine whether the statement is true or
Q82: Rewrite the linear programming problem as a
Q83: Use the simplex method for solving nonstandard
Q84: Use the Simplex Method for Solving Nonstandard
Q85: Use the simplex method for solving nonstandard
Q86: A farmer has 150 acres of land
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents