Deck 9: Hypothesis Testing
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/87
Play
Full screen (f)
Deck 9: Hypothesis Testing
1
A teacher who is trying to prove that a new method of teaching economics is more effective than a traditional one will conduct a:
A) one-tailed test
B) two-tailed test
C) point estimate of the population parameter
D) confidence interval
A) one-tailed test
B) two-tailed test
C) point estimate of the population parameter
D) confidence interval
one-tailed test
2
A two-tailed test is one where:
A) results in only one direction can lead to rejection of the null hypothesis
B) negative sample means lead to rejection of the null hypothesis
C) results in either of two directions can lead to rejection of the null hypothesis
D) no results lead to the rejection of the null hypothesis
A) results in only one direction can lead to rejection of the null hypothesis
B) negative sample means lead to rejection of the null hypothesis
C) results in either of two directions can lead to rejection of the null hypothesis
D) no results lead to the rejection of the null hypothesis
results in either of two directions can lead to rejection of the null hypothesis
3
Which of the following values is not typically used for 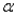 ?
?
A) 0.01
B) 0.05
C) 0.10
D) 0.50
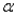 ?
?A) 0.01
B) 0.05
C) 0.10
D) 0.50
0.50
4
The form of the alternative hypothesis can be:
A) one-tailed only
B) two-tailed only
C) neither one nor two-tailed
D) one-tailed or two-tailed
A) one-tailed only
B) two-tailed only
C) neither one nor two-tailed
D) one-tailed or two-tailed

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
5
The alternative hypothesis is also known as the:
A) elective hypothesis
B) optional hypothesis
C) research hypothesis
D) null hypothesis
A) elective hypothesis
B) optional hypothesis
C) research hypothesis
D) null hypothesis

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
6
A null hypothesis is a statement about the value of a population parameter. It is usually the current thinking, or "status quo".

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
7
One-tailed alternative hypotheses are phrased in terms of:
A) ≠
B) < or >
C) =
D)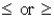
A) ≠
B) < or >
C) =
D)
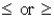

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
8
Smaller p-values indicate more evidence in support of:
A) the null hypothesis
B) the alternative hypothesis
C) the quality of the researcher
D) none of these choices
A) the null hypothesis
B) the alternative hypothesis
C) the quality of the researcher
D) none of these choices

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
9
The p-value of a sample is the probability of seeing a sample with
A) at most as much evidence in favor of the null hypothesis as the sample actually observed.
B) at most as much evidence in favor of the alternative hypothesis as the sample actually observed.
C) at least as much evidence in favor of the null hypothesis as the sample actually observed.
D) at least as much evidence in favor of the alternative hypothesis as the sample actually observed.
A) at most as much evidence in favor of the null hypothesis as the sample actually observed.
B) at most as much evidence in favor of the alternative hypothesis as the sample actually observed.
C) at least as much evidence in favor of the null hypothesis as the sample actually observed.
D) at least as much evidence in favor of the alternative hypothesis as the sample actually observed.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
10
Which of the following statements are true of the null and alternative hypotheses?
A) Exactly one hypothesis must be true.
B) Both hypotheses must be true.
C) It is possible for both hypotheses to be true.
D) It is possible for neither hypothesis to be true.
A) Exactly one hypothesis must be true.
B) Both hypotheses must be true.
C) It is possible for both hypotheses to be true.
D) It is possible for neither hypothesis to be true.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
11
Of type I and type II error, which is traditionally regarded as more serious?
A) Type I is considered to be more serious.
B) Type II is considered to be more serious.
C) Type I and Type II are equally serious.
D) Neither Type I or Type II is serious and both can be avoided.
A) Type I is considered to be more serious.
B) Type II is considered to be more serious.
C) Type I and Type II are equally serious.
D) Neither Type I or Type II is serious and both can be avoided.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
12
The value set for 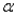 is known as the:
is known as the:
A) rejection level
B) acceptance level
C) significance level
D) error in the hypothesis test
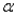 is known as the:
is known as the:A) rejection level
B) acceptance level
C) significance level
D) error in the hypothesis test

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
13
The = sign belongs in the null hypothesis.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
14
The null and alternative hypotheses divide all possibilities into:
A) two sets that overlap
B) two non-overlapping sets
C) two sets that may or may not overlap
D) as many sets as necessary to cover all possibilities
A) two sets that overlap
B) two non-overlapping sets
C) two sets that may or may not overlap
D) as many sets as necessary to cover all possibilities

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
15
In statistical analysis, the burden of proof lies traditionally with the:
A) alternative hypothesis
B) null hypothesis
C) analyst
D) facts presented to the statistical analyst
A) alternative hypothesis
B) null hypothesis
C) analyst
D) facts presented to the statistical analyst

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
16
A type II error occurs when:
A) the null hypothesis is incorrectly accepted when it is false
B) the null hypothesis is incorrectly rejected when it is true
C) the sample mean differs from the population mean
D) the test procedure itself is fundamentally biased
A) the null hypothesis is incorrectly accepted when it is false
B) the null hypothesis is incorrectly rejected when it is true
C) the sample mean differs from the population mean
D) the test procedure itself is fundamentally biased

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
17
The hypothesis that an analyst is trying to prove is called the:
A) elective hypothesis
B) alternative hypothesis
C) optional hypothesis
D) null hypothesis
A) elective hypothesis
B) alternative hypothesis
C) optional hypothesis
D) null hypothesis

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
18
A p-value is considered "convincing" if it is:
A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10
A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
19
The null hypothesis usually represents the:
A) theory the researcher would like to prove
B) preconceived ideas of the researcher
C) perceptions of the sample population
D) status quo of the situation being studied
A) theory the researcher would like to prove
B) preconceived ideas of the researcher
C) perceptions of the sample population
D) status quo of the situation being studied

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
20
A null hypothesis can only be rejected at the 5% significance level if and only if:
A) a 95% confidence interval includes the hypothesized value of the parameter
B) a 95% confidence interval does not include the hypothesized value of the parameter
C) the null hypothesis is biased
D) the null hypotheses includes sampling error
A) a 95% confidence interval includes the hypothesized value of the parameter
B) a 95% confidence interval does not include the hypothesized value of the parameter
C) the null hypothesis is biased
D) the null hypotheses includes sampling error

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
21
Larger p-values indicate more evidence in support of the:
A) null hypothesis
B) alternative hypothesis
C) quality of the researcher
D) none of these choices
A) null hypothesis
B) alternative hypothesis
C) quality of the researcher
D) none of these choices

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
22
The significance level 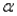 also determines the rejection region.
also determines the rejection region.
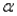 also determines the rejection region.
also determines the rejection region.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
23
The p-value of a test is the probability of observing a test statistic at least as extreme as the one computed given that the null hypothesis is true.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
24
The power of a test is the probability that we:
A) reject the null hypothesis when the alternative hypothesis is false
B) reject the null hypothesis when the alternative hypothesis is true
C) accept the null hypothesis when the alternative hypothesis is false
D) accept the null hypothesis when the alternative hypothesis is true
A) reject the null hypothesis when the alternative hypothesis is false
B) reject the null hypothesis when the alternative hypothesis is true
C) accept the null hypothesis when the alternative hypothesis is false
D) accept the null hypothesis when the alternative hypothesis is true

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
25
Which of the following values is typically used for 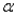 ?
?
A) 0.50
B) 0.40
C) 0.30
D) 0.10
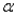 ?
?A) 0.50
B) 0.40
C) 0.30
D) 0.10

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
26
Type I errors are usually considered more "costly" although this can lead to conservative decision making.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
27
If a null hypothesis about a population mean 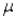 is rejected at the 0.025 level of significance, then it must also be rejected at the 0.01 level.
is rejected at the 0.025 level of significance, then it must also be rejected at the 0.01 level.
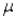 is rejected at the 0.025 level of significance, then it must also be rejected at the 0.01 level.
is rejected at the 0.025 level of significance, then it must also be rejected at the 0.01 level.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
28
A one-tailed alternative is one that is supported by evidence in either direction.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
29
A Type I error probability is represented by 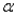 ; it is the probability of incorrectly rejecting a null hypothesis that is true.
; it is the probability of incorrectly rejecting a null hypothesis that is true.
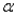 ; it is the probability of incorrectly rejecting a null hypothesis that is true.
; it is the probability of incorrectly rejecting a null hypothesis that is true.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
30
The analyst gets to choose the significance level 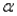 . It is typically chosen to be 0.50, but it is occasionally chosen to be 0.05.
. It is typically chosen to be 0.50, but it is occasionally chosen to be 0.05.
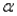 . It is typically chosen to be 0.50, but it is occasionally chosen to be 0.05.
. It is typically chosen to be 0.50, but it is occasionally chosen to be 0.05.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
31
A Type II error is committed when we incorrectly accept an alternative hypothesis that is false.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
32
Sample evidence is statistically significant at the 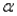 level only if the p-value is larger than
level only if the p-value is larger than 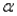 .
.
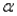 level only if the p-value is larger than
level only if the p-value is larger than 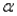 .
.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
33
The probability of making a Type I error and the level of significance 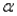 are the same.
are the same.
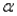 are the same.
are the same.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
34
The rejection region is the set of sample data that leads to the rejection of the alternative hypothesis.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
35
Which sign is possible in an alternative hypothesis?
A) >
B) <
C) ≠
D) All of these signs are possible
A) >
B) <
C) ≠
D) All of these signs are possible

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
36
The p-value of a test is the smallest level of significance 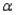 at which the null hypothesis can be rejected.
at which the null hypothesis can be rejected.
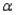 at which the null hypothesis can be rejected.
at which the null hypothesis can be rejected.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
37
A low p-value provides evidence for accepting the null hypothesis and rejecting the alternative.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
38
A type I error occurs when the:
A) null hypothesis is incorrectly accepted when it is false
B) null hypothesis is incorrectly rejected when it is true
C) sample mean differs from the population mean
D) test procedure itself is fundamentally biased
A) null hypothesis is incorrectly accepted when it is false
B) null hypothesis is incorrectly rejected when it is true
C) sample mean differs from the population mean
D) test procedure itself is fundamentally biased

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
39
An alternative hypothesis can have the signs >, <, or ≠.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
40
A professor of statistics refutes the claim that the proportion of Republican voters in Michigan is at most 45%. To test the claim, the hypotheses: 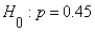 ,
, 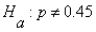 , should be used.
, should be used.
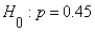 ,
, 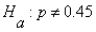 , should be used.
, should be used.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
41
If, instead, the test is conducted at the 0.10 significance level, would the decision regarding the salaries change? Explain why or why not. Answer this question without re-running the hypothesis test.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
42
The test statistic for a hypothesis test of a population proportion is the z-value.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
43
A null hypothesis can only be rejected at the 10% significance level if and only if:
A) a 90% confidence interval includes the hypothesized value of the parameter
B) a 90% confidence interval does not include the hypothesized value of the parameter
C) the null hypothesis is biased
D) the null hypotheses includes sampling error
A) a 90% confidence interval includes the hypothesized value of the parameter
B) a 90% confidence interval does not include the hypothesized value of the parameter
C) the null hypothesis is biased
D) the null hypotheses includes sampling error

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
44
Side-by-side box-plots are typically a good way to begin the analysis when comparing two populations.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
45
If, instead, the variances in the underlying populations are equal, would the decision regarding the salaries change? Explain why or why not. Answer this question without re-running the hypothesis test.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
46
The F distribution is a skewed distribution useful for testing equality of variances.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
47
(A) Assume that the national average weekly grocery bill for a five-person family is $131. Is the sample evidence statistically significant? If so, at what significance levels can you reject the null hypothesis?
(B) For which values of the sample mean (i.e., average weekly grocery bill) would you decide to reject the null hypothesis at the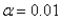 significance level? For which values of the sample mean would you decide to reject the null hypothesis at the 10% level of significance?
significance level? For which values of the sample mean would you decide to reject the null hypothesis at the 10% level of significance?
(B) For which values of the sample mean (i.e., average weekly grocery bill) would you decide to reject the null hypothesis at the
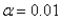 significance level? For which values of the sample mean would you decide to reject the null hypothesis at the 10% level of significance?
significance level? For which values of the sample mean would you decide to reject the null hypothesis at the 10% level of significance?
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
48
A test with a 0.05 significance level has a larger rejection region than a test with a 0.01 significance level.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
49
A marketing research consultant hired by Red Bull® is interested in determining if the proportion of customers who prefer Red Bull® to other energy drink brands is over 50%. A random sample of 200 consumers was selected from the market under investigation, 55% favored Red Bull® over other brands. Additional information is presented below. 
(A) If you were to conduct a hypothesis test to determine if greater than 50% of customers prefer Red Bull® to other brands, would you conduct a one-tail or a two-tail hypothesis test? Explain your answer.
(B) How many customers out of the 200 sampled must have favored Red Bull® in this case?
(C) Using a 5% significance level, can the marketing consultant conclude that the proportion of customers who prefer Red Bull® exceeds 50%? Explain your answer.
(D) If you were to use a 1% significance level, would the conclusion from (C) change? Explain your answer.

(A) If you were to conduct a hypothesis test to determine if greater than 50% of customers prefer Red Bull® to other brands, would you conduct a one-tail or a two-tail hypothesis test? Explain your answer.
(B) How many customers out of the 200 sampled must have favored Red Bull® in this case?
(C) Using a 5% significance level, can the marketing consultant conclude that the proportion of customers who prefer Red Bull® exceeds 50%? Explain your answer.
(D) If you were to use a 1% significance level, would the conclusion from (C) change? Explain your answer.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
50
When testing the equality of two population variances, the test statistic is the ratio of the population variances; namely 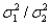 .
.
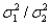 .
.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
51
The test statistic employed to test 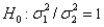 is
is 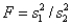 , which is F distributed with
, which is F distributed with 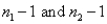 degrees of freedom.
degrees of freedom.
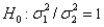 is
is 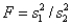 , which is F distributed with
, which is F distributed with 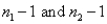 degrees of freedom.
degrees of freedom.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
52
Do graduates of undergraduate business programs with different majors tend to earn disparate starting salaries? Below you will find output from an analysis for randomly selected graduates with majors in accounting (Acct) and marketing (Mktg). 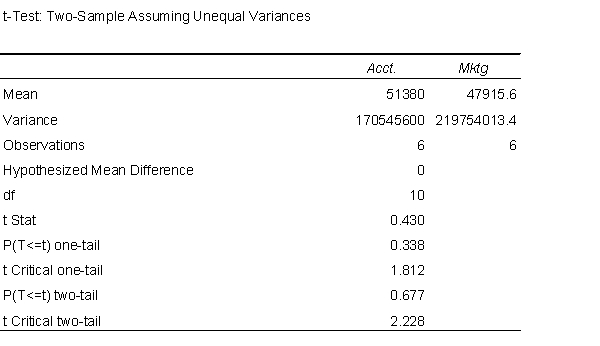
Assuming that the variances of the underlying populations are unequal, can you reject at a 5% significance level that the mean starting salaries for accounting and marketing majors are the same? Explain why or why not.

Assuming that the variances of the underlying populations are unequal, can you reject at a 5% significance level that the mean starting salaries for accounting and marketing majors are the same? Explain why or why not.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
53
The test statistic in a hypothesis test for a population proportion is the:
A) t-value calculated from the sample
B) z-value calculated from the sample
C) F-value calculated from the sample
D) the sample proportion
A) t-value calculated from the sample
B) z-value calculated from the sample
C) F-value calculated from the sample
D) the sample proportion

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
54
The power of a test is the probability of rejecting the null hypothesis when the alternative hypothesis is true.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
55
(A) Specify a hypothesis test to test the foundation's claim
(B) Compared to the most recent survey, is this sample evidence statistically significant at the 10% level?
(C) Compared to the most recent survey, is this sample evidence statistically significant at the 5% level?
(D) Report and interpret the p-value for this test
(E) Interpret the overall test for the foundation.
(B) Compared to the most recent survey, is this sample evidence statistically significant at the 10% level?
(C) Compared to the most recent survey, is this sample evidence statistically significant at the 5% level?
(D) Report and interpret the p-value for this test
(E) Interpret the overall test for the foundation.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
56
Suppose a firm that produces light bulbs wants to know whether it can say that its light bulbs typically last more than 1500 hours. Hoping to find support for their claim, the firm collects a random sample and records the lifetime (in hours) of each bulb. The information related to the hypothesis test is presented below. 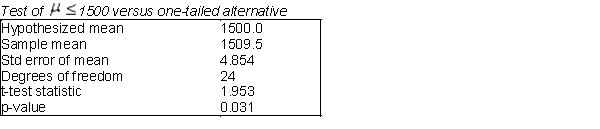
(A) Can the sample size be determined from the information above? Yes or no? If yes, what is the sample size in this case?
(B) The firm believes that the mean life is actually greater than 1500 hours, should you conduct a one-tailed or a two-tailed hypothesis test? Explain your answer, and state the appropriate null and alternative hypotheses.
(C) What is the sample mean of this data? If you use a 5% significance level, would you conclude that the mean life of the light bulbs is typically more than 1500 hours? Explain your answer.
(D) If you were to use a 1% significance level in this case, would you conclude that the mean life of the light bulbs is typically more than 1500 hours? Explain your answer.

(A) Can the sample size be determined from the information above? Yes or no? If yes, what is the sample size in this case?
(B) The firm believes that the mean life is actually greater than 1500 hours, should you conduct a one-tailed or a two-tailed hypothesis test? Explain your answer, and state the appropriate null and alternative hypotheses.
(C) What is the sample mean of this data? If you use a 5% significance level, would you conclude that the mean life of the light bulbs is typically more than 1500 hours? Explain your answer.
(D) If you were to use a 1% significance level in this case, would you conclude that the mean life of the light bulbs is typically more than 1500 hours? Explain your answer.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
57
A test with a 0.10 significance level has a larger rejection region than a test with a 0.05 significance level.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
58
In conducting hypothesis testing for difference between two means when samples are dependent (paired samples), the variable under consideration is 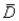 ; the sample mean difference between the pairs.
; the sample mean difference between the pairs.
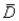 ; the sample mean difference between the pairs.
; the sample mean difference between the pairs.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
59
The retailing manager of Meijer supermarket chain in Michigan wants to determine whether product location has any effect on the sale of children toys. Two different aisle locations are considered: front and rear. A random sample of 18 stores is selected, with 6 stores randomly assigned to each aisle location. The size of the display area and price of the product are constant for all the stores. At the end of one-month trial period, the sales volumes (in thousands of dollars) of the product in each store are as shown below: 
At the 0.05 level of significance, is there evidence of a significant difference in average sales among the various aisle locations?

At the 0.05 level of significance, is there evidence of a significant difference in average sales among the various aisle locations?

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
60
(A) State the appropriate null and alternative hypotheses in this situation.
(B) Test this CEO's belief at the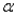 =.05 significance level and report the p-value. Do you find statistical support for his hypothesis that the proportion of women in similar sales positions across the country is less than 40%?
=.05 significance level and report the p-value. Do you find statistical support for his hypothesis that the proportion of women in similar sales positions across the country is less than 40%?
(C) Suppose the sample size above is 100, instead of 50, and the sample proportion is again 0.36. Would this change your results? Explain your answer.
(B) Test this CEO's belief at the
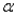 =.05 significance level and report the p-value. Do you find statistical support for his hypothesis that the proportion of women in similar sales positions across the country is less than 40%?
=.05 significance level and report the p-value. Do you find statistical support for his hypothesis that the proportion of women in similar sales positions across the country is less than 40%?
(C) Suppose the sample size above is 100, instead of 50, and the sample proportion is again 0.36. Would this change your results? Explain your answer.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
61
Do undergraduate business students who major in information systems (IS) earn, on average, higher annual starting salaries than their peers who major in marketing (Mktg)? To address this question with a statistical hypothesis test, a comparison should be done to determine whether the variances of annual starting salaries of the two types of majors are equal. Below you will find output from a test of 20 randomly selected IS majors and 20 randomly selected Mktg majors. 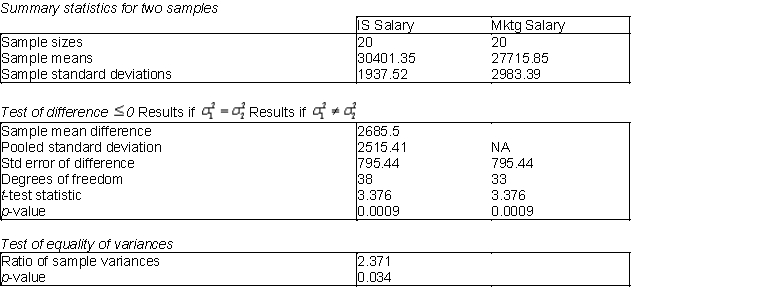
(A) Use the information above to perform the test of equal variance. Explain how the ratio of sample variances is calculated. What type of distribution is used to test for equal variances? Also, would you conclude that the variances are equal or not? Explain.
(B) Based on your conclusion in (A), which test statistic should be used in performing a test for the existence of a difference between population means?
(C) Using a 5% level of significance, is there sufficient evidence to conclude that IS majors earn, on average, a higher annual starting salaries than their peers who major in Mktg?
(D) Using a 1% level of significance, is there sufficient evidence to conclude that IS majors earn, on average, a higher annual starting salaries than their peers who major in Mktg? Explain your answer.

(A) Use the information above to perform the test of equal variance. Explain how the ratio of sample variances is calculated. What type of distribution is used to test for equal variances? Also, would you conclude that the variances are equal or not? Explain.
(B) Based on your conclusion in (A), which test statistic should be used in performing a test for the existence of a difference between population means?
(C) Using a 5% level of significance, is there sufficient evidence to conclude that IS majors earn, on average, a higher annual starting salaries than their peers who major in Mktg?
(D) Using a 1% level of significance, is there sufficient evidence to conclude that IS majors earn, on average, a higher annual starting salaries than their peers who major in Mktg? Explain your answer.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
62
An informal test for normality that utilizes a scatterplot and looks for clustering around a 45° line is known as a(n):
A) Lilliefors test
B) empirical cumulative distribution function
C) p-test
D) quantile-quantile plot
A) Lilliefors test
B) empirical cumulative distribution function
C) p-test
D) quantile-quantile plot

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
63
The chi-square test for normality makes a comparison between the observed histogram and a histogram based on normality.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
64
In a survey of 1,500 customers who did holiday shopping on line during the 2000 holiday season, 270 indicated that they were not satisfied with their experience. Of the customers that were not satisfied, 143 indicated that they did not receive the products in time for the holidays, while 1,197 of the customers that were satisfied with their experience indicated that they did receive the products in time for the holidays. The following complete summary of results were reported: 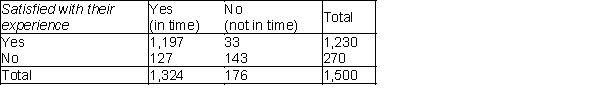
(A) Is there a significant difference in satisfaction between those who received their products in time for the holidays, and those who did not receive their products in time for the holidays? Test at the 0.01 level of significance.
(B) Find the p-value associated with the test in part (A) and interpret its meaning.
(C) Based on the results of (A) and (B), if you were the marketing director of a company selling products online, what would you do to improve the satisfaction of the customers?

(A) Is there a significant difference in satisfaction between those who received their products in time for the holidays, and those who did not receive their products in time for the holidays? Test at the 0.01 level of significance.
(B) Find the p-value associated with the test in part (A) and interpret its meaning.
(C) Based on the results of (A) and (B), if you were the marketing director of a company selling products online, what would you do to improve the satisfaction of the customers?

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
65
The Lilliefors test is used to test for normality.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
66
Which of the following statements is true regarding the chi-square goodness-of-fit test for normality?
A) The test does depend on which and how many categories we use for the histogram.
B) The test is not very effective unless the sample size is large, say, at least 80 or 100.
C) The test tends to be too sensitive if the sample size is really large.
D) None of these choices is true.
E) Choices a, b, and c are all true.
A) The test does depend on which and how many categories we use for the histogram.
B) The test is not very effective unless the sample size is large, say, at least 80 or 100.
C) The test tends to be too sensitive if the sample size is really large.
D) None of these choices is true.
E) Choices a, b, and c are all true.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
67
The chi-square goodness-of-fit test can be used to test for:
A) significance of sample statistics
B) difference between population means
C) normality
D) difference between population variances
A) significance of sample statistics
B) difference between population means
C) normality
D) difference between population variances

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
68
A real estate agency wants to compare the appraised values of single-family homes in two cities in Michigan. A sample of 60 listings in Lansing and 99 listings in Grand Rapids yields the following results (in thousands of dollars): 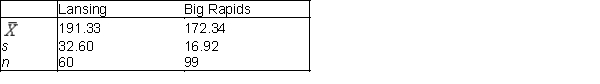
(A) Is there evidence of a significant difference in the average appraised values for single-family homes in the two Michigan cities? Use 0.05 level of significance.
(B) Have any of the assumptions made in (A) been violated? Explain.
(C) Construct a 95% confidence interval estimate of the difference between the population means of Lansing and Grand Rapids.
(D) Explain how to use the confidence interval in (C) to answer (A).

(A) Is there evidence of a significant difference in the average appraised values for single-family homes in the two Michigan cities? Use 0.05 level of significance.
(B) Have any of the assumptions made in (A) been violated? Explain.
(C) Construct a 95% confidence interval estimate of the difference between the population means of Lansing and Grand Rapids.
(D) Explain how to use the confidence interval in (C) to answer (A).

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
69
A statistics professor has just given a final examination in his linear models course. He is particularly interested in determining whether the distribution of 50 exam scores is normally distributed. The data are shown in the table below. Perform the Lilliefors test. Report and interpret the results of the test. 


Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
70
A Q-Q plot can be used to test for normality.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
71
What is the idea behind the chi-square test for independence?
A) to compare the quantile-quantile (Q-Q) plot with what would be expected under independence
B) to compare the actual counts in a contingency table with what would be expected under independence
C) to compare the cumulative distribution with what would be expected under independence
D) none of these choices
A) to compare the quantile-quantile (Q-Q) plot with what would be expected under independence
B) to compare the actual counts in a contingency table with what would be expected under independence
C) to compare the cumulative distribution with what would be expected under independence
D) none of these choices

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
72
The manager of a consulting firm in Lansing, Michigan, is trying to assess the effectiveness of computer skills training given to all new entry-level professionals. In an effort to make such an assessment, he administers a computer skills test immediately before and after the training program to each of 20 randomly chosen employees. The pre-training and post-training scores of these 20 individuals are shown in the table below. 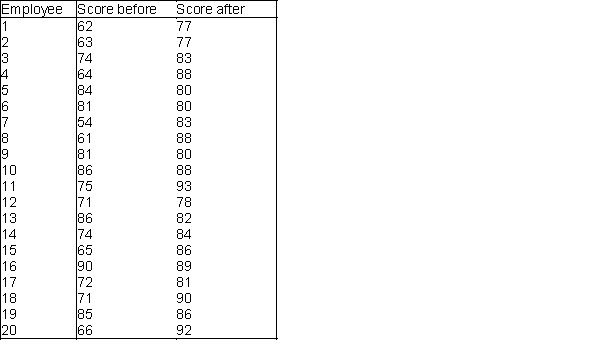
(A) Is this two-sample data or paired data. Explain your answer.
(B) Specify an appropriate hypothesis test.
(C) Using a 10% level of significance, do the given sample data support that the firm's training programs is effective in increasing the new employee's computer skills?
(D) Using a 1% level of significance, do the given sample data support that the firm's training programs is effective in increasing the new employee's working knowledge of computing?

(A) Is this two-sample data or paired data. Explain your answer.
(B) Specify an appropriate hypothesis test.
(C) Using a 10% level of significance, do the given sample data support that the firm's training programs is effective in increasing the new employee's computer skills?
(D) Using a 1% level of significance, do the given sample data support that the firm's training programs is effective in increasing the new employee's working knowledge of computing?

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
73
An investor wants to compare the risks associated with two different stocks. One way to measure the risk of a given stock is to measure the variation in the stock's daily price changes. The investor obtains a random sample of 20 daily price changes for stock 1 and 20 daily price changes for stock 2. These data are shown in the table below. Show how this investor can compare the risks associated with the two stocks by testing the null hypothesis that the variances of the stocks are equal. Use 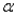 = 0.10 and interpret the results of the statistical test.
= 0.10 and interpret the results of the statistical test. 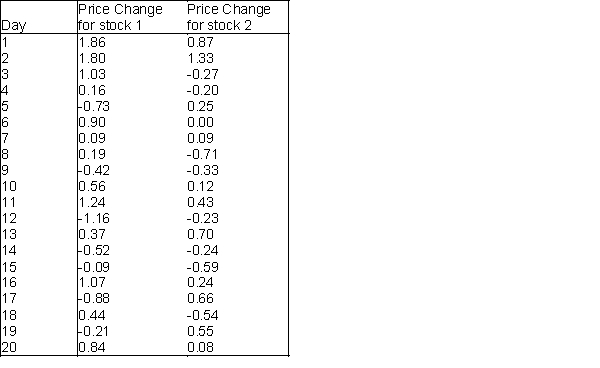
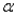 = 0.10 and interpret the results of the statistical test.
= 0.10 and interpret the results of the statistical test. 

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
74
(A) Construct a 90% confidence interval for the difference between the proportions of unacceptable assemblies generated by the two teams.
(B) Based on the confidence interval constructed in (A), is there sufficient evidence to conclude, at the 10% significance level, that the two teams differ with respect to their proportions of unacceptable assemblies?
(C) Is there sufficient evidence to conclude, at the 10% significance level, that the two teams differ with respect to their proportions of unacceptable assemblies? Conduct the appropriate hypothesis test.
(D) Calculate the p-value and explain how to use it for testing the null hypothesis of equal proportion.
(B) Based on the confidence interval constructed in (A), is there sufficient evidence to conclude, at the 10% significance level, that the two teams differ with respect to their proportions of unacceptable assemblies?
(C) Is there sufficient evidence to conclude, at the 10% significance level, that the two teams differ with respect to their proportions of unacceptable assemblies? Conduct the appropriate hypothesis test.
(D) Calculate the p-value and explain how to use it for testing the null hypothesis of equal proportion.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
75
The owner of a popular Internet-based auction site believes that more than half of the people who sell items on her site are women. To test this hypothesis, the owner sampled 1000 customers who sold items on her site and she found that 53% of the customers sampled were women. Some calculations are shown in the table below. 
(A) If you were to conduct a hypothesis test to determine if greater than 50% of customers who use this Internet-based site are women, would you conduct a one-tail or a two-tail hypothesis test? Explain your answer, and state the appropriate null and alternative hypotheses.
(B) How many customers out of the 1000 sampled must have been women in this case?
(C) Using a 5% significance level, can the owner of this site conclude that women make up more than 50% of her customers? Explain your answer.
(D) If you were to use a 1% significance level, would the conclusion from (C) change? Explain your answer.

(A) If you were to conduct a hypothesis test to determine if greater than 50% of customers who use this Internet-based site are women, would you conduct a one-tail or a two-tail hypothesis test? Explain your answer, and state the appropriate null and alternative hypotheses.
(B) How many customers out of the 1000 sampled must have been women in this case?
(C) Using a 5% significance level, can the owner of this site conclude that women make up more than 50% of her customers? Explain your answer.
(D) If you were to use a 1% significance level, would the conclusion from (C) change? Explain your answer.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
76
Which pair of tests is used to test for normality?
A) a t-test and an ANOVA test
B) an empirical cumulative distribution function test and an F-test
C) a chi-square test and a Lilliefors test
D) a quantile-quantile plot and a p-value test
A) a t-test and an ANOVA test
B) an empirical cumulative distribution function test and an F-test
C) a chi-square test and a Lilliefors test
D) a quantile-quantile plot and a p-value test

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
77
A chi-square goodness-of-fit test can be used to test for normality.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
78
Q-Mart is interested in comparing customers who used its own charge card with those who use other types of credit cards. Q-Mart would like to know if customers who use the Q-Mart card spend more money per visit, on average, than customers who use some other type of credit card. They have collected information on a random sample of 38 charge customers and the data is presented below. On average, the person using a Q-Mart card spends $192.81 per visit and customers using another type of card spend $104.47 per visit. 
(A) Given the information above, what is and
and 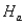 for this comparison? Also, does this represent a one-tailed or a two-tailed test? Explain your answer.
for this comparison? Also, does this represent a one-tailed or a two-tailed test? Explain your answer.
(B) What are the degrees of freedom for the t-statistic in this calculation? Explain how you would calculate the degrees of freedom in this case.
(C) What is the assumption in this case that allows you to use the pooled standard deviation for this test?
(D) Using a 5% level of significance, is there sufficient evidence for Q-Mart to conclude that customers who use the Q-Mart card charge, on average, more than those who use another charge card? Explain your answer.
(E) Using a 1% level of significance, is there sufficient evidence for Q-Mart to conclude that customers who use the Q-Mart card charge, on average, more than those who use another charge card? Explain your answer.

(A) Given the information above, what is
 and
and 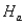 for this comparison? Also, does this represent a one-tailed or a two-tailed test? Explain your answer.
for this comparison? Also, does this represent a one-tailed or a two-tailed test? Explain your answer.
(B) What are the degrees of freedom for the t-statistic in this calculation? Explain how you would calculate the degrees of freedom in this case.
(C) What is the assumption in this case that allows you to use the pooled standard deviation for this test?
(D) Using a 5% level of significance, is there sufficient evidence for Q-Mart to conclude that customers who use the Q-Mart card charge, on average, more than those who use another charge card? Explain your answer.
(E) Using a 1% level of significance, is there sufficient evidence for Q-Mart to conclude that customers who use the Q-Mart card charge, on average, more than those who use another charge card? Explain your answer.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
79
A test for independence is applied to a contingency table with 4 rows and 4 columns. The degrees of freedom for this chi-square test must equal 9.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
80
Lilliefors test for normality compare two cumulative distribution functions (cdf's): the cdf from a normal distribution and the cdf corresponding to the given data (called the empirical cdf).

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck



