Deck 1: Functions and Applications
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/159
Play
Full screen (f)
Deck 1: Functions and Applications
1
Find the best-fit line associated with the set of points. , , ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
The chart shows second quarter total retail e-commerce sales in the U.S. in 1999, 2001 and 2003 ( represents 1999). Find the regression line. Round coefficients to two decimal places. Use the regression line to estimate second quarter retail e-commerce sales in 2000. Round your answer to two decimal places.
A)$3.04 billion
B) $18.24 billion
C) $12.16 billion
D) $6.08 billion
E) $6.69 billion
A)$3.04 billion
B) $18.24 billion
C) $12.16 billion
D) $6.08 billion
E) $6.69 billion
$6.08 billion
3
Find the coefficient of correlation of the line that best fits the data set.
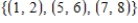
Please give the answer to four decimal places if necessary.
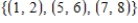
Please give the answer to four decimal places if necessary.
1
4
Use correlation coefficients to determine which of the given sets of data is worst fit by its associated regression line.
a)
b)
c)
A)a
B) b and c
C) c
D) a and b
E) b
a)
b)
c)
A)a
B) b and c
C) c
D) a and b
E) b

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
5
In 2004 the Texas Bureau of Economic Geology published a study on the economic impact of using carbon dioxide enhanced oil recovery (EOR) technology to extract additional oil from fields that have reached the end of their conventional economic life. The table gives the approximate number of jobs for the citizens of Texas that would be created at various levels of recovery. Find the regression line. Use the regression line to estimate the number of jobs that would be created at a recovery level of 85%.
A) million jobs
B) million jobs
C) million jobs
D) million jobs
E) million jobs
A) million jobs
B) million jobs
C) million jobs
D) million jobs
E) million jobs

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
6
Find the coefficient of correlation of the line that best fits the data set.
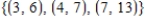
Please give the answer to four decimal places if necessary.
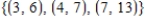
Please give the answer to four decimal places if necessary.

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
7
Find the coefficient of correlation of the line that best fits the data set.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
8
Find the best-fit line associated with the set of points. , ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
9
Find the best-fit line associated with the set of points. , , ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
10
Find the coefficient of correlation of the line that best fits the data set.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
11
The table shows soybean production, in millions of tons, in Brazil's Cerrados region, as a function of the cultivated area, in millions of acres. Use technology to obtain the regression line. Round coefficients to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
12
Find the coefficient of correlation of the line that best fits the data set.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
13
Find the coefficient of correlation of the line that best fits the data set.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
14
Find the coefficient of correlation of the line that best fits the data set.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
15
Find the regression line associated with the set of points. Round all coefficients to 4 decimal places. , ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
16
Following are approximate values of the Amex Gold BUGS Index. ( represents 2000)
Obtain the associated regression line. (Round coefficients to 2 decimal places if necessary.) Use your regression equation to project the 2001 sales.
A)65.33
B) 83.33
C) 93.33
D) 63.33
E) 94.33
Obtain the associated regression line. (Round coefficients to 2 decimal places if necessary.) Use your regression equation to project the 2001 sales.
A)65.33
B) 83.33
C) 93.33
D) 63.33
E) 94.33

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
17
Find the best-fit line associated with the set of points. , ,
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
18
Find the coefficient of correlation of the line that best fits the data set.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
19
Following are forecasts of worldwide annual cell phone handset sales. ( represents 2003)
Obtain the associated regression line. (Round coefficients to 2 decimal places if necessary.) Use your regression equation to project the 2017 sales.
A)1533.33
B) 1523.33
C) 1543.33
D) 1547.33
E) 1516.33
Obtain the associated regression line. (Round coefficients to 2 decimal places if necessary.) Use your regression equation to project the 2017 sales.
A)1533.33
B) 1523.33
C) 1543.33
D) 1547.33
E) 1516.33

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
20
The table shows the number of fiber-optic cable connections to homes in the U.S. from 2000 to 2004 ( represents 2000). Use technology to obtain the linear regression line, with regression coefficients rounded to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
21
The linear function is given. Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
22
a) Find correlation coefficient to the set of data. Round the answer to 4 decimal places if necessary.
r = __________
b) Find correlation coefficient to the set of data. Round the answer to 4 decimal places if necessary.
r = __________
c) Find correlation coefficient to the set of data. Round the answer to 4 decimal places if necessary.
r = __________
Use correlation coefficients to determine which of the given sets of data is best fit by its associated regression line.
__________
Use correlation coefficients to determine which of the given sets of data is worst fit by its associated regression line .
Is it a perfect fit for any of the data sets
r = __________
b) Find correlation coefficient to the set of data. Round the answer to 4 decimal places if necessary.
r = __________
c) Find correlation coefficient to the set of data. Round the answer to 4 decimal places if necessary.
r = __________
Use correlation coefficients to determine which of the given sets of data is best fit by its associated regression line.
__________
Use correlation coefficients to determine which of the given sets of data is worst fit by its associated regression line .
Is it a perfect fit for any of the data sets

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
23
Following are approximate values of the Amex Gold BUGS Index. 
(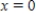 represents 2000)
represents 2000)
Complete the table.
Obtain the associated regression line. (Round coefficients to 2 decimal places if necessary.)
Slope: __________
Intercept: __________
Use your regression equation to project the 2001 sales. (Round the answer to 2 decimal places if necessary.)
__________

(
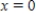 represents 2000)
represents 2000)Complete the table.

Obtain the associated regression line. (Round coefficients to 2 decimal places if necessary.)
Slope: __________
Intercept: __________
Use your regression equation to project the 2001 sales. (Round the answer to 2 decimal places if necessary.)
__________

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
24
A table of values for a linear function is given. Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
25
Sketch the straight line of the following equation.
A)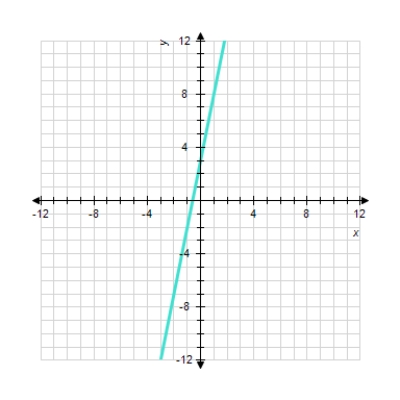
B)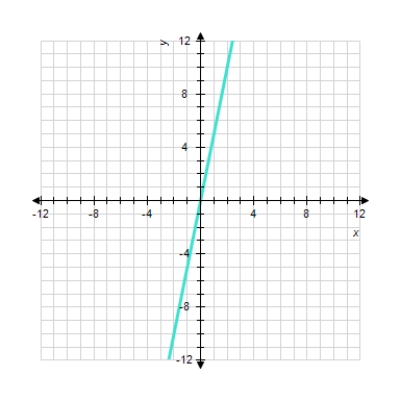
C)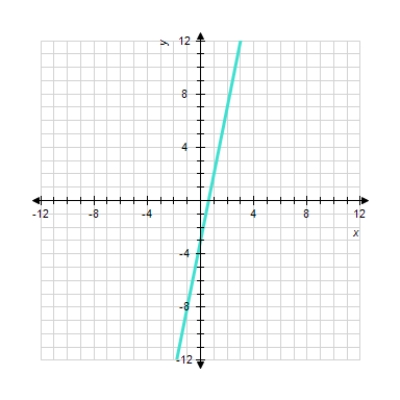
D)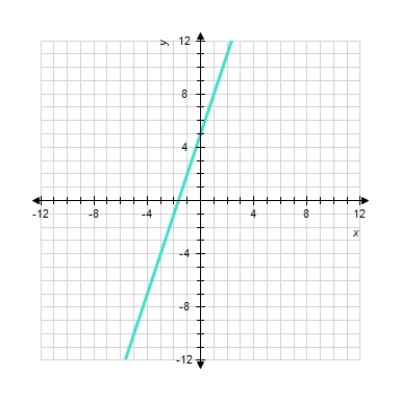
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
26
The chart shows second quarter total retail e-commerce sales in the U.S. in 2001, 2003 and 2005 ( 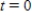 represents 2001). Find the regression line. Round coefficients to two decimal places.
represents 2001). Find the regression line. Round coefficients to two decimal places. 
y = __________ t + __________
Use the regression line to estimate second quarter retail e-commerce sales in 2002. Round your answers to two decimal places if necessary.
$__________ billion
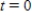 represents 2001). Find the regression line. Round coefficients to two decimal places.
represents 2001). Find the regression line. Round coefficients to two decimal places. 
y = __________ t + __________
Use the regression line to estimate second quarter retail e-commerce sales in 2002. Round your answers to two decimal places if necessary.
$__________ billion

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
27
Find the best-fit line associated with the set of points.
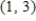 ,
, 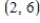 ,
, 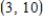
Please enter your answer as an equation of line in the form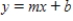 . Round m and b to the nearest hundredth if necessary.
. Round m and b to the nearest hundredth if necessary.
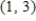 ,
, 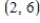 ,
, 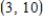
Please enter your answer as an equation of line in the form
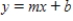 . Round m and b to the nearest hundredth if necessary.
. Round m and b to the nearest hundredth if necessary.
Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
28
Find the best-fit line associated with the set of points.
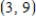 ,
, 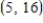 ,
, 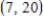 ,
, 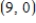
Please enter your answer as an equation of line in the form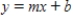 . Round m and b to the nearest hundredth if necessary.
. Round m and b to the nearest hundredth if necessary.
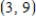 ,
, 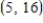 ,
, 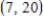 ,
, 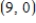
Please enter your answer as an equation of line in the form
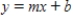 . Round m and b to the nearest hundredth if necessary.
. Round m and b to the nearest hundredth if necessary.
Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
29
In 2004 the Texas Bureau of Economic Geology published a study on the economic impact of using carbon dioxide enhanced oil recovery (EOR) technology to extract additional oil from fields that have reached the end of their conventional economic life. The table gives the approximate number of jobs for the citizens of Texas that would be created at various levels of recovery. Find the regression line. 
y = __________ x + __________
Use the regression line to estimate the number of jobs that would be created at a recovery level of 31%. Round your answers to three decimal places if necessary.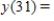 ___________ million jobs
___________ million jobs

y = __________ x + __________
Use the regression line to estimate the number of jobs that would be created at a recovery level of 31%. Round your answers to three decimal places if necessary.
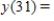 ___________ million jobs
___________ million jobs
Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
30
Find the coefficient of correlation of the line that best fits the data set.
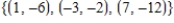
Please give the answer to four decimal places if necessary.
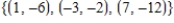
Please give the answer to four decimal places if necessary.

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
31
Find the coefficient of correlation of the line that best fits the data set.
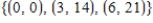
Please give the answer to four decimal places if necessary.
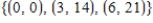
Please give the answer to four decimal places if necessary.

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
32
Find the best-fit line associated with the set of points.
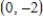 ,
, 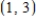 ,
, 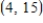 ,
, 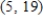
Please enter your answer as an equation of line in the form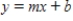 . Round m and b to the nearest hundredth if necessary.
. Round m and b to the nearest hundredth if necessary.
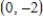 ,
, 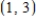 ,
, 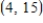 ,
, 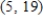
Please enter your answer as an equation of line in the form
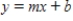 . Round m and b to the nearest hundredth if necessary.
. Round m and b to the nearest hundredth if necessary.
Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
33
Find the coefficient of correlation of the line that best fits the data set.
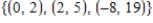
Please give the answer to four decimal places if necessary.
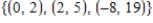
Please give the answer to four decimal places if necessary.

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
34
Find the best-fit line associated with the set of points.
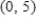 ,
, 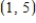 ,
, 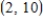
Please enter your answer as an equation of line in the form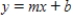 . Round m and b to the nearest hundredth if necessary.
. Round m and b to the nearest hundredth if necessary.
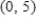 ,
, 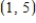 ,
, 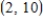
Please enter your answer as an equation of line in the form
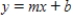 . Round m and b to the nearest hundredth if necessary.
. Round m and b to the nearest hundredth if necessary.
Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
35
A table of values for a linear function is given. Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
36
Find the equation of the given linear function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
37
Sketch the straight line with the equation.
A)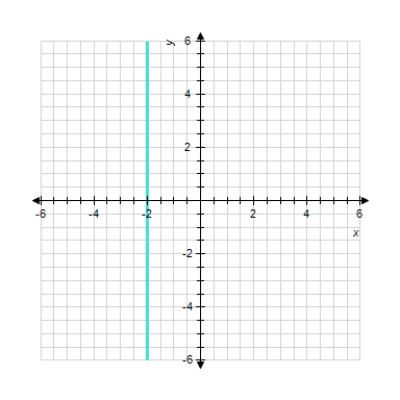
B)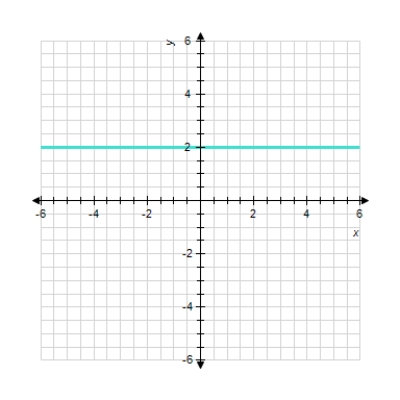
C)
D)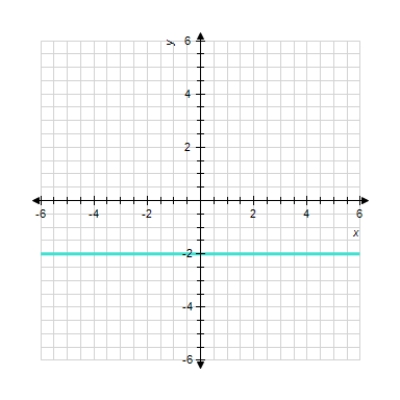
E)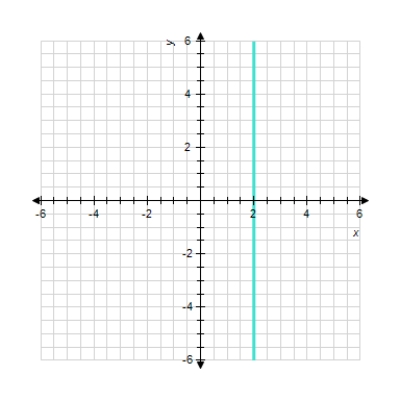
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
38
Decide which of the two given functions is linear and find its equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
39
Find the coefficient of correlation of the line that best fits the data set.
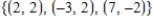
Please give the answer to four decimal places if necessary.
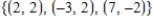
Please give the answer to four decimal places if necessary.

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
40
Following are forecasts of worldwide annual cell phone handset sales. 
(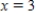 represents 2003)
represents 2003)
Complete the table.
Obtain the associated regression line. (Round coefficients to 2 decimal places if necessary.)
Slope: __________
Intercept: __________
Use your regression equation to project the 2016 sales. (Round the answer to 2 decimal places if necessary.)
__________

(
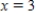 represents 2003)
represents 2003)Complete the table.

Obtain the associated regression line. (Round coefficients to 2 decimal places if necessary.)
Slope: __________
Intercept: __________
Use your regression equation to project the 2016 sales. (Round the answer to 2 decimal places if necessary.)
__________

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
41
Sketch the straight line with the equation.
A)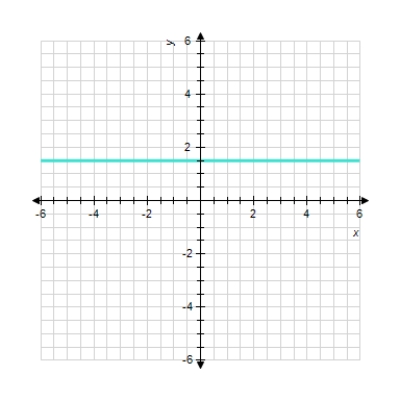
B)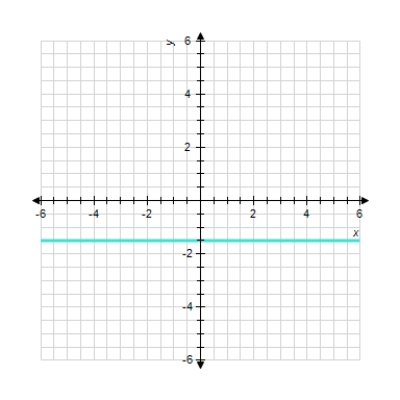
C)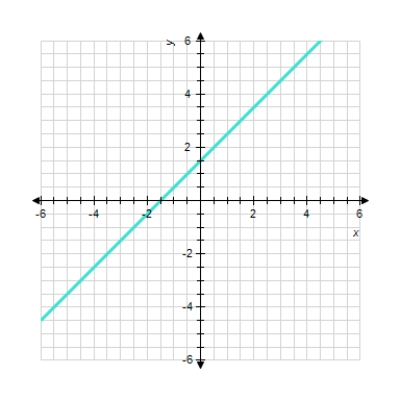
D)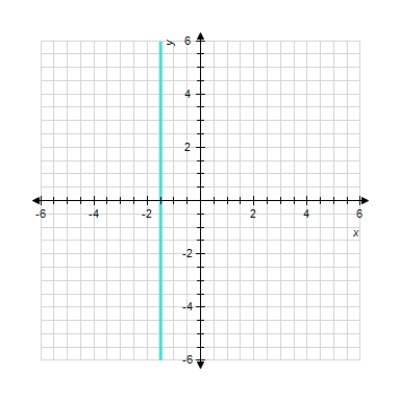
E)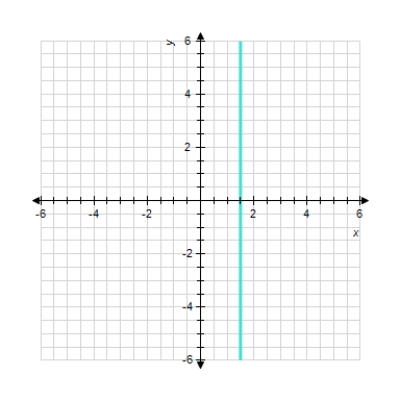
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
42
The demand for your college newspaper is 1800 copies per week if the paper is given a way free of charge, and the demand drops to 900 if the charge is $0.10 per copy. However, the university is prepared to supply only 700 copies per week free of charge but will supply 950 per week at $0.25 per copy. At what price should the college newspapers be sold so that there is neither a surplus nor a shortage of papers ?
A)$0.07
B) $0.06
C) $0.22
D) $0.17
E) $0.11
A)$0.07
B) $0.06
C) $0.22
D) $0.17
E) $0.11

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
43
Annual federal spending on Medicare increased more or less linearly from $45 billion in 1973 to $87 billion in 1994. Use these data to express s, the annual spending on Medicare (in billions of dollars), as a linear function of t, the number of years since 1973.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
44
Find the linear equation that is the straight line through (5, 5) and parallel to the line .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
45
The position of a model train, in feet along the railroad track, is given by after t seconds.
Where is the train after 10 seconds
A)13 feet
B) 38 feet
C) 16.5 feet
D) 65 feet
E) 35 feet
Where is the train after 10 seconds
A)13 feet
B) 38 feet
C) 16.5 feet
D) 65 feet
E) 35 feet

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
46
Calculate the slope of the straight line through the points and . Try to do the calculation mentally.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
47
A piano manufacture has a daily fixed cost of $1,300 and a marginal cost of $1,600 per piano. On a given day, what is the cost of manufacturing 3 pianos ?
A) $3,500
B) $8,700
C) $6,100
D) $5,500
A) $3,500
B) $8,700
C) $6,100
D) $5,500

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
48
You can sell 90 pet chias per week if they are marked as $1 each, but only 40 per week if they are marked $2 per chia. Your chia supplier is prepared to sell you 25 chias per week if they are marked $1 per chia, and 75 per week if they are marked $2 per chia. Write the associated linear demand and supply functions.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
49
You can sell 60 pet chias per week if they are marked as $1 each,but only 50 per week if they are marked $2 per chia. Your chia supplier is prepared to sell you 10 chias per week if they are marked $1 per chia, and 20 per week if they are marked $2 per chia. At what price should the chias be marked so that there is neither surplus nor a shortage of chias ?
A)$3.50
B) $3.64
C) $2.25
D) $3.55
E) $4.50
A)$3.50
B) $3.64
C) $2.25
D) $3.55
E) $4.50

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
50
Sketch the straight line with the equation.
A)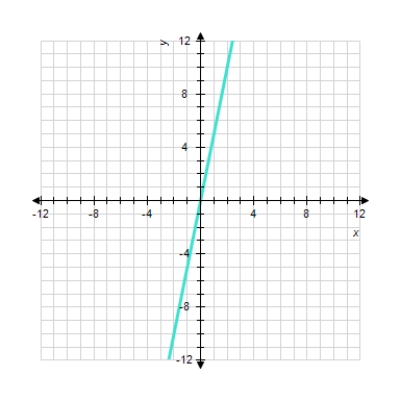
B)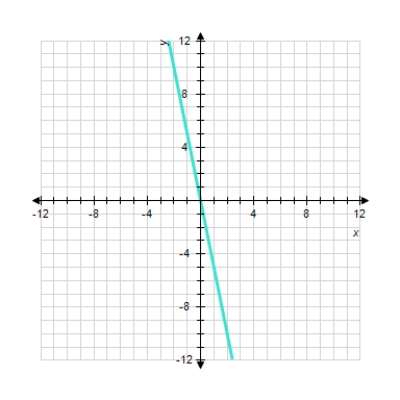
C)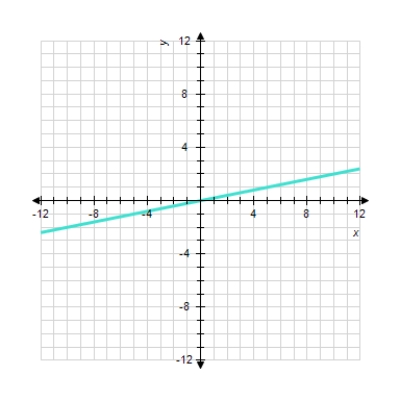
D)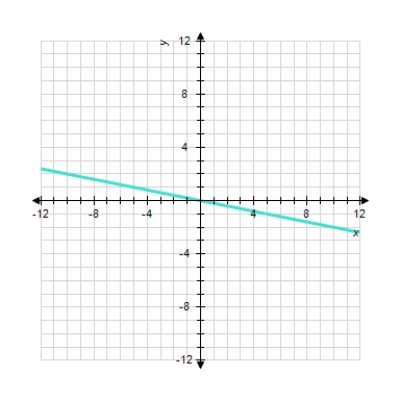
E)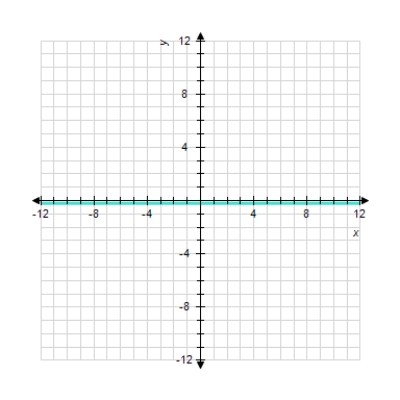
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
51
Estimate the slope of the line segment. 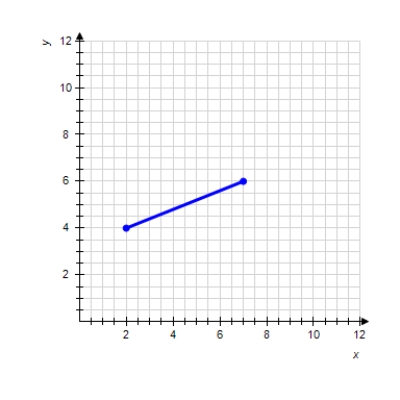
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
52
U.S. imports of pasta increased from 290 million pounds in 1990 , by an average of 52 million pounds per year. Estimate U.S. pasta import (in million pounds) in the year 2005, assuming the import trend continued.
A)2,295 million pounds
B) 1,122 million pounds
C) 342 million pounds
D) 780 million pounds
E) 1,070 million pounds
A)2,295 million pounds
B) 1,122 million pounds
C) 342 million pounds
D) 780 million pounds
E) 1,070 million pounds

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
53
Calculate the slope of the straight line through the points and . Try to do the calculations mentally.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
54
Find the linear equation that is the straight line through (25, -1) and increasing at a rate of 5 units of y per unit of x.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
55
Estimate the slope of the line segment. 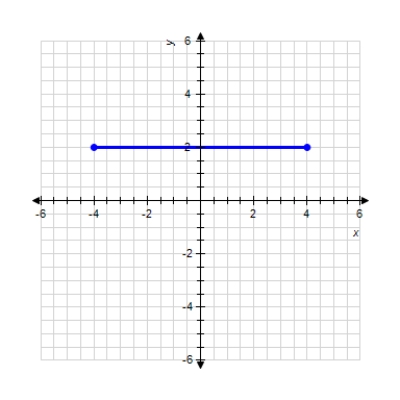
A)
B)
C)
D)
E) Undefined

A)
B)
C)
D)
E) Undefined

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
56
A piano manufacture has a daily fixed cost of $1,000 and a marginal cost of $1,500 per piano. Find the cost C of manufacturing x pianos in one day.
A) per day
B) per day
C) per day
D) per day
E) per day
A) per day
B) per day
C) per day
D) per day
E) per day

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
57
Calculate the slope of the straight line through the points (4, 3) and (9, 13).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
58
Find the linear equation that is the straight line through with slope .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
59
Find the linear equation that is the straight line through (1, 2) and (8, 30).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
60
Find the linear equation that is the straight line through with slope 5.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
61
A police car was traveling down Ocean Parkway in a high-speed chase from Jones Beach. The car was at Jones Beach at exactly 9:00 p.m. , and was at Oak Beach, 13 miles from Jones Beach, at exactly 9:04 p.m. How fast was the police car traveling (Round your answer to the nearest tenth.)
A)2.6 miles/min.
B) 4.3 miles/min.
C) 3.3 miles/min.
D) 2.5 miles/min.
E) 3.0 miles/min.
A)2.6 miles/min.
B) 4.3 miles/min.
C) 3.3 miles/min.
D) 2.5 miles/min.
E) 3.0 miles/min.

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
62
The position of a model train, in feet along the railroad track, is given by after t seconds.
When will the train have moved a distance of 33 feet
A)after 30 seconds
B) after 10 seconds
C) after 13 seconds
D) after 12 seconds
E) after 11 seconds
When will the train have moved a distance of 33 feet
A)after 30 seconds
B) after 10 seconds
C) after 13 seconds
D) after 12 seconds
E) after 11 seconds

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
63
The demand for your college newspaper is 2,000 copies per week if the paper is given a way free of charge, and the demand drops to 1,000 if the charge is $0.25 per copy. However, the university is prepared to supply only 600 copies per week free of charge but will supply 3,600 per week at $0.50 per copy. At what price should the college newspapers be sold so that there is neither a surplus nor a shortage of papers Round your answer to two decimal places.
$ __________
$ __________

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
64
Estimate the slope of the line segment.
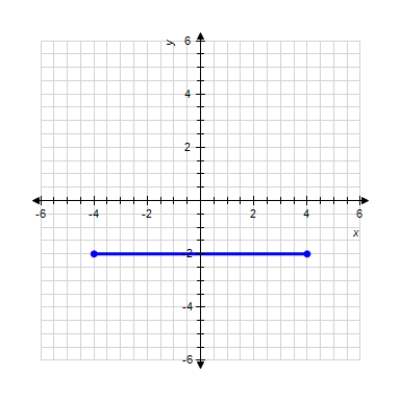


Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
65
You can sell 45 pet chias per week if they are marked as $4 each,but only 20 per week if they are marked $5 per chia.Your chia supplier is prepared to sell you 10 chias per week if they are marked $4 per chia, and 35 per week if they are marked $5 per chia. At what price should the chias be marked so that there is neither surplus nor a shortage of chias Round your answer to two decimal places. $ __________

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
66
The height of the falling sheet of paper, in feet from the ground, is given by after t seconds.
When will the sheet of paper reach the ground
A)after 9 seconds
B) after 7.5 seconds
C) after 15 seconds
D) after 6 seconds
E) after 3 seconds
When will the sheet of paper reach the ground
A)after 9 seconds
B) after 7.5 seconds
C) after 15 seconds
D) after 6 seconds
E) after 3 seconds

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
67
The Oliver company plans to market a new product. Based on its market studies, Oliver estimates that it can sell up to 5,000 units in 2005. The selling price will be $2 per unit. Variable costs are estimated to be 20% of total revenue. Fixed costs are estimated to be $5,600 for 2005. How many units should the company sell to break even ?
A)5,000 units
B) 5,600 units
C) 2,333 units
D) 3,500 units
E) 2,800 units
A)5,000 units
B) 5,600 units
C) 2,333 units
D) 3,500 units
E) 2,800 units

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
68
Calculate the slope of the straight line through the points (3, 3) and (8, 18). Try to do the calculations mentally.

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
69
In the Fahrenheit temperature scale, water freezes at 32°F and boils at 212°F. In the Celsius (or centigrade) scale, water freezes at 0°C and boils at 100°C. Assuming that the Fahrenheit temperature F and the Celsius temperature C are related by a linear equation, find the Fahrenheit temperature that correspond to 26°C, to the nearest degree.
A)46°F
B) 104°F
C) 79°F
D) 47°F
E) 58°F
A)46°F
B) 104°F
C) 79°F
D) 47°F
E) 58°F

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
70
A car that was being pursued by the police was at Jones Beach at exactly 7:57 p.m. , and passed Oak Beach (13 miles from Jones Beach) at exactly 8:06 p.m.,where it was overtaken by the police. How fast, in miles per minute, was the car traveling (Round your answer to the nearest tenth.)
A)
B)
C)
D)
E) .
A)
B)
C)
D)
E) .

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
71
A piano manufacture has a daily fixed cost of $1,400 and a marginal cost of $1,900 per piano. On a given day, what is the cost of manufacturing 3 pianos $ __________

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
72
Following are some approximate values of the Amex Gold BUGS Index.
Take t to be the year since 1995 and y to be the BUGS index.
Obtain a piecewise linear model of the gold BUGS index for 1995-2004.
A)
B)
C)
D)
E)
Take t to be the year since 1995 and y to be the BUGS index.
Obtain a piecewise linear model of the gold BUGS index for 1995-2004.
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
73
In the Fahrenheit temperature scale, water freezes at 32°F and boils at 212°F. In the Celsius (or centigrade) scale, water freezes at 0°C and boils at 100°C. Assuming that the Fahrenheit temperature F and the Celsius temperature C are related by a linear equation, find the Celsius temperature that correspond to 39°F, to the nearest degree.
A)54°C
B) 7°C
C) 4°C
D) 39°C
E) 38°C
A)54°C
B) 7°C
C) 4°C
D) 39°C
E) 38°C

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
74
Calculate the slope of the straight line through the points 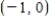 and
and 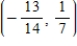 . Try to do the calculations mentally.
. Try to do the calculations mentally.
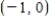 and
and 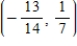 . Try to do the calculations mentally.
. Try to do the calculations mentally.
Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
75
The linear function is given. Find 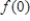 .
. 
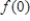 .
. 

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
76
The position of a model train, in feet along the railroad track, is given by after t seconds.
Where is the train after 2 seconds
__________ feet
Where is the train after 2 seconds
__________ feet

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
77
Calculate the slope of the straight line through the points (2.6, 5) and (6.6, -3). Try to do the calculations mentally.

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
78
The Snowtree cricket behaves in a rather interesting way: The rate at which it chirps depends linearly on the temperature. One summer evening you hear a cricket chirping at a rate of 140 chirps per minute, and you notice that the temperature is 80°F. Later in the evening, the cricket has slowed down to 120 chirps per minute, and you notice that the temperature has dropped to 75°F. What is the temperature if the cricket is chirping at a rate of 108 chirps per minute ?
A)72°F
B) 70°F
C) 78°F
D) 67°F
E) 76°F
A)72°F
B) 70°F
C) 78°F
D) 67°F
E) 76°F

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
79
In 1950 the number of retirees was approximately 150 per thousand people aged 20-64. In 1990 this number rose to approximately 200, and it is projected to rise to 275 in 2020. Model N as a piecewise linear function of the time t in years since 1950, and use your model to project the number of retires per thousand people aged 20-64 in 1965. (Round you answer to the nearest integer.)
A)169 people per thousand
B) 219 people per thousand
C) 203 people per thousand
D) 152 people per thousand
E) 118 people per thousand
A)169 people per thousand
B) 219 people per thousand
C) 203 people per thousand
D) 152 people per thousand
E) 118 people per thousand

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck
80
U.S. imports of pasta increased from 290 million pounds in 1990 (t = 0), by an average of 52 million pounds per year. Estimate U.S. pasta import (in million pounds) in the year 2010, assuming the import trend continued.
__________ million pounds
__________ million pounds

Unlock Deck
Unlock for access to all 159 flashcards in this deck.
Unlock Deck
k this deck



