Deck 5: Discrete Probability Distributions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/111
Play
Full screen (f)
Deck 5: Discrete Probability Distributions
1
i. A random variable is assigned numerical values based on the outcomes of an experiment.
ii. A random variable is a quantity resulting from a random experiment that can assume different values by chance.
Iii) The mean of a probability distribution is referred to as its expected value.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
ii. A random variable is a quantity resulting from a random experiment that can assume different values by chance.
Iii) The mean of a probability distribution is referred to as its expected value.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
(i), (ii) and (iii) are all correct statements
2
i. If we measure the weight of an eggnog carton, the variable is referred to as being a discrete random variable.
Ii) If we toss two coins and count the number of heads, there could be 0, 1 or 2 heads. Since the exact number of heads resulting from this experiment is due to chance, the number of heads appearing is a random variable.
Iii) A random variable may be either discrete or continuous.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
Ii) If we toss two coins and count the number of heads, there could be 0, 1 or 2 heads. Since the exact number of heads resulting from this experiment is due to chance, the number of heads appearing is a random variable.
Iii) A random variable may be either discrete or continuous.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
D
3
If the variance of a probability was computed to be 3.6 grams, what is the standard deviation?
A) 0.6
B) 1.9
C) 6.0
D) 12.96
E) None of the choices are correct
A) 0.6
B) 1.9
C) 6.0
D) 12.96
E) None of the choices are correct
B
4
A company is studying the number of monthly absences among its 125 employees. The following probability distribution shows the likelihood that people were absent 0, 1, 2, 3, 4, or 5 days last month.  What is the variance of the number of days absent?
What is the variance of the number of days absent?
A) 1.99
B) 1.41
C) 5.00
D) 55.52
 What is the variance of the number of days absent?
What is the variance of the number of days absent?A) 1.99
B) 1.41
C) 5.00
D) 55.52

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
5
What is the following table called? 
A) Probability distribution
B) Ogive
C) Standard deviation
D) Frequency table
E) None of the choices are correct

A) Probability distribution
B) Ogive
C) Standard deviation
D) Frequency table
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
6
i. The probability of a particular outcome, designated X, must always be between 0 and 100 inclusive.
ii. A random variable is a quantity resulting from a random experiment that can assume different values by chance.
Iii) The mean of a probability distribution is referred to as its expected value.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
ii. A random variable is a quantity resulting from a random experiment that can assume different values by chance.
Iii) The mean of a probability distribution is referred to as its expected value.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
7
i. The probability of a particular outcome, designated X, must always be between 0 and 1 inclusive.
ii. A random variable represents the outcomes of an experiment.
Iii) The mean of a probability distribution is referred to as its expected value.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
ii. A random variable represents the outcomes of an experiment.
Iii) The mean of a probability distribution is referred to as its expected value.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
8
A company is studying the number of monthly absences among its 125 employees. The following probability distribution shows the likelihood that people were absent 0, 1, 2, 3, 4, or 5 days last month.  What is the mean number of days absent?
What is the mean number of days absent?
A) 1.00
B) 0.40
C) 0.72
D) 2.5
 What is the mean number of days absent?
What is the mean number of days absent?A) 1.00
B) 0.40
C) 0.72
D) 2.5

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
9
The information below is the number of daily emergency assists made to skiers by the volunteer ski team at Alpine Ski Lodge for the last 50 days.
To explain, there were 22 days on which there were 2 emergency assists, and 9 days on which there were 3 emergency assists.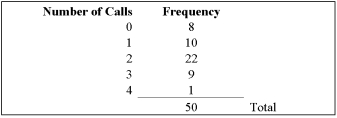
A) 1.56
B) 1.7
C) 1.66
D) 1.76
E) 1.77
To explain, there were 22 days on which there were 2 emergency assists, and 9 days on which there were 3 emergency assists.

A) 1.56
B) 1.7
C) 1.66
D) 1.76
E) 1.77

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
10
A tennis match requires that a player win three of five sets to win the match. If a player wins the first two sets, what is the probability that the player wins the match, assuming that each player is equally likely to win each match?
A) 0.5
B) 1/8 or 0.125
C) 7/8 or 0.875
D) Cannot be computed.
A) 0.5
B) 1/8 or 0.125
C) 7/8 or 0.875
D) Cannot be computed.

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
11
What is a listing of all possible outcomes of an experiment and their corresponding probability of occurrence called?
A) Random variable
B) Probability distribution
C) Subjective probability
D) Frequency distribution
E) None of the choices are correct
A) Random variable
B) Probability distribution
C) Subjective probability
D) Frequency distribution
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
12
Which of the following is correct about a probability distribution?
A) Sum of all possible outcomes must equal 1
B) Outcomes must be mutually exclusive
C) Probability of each outcome must be between 0 and 1 inclusive
D) All of the choices are correct
E) None of the choices are correct
A) Sum of all possible outcomes must equal 1
B) Outcomes must be mutually exclusive
C) Probability of each outcome must be between 0 and 1 inclusive
D) All of the choices are correct
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
13
The weight of an offensive linesman may be 205.15 pounds, 210.23 pounds, 225.05 pounds or 219.14 pounds. What is this an illustration of?
A) Continuous random variable
B) Discrete random variable
C) Complement rule
D) All of the choices are correct
E) None of the choices are correct
A) Continuous random variable
B) Discrete random variable
C) Complement rule
D) All of the choices are correct
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
14
What kind of distribution are the binomial and Poisson distributions?
A) Discrete
B) Continuous
C) Both discrete and continuous
D) Neither discrete or continuous
A) Discrete
B) Continuous
C) Both discrete and continuous
D) Neither discrete or continuous

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
15
The probabilities and the number of automobiles lined up at a Lakeside Olds at opening time (7:30 a.m.) for service are: 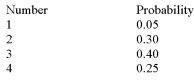 On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening?
On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening?
A) 1.96
B) 2.85
C) 7.00
D) 24.0
E) 5.58
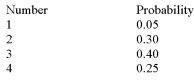 On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening?
On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening?A) 1.96
B) 2.85
C) 7.00
D) 24.0
E) 5.58

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
16
The probabilities and the number of automobiles lined up at a Lakeside Olds at opening time (7:30 a.m.) for service are: 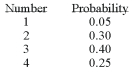 On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening?
On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening?
A) 10.00
B) 1.00
C) 2.85
D) 1.96
E) None of the choices are correct
 On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening?
On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening?A) 10.00
B) 1.00
C) 2.85
D) 1.96
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
17
A company is studying the number of monthly absences among its 125 employees. The following probability distribution shows the likelihood that people were absent 0, 1, 2, 3, 4, or 5 days last month.  Given the probability distribution, which of the following predictions is correct?
Given the probability distribution, which of the following predictions is correct?
A) 60% of the employees will have more than one day absent for a month
B) There is a 0.04 probability that an employee will be absent 1 day during a month
C) There is a 0.12 probability that an employee will be absent 2 days during a month
D) There is a 0.50 probability that an employee will be absent 0.72 days during a month.
 Given the probability distribution, which of the following predictions is correct?
Given the probability distribution, which of the following predictions is correct?A) 60% of the employees will have more than one day absent for a month
B) There is a 0.04 probability that an employee will be absent 1 day during a month
C) There is a 0.12 probability that an employee will be absent 2 days during a month
D) There is a 0.50 probability that an employee will be absent 0.72 days during a month.

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
18
Belk Department Store is having a special sale this weekend. Customers charging purchases of more than $50 to the Belk credit card will be given a special Belk lottery card. The customer will scratch the card, which will indicate the amount to be taken off the total amount of purchase. Listed below is the amount of the prize and the percent of the time that amount will be deducted from the total amount of the purchase. 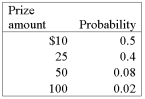 Determine the mean and standard deviation of the prize amounts.
Determine the mean and standard deviation of the prize amounts.
A) Mean is $21, standard deviation is $26.66
B) Mean is $21, standard deviation is $710.50
C) Mean is $20, standard deviation is $25.00
D) Mean is $20, standard deviation is $26.66
E) Mean is $46.25, standard deviation is $710.50
 Determine the mean and standard deviation of the prize amounts.
Determine the mean and standard deviation of the prize amounts.A) Mean is $21, standard deviation is $26.66
B) Mean is $21, standard deviation is $710.50
C) Mean is $20, standard deviation is $25.00
D) Mean is $20, standard deviation is $26.66
E) Mean is $46.25, standard deviation is $710.50

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
19
The probabilities and the number of automobiles lined up at a Lakeside Olds at opening time (7:30 a.m.) for service are:  On a typical day, what is the standard deviation of the number of cars that Lakeside Olds can expect to be lined up at opening?
On a typical day, what is the standard deviation of the number of cars that Lakeside Olds can expect to be lined up at opening?
A) 1.96
B) 2.85
C) 0.7275
D) 0.2400
E) 0.05
 On a typical day, what is the standard deviation of the number of cars that Lakeside Olds can expect to be lined up at opening?
On a typical day, what is the standard deviation of the number of cars that Lakeside Olds can expect to be lined up at opening?A) 1.96
B) 2.85
C) 0.7275
D) 0.2400
E) 0.05

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
20
i. A probability distribution is a mutually exclusive listing of experimental outcomes that can occur by chance and their corresponding probabilities.
Ii) The probability of a particular outcome, designated X, must always be between 0 and 10 inclusive.
iii. The standard deviation of a probability distribution is referred to as its expected value.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
Ii) The probability of a particular outcome, designated X, must always be between 0 and 10 inclusive.
iii. The standard deviation of a probability distribution is referred to as its expected value.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
21
i. For a binomial distribution, each trial has a known number of successes. For example, a four question multiple-choice test can only have zero, one, two, three and four successes (number correct).
ii. To construct a binomial probability distribution, the number of trials and the probability of success must be known.
Iii) A binomial distribution has a characteristic that the trials are independent, which means that the outcome of one trial does not affect the outcome of any other trial.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
ii. To construct a binomial probability distribution, the number of trials and the probability of success must be known.
Iii) A binomial distribution has a characteristic that the trials are independent, which means that the outcome of one trial does not affect the outcome of any other trial.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
22
Which of the following is NOT a characteristic of a binomial probability distribution?
A) Each outcome is mutually exclusive
B) Each trial is independent
C) Probability of success remains constant from trial to trial
D) Each outcome results from two trials
E) All of the choices are correct are characteristics of the binomial distribution
A) Each outcome is mutually exclusive
B) Each trial is independent
C) Probability of success remains constant from trial to trial
D) Each outcome results from two trials
E) All of the choices are correct are characteristics of the binomial distribution

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
23
In a large metropolitan area, past records revealed that 30 percent of all the high school graduates go to college. From 20 graduates selected at random, what is the probability that exactly 8 will go to college?
A) 0.114
B) 0.887
C) 0.400
D) 0.231
E) None of the choices are correct
A) 0.114
B) 0.887
C) 0.400
D) 0.231
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
24
Judging from recent experience, 5 percent of the computer keyboards produced by an automatic, high- speed machine are defective. What is the probability that out of six keyboards selected at random, exactly zero keyboards will be defective?
A) 0.001
B) 0.167
C) 0.735
D) 0.500
E) None of the choices are correct
A) 0.001
B) 0.167
C) 0.735
D) 0.500
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
25
What must you know to develop a binomial probability distribution?
A) Probability of success
B) Number of trials
C) Number of successes
D) "a" and "b" only
E) "a" and "c" only
A) Probability of success
B) Number of trials
C) Number of successes
D) "a" and "b" only
E) "a" and "c" only

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
26
A true-false test consists of six questions. If you guess the answer to each question, what is the probability of getting all six questions correct?
A) 0
B) 0.016
C) 0.062
D) 0.250
E) None of the choices are correct
A) 0
B) 0.016
C) 0.062
D) 0.250
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
27
Chances are 50-50 that a newborn baby will be a girl. For families with five children, what is the probability that all the children are girls?
A) 0.100
B) 0.031
C) 0.001
D) 0.250
E) None of the choices are correct
A) 0.100
B) 0.031
C) 0.001
D) 0.250
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
28
The information below is the number of daily emergency assists made to skiers by the volunteer ski team at Alpine Ski Lodge for the last 50 days. To explain, there were 22 days on which there were 2 emergency assists, and 9 days on which there were 3 emergency assists. 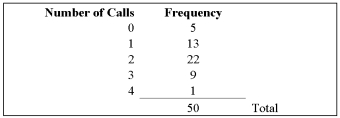 Convert this information to a probability distribution, and determine the mean number of assists per day.
Convert this information to a probability distribution, and determine the mean number of assists per day.
A) 1.56
B) 1.7
C) 1.66
D) 1.76
E) 1.77
 Convert this information to a probability distribution, and determine the mean number of assists per day.
Convert this information to a probability distribution, and determine the mean number of assists per day.A) 1.56
B) 1.7
C) 1.66
D) 1.76
E) 1.77

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
29
A multiple-choice test consists of five questions, each with A-E answers. If you guess the answer to each question, what is the probability of getting three or more questions correct?
A) 5.1%
B) 0.64%
C) 0.032%
D) 5.79%
E) None of the choices are correct
A) 5.1%
B) 0.64%
C) 0.032%
D) 5.79%
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
30
A multiple-choice test consists of six questions, each with A-E answers. If you guess the answer to each question, how many questions can you expect to get correct?
A) 1
B) 3
C) 2
D) 1.2
E) None of the choices are correct
A) 1
B) 3
C) 2
D) 1.2
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
31
A true-false test consists of five questions. If you guess the answer to each question, what is the probability of getting three or more questions correct?
A) 15.6%
B) 31.25%
C) 50%
D) 100%
E) None of the choices are correct
A) 15.6%
B) 31.25%
C) 50%
D) 100%
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
32
A true-false test consists of five questions. If you guess the answer to each question, what is the probability of getting all five questions correct?
A) 0%
B) 3.1%
C) 6.2%
D) 100%
E) None of the choices are correct
A) 0%
B) 3.1%
C) 6.2%
D) 100%
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
33
A multiple-choice test consists of five questions, each with A-E answers. If you guess the answer to each question, what is the probability of getting four or more questions correct?
A) 5.1%
B) 0.64%
C) 0.032%
D) Less than 1%
E) None of the choices are correct
A) 5.1%
B) 0.64%
C) 0.032%
D) Less than 1%
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
34
Let X represent the number of children in a Canadian household. The probability distribution of X is as follows: 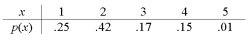 Determine the expected number of children in a randomly selected Canadian household.
Determine the expected number of children in a randomly selected Canadian household.
A) 2.25
B) 2.0
C) 2.5
D) 2.75
E) 3.0
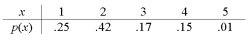 Determine the expected number of children in a randomly selected Canadian household.
Determine the expected number of children in a randomly selected Canadian household.A) 2.25
B) 2.0
C) 2.5
D) 2.75
E) 3.0

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
35
Sixty percent of the customers of a fast food chain order the Whopper, french fries and a drink. If a random sample of 15 cash register receipts is selected, what is the probability that 10 or more will show that the above three food items were ordered?
A) 1,000
B) 0.186
C) 0.403
D) 0.000
E) None of the choices are correct
A) 1,000
B) 0.186
C) 0.403
D) 0.000
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
36
On a very hot summer day, 5 percent of the production employees at Midland States Steel are absent from work. The production employees are to be selected at random for a special in-depth study on absenteeism. What is the probability of selecting 10 production employees at random on a hot summer day and finding that none of them are absent?
A) 0.002
B) 0.344
C) 0.599
D) 0.100
E) None of the choices are correct
A) 0.002
B) 0.344
C) 0.599
D) 0.100
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
37
Which is true for a binomial distribution?
A) There are three or more possible outcomes
B) Probability of success remains the same from trial to trial
C) Value of p is equal to 1.50
D) All of the choices are correct are correct
E) None of the choices are correct are correct
A) There are three or more possible outcomes
B) Probability of success remains the same from trial to trial
C) Value of p is equal to 1.50
D) All of the choices are correct are correct
E) None of the choices are correct are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
38
i. A binomial distribution has a characteristic that an outcome of an experiment is classified into one of two mutually exclusive categories (a success or a failure).
ii. A binomial distribution has the characteristic that the probability of a success stays the same for each trial, but the probability of a failure varies from trial to trial.
iii. The mean of a binomial probability distribution can be determined by multiplying the probability of a failure by the number of trials.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
ii. A binomial distribution has the characteristic that the probability of a success stays the same for each trial, but the probability of a failure varies from trial to trial.
iii. The mean of a binomial probability distribution can be determined by multiplying the probability of a failure by the number of trials.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
39
Which one of the following is NOT a condition of the binomial distribution?
A) Independent trials
B) Only two outcomes
C) Probability of success remains constant from trial to trial
D) At least 10 observations
E) None of the choices are correct
A) Independent trials
B) Only two outcomes
C) Probability of success remains constant from trial to trial
D) At least 10 observations
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
40
i. The mean of a binomial distribution is the product of the probability of success and the number of repetitions of the experiment.
ii. The binomial probability distribution is always negatively skewed.
Iii) A binomial distribution has the characteristic that the probability of a success stays the same for each trial, but the probability of a failure varies from trial to trial.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
ii. The binomial probability distribution is always negatively skewed.
Iii) A binomial distribution has the characteristic that the probability of a success stays the same for each trial, but the probability of a failure varies from trial to trial.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
41
Affirmative action commitments by industrial organizations have led to an increase in the number of women in executive positions. Satellite Office Systems has vacancies for two executives that it will fill from among four women and six men.
This is an example of what type of probability distribution?
A) Continuous probability distribution
B) Poisson probability distribution
C) Binomial probability distribution
D) Hypergeometric probability distribution
This is an example of what type of probability distribution?
A) Continuous probability distribution
B) Poisson probability distribution
C) Binomial probability distribution
D) Hypergeometric probability distribution

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
42
When surveyed for brand recognition, 98% of consumers recognize Coke. A new survey of 800 randomly selected consumers is to be conducted. For such a group of 800, determine the mean and standard deviation for the number who recognize the Coke brand name. Considering as unusual a result that differs from the mean by more than two standard deviations, it ( (is/is not) ) unusual to get 775 consumers who recognize the Coke brand name.
A) 16, 3.96, is not
B) 16, 16, is
C) 784, 3.96, is
D) 874, 16, is not
E) None of the choices are correct
A) 16, 3.96, is not
B) 16, 16, is
C) 784, 3.96, is
D) 874, 16, is not
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
43
If n = 100 and p = 1/5, determine the mean and standard deviation of this binomial distribution.
A) 20, 16
B) 20, 4
C) 500, 200
D) 200, 16
E) None of the choices are correct
A) 20, 16
B) 20, 4
C) 500, 200
D) 200, 16
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
44
Judging from recent experience, 5 percent of the computer keyboards produced by an automatic, high- speed machine are defective. If six keyboards are randomly selected, what is the probability that more than 3 of the keyboards are defective?
A) 0.167
B) 0.0001
C) 0.0000
D) 1.00
E) 0.2321
A) 0.167
B) 0.0001
C) 0.0000
D) 1.00
E) 0.2321

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
45
Judging from recent experience, 5 percent of the computer keyboards produced by an automatic, high- speed machine are defective. If six keyboards are randomly selected, what is the probability that none of the keyboards are defective?
A) 0.167
B) 0.735
C) 0.500
D) 1.00
E) 0.2321
A) 0.167
B) 0.735
C) 0.500
D) 1.00
E) 0.2321

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
46
Elly's hot dog emporium is famous for its chilidogs. Some customers order the hot dogs with hot peppers, while many do not care for that added bit of zest. Elly's latest sales indicate that 30% of
The customers ordering her chilidogs order it with hot peppers. Suppose 18 customers are selected at random. What is the probability that between two and six people inclusive want hot peppers?
A) 0.015
B) 0.15
C) 0.708
D) 0.807
E) None of the choices are correct
The customers ordering her chilidogs order it with hot peppers. Suppose 18 customers are selected at random. What is the probability that between two and six people inclusive want hot peppers?
A) 0.015
B) 0.15
C) 0.708
D) 0.807
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
47
Elly's hot dog emporium is famous for its chilidogs. Some customers order the hot dogs with hot peppers, while many do not care for that added bit of zest. Elly's latest sales indicate that 30% of the customers ordering her chilidogs order it with hot peppers. Suppose 18 customers are selected at random.
What is the probability that exactly ten customers will ask for hot peppers?
A) 0.015
B) 0.15
C) 0.708
D) 0.00
E) None of the choices are correct
What is the probability that exactly ten customers will ask for hot peppers?
A) 0.015
B) 0.15
C) 0.708
D) 0.00
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
48
Elly's hot dog emporium is famous for its chilidogs. Some customers order the hot dogs with hot peppers, while many do not care for that added bit of zest. Elly's latest sales indicate that 30% of
The customers ordering her chilidogs order it with hot peppers. Suppose 18 customers are selected at random. What is the probability that fifteen or more customers will want hot peppers?
A) 0.015
B) 0.15
C) 0.708
D) 0.807
E) None of the choices are correct
The customers ordering her chilidogs order it with hot peppers. Suppose 18 customers are selected at random. What is the probability that fifteen or more customers will want hot peppers?
A) 0.015
B) 0.15
C) 0.708
D) 0.807
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
49
Sixty percent of the customers of a fast food chain order a hamburger, french fries and a drink. If a random sample of 15 cash register receipts is selected, what is the probability that 10 or more will show that the above three food items were ordered?
A) 1.000
B) 0.7827
C) 0.9095
D) 0.2173
E) 0.0905
A) 1.000
B) 0.7827
C) 0.9095
D) 0.2173
E) 0.0905

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
50
David's gasoline station offers 4 cents off per litre if the customer pays in cash and does not use a credit card. Past evidence indicates that 40% of all customers pay in cash. During a one-hour period twenty-five customers buy gasoline at this station. This situation is an example of what type of discrete probability distribution?
A) Continuous probability distribution
B) Poisson probability distribution
C) Binomial probability distribution
D) Hypergeometric probability distribution
A) Continuous probability distribution
B) Poisson probability distribution
C) Binomial probability distribution
D) Hypergeometric probability distribution

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
51
Carlson Jewellers permits the return of their diamond wedding rings, provided the return occurs within two weeks of the purchase date. Their records reveal that 10 percent of the diamond wedding rings are returned. Five different customers buy five rings. What is the probability that all will be returned?
A) 0.00250
B) 0.59049
C) 0.00590
D) 0.00045
E) 0.00001
A) 0.00250
B) 0.59049
C) 0.00590
D) 0.00045
E) 0.00001

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
52
An insurance agent has appointments with four prospective clients tomorrow. From past experience the agent knows that the probability of making a sale on any appointment is 1 out of 5. Using the rules of probability, what is the likelihood that the agent will sell a policy to 3 of the 4 prospective clients?
A) 0.250
B) 0.500
C) 0.410
D) 0.026
E) None of the choices are correct
A) 0.250
B) 0.500
C) 0.410
D) 0.026
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
53
David's gasoline station offers 4 cents off per litre if the customer pays in cash and does not use a credit card. Past evidence indicates that 40% of all customers pay in cash. During a one-hour period twenty-five customers buy gasoline at this station.
What is the probability that more than ten and less than fifteen customers pay in cash?
A) 0.541
B) 0.401
C) 0.380
D) 0.562
E) None of the choices are correct
What is the probability that more than ten and less than fifteen customers pay in cash?
A) 0.541
B) 0.401
C) 0.380
D) 0.562
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
54
A multiple-choice test consists of ten questions, each with A-E answers. If you guess the answer to each question, how many questions can you expect to get correct? Also find the standard deviation of the number of questions you can expect to get correct.
A) 1, 1.6
B) 3, 1.3
C) 2, 1.3
D) 1.2, 1.6
E) None of the choices are correct
A) 1, 1.6
B) 3, 1.3
C) 2, 1.3
D) 1.2, 1.6
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
55
David's gasoline station offers 4 cents off per litre if the customer pays in cash and does not use a credit card. Past evidence indicates that 40% of all customers pay in cash. During a one-hour period twenty-five customers buy gasoline at this station.
What is the probability that at least ten pay in cash?
A) 0.416
B) 0.575
C) 0.586
D) 0.425
E) None of the choices are correct
What is the probability that at least ten pay in cash?
A) 0.416
B) 0.575
C) 0.586
D) 0.425
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
56
Sixty percent of the customers of a fast food chain order a hamburger, french fries and a drink. If a random sample of 15 cash register receipts is selected, what is the probability that 10 will show that the above three food items were ordered?
A) .1859
B) 0.7827
C) 0.2066
D) 0.2173
E) 0.4032
A) .1859
B) 0.7827
C) 0.2066
D) 0.2173
E) 0.4032

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
57
David's gasoline station offers 4 cents off per litre if the customer pays in cash and does not use a credit card. Past evidence indicates that 40% of all customers pay in cash. During a one-hour period twenty-five customers buy gasoline at this station.
What is the probability that no more than twenty pay in cash?
A) 0.0
B) 0.1
C) 0.9
D) 1.0
E) None of the choices are correct
What is the probability that no more than twenty pay in cash?
A) 0.0
B) 0.1
C) 0.9
D) 1.0
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
58
When surveyed for brand recognition, 98% of consumers recognize Coke. A new survey of 800 randomly selected consumers is to be conducted. For such a group of 800, determine the mean and standard deviation for the number who recognize the Coke brand name. Considering as unusual a result that differs from the mean by more than two standard deviations, it ( (is/is not) ) unusual to get 790 consumers who recognize the Coke brand name.
A) 16, 3.96, is not
B) 16, 16, is
C) 784, 3.96, is not
D) 784, 3.96, is
E) None of the choices are correct
A) 16, 3.96, is not
B) 16, 16, is
C) 784, 3.96, is not
D) 784, 3.96, is
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
59
Sixty percent of the customers of a fast food chain order a hamburger, french fries and a drink. If a random sample of 15 cash register receipts is selected, what is the probability that 10 or more will show that the above three food items were ordered?
A) 1.000
B) 0.7827
C) 0.9095
D) 0.2173
E) 0.0905
A) 1.000
B) 0.7827
C) 0.9095
D) 0.2173
E) 0.0905

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
60
If n = 900 and p = 1/3, determine the mean and standard deviation of this binomial distribution.
A) 2,700, 200
B) 2,700, 14.14
C) 300, 200
D) 300, 14.14
E) None of the choices are correct
A) 2,700, 200
B) 2,700, 14.14
C) 300, 200
D) 300, 14.14
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
61
Sweetwater & Associates write weekend trip insurance at a very nominal charge. Records show that the probability that a motorist will have an accident during the weekend and file a claim is 0.00555. Suppose they wrote 400 policies for the coming weekend, what is the probability that exactly two claims will be filed?
A) 0.8187
B) 0.2500
C) 0.0164
D) 0.0001
E) None of the choices are correct
A) 0.8187
B) 0.2500
C) 0.0164
D) 0.0001
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
62
Affirmative action commitments by industrial organizations have led to an increase in the number of women in executive positions. Satellite Office Systems has vacancies for two executives that it will fill from among four women and six men.
What is the probability that at least one woman is selected?
A) 8/15
B) 3/5
C) 2/3
D) 3/4
E) None of the choices are correct
What is the probability that at least one woman is selected?
A) 8/15
B) 3/5
C) 2/3
D) 3/4
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
63
Affirmative action commitments by industrial organizations have led to an increase in the number of women in executive positions. Satellite Office Systems has vacancies for two executives that it will fill from among four women and six men.
What is the probability that no woman is selected?
A) 1/5
B) 1/3
C) 2/15
D) 8/15
E) None of the choices are correct
What is the probability that no woman is selected?
A) 1/5
B) 1/3
C) 2/15
D) 8/15
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
64
An insurance agent has appointments with four prospective clients tomorrow. From past experience the agent knows that the probability of making a sale on any appointment is 1 out of 5. Using the rules of probability, what is the likelihood that the agent will sell a policy to at least 3 of the 4 prospective clients?
A) 0.0016
B) 0.4096
C) 0.0272
D) 0.0256
E) None of the choices are correct
A) 0.0016
B) 0.4096
C) 0.0272
D) 0.0256
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
65
How is a Poisson distribution skewed?
A) Positively
B) Negatively
C) Symmetrical
D) All of the choices are correct
E) None of the choices are correct
A) Positively
B) Negatively
C) Symmetrical
D) All of the choices are correct
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
66
The production department has installed a new spray machine to paint automobile doors. As is common with most spray guns, unsightly blemishes often appear because of improper mixture or other problems. A worker counted the number of blemishes on each door. Most doors had no blemishes; a few had one; a very few had two, and so on. The average number was 0.5 per door. The distribution
Of blemishes followed the Poisson distribution. Out of 10,000 doors painted, about how many would have no blemishes?
A) About 6,065
B) About 3,935
C) About 5,000
D) About 500
E) None of the choices are correct
Of blemishes followed the Poisson distribution. Out of 10,000 doors painted, about how many would have no blemishes?
A) About 6,065
B) About 3,935
C) About 5,000
D) About 500
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
67
A statistic professor finds she averages five e-mail messages per day from students. Assume the number of messages approximates a Poisson distribution.
What is the probability that on a randomly selected day she will have no messages?
A) 0.0067
B) zero
C) 0.0335
D) Impossible to have no messages
E) None of the choices are correct
What is the probability that on a randomly selected day she will have no messages?
A) 0.0067
B) zero
C) 0.0335
D) Impossible to have no messages
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
68
A manufacturer of headache medicine claims it is 70 percent effective within a few minutes. That is, out of every 100 users 70 get relief within a few minutes. A group of 12 patients are given the medicine. If the claim is true, what is the probability that 8 have relief within a few minutes?
A) 0.001
B) 0.168
C) 0.667
D) 0.231
E) None of the choices are correct
A) 0.001
B) 0.168
C) 0.667
D) 0.231
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
69
A statistic professor finds she averages five e-mail messages per day from students. Assume the number of messages approximates a Poisson distribution.
What is the probability that on a randomly selected day she will have two messages?
A) 0.0067
B) 0.0014
C) 0.420
D) 0.084
E) None of the choices are correct
What is the probability that on a randomly selected day she will have two messages?
A) 0.0067
B) 0.0014
C) 0.420
D) 0.084
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
70
A machine shop has 100 drill presses and other machines in constant use. The probability that a machine will become inoperative during a given day is 0.0555. During some days no machines are inoperative, but during some days, one, two, three or more are broken down. What is the probability that fewer than two machines will be inoperative during a particular day?
A) 0.0200
B) 0.1637
C) 0.8187
D) 0.9824
E) None of the choices are correct
A) 0.0200
B) 0.1637
C) 0.8187
D) 0.9824
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
71
Affirmative action commitments by industrial organizations have led to an increase in the number of women in executive positions. Satellite Office Systems has vacancies for two executives that it will fill from among four women and six men. What is the probability that exactly one woman is selected?
A) 8/15 = 0.533
B) 3/5 = 0.60
C) 2/3 = 0.667
D) 3/4 = 0.75
E) None of the choices are correct
A) 8/15 = 0.533
B) 3/5 = 0.60
C) 2/3 = 0.667
D) 3/4 = 0.75
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
72
A statistic professor finds she averages five e-mail messages per day from students. Assume the number of messages approximates a Poisson distribution.
What is the probability that on a randomly selected day she will have five messages?
A) 0.0067
B) 0.875
C) 0.175
D) 1.0
E) None of the choices are correct
What is the probability that on a randomly selected day she will have five messages?
A) 0.0067
B) 0.875
C) 0.175
D) 1.0
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
73
In which of the following discrete distribution does the probability of a success vary from one trial to the next?
A) Binomial
B) Poisson
C) Hypergeometric
D) All of the choices are correct
E) None of the choices are correct
A) Binomial
B) Poisson
C) Hypergeometric
D) All of the choices are correct
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
74
In a Poisson distribution the mean is equal to
A) n π.
B)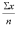 .
.
C) e - x.
D)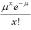 .
.
E) zero.
A) n π.
B)
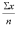 .
.C) e - x.
D)
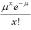 .
.E) zero.

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
75
Sweetwater & Associates write weekend trip insurance at a very nominal charge. Records show that the probability that a motorist will have an accident during the weekend and file a claim is 0.00555. Suppose they wrote 900 policies for the coming weekend, how many claims could they expect to be filed?
A) We have been given insufficient information to make such a prediction.
B) Sweetwater & Associates would normally expect to have 18 claims filed.
C) Sweetwater & Associates would normally expect to have 2 claims filed.
D) Sweetwater & Associates would be surprised to have a single claim filed.
A) We have been given insufficient information to make such a prediction.
B) Sweetwater & Associates would normally expect to have 18 claims filed.
C) Sweetwater & Associates would normally expect to have 2 claims filed.
D) Sweetwater & Associates would be surprised to have a single claim filed.

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
76
i. As a general rule of thumb, if the items selected for a sample are not replaced and the sample size is less than 5 percent of the population, the binomial distribution can be used to approximate the hypergeometric distribution.
Ii) If the probability of success does not remain the same from trial to trial when sampling is done without replacement, the hypergeometric distribution should be applied.
Iii) In the hypergeometric distribution the probability of a success is not the same on each trail.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false
Ii) If the probability of success does not remain the same from trial to trial when sampling is done without replacement, the hypergeometric distribution should be applied.
Iii) In the hypergeometric distribution the probability of a success is not the same on each trail.
A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
77
What is the only variable in the Poisson probability formula?
A) π
B) x
C) e
D) P
E) None of the choices are correct
A) π
B) x
C) e
D) P
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
78
A hybrid-grower is experiencing trouble with corn borers. A random check of 5,000 ears revealed the following: many of the ears contained no borers. Some ears had one borer; a few had two borers; and so on. The distribution of the number of borers per ear approximated the Poisson distribution. The grower counted 3,500 borers in the 5,000 ears. What is the probability that an ear of corn selected at random will contain no borers?
A) 0.3476
B) 0.4966
C) 1.000
D) 0.0631
E) None of the choices are correct
A) 0.3476
B) 0.4966
C) 1.000
D) 0.0631
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
79
Which of the following is a requirement for use of the hypergeometric distribution?
A) Only 2 possible outcomes
B) Trials are independent
C) Probability of a success is greater than 1.0
D) All of the choices are correct
E) None of the choices are correct is correct
A) Only 2 possible outcomes
B) Trials are independent
C) Probability of a success is greater than 1.0
D) All of the choices are correct
E) None of the choices are correct is correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck
80
Sweetwater & Associates write weekend trip insurance at a very nominal charge. Records show that the probability that a motorist will have an accident during the weekend and file a claim is 0.00555. Suppose they wrote 400 policies for the coming weekend, how many claims could they expect to be filed?
A) 200
B) 20
C) 2
D) 0.2
E) None of the choices are correct
A) 200
B) 20
C) 2
D) 0.2
E) None of the choices are correct

Unlock Deck
Unlock for access to all 111 flashcards in this deck.
Unlock Deck
k this deck



