Deck 13: Methods for Quality Improvement: Statistical Process Control Available on CD
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/89
Play
Full screen (f)
Deck 13: Methods for Quality Improvement: Statistical Process Control Available on CD
1
Does the following control chart represent a process that is in control or out of control? If it
is out of control, explain how you arrived at this conclusion.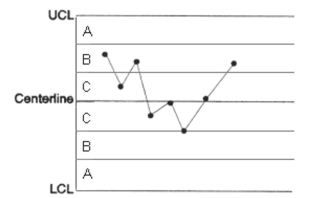
is out of control, explain how you arrived at this conclusion.

in control
2
Does the following control chart represent a process that is in control or out of control? If it is out of control, explain how you arrived at this conclusion.
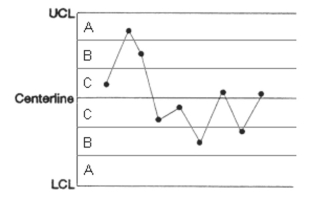

in control
3
(Situation E) A machine at K-Company fills boxes with bran flake cereal. The target weight for the filled boxes is 24 ounces. The company would like to use control charts to monitor the performance of the machine. The company decides to sample and weigh 10 consecutive boxes of cereal at randomly selected times over a two-week period. Twenty measurement times are selected and the following information is recorded.
-Find the upper and lower control limits for the R-chart.
-Find the upper and lower control limits for the R-chart.
Upper Control Limit
Lower Control Limit
Lower Control Limit
4
_______ are boundary points that define the acceptable values for an output variable.
A) Control bounds
B) Tolerance limits
C) Capability limits
D) Specification limits
A) Control bounds
B) Tolerance limits
C) Capability limits
D) Specification limits

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
5
(Situation D) A walk-in freezer thermostat at a restaurant is set at 5°F. Because of the perishability of the food in the freezer, the restaurant manager has decided to begin monitoring the temperature inside the freezer. The managers used a precision thermometer to take sample temperature readings at five randomly chosen times per day for 10 days. The data are presented below.
-Calculate the centerline of the R-chart.
-Calculate the centerline of the R-chart.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
6
The table below shows the data from samples of size randomly chosen from the outputs of a process on 20 different days.
a.
b.
c.
a.
b.
c.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
7
Does the following control chart represent a process that is in control or out of control? If it is out of control, explain how you arrived at this conclusion. 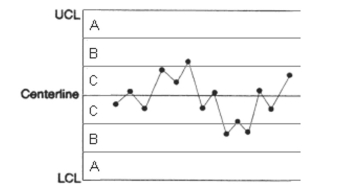


Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
8
Does the following control chart represent a process that is in control or out of control? If it is out of control, explain how you arrived at this conclusion. 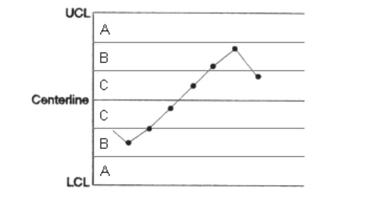


Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
9
(Situation E) A machine at K-Company fills boxes with bran flake cereal. The target weight for the filled boxes is 24
ounces. The company would like to use control charts to monitor the performance of the machine. The company decides
to sample and weigh 10 consecutive boxes of cereal at randomly selected times over a two-week period. Twenty
measurement times are selected and the following information is recorded.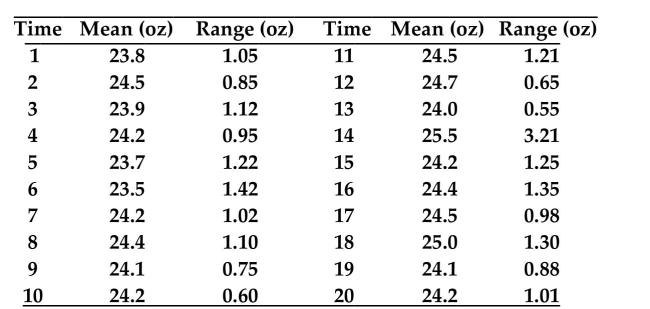
Calculate the centerline for constructing the
ounces. The company would like to use control charts to monitor the performance of the machine. The company decides
to sample and weigh 10 consecutive boxes of cereal at randomly selected times over a two-week period. Twenty
measurement times are selected and the following information is recorded.

Calculate the centerline for constructing the

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
10
The table below shows the data from samples of size randomly chosen from the outputs of a process on 20 different days. Assume the specification limits are USL and .
a. Assuming the process is under control, construct a capability analysis diagram for the process.
b. Find the percentage of data items that fall outside the specification limits.
c. Is the process capable? Support your answer with a numerical measure of capability.
a. Assuming the process is under control, construct a capability analysis diagram for the process.
b. Find the percentage of data items that fall outside the specification limits.
c. Is the process capable? Support your answer with a numerical measure of capability.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
11
(Situation E) A machine at K-Company fills boxes with bran flake cereal. The target weight for the filled boxes is 24 ounces. The company would like to use control charts to monitor the performance of the machine. The company decides to sample and weigh 10 consecutive boxes of cereal at randomly selected times over a two-week period. Twenty measurement times are selected and the following information is recorded.
-
-

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
12
The upper and lower control limits are usually a distance of _______ from the centerline.
A) 3 standard deviations
B) 1 standard deviation
C) 2 standard deviations
D) 3.5 standard deviations
A) 3 standard deviations
B) 1 standard deviation
C) 2 standard deviations
D) 3.5 standard deviations

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
13
The process of monitoring and eliminating variation in order to keep a process in a state of control or to bring a process into control is called
A) a process distribution.
B) random behavior.
C) statistical process control.
D) a control chart.
A) a process distribution.
B) random behavior.
C) statistical process control.
D) a control chart.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
14
(Situation B) A manufacturing company makes hemostats for hospital emergency rooms. The company is interested in implementing statistical process control procedures in its production operation. The production manager believes that the proportion of defective hemostats generated by the process is about 3%. The company collected one sample of 300 consecutively manufactured hemostats each day for 20 days.
The data are shown below.
-Construct the p-chart and interpret it.
The data are shown below.
-Construct the p-chart and interpret it.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
15
The capability index for a process centered on the desired mean is
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
16
Does the following control chart represent a process that is in control or out of control? If it is out of control, explain how you arrived at this conclusion. 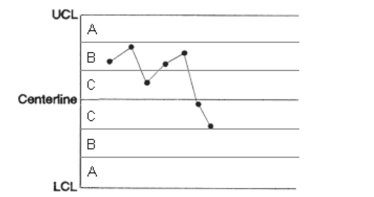


Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
17
(Situation E) A machine at K-Company fills boxes with bran flake cereal. The target weight for the filled boxes is 24 ounces. The company would like to use control charts to monitor the performance of the machine. The company decides to sample and weigh 10 consecutive boxes of cereal at randomly selected times over a two-week period. Twenty measurement times are selected and the following information is recorded.
-Does the following control chart represent a process that is in control or out of control? If it is out of control, explain how you arrived at this conclusion.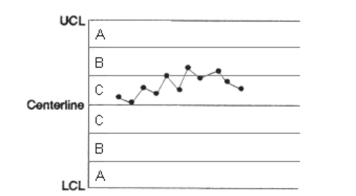
-Does the following control chart represent a process that is in control or out of control? If it is out of control, explain how you arrived at this conclusion.


Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
18
The primary goal of quality-improvement activities is
A) to reduce variation.
B) to increase the mean.
C) to increase variation.
D) to change the process.
A) to reduce variation.
B) to increase the mean.
C) to increase variation.
D) to change the process.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
19
A process is considered capable if
A)
B) .
C) .
D) .
A)
B) .
C) .
D) .

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
20
(Situation D) A walk-in freezer thermostat at a restaurant is set at 5°F. Because of the perishability of the food in the freezer, the restaurant manager has decided to begin monitoring the temperature inside the freezer. The managers used a precision thermometer to take sample temperature readings at five randomly chosen times per day for 10 days. The data are presented below.
-Calculate the upper and lower control limits for the R-chart.
-Calculate the upper and lower control limits for the R-chart.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
21
The quality of a product is indicated by the extent to which it satisfies the needs and preferences of its manufacturer.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
22
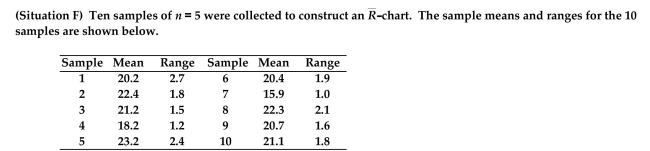
(Situation C) Ten samples of were collected to construct an -chart. The sample means and ranges for the 10 samples are shown below.
-Calculate the upper and lower control limits for the
-Calculate the upper and lower control limits for the

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
23
With rare exceptions, all items produced by a process are identical.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
24


Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
25
Does the following control chart represent a process that is in control or out of control? If it is out of control, explain how you arrived at this conclusion. 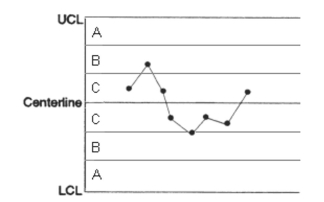


Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
26
(Situation E) A machine at K-Company fills boxes with bran flake cereal. The target weight for the filled boxes is 24 ounces. The company would like to use control charts to monitor the performance of the machine. The company decides to sample and weigh 10 consecutive boxes of cereal at randomly selected times over a two-week period. Twenty measurement times are selected and the following information is recorded.
-Calculate the upper and lower control limits for the
-Calculate the upper and lower control limits for the

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
27
(Situation E) A machine at K-Company fills boxes with bran flake cereal. The target weight for the filled boxes is 24 ounces. The company would like to use control charts to monitor the performance of the machine. The company decides to sample and weigh 10 consecutive boxes of cereal at randomly selected times over a two-week period. Twenty measurement times are selected and the following information is recorded.
-Calculate the centerline of the
-Calculate the centerline of the

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
28
Does the following control chart represent a process that is in control or out of control? If it is out of control, explain how you arrived at this conclusion. 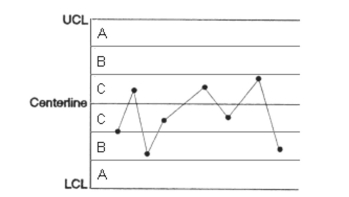


Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
29
(Situation B) A manufacturing company makes hemostats for hospital emergency rooms. The company is interested in implementing statistical process control procedures in its production operation. The production manager believes that the proportion of defective hemostats generated by the process is about 3%. The company collected one sample of 300 consecutively manufactured hemostats each day for 20 days. The data are shown below.
-Find the upper and lower control limits for the p-chart.
-Find the upper and lower control limits for the p-chart.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
30
(Situation D) A walk-in freezer thermostat at a restaurant is set at 5°F. Because of the perishability of the food in the freezer, the restaurant manager has decided to begin monitoring the temperature inside the freezer. The managers used a precision thermometer to take sample temperature readings at five randomly chosen times per day for 10 days. The data are presented below.
-Create the R-chart and interpret it.
-Create the R-chart and interpret it.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
31
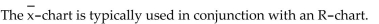

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
32
If all points fall between the control limits, then we may safely conclude that the process is in statistical control.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
33
Control charts are used to help us differentiate between process variation due to common causes and special causes.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
34
(Situation C) Ten samples of were collected to construct an -chart. The sample means and ranges for the 10 samples are shown below.
-Calculate the centerline for constructing the
-Calculate the centerline for constructing the

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
35
The R-chart is used to detect changes in process variation.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
36
The centerline of a control chart is drawn at the level of the median of the sample.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
37
People, machines, and raw materials can all contribute to the variability in the output of a system.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
38


Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
39
When n ≤ 6, the R-chart contains only one control limit, the lower control limit.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
40
The table below shows the data from samples of size randomly chosen from the outputs of a process on 20 different days.
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
41
When using a cause-and-effect diagram in process diagnosis, you begin by specifying the cause of interest and then move forward to identify potential effects of this cause.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
42
The elimination of common causes of variation is typically the responsibility of workers, not management.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
43
The p-chart is based on the assumption that the number of defective units in each sample is a binomial random variable.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
44
The variation in the output of processes that are out of control can be entirely attributed to random behavior.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
45
The distribution that describes the output variable of a process may change over time.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
46
Statistical process control consists of monitoring process variation, diagnosing causes of variation, and eliminating those causes.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
47
A process may be in control but still not be capable of producing output that is acceptable to customers.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
48
A process is in control and has a normally distributed output distribution with mean of 1000 and a standard deviation of 100 . The upper and lower specification limits for the process are 1060 and 940 , respectively. Find the value of the process. Is the system capable?
A) ; the system is capable.
B) ; the system is not capable.
C) ; the system is capable.
D) ; the system is not capable.
A) ; the system is capable.
B) ; the system is not capable.
C) ; the system is capable.
D) ; the system is not capable.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
49
Find the process spread when 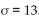
A) 39
B) 156
C) 26
D) 78
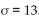
A) 39
B) 156
C) 26
D) 78

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
50
If the R-chart indicates that the process variation is in control, then it makes sense to construct and interpret the chart.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
51
An in-control, centered process that follows a normal distribution has a 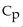 of 3.0. How many standard deviations away from the process mean is the upper specification limit?
of 3.0. How many standard deviations away from the process mean is the upper specification limit?
A) 9
B) 3
C) 18
D) 6
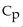 of 3.0. How many standard deviations away from the process mean is the upper specification limit?
of 3.0. How many standard deviations away from the process mean is the upper specification limit?A) 9
B) 3
C) 18
D) 6

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
52
An unbiased estimator for ? can be found by dividing the mean of the ranges, by an appropriate constant.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
53
Conformance refers to the extent to which a good or service can be adapted for use in new situations.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
54
Estimate the process spread when s 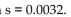
A) 0.0096
B) 0.0064
C) 0.0384
D) 0.0192
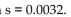
A) 0.0096
B) 0.0064
C) 0.0384
D) 0.0192

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
55
In constructing a p-chart, it is advisable to use a much smaller sample size than is typically used for and R-charts.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
56
The diagnosis phase of statistical process control is concerned with tracking down causes of variation.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
57
When constructing an -chart, is used as the estimator of .

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
58
Performance, reliability, and durability are some of the factors used to evaluate quality.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
59
A process adds value to the inputs of the process.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
60
(Situation B) A manufacturing company makes hemostats for hospital emergency rooms. The company is interested in implementing statistical process control procedures in its production operation. The production manager believes that the proportion of defective hemostats generated by the process is about 3%. The company collected one sample of 300
consecutively manufactured hemostats each day for 20 days. The data are shown below.
-Calculate the centerline used in constructing a
A) .0317
B) .0297
C) .0593
D) .0245
consecutively manufactured hemostats each day for 20 days. The data are shown below.
-Calculate the centerline used in constructing a
A) .0317
B) .0297
C) .0593
D) .0245

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
61
Capability analysis is used to determine when process variation is unacceptably high.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
62
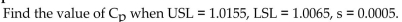
A) 0.3333
B) 18
C) 3
D) 0.0556

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
63
(Situation D) A walk-in freezer thermostat at a restaurant is set at 5°F. Because of the perishability of the food in the freezer, the restaurant manager has decided to begin monitoring the temperature inside the freezer. The managers used a precision thermometer to take sample temperature readings at five randomly chosen times per day for 10 days. The data
are presented below.
-Calculate the centerline for constructing the chart.
A) 5.3062
B) 10.245
C) 5.1224
D) 5.0700
are presented below.
-Calculate the centerline for constructing the chart.
A) 5.3062
B) 10.245
C) 5.1224
D) 5.0700

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
64
Estimate the process spread when s 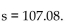
A) 321.24
B) 214.16
C) 1284.96
D) 642.48
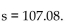
A) 321.24
B) 214.16
C) 1284.96
D) 642.48

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
65
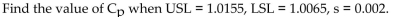
A) 0.375
B) 4.5
C) 0.75
D) 1.5

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
66
Most processes are naturally in a state of statistical control.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
67
(Situation A) To construct a p-chart for a manufacturing process, 20 samples of size 100 were selected. The results are shown below:
-Calculate the upper and lower control limits for the p-chart.
A)
B)
C)
D)
-Calculate the upper and lower control limits for the p-chart.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
68
The p-chart is typically used to monitor the proportion of units that conform to specification.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
69
Special causes of variation can often be diagnosed and eliminated by workers or their immediate supervisors.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
70
Find the specification spread when USL 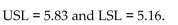
A) 0.77
B) 1.67
C) 2
D) 0.67
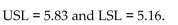
A) 0.77
B) 1.67
C) 2
D) 0.67

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
71
Control charts are the tool of choice for continuously monitoring processes.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
72
A business that operates out-of-control processes risks losing its customers and threatens its own survival.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
73
Control charts may only be used for quantitative quality variables.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
74
A system receives inputs from its environment, transforms those inputs to outputs, and delivers them to its environment.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
75
(Situation A) To construct a p-chart for a manufacturing process, 20 samples of size 100 were selected. The results areshown below:
-Calculate the centerline used in constructing a p-chart.
A) .0706
B) .0532
C) .0765
D) .0266
-Calculate the centerline used in constructing a p-chart.
A) .0706
B) .0532
C) .0765
D) .0266

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
76
The upper and lower control limits are positioned so that when the process is in control the probability of an individual value of the output variable falling outside the control limits is very large.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
77
If quality is designed into products and process management is used in their production, massinspection of finished products will not be necessary.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
78
If a capability analysis study indicates that an in-control process is not capable, it is usually off-centeredness, rather than variation, that is the culprit.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
79
Control limits and specification limits are essentially the same thing.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
80
Control charts are useful for evaluating the past performance of a process, for monitoring its current performance, and for predicting future performance.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck



