Deck 9: Introduction to Hypothesis Testing
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/183
Play
Full screen (f)
Deck 9: Introduction to Hypothesis Testing
1
In a one-tailed hypothesis test, the larger the significance level, the greater the critical value will be.
False
2
In hypothesis testing, the null hypothesis is the hypothesis that the researcher believes is true and wishes to prove.
False
3
A conclusion to "not reject" the null hypothesis is the same as the decision to "accept the null hypothesis".
False
4
In a hypothesis test, the p-value measures the probability that the alternative hypothesis is true.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
5
A sample is used to obtain a 95 percent confidence interval for the mean of a population. The confidence interval goes from 15 to 19. If the same sample had been used to test the null hypothesis that the mean of the population is equal to 20 versus the alternative hypothesis that the mean of the population differs from 20, the null hypothesis could be rejected at a level of significance of 0.05.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
6
In conducting a hypothesis test where the conclusion is to reject the null hypothesis, then either a correct decision has been made or else a Type I error.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
7
A local medical center has advertised that the mean wait for services will be less than 15 minutes. In an effort to test whether this claim can be substantiated, a random sample of 100 customers was selected and their wait times were recorded. The mean wait time was 17.0 minutes. Based on this sample result, there is sufficient evidence to reject the medical center's claim.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
8
Hypothesis testing and confidence interval estimation are essentially two totally different statistical procedures and share little in common with each other.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
9
Whenever possible, in establishing the null and alternative hypotheses, the research hypothesis should be made the alternative hypothesis.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
10
When using the t-distribution in a hypothesis test, the population does not need to be assumed normally distributed.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
11
A one-tailed hypothesis for a population mean with a significance level equal to .05 will have a critical value equal to z = .45.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
12
The following is an appropriate statement of the null and alternate hypotheses for a test of a population mean: H0 : μ = 45 HA : μ < 50

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
13
The null and alternate hypotheses must be opposites of each other.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
14
In a two-tailed hypothesis test the area of both tails in the rejection region is equal to α.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
15
If the sample data lead the decision maker to reject the null hypothesis, the alpha level is the maximum probability of committing a Type II error.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
16
The Adams Shoe Company believes that the mean size for men's shoes is now more than 10 inches. To test this, it has selected a random sample of n = 100 men. Assuming that the test is to be conducted using a .05 level of significance, a p-value of .07 would lead the company to conclude that its belief is correct.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
17
If a hypothesis test is conducted for a population mean, a null and alternative hypothesis of the form: H0 : μ = 100 HA : μ ≠ 100 will result in a one-tailed hypothesis test since the sample result can fall in only one tail.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
18
In testing a hypothesis, statements for the null and alternative hypotheses as well as the selection of the level of significance should precede the collection and examination of the data.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
19
When the decision maker has control over the null and alternative hypotheses, the alternative hypotheses should be the "research" hypothesis.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
20
A local medical center has advertised that the mean wait for services will be less than 15 minutes. Given this claim, the hypothesis test for the population mean should be a one-tailed test with the rejection region in the lower (left-hand) tail of the sampling distribution.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
21
If a hypothesis test leads to incorrectly rejecting the null hypothesis, a Type II statistical error has been made.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
22
The critical value in a null hypothesis test is called alpha.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
23
Of the two types of statistical errors, the one that decision makers have most control over is Type I error.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
24
Type II error is failing to reject the null hypothesis when the null is actually false.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
25
The significance level in a hypothesis test corresponds to the maximum probability that a Type I error will be committed.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
26
A toy store that has a 12% market share launches a marketing campaign. At the end of the campaign period the company conducts a survey in order to assess whether its market share has increased. If this claim is to be tested, the null and alternative hypotheses are: H0 : p = 12% Ha : p > 12%

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
27
The state insurance commissioner believes that the mean automobile insurance claim filed in her state exceeds $1,700. To test this claim, the agency has selected a random sample of 20 claims and found a sample mean equal to $1,733 and a sample standard deviation equal to $400. They plan to conduct the test using a 0.05 significance level. Based on this, the null hypothesis should be rejected if > $1,854.66 approximately.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
28
The state insurance commissioner believes that the mean automobile insurance claim filed in her state exceeds $1,700. To test this claim, the agency has selected a random sample of 20 claims and found a sample mean equal to $1,733 and a sample standard deviation equal to $400. They plan to conduct the test using a 0.05 significance level. Given this, the appropriate null and alternative hypotheses are H0 : ≤ $1,700 HA : > $1,700

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
29
The loan manager for State Bank and Trust has claimed that the mean loan balance on outstanding loans at the bank is over $14,500. To test this at a significance level of 0.05, a random sample of n = 100 loan accounts is selected. Assuming that the population standard deviation is known to be $3,000, the value of that corresponds to the critical value is approximately $14,993.50.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
30
If the probability of a Type I error is set at 0.05, then the probability of a Type II error will be 0.95.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
31
The director of the city Park and Recreation Department claims that the mean distance people travel to the city's greenbelt is more than 5.0 miles. Assume that the population standard deviation is known to be 1.2 miles and the significance level to be used to test the hypothesis is 0.05 when a sample size of n = 64 people are surveyed. Given this information, if the sample mean is 15.90 miles, the null hypothesis should be rejected.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
32
When a battery company claims that their batteries last longer than 100 hours and a consumer group wants to test this claim, the hypotheses should be: H0 : μ ≤ 100 HA : μ > 100

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
33
A large tire manufacturing company has claimed that its top line tire will average more than 80,000 miles. If a consumer group wished to test this claim, they would formulate the following null and alternative hypotheses: H0 : μ ≥ 80,000 Hα : μ ≠ 80,000

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
34
A report recently published in a major business periodical stated that the average salary for female managers is less than $50,000. If we were interested in testing this, the following null and alternative hypotheses would be established: H0 : μ ≥ 50,000 Hα : μ < 50,000

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
35
A large tire manufacturing company has claimed that its top line tire will average more than 80,000 miles. If a consumer group wished to test this claim, the research hypothesis would be: Ha : μ > 80,000 miles.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
36
The director of the city Park and Recreation Department claims that the mean distance people travel to the city's greenbelt is more than 5.0 miles. Assuming that the population standard deviation is known to be 1.2 miles and the significance level to be used to test the hypothesis is 0.05 when a sample size of n = 64 people are surveyed, the critical value is approximately 4.75 miles.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
37
A report recently submitted to the managing partner for a market research company stated "the hypothesis test may have resulted in either a Type I or a Type II error. We won't know which one occurred until later." This statement is one that we might correctly make for any hypothesis that we have conducted.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
38
The loan manager for State Bank and Trust has claimed that the mean loan balance on outstanding loans at the bank is over $14,500. To test this at a significance level of 0.05, a random sample of n = 100 loan accounts is selected. Assuming that the population standard deviation is known to be $3,000, the null and alternative hypotheses to be tested are: H0 : μ ≤ $14,500 HA : μ > $14,500

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
39
When someone has been accused of a crime the null hypothesis is: H0 : innocent. In this case, a Type I error would be convicting an innocent person.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
40
A two-tailed hypothesis test with α = 0.05 is similar to a 95 percent confidence interval.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
41
When using the p-value method for a two-tailed hypothesis, the p-value is found by finding the area in the tail beyond the test statistic, then doubling it.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
42
When using the p-value method, the null hypothesis is rejected when the calculated p-value > α.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
43
A two-tailed hypothesis test is used when the null hypothesis looks like the following: H0 : = 100.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
44
A company that makes and markets a device that is aimed at helping people quit smoking claims that at least 70 percent of the people who have used the product have quit smoking. To test this, a random sample of n = 100 product users was selected. Of these, 65 people were found to have quit smoking. Given these results, the test statistic value is z = -1.0911.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
45
One claim states the IRS conducts audits for not more than 5 percent of total tax returns each year. In order to test this claim statistically, the appropriate null and alternative hypotheses are: H0 : μ ≤ 0.05 Ha : μ > 0.05

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
46
A major package delivery company claims that at least 95 percent of the packages it delivers reach the destination on time. As part of the evidence in a lawsuit against the package company, a random sample of n = 200 packages was selected. A total of 188 of these packages were delivered on time. Using a significance level of .05, the test statistic for this test is approximately z = -0.65.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
47
When testing a hypothesis involving population proportions, an increase in sample size will result in a smaller chance of making a Type I statistical error.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
48
When deciding the null and alternative hypotheses, the rule of thumb is that if the claim contains the equality (e.g., at least, at most, no different from, etc.), the claim becomes the null hypothesis. If the claim does not contain the equality (e.g., less than, more than, different from), the claim is the alternative hypothesis.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
49
When the hypothesized proportion is close to 0.50, the spread in the sampling distribution of is greater than when the hypothesized proportion is close to 0.0 or 1.0.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
50
A cell phone company believes that 90 percent of their customers are satisfied. They survey a sample of n = 100 customers and find that 82 say they are satisfied. In calculating the standard error of the sampling distribution (σp) the proportion to use is 0.82.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
51
Aceco has a contract with a supplier to ship parts that contain no more than three percent defects. When a large shipment of parts comes in, Aceco samples n = 150. Based on the results of the sample, they either accept the shipment or reject it. If Aceco wants no more than a 0.10 chance of rejecting a good shipment, the cut-off between accepting and rejecting should be 0.0478 or 4.78 percent of the sample.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
52
The executive director of the United Way believes that more than 24 percent of the employees in the high-tech industry have made voluntary contributions to the United Way. In order to test this statistically, the appropriate null and alternative hypotheses are: H0 : ≤ .24 HA : > .24

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
53
Lube-Tech is a major chain whose primary business is performing lube and oil changes for passenger vehicles. The national operations manager has stated in an industry newsletter that the mean number of miles between oil changes for all passenger cars exceeds 4,200 miles. To test this, an industry group has selected a random sample of 100 vehicles that have come into a lube shop and determined the number of miles since the last oil change and lube. The sample mean was 4,278 and the standard deviation was known to be 780 miles. Based on this information, the p-value for the hypothesis test is less than 0.10.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
54
Lube-Tech is a major chain whose primary business is performing lube and oil changes for passenger vehicles. The national operations manager has stated in an industry newsletter that the mean number of miles between oil changes for all passenger cars exceeds 4,200 miles. To test this, an industry group has selected a random sample of 100 vehicles that have come into a lube shop and determined the number of miles since the last oil change and lube. The sample mean was 4,278 and the sample standard deviation was 780 miles. Based on this information, the test statistic is approximately t = 1.000.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
55
Generally, it is possible to appropriately test a null and alternative hypotheses using the test statistic approach and reach a different conclusion than would be reached if the p-value approach were used.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
56
A company that makes and markets a device that is aimed at helping people quit smoking claims that at least 70 percent of the people who have used the product have quit smoking. To test this, a random sample of n = 100 product users was selected. The critical value for the hypothesis test using a significance level of 0.05 would be approximately -1.645.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
57
Lube-Tech is a major chain whose primary business is performing lube and oil changes for passenger vehicles. The national operations manager has stated in an industry newsletter that the mean number of miles between oil changes for all passenger cars exceeds 4,200 miles. To test this, an industry group has selected a random sample of 100 vehicles that have come into a lube shop and determined the number of miles since the last oil change and lube. The sample mean was 4,278 and the standard deviation was known to be 780 miles. Based on a significance level of 0.10, the critical value for the test is approximately z = 1.28.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
58
A major package delivery company claims that at least 95 percent of the packages it delivers reach the destination on time. As part of the evidence in a lawsuit against the package company, a random sample of n = 200 packages was selected. A total of 188 of these packages were delivered on time. Using a significance level of 0.05, the critical value for this hypothesis test is approximately 0.90.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
59
A cell phone company believes that 90 percent of its customers are satisfied with their service. They survey n = 30 customers. Based on this, it is acceptable to assume the sample distribution is normally distributed.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
60
For testing a research hypothesis, the burden of proof that a new product is no better than the original is placed on the new product, and the research hypothesis is formulated as the null hypothesis.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
61
Which of the following would be an appropriate null hypothesis?
A) The mean of a population is equal to 55.
B) The mean of a sample is equal to 55.
C) The mean of a population is greater than 55.
D) The mean of a sample is greater than 55.
A) The mean of a population is equal to 55.
B) The mean of a sample is equal to 55.
C) The mean of a population is greater than 55.
D) The mean of a sample is greater than 55.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
62
If an economist wishes to determine whether there is evidence that average family income in a community near Seattle exceeds $125,000. An appropriate alternative hypothesis is:
A) μ = 125,000.
B) μ > 125,000.
C) μ ≤ 125,000.
D) μ ≥ 125,000.
A) μ = 125,000.
B) μ > 125,000.
C) μ ≤ 125,000.
D) μ ≥ 125,000.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
63
Which of the following statements is true?
A) The decision maker controls the probability of making a Type I statistical error.
B) Alpha represents the probability of making a Type II error.
C) Alpha and beta are directly related such that when one is increased the other will increase also.
D) The alternative hypothesis should contain the equality.
A) The decision maker controls the probability of making a Type I statistical error.
B) Alpha represents the probability of making a Type II error.
C) Alpha and beta are directly related such that when one is increased the other will increase also.
D) The alternative hypothesis should contain the equality.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
64
A major airline has stated in an industry report that its mean onground time between domestic flights is less than 18 minutes. To test this, the company plans to sample 36 randomly selected flights and use a significance level of 0.10. Assuming that the population standard deviation is known to be 4.0 minutes, the probability that the null hypothesis will be "accepted" if the true population mean is 16 minutes is approximately 0.955.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
65
When someone is on trial for suspicion of committing a crime, the hypotheses are: H0 : innocent HA : guilty Which of the following is correct?
A) Type I error is acquitting a guilty person.
B) Type I error is convicting an innocent person.
C) Type II error is acquitting an innocent person.
D) Type II error is convicting an innocent person.
A) Type I error is acquitting a guilty person.
B) Type I error is convicting an innocent person.
C) Type II error is acquitting an innocent person.
D) Type II error is convicting an innocent person.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
66
A city newspaper has stated that the average time required to sell a used car advertised in the paper is less than 5 days. Assuming that the population standard deviation is 2.1 days, if the "true" population mean is 4.1 days and a sample size of n = 49 is used with an alpha equal to 0.05, the probability that the hypothesis test will lead to a Type II error is approximately .0869.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
67
If a decision maker wishes to reduce the chance of making a Type II error, one option is to increase the sample size.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
68
In a hypothesis test involving a population mean, which of the following would be an acceptable formulation?
A)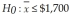
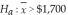
B)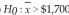
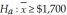
C)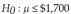
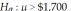
D) None of the above is a correct formulation.
A)
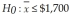
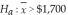
B)
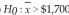
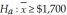
C)
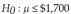
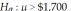
D) None of the above is a correct formulation.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
69
If a decision maker is concerned that the chance of making a Type II error is too large, one option that will help reduce the risk is to reduce the significance level.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
70
The director of the city Park and Recreation Department claims that the mean distance people travel to the city's greenbelt is more than 5.0 miles. Assuming that the population standard deviation is known to be 1.2 miles and the significance level to be used to test the hypothesis is 0.05 when a sample size of n = 64 people are surveyed, the probability of a Type II error is approximately .4545 when the "true" population mean is 5.5 miles.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
71
In a hypothesis test, increasing the sample size will generally result in a smaller chance of making a Type I error since sampling error is likely to be reduced.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
72
Choosing an alpha of 0.01 will cause beta to equal 0.99.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
73
To calculate beta requires making a "what if" assumption about the true population parameter, where the "what-if" value is one that would cause the null hypothesis to be false.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
74
The chance of making a Type II statistical error increases if the "true" population mean is closer to the hypothesized population mean, all other factors held constant.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
75
The probability of a Type II error decreases as the "true" population value gets farther from the hypothesized population value, given that everything else is held constant.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
76
An article in an operations management journal recently stated that a formal hypothesis test rejected the hypothesis that mean employee productivity was less than $45.70 per hour in the wood processing industry. Given this conclusion, it is possible that a Type I statistical error was committed.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
77
If we are performing a two-tailed test of whether μ = 100, the probability of detecting a shift of the mean to 105 will be ________ the probability of detecting a shift of the mean to 110.
A) less than
B) greater than
C) equal to
D) not comparable to
A) less than
B) greater than
C) equal to
D) not comparable to

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
78
Type II errors are typically greater for two-tailed hypothesis tests than for one-tailed tests.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
79
If the p value is less than α in a two-tailed test,
A) the null hypothesis should not be rejected.
B) the null hypothesis should be rejected.
C) a one-tailed test should be used.
D) More information is needed to reach a conclusion about the null hypothesis.
A) the null hypothesis should not be rejected.
B) the null hypothesis should be rejected.
C) a one-tailed test should be used.
D) More information is needed to reach a conclusion about the null hypothesis.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck
80
A major airline has stated in an industry report that its mean onground time between domestic flights is less than 18 minutes. To test this, the company plans to sample 36 randomly selected flights and use a significance level of .10. Assuming that the population standard deviation is known to be 4.0 minutes, if the true population mean is 16 minutes, the decision maker could end up making either a Type I or a Type II error depending on the sample result.

Unlock Deck
Unlock for access to all 183 flashcards in this deck.
Unlock Deck
k this deck



