Deck 7: Costs
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/34
Play
Full screen (f)
Deck 7: Costs
1
A firm producing hockey sticks has a production function given by 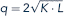
In the short run, the firm s amount of capital equipment is fixed at K = 100. The rental rate for K is v = $1, and the wage rate for L is w = $4.
a. Calculate the firm s short-run total cost function. Calculate the short-run average cost function.
b. The firm s short-run marginal cost function is given by SMC = q/50. What are the STC, SAC, and SMC for the firm if it produces 25 hockey sticks? Fifty hockey sticks? One hun-dred hockey sticks? Two hundred hockey sticks?
c. Graph the SAC and the SMC curves for the firm. Indicate the points found in part b.
d. Where does the SMC curve intersect the SAC curve? Explain why the SMC curve will always intersect the SAC at its lowest point.
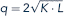
In the short run, the firm s amount of capital equipment is fixed at K = 100. The rental rate for K is v = $1, and the wage rate for L is w = $4.
a. Calculate the firm s short-run total cost function. Calculate the short-run average cost function.
b. The firm s short-run marginal cost function is given by SMC = q/50. What are the STC, SAC, and SMC for the firm if it produces 25 hockey sticks? Fifty hockey sticks? One hun-dred hockey sticks? Two hundred hockey sticks?
c. Graph the SAC and the SMC curves for the firm. Indicate the points found in part b.
d. Where does the SMC curve intersect the SAC curve? Explain why the SMC curve will always intersect the SAC at its lowest point.
The production function is given by: 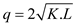 In the short run:
In the short run:
The capital equipment is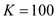 .
.
The rent rate is given by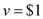 .
.
The wage rate is given by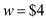 .
.
a) The production function is: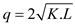 For
For 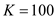
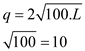 Therefore,
Therefore,  Short term total cost will be given by:
Short term total cost will be given by: 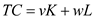 Therefore,
Therefore, 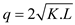 0
0
The Short Run Average Cost is given by: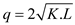 1
1
b) The Short Run Marginal Cost function is given by: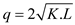 2
2
The STC for 25 hockey sticks will be: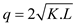 3
3
The SAC will be: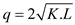 4
4
The SMC will be: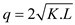 5
5
In case of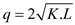 6
6 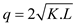 7
7
The SAC will be: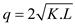 8
8
The SMC will be: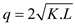 9
9
In case of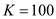 0
0 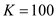 1
1 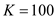 2
2 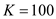 3
3
In case of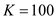 4
4 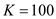 5
5 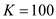 6
6 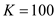 7
7
c) In the figure given below average and marginal cost are taken on the vertical axis and quantity is taken on the horizontal axis.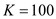 8
8
d) If the marginal cost is less than the average cost of all the previous units then the average cost will come down, similarly, if the marginal cost is greater than the average cost of all the previous units then the average cost will also increase, therefore, the MC and AC will intersect only where AC is minimum.
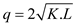 In the short run:
In the short run:The capital equipment is
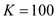 .
.The rent rate is given by
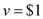 .
.The wage rate is given by
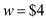 .
.a) The production function is:
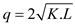 For
For 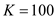
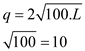 Therefore,
Therefore,  Short term total cost will be given by:
Short term total cost will be given by: 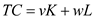 Therefore,
Therefore, 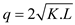 0
0The Short Run Average Cost is given by:
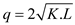 1
1b) The Short Run Marginal Cost function is given by:
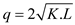 2
2The STC for 25 hockey sticks will be:
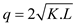 3
3The SAC will be:
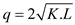 4
4The SMC will be:
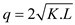 5
5In case of
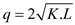 6
6 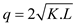 7
7The SAC will be:
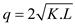 8
8The SMC will be:
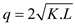 9
9In case of
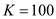 0
0 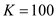 1
1 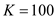 2
2 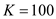 3
3In case of
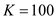 4
4 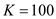 5
5 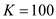 6
6 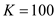 7
7c) In the figure given below average and marginal cost are taken on the vertical axis and quantity is taken on the horizontal axis.
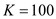 8
8d) If the marginal cost is less than the average cost of all the previous units then the average cost will come down, similarly, if the marginal cost is greater than the average cost of all the previous units then the average cost will also increase, therefore, the MC and AC will intersect only where AC is minimum.
2
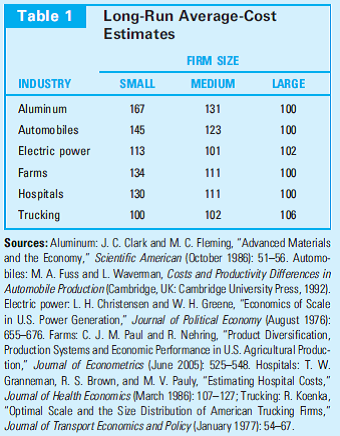
Findings on Firms' Average Costs
Most studies of firms' long-run costs have found that average-cost curves have a modified L-shape, such as the one shown in Figure 1. Average costs tend to decline as larger output levels are examined until some minimum efficient scale, q, is reached. Above that output, average costs tend to flatten out as larger scales of output are about equally efficient. Knowing about such cost patterns can often go a long way in explaining how industries evolve over time.
Some Empirical Evidence
Table 1 reports the results of representative studies of long-run average-cost curves for a variety of industries. Entries in the table represent the long-run average cost for a firm of a particular size (small, medium, or large) as a percentage of the minimal average-cost firm in the industry. For example, the data for hospitals indicate that small hospitals have average costs that are about 29:6 percent greater than average costs for large ones. This cost disadvantage of small hospitals can go a long way toward explaining the decline in rural hospitals in the United States in recent years. The large hospitals in the study appear to be large enough to exhaust available efficiencies.
Diseconomies of Scale
The only industry in Table 1 that appears to suffer cost disadvantages of large-scale operations is trucking. Higher costs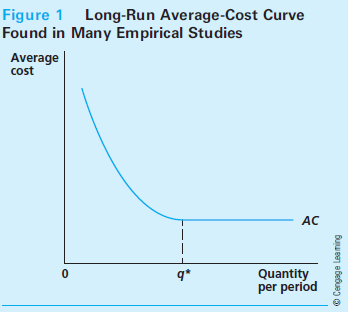
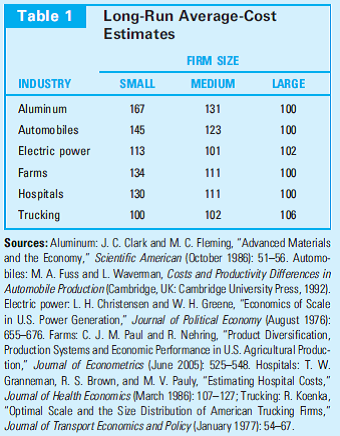
for large trucking firms may arise because they are more likely to be unionized or because it is harder to monitor many drivers' activities. In order to control their costs, many large trucking firms (especially package delivery firms like UPS or Federal Express) have adopted a number of efficiency-enhancing incentives for their drivers.
Is the minimum efficient scale for an industry constant over time? Choose one industry from the table and speculate on how technology may increase or decrease the minimum efficient scale in the future.
Most studies of firms' long-run costs have found that average-cost curves have a modified L-shape, such as the one shown in Figure 1. Average costs tend to decline as larger output levels are examined until some minimum efficient scale, q, is reached. Above that output, average costs tend to flatten out as larger scales of output are about equally efficient. Knowing about such cost patterns can often go a long way in explaining how industries evolve over time.
Some Empirical Evidence
Table 1 reports the results of representative studies of long-run average-cost curves for a variety of industries. Entries in the table represent the long-run average cost for a firm of a particular size (small, medium, or large) as a percentage of the minimal average-cost firm in the industry. For example, the data for hospitals indicate that small hospitals have average costs that are about 29:6 percent greater than average costs for large ones. This cost disadvantage of small hospitals can go a long way toward explaining the decline in rural hospitals in the United States in recent years. The large hospitals in the study appear to be large enough to exhaust available efficiencies.
Diseconomies of Scale
The only industry in Table 1 that appears to suffer cost disadvantages of large-scale operations is trucking. Higher costs


for large trucking firms may arise because they are more likely to be unionized or because it is harder to monitor many drivers' activities. In order to control their costs, many large trucking firms (especially package delivery firms like UPS or Federal Express) have adopted a number of efficiency-enhancing incentives for their drivers.
Is the minimum efficient scale for an industry constant over time? Choose one industry from the table and speculate on how technology may increase or decrease the minimum efficient scale in the future.
Minimum efficient scale is the scale of production where internal economies of scale have been fully exploited. This corresponds to the lowest point on the long run average cost curve.
Minimum efficient scale for an industry can be constant for a time period till the industry produces output at the minimum attainable average costs. Beyond the minimum efficient scale of production, as the industry continues to grow it will see no further cost benefits.
In order to determine the efficient scale of production, look at the growth of the industry from medium to large. For the Aluminum industry, the cost falls as the industry grows in size and starts using up its internal economies of scale. The cost falls further when the industry achieves a large scale with the improvement in technology and other factors.
Some of the other industries like Trucking do not see a decline in their average costs with an increase in the scale of production.
Minimum efficient scale for an industry can be constant for a time period till the industry produces output at the minimum attainable average costs. Beyond the minimum efficient scale of production, as the industry continues to grow it will see no further cost benefits.
In order to determine the efficient scale of production, look at the growth of the industry from medium to large. For the Aluminum industry, the cost falls as the industry grows in size and starts using up its internal economies of scale. The cost falls further when the industry achieves a large scale with the improvement in technology and other factors.
Some of the other industries like Trucking do not see a decline in their average costs with an increase in the scale of production.
3
The distinction between marginal and average cost can be made with some simple algebra. Here are three total cost functions:
i. TC = 10q
ii. TC = 40 + 10q
iii. TC = -40 + 10q
a. Explain why all three of these functions have the same marginal cost (10).
b. How does average cost compare to marginal cost for these three functions? (Note that average cost is only meaningful for q 4 for function iii.)c. Explain why average cost approaches marginal cost for large values of q.
d. Graph the average and marginal cost curves for these three functions. Explain the role of the constant term in the functions.
i. TC = 10q
ii. TC = 40 + 10q
iii. TC = -40 + 10q
a. Explain why all three of these functions have the same marginal cost (10).
b. How does average cost compare to marginal cost for these three functions? (Note that average cost is only meaningful for q 4 for function iii.)c. Explain why average cost approaches marginal cost for large values of q.
d. Graph the average and marginal cost curves for these three functions. Explain the role of the constant term in the functions.
The given total cost functions are:
i)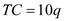 ii)
ii) 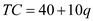 iii)
iii) 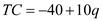 (a)All these functions have the same marginal cost because the marginal cost is determined by the variable costs and the variable costs is same in each of the three functions; hence the marginal cost of all the three functions is same.
(a)All these functions have the same marginal cost because the marginal cost is determined by the variable costs and the variable costs is same in each of the three functions; hence the marginal cost of all the three functions is same.
(b)The average cost and the marginal cost of the first function is: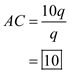
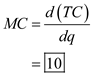 In this case, marginal cost and average cost are equal to each other.
In this case, marginal cost and average cost are equal to each other.
The average cost and the marginal cost of the second function is: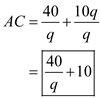
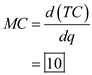 In this case, Average cost is greater than marginal cost at each positive level of output. As quantity tends to infinity, then only the average cost and marginal cost becomes equals.
In this case, Average cost is greater than marginal cost at each positive level of output. As quantity tends to infinity, then only the average cost and marginal cost becomes equals.
The average cost and the marginal cost of the third function is: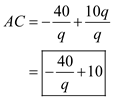
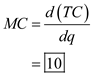 In this case, Average cost is less than marginal cost at each positive level of output. As quantity tends to infinity, then only the average cost and marginal cost becomes equals.
In this case, Average cost is less than marginal cost at each positive level of output. As quantity tends to infinity, then only the average cost and marginal cost becomes equals.
(c)The AC would approach the MC for large values of q ' s , because in case of really large values of q , the fixed cost component would approach 0 and AC would approach MC.
(d)The graph below shows the total cost, marginal cost and average cost for all the three functions:
Graph of TC =10 q is as follows: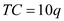 0
0
The graph of TC =40+10 q is as follows: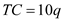 1
1
The graph of TC =-40+10 q is as follows: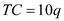 2
2
i)
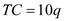 ii)
ii) 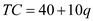 iii)
iii) 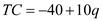 (a)All these functions have the same marginal cost because the marginal cost is determined by the variable costs and the variable costs is same in each of the three functions; hence the marginal cost of all the three functions is same.
(a)All these functions have the same marginal cost because the marginal cost is determined by the variable costs and the variable costs is same in each of the three functions; hence the marginal cost of all the three functions is same.(b)The average cost and the marginal cost of the first function is:
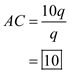
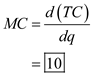 In this case, marginal cost and average cost are equal to each other.
In this case, marginal cost and average cost are equal to each other.The average cost and the marginal cost of the second function is:
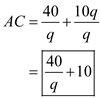
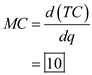 In this case, Average cost is greater than marginal cost at each positive level of output. As quantity tends to infinity, then only the average cost and marginal cost becomes equals.
In this case, Average cost is greater than marginal cost at each positive level of output. As quantity tends to infinity, then only the average cost and marginal cost becomes equals.The average cost and the marginal cost of the third function is:
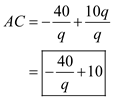
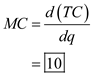 In this case, Average cost is less than marginal cost at each positive level of output. As quantity tends to infinity, then only the average cost and marginal cost becomes equals.
In this case, Average cost is less than marginal cost at each positive level of output. As quantity tends to infinity, then only the average cost and marginal cost becomes equals.(c)The AC would approach the MC for large values of q ' s , because in case of really large values of q , the fixed cost component would approach 0 and AC would approach MC.
(d)The graph below shows the total cost, marginal cost and average cost for all the three functions:
Graph of TC =10 q is as follows:
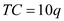 0
0The graph of TC =40+10 q is as follows:
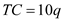 1
1The graph of TC =-40+10 q is as follows:
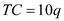 2
2 4
Findings on Firms' Average Costs
Most studies of firms' long-run costs have found that average-cost curves have a modified L-shape, such as the one shown in Figure 1. Average costs tend to decline as larger output levels are examined until some minimum efficient scale, q, is reached. Above that output, average costs tend to flatten out as larger scales of output are about equally efficient. Knowing about such cost patterns can often go a long way in explaining how industries evolve over time.
Some Empirical Evidence
Table 1 reports the results of representative studies of long-run average-cost curves for a variety of industries. Entries in the table represent the long-run average cost for a firm of a particular size (small, medium, or large) as a percentage of the minimal average-cost firm in the industry. For example, the data for hospitals indicate that small hospitals have average costs that are about 29:6 percent greater than average costs for large ones. This cost disadvantage of small hospitals can go a long way toward explaining the decline in rural hospitals in the United States in recent years. The large hospitals in the study appear to be large enough to exhaust available efficiencies.
Diseconomies of Scale
The only industry in Table 1 that appears to suffer cost disadvantages of large-scale operations is trucking. Higher costs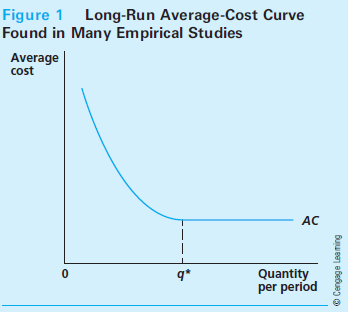
for large trucking firms may arise because they are more likely to be unionized or because it is harder to monitor many drivers' activities. In order to control their costs, many large trucking firms (especially package delivery firms like UPS or Federal Express) have adopted a number of efficiency-enhancing incentives for their drivers.
If small farms are inefficient, why haven't these disappeared? Could the localvore movement or the lure of the traditional farming lifestyle provide explanations?
Most studies of firms' long-run costs have found that average-cost curves have a modified L-shape, such as the one shown in Figure 1. Average costs tend to decline as larger output levels are examined until some minimum efficient scale, q, is reached. Above that output, average costs tend to flatten out as larger scales of output are about equally efficient. Knowing about such cost patterns can often go a long way in explaining how industries evolve over time.
Some Empirical Evidence
Table 1 reports the results of representative studies of long-run average-cost curves for a variety of industries. Entries in the table represent the long-run average cost for a firm of a particular size (small, medium, or large) as a percentage of the minimal average-cost firm in the industry. For example, the data for hospitals indicate that small hospitals have average costs that are about 29:6 percent greater than average costs for large ones. This cost disadvantage of small hospitals can go a long way toward explaining the decline in rural hospitals in the United States in recent years. The large hospitals in the study appear to be large enough to exhaust available efficiencies.
Diseconomies of Scale
The only industry in Table 1 that appears to suffer cost disadvantages of large-scale operations is trucking. Higher costs


for large trucking firms may arise because they are more likely to be unionized or because it is harder to monitor many drivers' activities. In order to control their costs, many large trucking firms (especially package delivery firms like UPS or Federal Express) have adopted a number of efficiency-enhancing incentives for their drivers.
If small farms are inefficient, why haven't these disappeared? Could the localvore movement or the lure of the traditional farming lifestyle provide explanations?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
5
Returning to the gumball producer in Problem 7.2, let's look at the possibility that producing these delectable treats does not necessarily experience constant returns to scale. 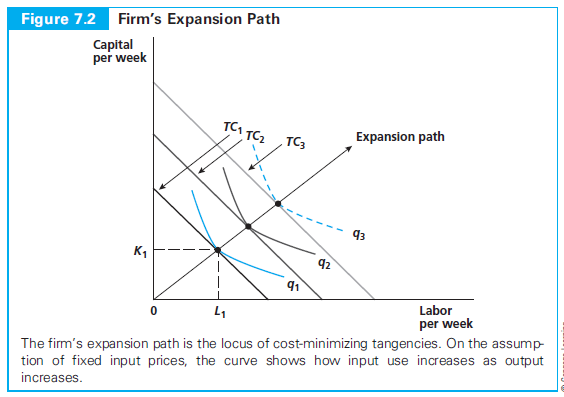
a. In Problem 7.2, we showed that the cost function for gumballs was given by TC =q (2v + w), where q is output of gumballs (in thousands), v is the rental rate for gumball presses, and w is the hourly wage. What sort of returns to scale did the underlying production function have? Does the cost function show economies or diseconomies of scale?
b. Suppose instead that the gumball cost function is given by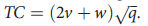 . Does this function show economies or diseconomies of scale? What does the graph of the total cost curve for this function look like? What do the implies average and marginal cost curves look like?
. Does this function show economies or diseconomies of scale? What does the graph of the total cost curve for this function look like? What do the implies average and marginal cost curves look like?
c. Suppose now that the gumball cost function is TC = (2v + w)q 2. Does this function show economies or diseconomies of scale? Illustrate this by graphing the total, average, and marginal cost curves for this function.
d. Economists sometimes measure the degree of economies of scale by S = AC/MC. This measure makes sense: if S 1, then AC MC, implying AC slopes up (because MC pulls it up), in which case we have diseconomies of scale. On the other hand, if S 1, then AC MC, implying AC slopes down (because MC pulls it down) and we have (positive) economies of scale. Suppose that the gumball production has associated total and marginal cost functions TC = (2v + w)q a and MC = a(2v + w)q a?1. Compute S in this case, and show how it relates to a. Discuss how the presence of economies or diseconomies of scale relates to a.

a. In Problem 7.2, we showed that the cost function for gumballs was given by TC =q (2v + w), where q is output of gumballs (in thousands), v is the rental rate for gumball presses, and w is the hourly wage. What sort of returns to scale did the underlying production function have? Does the cost function show economies or diseconomies of scale?
b. Suppose instead that the gumball cost function is given by
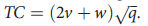 . Does this function show economies or diseconomies of scale? What does the graph of the total cost curve for this function look like? What do the implies average and marginal cost curves look like?
. Does this function show economies or diseconomies of scale? What does the graph of the total cost curve for this function look like? What do the implies average and marginal cost curves look like? c. Suppose now that the gumball cost function is TC = (2v + w)q 2. Does this function show economies or diseconomies of scale? Illustrate this by graphing the total, average, and marginal cost curves for this function.
d. Economists sometimes measure the degree of economies of scale by S = AC/MC. This measure makes sense: if S 1, then AC MC, implying AC slopes up (because MC pulls it up), in which case we have diseconomies of scale. On the other hand, if S 1, then AC MC, implying AC slopes down (because MC pulls it down) and we have (positive) economies of scale. Suppose that the gumball production has associated total and marginal cost functions TC = (2v + w)q a and MC = a(2v + w)q a?1. Compute S in this case, and show how it relates to a. Discuss how the presence of economies or diseconomies of scale relates to a.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
6
Some commuter groups argue that congestion tolls are unfair because they hit workers who have to commute at certain hours rather than those who drive off-peak in their spare time. Wouldn't a system of uniform (by time of day) tolls be fairer? Regardless of toll schedules, how should toll revenues be used?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
7
Young homeowners often get bad advice that confuses accounting and economic costs. What is the fallacy in each of the following pieces of advice? Can you alter the advice so that it makes sense?
1. Owning is always better than renting. Rent payments are just money down a "rat hole"-making house payments as an owner means that you are accumulating a real asset.
2. One should pay off a mortgage as soon as possible. Being able to close out your mortgage and burn the papers is one of the great economic joys of your life!
1. Owning is always better than renting. Rent payments are just money down a "rat hole"-making house payments as an owner means that you are accumulating a real asset.
2. One should pay off a mortgage as soon as possible. Being able to close out your mortgage and burn the papers is one of the great economic joys of your life!

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
8
Leonardo is a mechanically minded person who always builds things to help him understand his courses. To help in his understanding of average and marginal cost curves, he draws a TC-q axis pair on a board and attaches a thin wood pointer by a single nail through the origin. He now claims that he can find the level of output for which average cost is a minimum for any cost curve by the following mechanical process: (1) Draw the total cost curve on his graph; (2) rotate his pointer until it is precisely tangent to the total cost curve he has drawn; and (3) find the quantity that corresponds to this tangency. Leonardo claims that this is the quantity where average cost is minimized. Is he right? For which of the total cost curves in Figure would this procedure work? When would it not work?
Figure Possible Shapes of the Total Cost Curve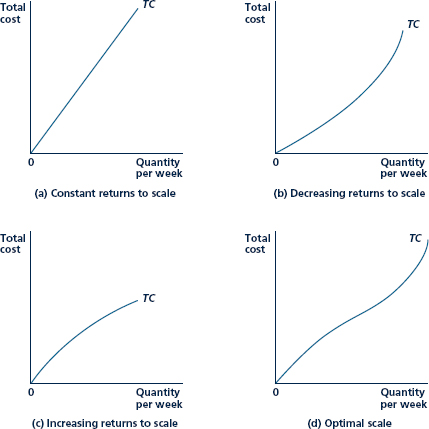
The shape of the total cost curve depends on the nature of the production function. Panela represents constant eturns to scale: As output expands, input costs expand proportionately. Panel b and panel c show decreasing returns to scale and increasing returns to scale, respectively. Panel d represents costs where the firm has an "optimal scale" of operations.
Figure Possible Shapes of the Total Cost Curve

The shape of the total cost curve depends on the nature of the production function. Panela represents constant eturns to scale: As output expands, input costs expand proportionately. Panel b and panel c show decreasing returns to scale and increasing returns to scale, respectively. Panel d represents costs where the firm has an "optimal scale" of operations.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
9
Standing in line at a theme park can certainly reduce the enjoyment of your visit. What are some of the ways that theme park operators have created incentives to use popular attractions at off-peak hours?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
10
A widget manufacturer has an infinitely substitu-table production function of the form
q = 2K + L
a. Graph the isoquant maps for q = 20, q = 40, and q = 60. What is the RTS along these iso-quants?
b. If the wage rate (w) is $1 and the rental rate on capital (v) is $1, what cost-minimizing combination of K and L will the manufacturer employ for the three different production levels in part a? What is the manufacturer's expansion path?
c. How would your answer to part b change if v rose to $3 with w remaining at $1?
q = 2K + L
a. Graph the isoquant maps for q = 20, q = 40, and q = 60. What is the RTS along these iso-quants?
b. If the wage rate (w) is $1 and the rental rate on capital (v) is $1, what cost-minimizing combination of K and L will the manufacturer employ for the three different production levels in part a? What is the manufacturer's expansion path?
c. How would your answer to part b change if v rose to $3 with w remaining at $1?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
11
Venture capitalist Sarah purchases two firms to produce widgets. Each firm produces identical products and each has a production function given by 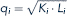
where
i=1,2
The firms differ, however, in the amount of capital equipment each has. In particular, firm 1 has K1 = 25, whereas firm 2 has K2 = 100. The marginal product of labor is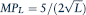 for firm 1, and
for firm 1, and  for firm 2. Rental rates for K and L are given by w = v = $1.
for firm 2. Rental rates for K and L are given by w = v = $1.
a. If Sarah wishes to minimize short-run total costs of widget production, how would output be allocated between the two firms?
b. Given that output is optimally allocated between the two firms, calculate the short-run total and average cost curves. What is the marginal cost of the 100th widget? The 125th widget? The 200th widget?
c. How should Sarah allocate widget production between the two firms in the long run? Calculate the long-run total and average cost curves for widget production.
d. How would your answer to part c change if both firms exhibited diminishing returns to scale?
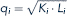
where
i=1,2
The firms differ, however, in the amount of capital equipment each has. In particular, firm 1 has K1 = 25, whereas firm 2 has K2 = 100. The marginal product of labor is
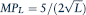 for firm 1, and
for firm 1, and  for firm 2. Rental rates for K and L are given by w = v = $1.
for firm 2. Rental rates for K and L are given by w = v = $1.a. If Sarah wishes to minimize short-run total costs of widget production, how would output be allocated between the two firms?
b. Given that output is optimally allocated between the two firms, calculate the short-run total and average cost curves. What is the marginal cost of the 100th widget? The 125th widget? The 200th widget?
c. How should Sarah allocate widget production between the two firms in the long run? Calculate the long-run total and average cost curves for widget production.
d. How would your answer to part c change if both firms exhibited diminishing returns to scale?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
12
What is so "special" about banks and their connection to the financial system? Should banks be subject to more regulations than should be applied to firms in other industries? What would be the underlying reason for such regulation and how might an efficient regulatory regime be designed? How does the global reach of banks complicate the regulatory problem?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
13
Trump Airlines is thinking of buying a new plane for its shuttle service. Why does the economist's notion of cost suggest that Trump should consider the plane's price in deciding whether it is a profitable investment but that, once bought, the plane's price is not directly relevant to Trump's profit-maximizing decisions? In such a case of "sunk costs," which cost should be used for deciding where to use the plane?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
14
Late Bloomer is taking a course in microeconomics. Grading in the course is based on 10 weekly quizzes, each with a 100-point maximum. On the first quiz, Late Bloomer receives a 10. In each succeeding week, he raises his score by 10 points, scoring a 100 on the final quiz of the year.
a. Calculate Late Bloomer's quiz average for each week of the semester. Why, after the first week, is his average always lower than his current week's quiz?
b. To help Late Bloomer, his kindly professor has decided to add 40 points to the total of his quiz scores before computing the average. Recompute Late Bloomer's weekly averages given this professorial gift.
c. Explain why Late Bloomer's weekly quiz averages now have a U-shape. What is his lowest average during the term?
d. Explain the relevance of this problem to the construction of cost curves. Why does the presence of a "fixed cost of 40 points result in a U-shaped curve? Are Late Bloomer s average and marginal test scores equal at his minimum average?
a. Calculate Late Bloomer's quiz average for each week of the semester. Why, after the first week, is his average always lower than his current week's quiz?
b. To help Late Bloomer, his kindly professor has decided to add 40 points to the total of his quiz scores before computing the average. Recompute Late Bloomer's weekly averages given this professorial gift.
c. Explain why Late Bloomer's weekly quiz averages now have a U-shape. What is his lowest average during the term?
d. Explain the relevance of this problem to the construction of cost curves. Why does the presence of a "fixed cost of 40 points result in a U-shaped curve? Are Late Bloomer s average and marginal test scores equal at his minimum average?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
15
Suppose a firm faces a wage rate of 10 and a capital rental rate of 4. In the following two situations, how much of each input should this firm hire in order to minimize the cost of producing an output of 100 units? What are the firm's total costs? How would the firm's total costs change if capital rental rates rose to 10?
1. The firm produces with a fixed-proportions production function that requires 0.1 labor hours and 0.2 machine hours for each unit of output.
2. The firm's production function is given by Q = 10L + 5K.
1. The firm produces with a fixed-proportions production function that requires 0.1 labor hours and 0.2 machine hours for each unit of output.
2. The firm's production function is given by Q = 10L + 5K.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
16
In Problem 6.7, we introduced the Cobb-Douglas production function of the form q = K a L b. The cost function that can be derived from this production function is 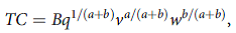
Where B is a constant, and v and w are the costs of K and L, respectively. The marginal cost function is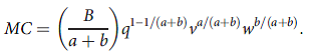
a. To understand these functions, suppose a = b = 0:5. Does this function exhibit constant returns to scale? What is the cost function now? Does the cost function exhibit economies or diseconomies of scale? How "important" are each of the input prices in this function?
b. Now return to the Cobb-Douglas cost function in its more general form. Discuss the role of the exponent of q. How does the value of this exponent relate to the returns to scale exhibited by its underlying production function? How do the returns to scale in the production function affect the shape of the firm's total cost curve?
c. Refer to problem 7.6d for a definition of S, a measure of the degree of economies of scale. Compute S for the general Cobb-Douglas form. Relate S to the exponent on q in the cost function and the returns to scale found in the previous part of this question.
d. Discuss how the relative sizes of a and b affect this cost function. Explain how the sizes of these exponents affect the extent to which the total cost function is shifted by changes in each of the input prices.
e. Taking natural logarithms of the Cobb-Douglas cost function yields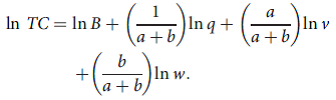
Why might this form of the function be especially useful? What do the coefficients of the log terms in the function tell you?
f. The cost function in part d can be generalized by adding more terms. This new function is called the "Translog Cost Function," and it is used in much empirical research. A nice introduction to the function is provided by the Christenson and Greene paper on electric power generation references in Table 1 of Application 7.3. The paper also contains an estimate of the Cobb-Douglas cost function that is of the general form given in part d. Can you find this in the paper?
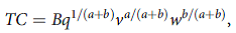
Where B is a constant, and v and w are the costs of K and L, respectively. The marginal cost function is
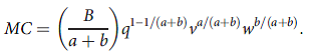
a. To understand these functions, suppose a = b = 0:5. Does this function exhibit constant returns to scale? What is the cost function now? Does the cost function exhibit economies or diseconomies of scale? How "important" are each of the input prices in this function?
b. Now return to the Cobb-Douglas cost function in its more general form. Discuss the role of the exponent of q. How does the value of this exponent relate to the returns to scale exhibited by its underlying production function? How do the returns to scale in the production function affect the shape of the firm's total cost curve?
c. Refer to problem 7.6d for a definition of S, a measure of the degree of economies of scale. Compute S for the general Cobb-Douglas form. Relate S to the exponent on q in the cost function and the returns to scale found in the previous part of this question.
d. Discuss how the relative sizes of a and b affect this cost function. Explain how the sizes of these exponents affect the extent to which the total cost function is shifted by changes in each of the input prices.
e. Taking natural logarithms of the Cobb-Douglas cost function yields
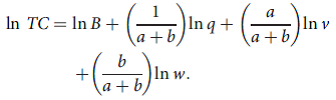
Why might this form of the function be especially useful? What do the coefficients of the log terms in the function tell you?
f. The cost function in part d can be generalized by adding more terms. This new function is called the "Translog Cost Function," and it is used in much empirical research. A nice introduction to the function is provided by the Christenson and Greene paper on electric power generation references in Table 1 of Application 7.3. The paper also contains an estimate of the Cobb-Douglas cost function that is of the general form given in part d. Can you find this in the paper?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
17
Suppose that the Acme Gumball Company has a fixed proportions production function that requires it to use two gumball presses and one worker to produce 1000 gumballs per hour.
a. Explain why the cost per hour of producing 1000 gumballs is 2v + w (where v is the hourly rent for gumball presses and w is the hourly wage).
b. Assume Acme can produce any number of gumballs they want using this technology. Explain why the cost function in this case would be TC = q(2v + w), where q is output of gumballs per hour, measured in thousands of gumballs.
c. What is the average and marginal cost of gum-ball production (again, measure output in thousands of gumballs)?
d. Graph the average and marginal cost curves for gumballs assuming v = 3, w = 5.
e. Now graph these curves for v = 6, w = 5. Explain why these curves have shifted.
a. Explain why the cost per hour of producing 1000 gumballs is 2v + w (where v is the hourly rent for gumball presses and w is the hourly wage).
b. Assume Acme can produce any number of gumballs they want using this technology. Explain why the cost function in this case would be TC = q(2v + w), where q is output of gumballs per hour, measured in thousands of gumballs.
c. What is the average and marginal cost of gum-ball production (again, measure output in thousands of gumballs)?
d. Graph the average and marginal cost curves for gumballs assuming v = 3, w = 5.
e. Now graph these curves for v = 6, w = 5. Explain why these curves have shifted.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
18
Beth is a mathematical whiz. She has been reading this chapter and remarks, "All this short-run/long-run stuff is a trivial result of the mathematical fact that the minimum value for any function must be as small as or smaller than the minimum value for the same function when some additional constraints are attached." Use Beth's insight to explain the following:
a. Why short-run total costs must be equal to or greater than long-run total costs for any given output level
b. Why short-run average cost must be equal to or greater than long-run average cost for any given output level
c. That you cannot make a definite statement about the relationship between short-run and long-run marginal cost
a. Why short-run total costs must be equal to or greater than long-run total costs for any given output level
b. Why short-run average cost must be equal to or greater than long-run average cost for any given output level
c. That you cannot make a definite statement about the relationship between short-run and long-run marginal cost

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
19
Farmer McDonald was heard to complain, "Although my farm is still profitable, I just can't afford to stay in this business any longer. I'm going to sell out and start a fast-food business." In what sense is McDonald using the word profitable here? Explain why his statement might be correct if he means profits in the accountant's sense but would be dubious if he is referring to economic profits.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
20
In the numerical example of Hamburger Heaven s production function in Chapter 6, we examined the consequences of the invention of a self-flipping burger that changed the production function to 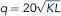
a. Assuming this shift does not change the cost-minimizing expansion path (which requires K = L), how are long-run total, average, and marginal costs affected? (See the numerical example at the end of Chapter 7.)b. More generally, technical progress in hamburger production might be reflected by
where r is the annual rate of technical progress (that is, a rate of increase of 3 percent would have r =.03). How will the year-to-year change in the average cost of a hamburger be related to the value of r?
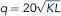
a. Assuming this shift does not change the cost-minimizing expansion path (which requires K = L), how are long-run total, average, and marginal costs affected? (See the numerical example at the end of Chapter 7.)b. More generally, technical progress in hamburger production might be reflected by

where r is the annual rate of technical progress (that is, a rate of increase of 3 percent would have r =.03). How will the year-to-year change in the average cost of a hamburger be related to the value of r?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
21
Suppose that there are to be 10 quizzes in your economics course. You have scored 80 on every one of the first 5 quizzes.
1. What will happen to your average for the course if your grade falls to 60 on each of the next 2 quizzes?
2. What will you have to score on the final 3 quizzes in the course to get your average back to 80?
3. Explain how this example illustrates the relationship between average and marginal costs studied in this section.
1. What will happen to your average for the course if your grade falls to 60 on each of the next 2 quizzes?
2. What will you have to score on the final 3 quizzes in the course to get your average back to 80?
3. Explain how this example illustrates the relationship between average and marginal costs studied in this section.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
22
Taxes can obviously affect firms' costs. Explain how each of the following taxes would affect total, average, and marginal cost. Be sure to consider whether the tax would have a different effect depending on whether one discusses short-run or long-run costs:
a. A franchise tax of $10,000 that the firm must pay in order to operate
b. An output tax of $2 on each unit of output
c. An employment tax on each worker s wages
d. A capital use tax on each machine the firm uses
a. A franchise tax of $10,000 that the firm must pay in order to operate
b. An output tax of $2 on each unit of output
c. An employment tax on each worker s wages
d. A capital use tax on each machine the firm uses

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
23
The long-run total cost function for a firm producing skateboards is 
Where q is the number of skateboards per week.
a. What is the general shape of this total cost function?
b. Calculate the average cost function for skateboards. What shape does the graph of this function have? At what level of skateboard output does average cost reach a minimum? What is the average cost at this level of output?
c. The marginal cost function for skateboards is given by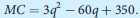
Show that this marginal cost curve intersects average cost at its minimum value.
d. Graph the average and marginal cost curves for skateboard production.

Where q is the number of skateboards per week.
a. What is the general shape of this total cost function?
b. Calculate the average cost function for skateboards. What shape does the graph of this function have? At what level of skateboard output does average cost reach a minimum? What is the average cost at this level of output?
c. The marginal cost function for skateboards is given by
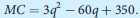
Show that this marginal cost curve intersects average cost at its minimum value.
d. Graph the average and marginal cost curves for skateboard production.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
24
In our numerical example, Hamburger Heaven s expansion path requires K = L because w (the wage) and v (the rental rate of grills) are equal. More generally, for this type of production function, it can be shown that
K /L = w/v
for cost minimization. Hence, relative input usage is determined by relative input prices.
a. Suppose both wages and grill rents rise to $10 per hour. How would this affect the firm s expansion path? How would long-run average and marginal cost be affected? What can you conclude about the effect of uniform inflation of input costs on the costs of hamburger pro-duction?
b. Suppose wages rise to $20 but grill rents stay fixed at $5. How would this affect the firm s expansion path? How would this affect the long-run average and marginal cost of hamburger production? Why does a multiplication of the wage by four result in a much smaller increase in average costs?
c. In the numerical example in Chapter 6, we explored the consequences of technical progress in hamburger flipping. Specifically, we assumed that the hamburger production function shifted for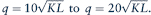 How would this shift offset the cost increases in part a? That is, what cost curves are implied by this new production function with v = w = 10? How do these compare with the original curves shown in Figure?
How would this shift offset the cost increases in part a? That is, what cost curves are implied by this new production function with v = w = 10? How do these compare with the original curves shown in Figure?
d. Answer part c with the input costs in part b of this problem (v = 5, w = 20). What do you conclude about the ability of technical progress to offset rising input costs?
Figure Total, Average, and Marginal Cost Curves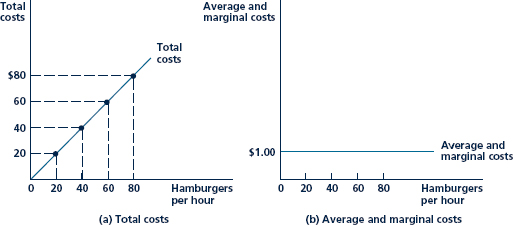
The total cost curve is simply a straight line through the origin reflecting constant returns to scale. Long-run average and marginal costs are constant at $1 per hamburger.
K /L = w/v
for cost minimization. Hence, relative input usage is determined by relative input prices.
a. Suppose both wages and grill rents rise to $10 per hour. How would this affect the firm s expansion path? How would long-run average and marginal cost be affected? What can you conclude about the effect of uniform inflation of input costs on the costs of hamburger pro-duction?
b. Suppose wages rise to $20 but grill rents stay fixed at $5. How would this affect the firm s expansion path? How would this affect the long-run average and marginal cost of hamburger production? Why does a multiplication of the wage by four result in a much smaller increase in average costs?
c. In the numerical example in Chapter 6, we explored the consequences of technical progress in hamburger flipping. Specifically, we assumed that the hamburger production function shifted for
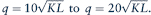 How would this shift offset the cost increases in part a? That is, what cost curves are implied by this new production function with v = w = 10? How do these compare with the original curves shown in Figure?
How would this shift offset the cost increases in part a? That is, what cost curves are implied by this new production function with v = w = 10? How do these compare with the original curves shown in Figure?d. Answer part c with the input costs in part b of this problem (v = 5, w = 20). What do you conclude about the ability of technical progress to offset rising input costs?
Figure Total, Average, and Marginal Cost Curves

The total cost curve is simply a straight line through the origin reflecting constant returns to scale. Long-run average and marginal costs are constant at $1 per hamburger.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
25
Explain why the assumption of cost minimization implies that the total cost curve must have a positive slope: An increase in output must always increase total cost.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
26
Use Figure to explain why a rise in the price of an input must increase the total cost of producing any given output level. What does this result suggest about how such a price increase shifts the AC curve? Do you think it is possible to draw any definite conclusion about how the MC curve would be affected?
Figure Minimizing the Costs of Producing q 1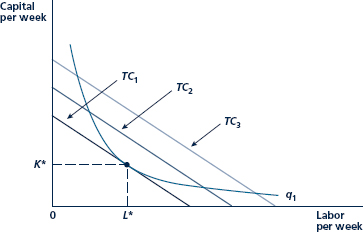
A firm is assumed to choose capital (K) and labor (L) to minimize total costs. The condition for this minimization is that the rate at which L can be substituted for K (while keeping q = q 1 ) should be equal to the rate at which these nputs can be traded in the market. In other words, the RTS (of L for K) should be set equal to the price ratio w/v. This tangency is shown here in that costs are minimized at TC1 by choosing inputs K* and L*.
Figure Minimizing the Costs of Producing q 1

A firm is assumed to choose capital (K) and labor (L) to minimize total costs. The condition for this minimization is that the rate at which L can be substituted for K (while keeping q = q 1 ) should be equal to the rate at which these nputs can be traded in the market. In other words, the RTS (of L for K) should be set equal to the price ratio w/v. This tangency is shown here in that costs are minimized at TC1 by choosing inputs K* and L*.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
27
Give an intuitive explanation for the following questions about Figure :
1. Why does SAC exceed AC for every level of output except q*?
2. Why does SMC exceed MC for output levels greater than q*?
3. What would happen to this figure if the firm increased its short-run level of capital beyond K*?
Figure Short-Run and Long-Run Average and Marginal Cost Curves at Optimal Output Level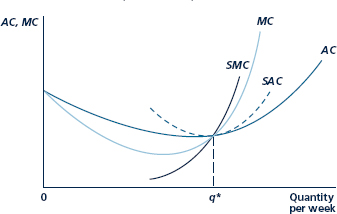
When long-run average cost is U-shaped and reaches a minimum at q*, SAC and SMC will also pass through this point. For increases in output above q*, short-run costs are higher than long-run costs.
1. Why does SAC exceed AC for every level of output except q*?
2. Why does SMC exceed MC for output levels greater than q*?
3. What would happen to this figure if the firm increased its short-run level of capital beyond K*?
Figure Short-Run and Long-Run Average and Marginal Cost Curves at Optimal Output Level

When long-run average cost is U-shaped and reaches a minimum at q*, SAC and SMC will also pass through this point. For increases in output above q*, short-run costs are higher than long-run costs.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
28
Many regulated firms believe that they had an "implicit contract" with tate regulators to ensure a fair return on their investments. What kind of incentives would such a contract provide to the firms in their decisions about what types of equipment to buy?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
29
Trapper Joe, the fur trader, has found that his production function in acquiring pelts is given by 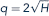
where q = the number of pelts acquired in a day, and H = the number of hours Joe s employees spend hunting and trapping in one day. Joe pays his employees $8 an hour.
a. Calculate Joe s total and average cost curves (as a function of q).
b. What is Joe s total cost for the day if he acquires four pelts? Six pelts? Eight pelts? What is Joe s average cost per pelt for the day if he acquires four pelts? Six pelts? Eight pelts?
c. Graph the cost curves from part a and indicate the points from part b. Explain why the cost curves have the shape they do.
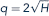
where q = the number of pelts acquired in a day, and H = the number of hours Joe s employees spend hunting and trapping in one day. Joe pays his employees $8 an hour.
a. Calculate Joe s total and average cost curves (as a function of q).
b. What is Joe s total cost for the day if he acquires four pelts? Six pelts? Eight pelts? What is Joe s average cost per pelt for the day if he acquires four pelts? Six pelts? Eight pelts?
c. Graph the cost curves from part a and indicate the points from part b. Explain why the cost curves have the shape they do.

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
30
How would the possibility that equipment may become obsolete be handled in unregulated markets? That is, how could this possibility be reflected in an unregulated firm's economic costs?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
31
Suppose a firm had a production function with linear isoquants, implying that its two inputs were perfect substitutes for each other. What would determine the firm's expansion path in this case? For the opposite case of a fixed-portions production function, what would the firm's expansion path be?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
32
Explain how different types of social responsibility policies might cause firms to opt for input choices such as A or C in Figure.
FIGURE Possible Extra Costs of Corporate Social Responsibility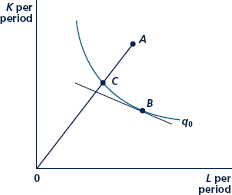
A firm pursuing socially responsible goals might opt to produce q0 using input combination A. This would involve more of both inputs than necessary (compare A to C) and use of an input combination that was not cost minimizing (compare C to B).
FIGURE Possible Extra Costs of Corporate Social Responsibility

A firm pursuing socially responsible goals might opt to produce q0 using input combination A. This would involve more of both inputs than necessary (compare A to C) and use of an input combination that was not cost minimizing (compare C to B).

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
33
An increase in the wages of fast-food workers will increase McDonald's costs.
1. How will the extent of the increase in McDonald's costs depend on whether labor costs account for a large or a small fraction of the firm's total costs?
2. How will the extent of the increase in McDonald's costs depend on whether the firm is able to substitute capital for labor?
1. How will the extent of the increase in McDonald's costs depend on whether labor costs account for a large or a small fraction of the firm's total costs?
2. How will the extent of the increase in McDonald's costs depend on whether the firm is able to substitute capital for labor?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck
34
Would a firm that followed socially responsible policies be violating its duty to its shareholders? Under what conditions might this be the case? When might it not be the case?

Unlock Deck
Unlock for access to all 34 flashcards in this deck.
Unlock Deck
k this deck



