Deck 10: Choices Involving Time
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/28
Play
Full screen (f)
Deck 10: Choices Involving Time
1
Garfield cares only about the amount of lasagna he eats this year and the amount of lasagna he eats next year. We will use L 0 to stand for lasagna this year and L 1 to stand for lasagna next year. His preferences correspond to the utility function U ( L 0 , L 1 ) = ( L 0 Z )( L 1 Z ), where Z is some fixed number. Suppose he earns $500 this year and nothing next year. Lasagna costs $10 per kilogram in both years and the interest rate (expressed as a decimal) is R. Find a formula showing how much Garfield will save as a function of the interest rate (as well as Z ). When the interest rate rises, does Garfield save more or less How does the answer depend upon Z
Let P 0 and P 1 stand for the price of lasagna this year and the price of lasagna next year, respectively. According to the information given,
 Write the slope of the budget line. The slope of the budget line is
Write the slope of the budget line. The slope of the budget line is
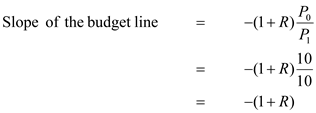 Find the marginal rate of substitution for lasagna this year with lasagna next year. To do that, first find the marginal utility of lasagna this year and the marginal utility of lasagna next year. The marginal utility of lasagna this year is the partial derivative of the utility function with respect to lasagna this year, i.e.,
Find the marginal rate of substitution for lasagna this year with lasagna next year. To do that, first find the marginal utility of lasagna this year and the marginal utility of lasagna next year. The marginal utility of lasagna this year is the partial derivative of the utility function with respect to lasagna this year, i.e.,

 The marginal utility of lasagna next year is the partial derivative of the utility function with respect to lasagna next year, i.e.,
The marginal utility of lasagna next year is the partial derivative of the utility function with respect to lasagna next year, i.e.,

 Now the marginal rate of substitution for lasagna this year with lasagna next year is
Now the marginal rate of substitution for lasagna this year with lasagna next year is
 The tangency condition for utility maximization is
The tangency condition for utility maximization is
 Write the equation for the budget line as
Write the equation for the budget line as
 Insert equation (1) in the above expression,
Insert equation (1) in the above expression,


 Let S stand for saving. The formula for saving can be found as
Let S stand for saving. The formula for saving can be found as
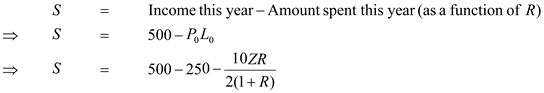
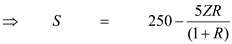 The above expression is saving as a function of interest rate.
The above expression is saving as a function of interest rate.
If the value of Z is positive, then, Garfield saves less as interest rate rises. If the value of Z is negative, then, Garfield saves more as interest rate falls.
 Write the slope of the budget line. The slope of the budget line is
Write the slope of the budget line. The slope of the budget line is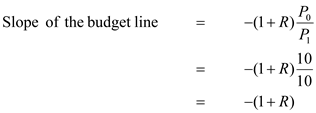 Find the marginal rate of substitution for lasagna this year with lasagna next year. To do that, first find the marginal utility of lasagna this year and the marginal utility of lasagna next year. The marginal utility of lasagna this year is the partial derivative of the utility function with respect to lasagna this year, i.e.,
Find the marginal rate of substitution for lasagna this year with lasagna next year. To do that, first find the marginal utility of lasagna this year and the marginal utility of lasagna next year. The marginal utility of lasagna this year is the partial derivative of the utility function with respect to lasagna this year, i.e., 
 The marginal utility of lasagna next year is the partial derivative of the utility function with respect to lasagna next year, i.e.,
The marginal utility of lasagna next year is the partial derivative of the utility function with respect to lasagna next year, i.e., 
 Now the marginal rate of substitution for lasagna this year with lasagna next year is
Now the marginal rate of substitution for lasagna this year with lasagna next year is The tangency condition for utility maximization is
The tangency condition for utility maximization is  Write the equation for the budget line as
Write the equation for the budget line as Insert equation (1) in the above expression,
Insert equation (1) in the above expression,

 Let S stand for saving. The formula for saving can be found as
Let S stand for saving. The formula for saving can be found as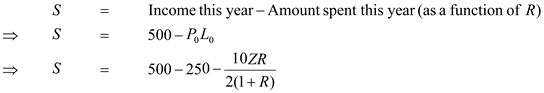
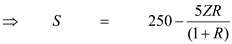 The above expression is saving as a function of interest rate.
The above expression is saving as a function of interest rate.If the value of Z is positive, then, Garfield saves less as interest rate rises. If the value of Z is negative, then, Garfield saves more as interest rate falls.
2
Interest rates are generally positive. Why do you think that is the case
Interest rates can be understood as the money one earns when one lends one's surplus funds. If interest rates would be negative, then people with surplus funds would have no incentive to lend their funds. This means supply of funds would then be zero. Therefore, for the credit markets to exist, it is necessary that interest rates be positive. The nominal interest rate is composing of real interest rate and inflation. Technically, nominal interest rate has to be positive, irrespective of real interest rate.
3
Calculate the PDV of: (a) $1 delivered 7 years from now if the interest rate is 5 percent; (b) $1 delivered 12 years from now if the interest rate is 7 percent; (c) $1 delivered 100 years from now if the interest rate is 2 percent.
The objective of the following analysis is to find the Present Discounted Value (PDV) of $1 delivered
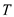 years from now assuming
years from now assuming
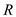 is the rate of interest.
is the rate of interest.
(a)Given data is:
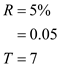 The PDV (Present Discounted Value) of $ 1 delivered 7 years from now, given that the interest rate is 5% is:
The PDV (Present Discounted Value) of $ 1 delivered 7 years from now, given that the interest rate is 5% is:
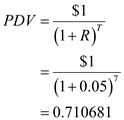 Hence, the PDV of $1 delivered in 7 years is $0.710681 or 71.07 cents
Hence, the PDV of $1 delivered in 7 years is $0.710681 or 71.07 cents
(b)Given data is:
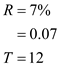 The PDV (Present Discounted Value) of $ 1 delivered 12 years from now, given that the interest rate is 7% is:
The PDV (Present Discounted Value) of $ 1 delivered 12 years from now, given that the interest rate is 7% is:
 Hence, the PDV of $1 delivered in 12 years is $0.444012 or 44.40 cents
Hence, the PDV of $1 delivered in 12 years is $0.444012 or 44.40 cents
(c)Given data is:
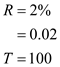 The PDV (Present Discounted Value) of $ 1 delivered 100 years from now, given that the interest rate is 2% is:
The PDV (Present Discounted Value) of $ 1 delivered 100 years from now, given that the interest rate is 2% is:
 Hence, the PDV of $1 delivered in 100 years is $0.138033 or 13.80 cents
Hence, the PDV of $1 delivered in 100 years is $0.138033 or 13.80 cents
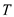 years from now assuming
years from now assuming 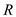 is the rate of interest.
is the rate of interest.(a)Given data is:
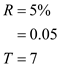 The PDV (Present Discounted Value) of $ 1 delivered 7 years from now, given that the interest rate is 5% is:
The PDV (Present Discounted Value) of $ 1 delivered 7 years from now, given that the interest rate is 5% is: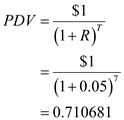 Hence, the PDV of $1 delivered in 7 years is $0.710681 or 71.07 cents
Hence, the PDV of $1 delivered in 7 years is $0.710681 or 71.07 cents(b)Given data is:
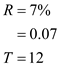 The PDV (Present Discounted Value) of $ 1 delivered 12 years from now, given that the interest rate is 7% is:
The PDV (Present Discounted Value) of $ 1 delivered 12 years from now, given that the interest rate is 7% is: Hence, the PDV of $1 delivered in 12 years is $0.444012 or 44.40 cents
Hence, the PDV of $1 delivered in 12 years is $0.444012 or 44.40 cents(c)Given data is:
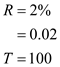 The PDV (Present Discounted Value) of $ 1 delivered 100 years from now, given that the interest rate is 2% is:
The PDV (Present Discounted Value) of $ 1 delivered 100 years from now, given that the interest rate is 2% is: Hence, the PDV of $1 delivered in 100 years is $0.138033 or 13.80 cents
Hence, the PDV of $1 delivered in 100 years is $0.138033 or 13.80 cents 4
In each of the following cases, calculate the balance in a bank account after the specified number of years, given the specified initial deposit and rate of interest.
a. $300 deposited at 8 percent interest for 15 years.
b. $4,200 deposited at 3 percent interest for 40 years.
c. $525 deposited at 6 percent interest for 5 years.
a. $300 deposited at 8 percent interest for 15 years.
b. $4,200 deposited at 3 percent interest for 40 years.
c. $525 deposited at 6 percent interest for 5 years.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
5
Charlie cares only about the amount of chocolate he eats this year and the amount of chocolate he eats next year. We will use C 0 to stand for chocolate this year and C 1 to stand for chocolate next year. His preferences correspond to the utility function
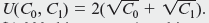 Suppose he earns $1,000 this year and nothing next year. Chocolate costs $2 per ounce this year, but will cost $4 per ounce next year. The interest rate (expressed as a decimal) is R. Find a formula showing how much Charlie will save as a function of the interest rate. When the interest rate rises, does Charlie save more or less
Suppose he earns $1,000 this year and nothing next year. Chocolate costs $2 per ounce this year, but will cost $4 per ounce next year. The interest rate (expressed as a decimal) is R. Find a formula showing how much Charlie will save as a function of the interest rate. When the interest rate rises, does Charlie save more or less
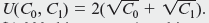 Suppose he earns $1,000 this year and nothing next year. Chocolate costs $2 per ounce this year, but will cost $4 per ounce next year. The interest rate (expressed as a decimal) is R. Find a formula showing how much Charlie will save as a function of the interest rate. When the interest rate rises, does Charlie save more or less
Suppose he earns $1,000 this year and nothing next year. Chocolate costs $2 per ounce this year, but will cost $4 per ounce next year. The interest rate (expressed as a decimal) is R. Find a formula showing how much Charlie will save as a function of the interest rate. When the interest rate rises, does Charlie save more or less
Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
6
During the 1984 presidential election campaign, Democratic hopeful Walter Mondale criticized the Reagan administration's economic record by arguing that high real interest rates were choking off investment and consumer spending. Vice-President George H.W. Bush responded famously that interest rates had fallen dramatically from a peak of 21 percent at the end of the Carter administration, and that "the real interest rate is what you pay when you go down and try to buy a TV set or buy a car." Evaluate the logic of Mr. Bush's argument.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
7
Calculate the PDV of promised payments for a 15 year bond with a $4,000 coupon and a face value of $50,000 at interest rates of 5 percent, 10 percent, and 15 percent. What if the interest rate is 0 percent

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
8
In each of the following cases, calculate the PDV of the specified amount under the specified circumstances.
a. $6,300 received in eight years, at an interest rate of 4 percent.
b. $115,000 received in two years, at an interest rate of 18 percent.
c. $45,000 received in 100 years, at an interest rate of 1 percent.
a. $6,300 received in eight years, at an interest rate of 4 percent.
b. $115,000 received in two years, at an interest rate of 18 percent.
c. $45,000 received in 100 years, at an interest rate of 1 percent.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
9
Lucy cares only about the amount of lemonade she drinks this year and the amount of lemonade she drinks next year. We will use L 0 to stand for lemonade this year and L 1 to stand for lemonade next year. Her preferences correspond to the utility function
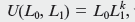 where k is a positive number. Suppose she earns $50 this year and $55 next year. Lemonade costs $1 per gallon in both years and the interest rate is 10 percent. Determine how much Lucy will save or borrow for the following values of k: 0.8, 0.9, 1.0, 1.1. Next, continue to assume that Lucy can save at an interest rate of 10 percent, but that she pays an interest rate of 25 percent when she borrows. Draw her budget line. Determine how much she will save or borrow for the same four values of k.
where k is a positive number. Suppose she earns $50 this year and $55 next year. Lemonade costs $1 per gallon in both years and the interest rate is 10 percent. Determine how much Lucy will save or borrow for the following values of k: 0.8, 0.9, 1.0, 1.1. Next, continue to assume that Lucy can save at an interest rate of 10 percent, but that she pays an interest rate of 25 percent when she borrows. Draw her budget line. Determine how much she will save or borrow for the same four values of k.
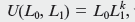 where k is a positive number. Suppose she earns $50 this year and $55 next year. Lemonade costs $1 per gallon in both years and the interest rate is 10 percent. Determine how much Lucy will save or borrow for the following values of k: 0.8, 0.9, 1.0, 1.1. Next, continue to assume that Lucy can save at an interest rate of 10 percent, but that she pays an interest rate of 25 percent when she borrows. Draw her budget line. Determine how much she will save or borrow for the same four values of k.
where k is a positive number. Suppose she earns $50 this year and $55 next year. Lemonade costs $1 per gallon in both years and the interest rate is 10 percent. Determine how much Lucy will save or borrow for the following values of k: 0.8, 0.9, 1.0, 1.1. Next, continue to assume that Lucy can save at an interest rate of 10 percent, but that she pays an interest rate of 25 percent when she borrows. Draw her budget line. Determine how much she will save or borrow for the same four values of k.
Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
10
People like to have a stable living standard from one year to the next. Yet studies show that the level of consumption drops suddenly, predictably, and significantly at retirement [contrary to Figure 10.9(a)]. Why Can you think of an explanation that is consistent with the approach to saving taken in Section 10.2

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
11
Suppose the nominal interest rate is 4 percent and the inflation rate is 1 percent. What is the real interest rate What if the nominal interest rate is 5 percent and the inflation rate is 2 percent In general, if the nominal interest rate and the inflation rate both rise by one percentage point, does the real interest rate change What about the approximate value of the real interest rate given by formula (8)

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
12
Calculate the PDV of the following bonds.
a. A 5-year bond with an annual coupon payment of $200, and a principle payment at maturity of $5,000, at an interest rate of 4 percent.
b. A 15-year bond with an annual coupon payment of $50, and a principle payment at maturity of $600, at an interest rate of 10 percent.
a. A 5-year bond with an annual coupon payment of $200, and a principle payment at maturity of $5,000, at an interest rate of 4 percent.
b. A 15-year bond with an annual coupon payment of $50, and a principle payment at maturity of $600, at an interest rate of 10 percent.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
13
Two mutually exclusive projects, A and B, require the same up-front investment in the first year. Project A generates positive net cash flows in years 2 through 5 and nothing after that. Project B generates nothing in years 2 through 5; positive net cash flows in years 6 through 10; and nothing after that. At an interest rate of 10 percent, project A is a better investment than project B. Explain intuitively why project A must be better than project B at higher interest rates as well.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
14
Assume that Angela earns nothing this year and $100 next year. To consume food this year, she must borrow. Repeat Worked-Out Problem 10.4. This time, write a formula for her borrowing as a function of the interest rate. According to your formula, does she borrow more or less as the interest rate rises Why does the interest rate affect borrowing in this exercise, even though it didn't affect saving in Worked-Out Problem 10.4
Worked-Out Problem 10.4

Worked-Out Problem 10.4


Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
15
Consider a bond with a face value of $1,000 and a coupon of $100. Suppose the interest rate is 10 percent. Does the PDV of the promised payments depend on the bond's maturity Why or why not (Hint: Think about putting $1,000 in a bank account that pays 10 percent interest. If you withdraw $100 at the end of each year, what do you always have left)

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
16
A project requires a cash investment in the first year and generates a positive net cash flow in all future years. Knowing only that Janet is more patient than Michael, would you say she is more likely to undertake the project than Michael Justify your answer.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
17
Compute the NPVs for the projects described in worked-out problem 10.4 at the following interest rates: 8 percent, 12 percent, 16 percent.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
18
Repeat Worked-Out Problem 10.4 (page 328), but assume that Angela earns $70 this year and $30 next year.
Worked-Out Problem 10.4

Worked-Out Problem 10.4


Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
19
Make your best guess about the various financial costs and benefits of attending your current educational institution (assuming that you complete your degree). Think about your likely earnings with and without the degree, the cost of tuition and books, and any other factors you consider relevant. Based on your estimates, compute the PDV and the IRR of your investment in human capital.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
20
Repeat Worked-Out Problem 10.4 (page 328), but assume that in Angela's view, one kg of food this year and one kg of food next year are perfect complements.. In other words, assume that her indifference curves are L-shaped, and that the corner of the L in each curve lies on the 45-degree line. How would you interpret her preferences
Worked-Out Problem 10.4

Worked-Out Problem 10.4


Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
21
(Calculus version below.) Garfield cares only about the amount of lasagna he eats this year and the amount of lasagna he eats next year. We will use L 0 to stand for lasagna this year and L 1 to stand for lasagna next year. His preferences correspond to the utility function U ( L 0 , L 1 ) = ( L 0 Z )( L 1 Z ), where Z is some fixed number. For that utility function, the marginal utility of lasagna this year is MU 0 = L 1 Z , and the marginal utility of lasagna next year is MU 1 = L 0 Z. Suppose he earns $500 this year and nothing next year. Lasagna costs $10 per kilogram in both years and the interest rate (expressed as a decimal) is R. Find a formula showing how much Garfield will save as a function of the interest rate (as well as Z ). When the interest rate rises, does Garfield save more or less How does the answer depend upon Z

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
22
(Calculus version below.) Charlie cares only about the amount of chocolate he eats this year and the amount of chocolate he eats next year. We will use C 0 to stand for chocolate this year and C 1 to stand for chocolate next year. His preferences correspond to the utility function
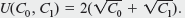 For that utility function, the marginal utility of chocolate this year is
For that utility function, the marginal utility of chocolate this year is
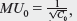 and the marginal utility of chocolate next year is MU 1 =
and the marginal utility of chocolate next year is MU 1 =
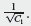 Suppose he earns $1,000 this year and nothing next year. Chocolate costs $2 per ounce this year, but will cost $4 per ounce next year. The interest rate (expressed as a decimal) is R. Find a formula showing how much Charlie will save as a function of the interest rate. When the interest rate rises, does Charlie save more or less
Suppose he earns $1,000 this year and nothing next year. Chocolate costs $2 per ounce this year, but will cost $4 per ounce next year. The interest rate (expressed as a decimal) is R. Find a formula showing how much Charlie will save as a function of the interest rate. When the interest rate rises, does Charlie save more or less
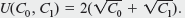 For that utility function, the marginal utility of chocolate this year is
For that utility function, the marginal utility of chocolate this year is 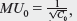 and the marginal utility of chocolate next year is MU 1 =
and the marginal utility of chocolate next year is MU 1 = 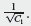 Suppose he earns $1,000 this year and nothing next year. Chocolate costs $2 per ounce this year, but will cost $4 per ounce next year. The interest rate (expressed as a decimal) is R. Find a formula showing how much Charlie will save as a function of the interest rate. When the interest rate rises, does Charlie save more or less
Suppose he earns $1,000 this year and nothing next year. Chocolate costs $2 per ounce this year, but will cost $4 per ounce next year. The interest rate (expressed as a decimal) is R. Find a formula showing how much Charlie will save as a function of the interest rate. When the interest rate rises, does Charlie save more or less
Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
23
(Calculus version below.) Lucy cares only about the amount of lemonade she drinks this year and the amount of lemonade she drinks next year. We will use L 0 to stand for lemonade this year and L 1 to stand for lemonade next year. Her preferences correspond to the utility function
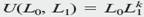 , where k is a positive number. For that utility function, the marginal utility of lemonade this year is
, where k is a positive number. For that utility function, the marginal utility of lemonade this year is
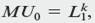 and the marginal utility of lemonade next year is
and the marginal utility of lemonade next year is
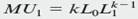 . Suppose she earns $50 this year and $55 next year. Lemonade costs $1 per gallon in both years and the interest rate is 10 percent. Determine how much Lucy will save or borrow for the following values of k: 0.8, 0.9, 1.0, 1.1. Next, continue to assume that Lucy can save at an interest rate of 10 percent, but that she pays an interest rate of 25 percent when she borrows. Draw her budget line. Determine how much she will save or borrow for the same four values of k.
. Suppose she earns $50 this year and $55 next year. Lemonade costs $1 per gallon in both years and the interest rate is 10 percent. Determine how much Lucy will save or borrow for the following values of k: 0.8, 0.9, 1.0, 1.1. Next, continue to assume that Lucy can save at an interest rate of 10 percent, but that she pays an interest rate of 25 percent when she borrows. Draw her budget line. Determine how much she will save or borrow for the same four values of k.
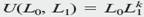 , where k is a positive number. For that utility function, the marginal utility of lemonade this year is
, where k is a positive number. For that utility function, the marginal utility of lemonade this year is 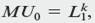 and the marginal utility of lemonade next year is
and the marginal utility of lemonade next year is 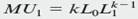 . Suppose she earns $50 this year and $55 next year. Lemonade costs $1 per gallon in both years and the interest rate is 10 percent. Determine how much Lucy will save or borrow for the following values of k: 0.8, 0.9, 1.0, 1.1. Next, continue to assume that Lucy can save at an interest rate of 10 percent, but that she pays an interest rate of 25 percent when she borrows. Draw her budget line. Determine how much she will save or borrow for the same four values of k.
. Suppose she earns $50 this year and $55 next year. Lemonade costs $1 per gallon in both years and the interest rate is 10 percent. Determine how much Lucy will save or borrow for the following values of k: 0.8, 0.9, 1.0, 1.1. Next, continue to assume that Lucy can save at an interest rate of 10 percent, but that she pays an interest rate of 25 percent when she borrows. Draw her budget line. Determine how much she will save or borrow for the same four values of k.
Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
24
The net cash flows from an investment project are $15,000 in the first year, $11,000 in the second year, +$5,000 in the third year, +$1,800 in the fourth year, + $7,000 in the fifth year, +$6,400 in the sixth year, +$4,300 in the seventh year, and + $6,200 in the eighth year. Compute the NPV of this project at the following interest rates: 2 percent, 5 percent, 8 percent.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
25
For each of the following investments, compute the IRR.
a. The net cash flows are $12,000 in the first year and + $15,000 in the second year. (Use the formula for two- period investments given in the text.)b. The net cash flows are $10,000 in the first year, +$5,000 in the second year, and + $6,000 in the third year. (Use the quadratic formula.)c. The net cash flows are the same as in exercise 10.9. (Use either the search method illustrated in worked-out problem 10.4 on page 358, or the automatic search feature of a spreedsheet program.)
a. The net cash flows are $12,000 in the first year and + $15,000 in the second year. (Use the formula for two- period investments given in the text.)b. The net cash flows are $10,000 in the first year, +$5,000 in the second year, and + $6,000 in the third year. (Use the quadratic formula.)c. The net cash flows are the same as in exercise 10.9. (Use either the search method illustrated in worked-out problem 10.4 on page 358, or the automatic search feature of a spreedsheet program.)

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
26
The net cash flows from an investment are X dollars in the first year, positive Y dollars in year T, and zero in all other years. Write a formula for the NPV. Set the NPV equal to zero, and solve for the IRR in terms of X and Y.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
27
Calculate the yield to maturity for each of the following bonds.
a. A 20-year bond with a coupon of $100, principle payment at maturity of $2,000, and a current price of $2,000.
b. A 5-year bond with a coupon of $500, principle payment at maturity of $10,000, and a current price of $8,000.
a. A 20-year bond with a coupon of $100, principle payment at maturity of $2,000, and a current price of $2,000.
b. A 5-year bond with a coupon of $500, principle payment at maturity of $10,000, and a current price of $8,000.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
28
A project that requires an investment of $6,000 in the first year generates a positive net cash flow of $15,000 in the fourth year. It leaves the investor with an obligation to pay $10,000 in the eighth year, and yields no net cash flow in any other year.
a. Find the NPV of this investment at the following interest rates: 0 percent, 5 percent, 10 percent, 15 percent, 20 percent.
b. This investment has two different IRRs. Find them, using the method illustrated in worked-out problem 10.4 (page 358).
c. At what interest rates is this project profitable (Create a graph similar to Figure 10.11 on page 355.) Is it always profitable when one of the IRRs exceeds the interest rate What if both of the IRRs exceed the interest rate
d. Why does this example produce two IRRs How does it differ from the examples presented in this chapter (Hint: Using a spreadsheet, graph the NPV against the interest rate. How does this graph change when you reduce the value of the payment in the eighth year from $10,000 to some smaller number What happens to the IRRs)
a. Find the NPV of this investment at the following interest rates: 0 percent, 5 percent, 10 percent, 15 percent, 20 percent.
b. This investment has two different IRRs. Find them, using the method illustrated in worked-out problem 10.4 (page 358).
c. At what interest rates is this project profitable (Create a graph similar to Figure 10.11 on page 355.) Is it always profitable when one of the IRRs exceeds the interest rate What if both of the IRRs exceed the interest rate
d. Why does this example produce two IRRs How does it differ from the examples presented in this chapter (Hint: Using a spreadsheet, graph the NPV against the interest rate. How does this graph change when you reduce the value of the payment in the eighth year from $10,000 to some smaller number What happens to the IRRs)

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck



