Deck 7: Technology and Production
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/36
Play
Full screen (f)
Deck 7: Technology and Production
1
A firm has monthly production function
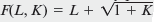 K, where L is worker hours per month and K is square feet of manufacturing space.
K, where L is worker hours per month and K is square feet of manufacturing space.
a. Does the firm's technology satisfy the Productive Inputs Principle
b. What is the firm's MRTS LK at input combination ( L , K ) Does the firm's technology have a declining MRTS
c. Does the firm have increasing, decreasing, or constant returns to scale
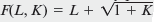 K, where L is worker hours per month and K is square feet of manufacturing space.
K, where L is worker hours per month and K is square feet of manufacturing space.a. Does the firm's technology satisfy the Productive Inputs Principle
b. What is the firm's MRTS LK at input combination ( L , K ) Does the firm's technology have a declining MRTS
c. Does the firm have increasing, decreasing, or constant returns to scale
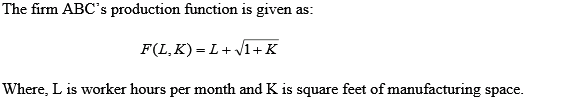
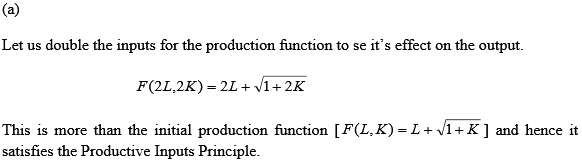
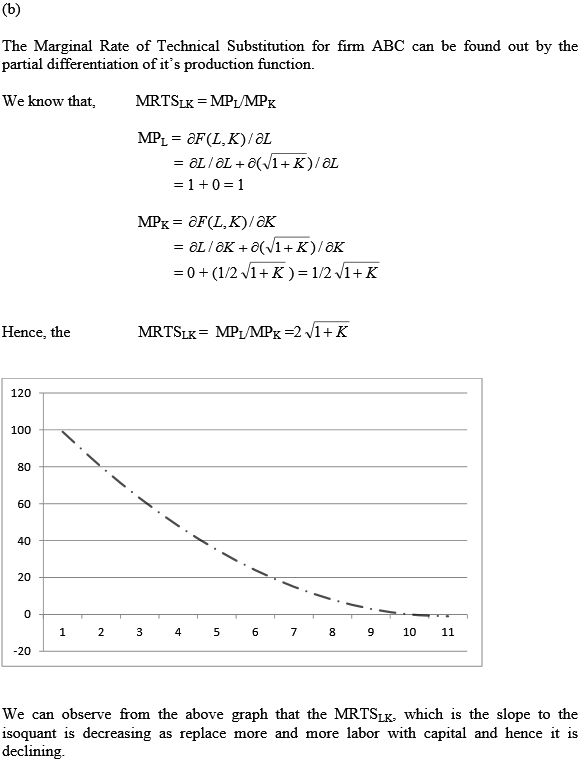
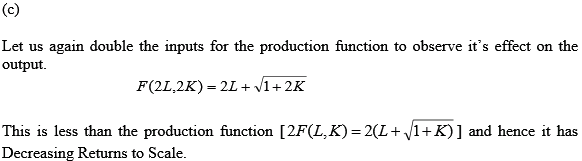
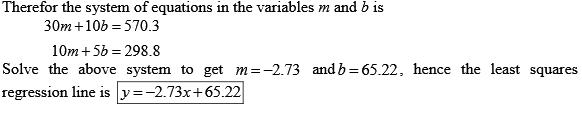
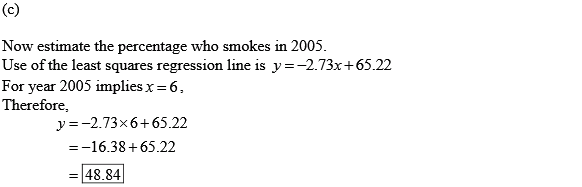















2
"The marginal product of labor can never be negative." Is this statement correct What does it assume
"The Marginal Product of Labor MP L can never be negative", cannot be always correct. This statement assumes that every unit addition of labor would increase the output more than the per unit average output by the already employed labor.
Q = F(L)
AP L = Q/L = F(L) / L
MP L = Q/L
To elucidate the matter more clearly let us form a table with Q, AP L and MP L
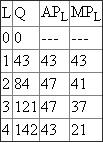 As we can clearly observe from the example above that MP L cannot always be positive. When we increase Labor in a production function, there comes a point where any further increase would decrease the marginal productivity rather than increasing it.
As we can clearly observe from the example above that MP L cannot always be positive. When we increase Labor in a production function, there comes a point where any further increase would decrease the marginal productivity rather than increasing it.
Q = F(L)
AP L = Q/L = F(L) / L
MP L = Q/L
To elucidate the matter more clearly let us form a table with Q, AP L and MP L
 As we can clearly observe from the example above that MP L cannot always be positive. When we increase Labor in a production function, there comes a point where any further increase would decrease the marginal productivity rather than increasing it.
As we can clearly observe from the example above that MP L cannot always be positive. When we increase Labor in a production function, there comes a point where any further increase would decrease the marginal productivity rather than increasing it. 3
Suppose that Noah and Naomi decide instead to produce garden chairs. There are two different methods for producing garden chairs with two assembly workers: A and B. There are three methods with three assembly workers: methods C, D, and E. The weekly output of each method is: 24 from A, 36 from B, 30 from C, 44 from D, and 24 from E. Which of these production methods are efficient
The objective of the following analysis is to determine the production method that is efficient.
A production method is considered the most efficient which produces relatively larger level of output while using the same number of factor inputs.
It shall be noted that comparison for different production methods could be made between those productions methods under which the number of factor-inputs used are the same but producing different levels of output.
Thus, that production method is considered the most efficient which produces relatively larger level of output while using the same number of factor inputs.
The different production methods with level of output and number of worked used is given as follows:
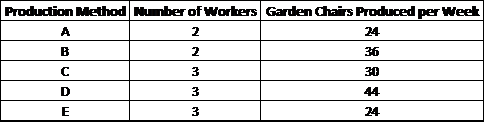 Thus, it can be observed that between production method A and B that uses 2 workers each, the level of output produced by B is relatively higher at 36 Garden chairs per week. Hence, production method B is most efficient.
Thus, it can be observed that between production method A and B that uses 2 workers each, the level of output produced by B is relatively higher at 36 Garden chairs per week. Hence, production method B is most efficient.
On the other hand, between production methods C, D and E that uses 3 workers each, the level of output produced by D is relatively higher at 44 Garden chairs per week.
Hence, the production method D is most efficient.
Thus, the result is:

A production method is considered the most efficient which produces relatively larger level of output while using the same number of factor inputs.
It shall be noted that comparison for different production methods could be made between those productions methods under which the number of factor-inputs used are the same but producing different levels of output.
Thus, that production method is considered the most efficient which produces relatively larger level of output while using the same number of factor inputs.
The different production methods with level of output and number of worked used is given as follows:
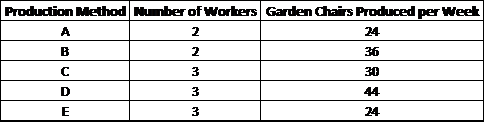 Thus, it can be observed that between production method A and B that uses 2 workers each, the level of output produced by B is relatively higher at 36 Garden chairs per week. Hence, production method B is most efficient.
Thus, it can be observed that between production method A and B that uses 2 workers each, the level of output produced by B is relatively higher at 36 Garden chairs per week. Hence, production method B is most efficient.On the other hand, between production methods C, D and E that uses 3 workers each, the level of output produced by D is relatively higher at 44 Garden chairs per week.
Hence, the production method D is most efficient.
Thus, the result is:

4
The law firm of Dewey, Cheetham, and Howe files claims against unscrupulous car repair shops that take advantage of consumers who know nothing about cars. A lawyer who takes a 20-minute break after every two hours of work can process one claim with six hours of work. If the lawyer takes a 20-minute break after every four hours of work she can process one claim with eight hours of work. If she takes a 20-minute break after every hour of work, she is a claim superwoman and can process one claim with just five hours of work. What is the efficient method of production for Dewey, Cheetham, and Howe Graph its production possibilities set. What is its production function

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
5
There are two production plants for producing widgets out of labor and capital. Plant l's weekly production function is F 1 ( L , K ) = 12 (LK ) 1/4 and plant 2's weekly production function is F 2 ( L , K ) = (LK ) 1/2.
a. Do these technologies satisfy the Productive Inputs Principle
b. Derive the MRTS LK for each of the plants at input combination ( L , K ). Do the plants have declining MRTSs
c. Do the plants have increasing, decreasing, or constant returns to scale
a. Do these technologies satisfy the Productive Inputs Principle
b. Derive the MRTS LK for each of the plants at input combination ( L , K ). Do the plants have declining MRTSs
c. Do the plants have increasing, decreasing, or constant returns to scale

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
6
Is the following statement true or false: "If a firm has two plants for producing output using only labor and plant B is more productive than plant A, a manager who wants to get the most output from a fixed amount of labor should always assign more labor to plant B." If you think this is true, say why. If you think it is false, give an example to prove your point.

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
7
Suppose that Noah and Naomi's production function is Q = F ( L ) = 25 L. How many garden benches can they produce with one worker With two workers With three workers With four workers Graph their production function for up to four workers. Suppose now that the number of workers they hire is finely divisible (that is, they can hire workers for a fraction of a week). Graph their production function in this case. What if instead their production function is Q = F ( L ) =
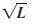
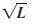

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
8
Pete and Mary run a firm that packs coffee beans. The number of pounds of coffee they pack depends on the number of workers they hire. They are able to hire workers for a fraction of a day. The number of pounds of coffee they pack in a day is given by the production function Q = F ( L ) = 10 L , where L is the number of hours of labor they hire. Graph their production function.

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
9
Suppose that a firm's production function is Q = F ( L ) = L 3 200 L 2 + 10,000 L. At what amount of labor input are the firm's average and marginal product of labor equal Confirm that the average and marginal product curves satisfy the relationship discussed in the text.

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
10
You run a firm that has two plants in the same city. The two plants use the same two inputs to produce your output. A consulting firm just told you that the MRTS is different across your plants. Are you assigning your inputs correctly [ Hint: Look back at Example 4.3's discussion of gains from trade on page 111.]

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
11
Suppose that a firm uses both labor ( L ) and capital ( K) as inputs and has the long-run production function Q = F ( L, K ) = 10
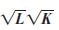 . If its capital is fixed at K = 10 in the short run, what is its short-run production function How much does it produce in the short run (using efficient production methods) if it hires one worker Two workers Three
. If its capital is fixed at K = 10 in the short run, what is its short-run production function How much does it produce in the short run (using efficient production methods) if it hires one worker Two workers Three
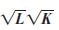 . If its capital is fixed at K = 10 in the short run, what is its short-run production function How much does it produce in the short run (using efficient production methods) if it hires one worker Two workers Three
. If its capital is fixed at K = 10 in the short run, what is its short-run production function How much does it produce in the short run (using efficient production methods) if it hires one worker Two workers Three
Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
12
Suppose that in Table 7.3 (page 218) the number of garden benches produced by 0, 1, 2, 3, and 4 workers is instead 0, 35, 70, 99, and 112. Calculate the marginal and average products of labor that would have resulted. Check that the relation between these average and marginal products satisfies the properties that we discussed in Section 7.2.

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
13
Suppose that John, April, and Tristan have two production plants for producing orange juice. They have a total of 850 crates of oranges. Plant 1's production function is F 1 ( O 2 ) = (1,000 × O 1 ) 0.5( O 1 ) 2 , while plant 2's is F 2 ( O 2 ) = (1,200 × O 2 ) × 0.5( O 2 ) 2 , where O 1 and O 2 are the number of crates of oranges assigned to each of the two plants. What is the best assignment of oranges between the two plants

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
14
You read in the newspaper that XYZ Corporation's average labor productivity fell this past year. Does this necessarily mean that XYZ Corporation is less productive than it was a year ago

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
15
Consider again the production function Q = F ( L ) = 25 L. Suppose that labor is finely divisible. Draw graphs like those in Figures 7.3, 7.5, and 7.6: one pair of graphs to derive the average product curve, one pair of graphs to derive the marginal product curve, and one graph showing both curves. How does the last graph reflect the relationship between a firm's average and marginal product

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
16
Emily draws cartoons that she sells to her classmates. Her average product of labor is five cartoons per hour if she works for one hour, four per hour if she works for two hours, three per hour if she works for three hours, and two-and-a-half per hour if she works for four hours. What is her marginal product of labor at one, two, three, and four hours of work

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
17
Suppose that John, April and Tristan have two production plants for producing orange juice. They have a total of 850 crates of oranges. The production function for the first plant is F 1 ( O 1 ) = 1,000( O 1 ) 0.5( O 1 ) 2 , where O 1 is the number of crates of oranges assigned to plant 1. The production function for the second plant is F 2 ( O 2 ) = 900( O 2 ) (O 2 ) 2 , where O 2 is the number of crates of oranges assigned to plant 2. What is the best assignment of oranges between the two plants

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
18
Suppose that John, April, Tristan have a total of 700 crates of oranges and that the marginal product of oranges in plant 1 is
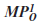 =1,000 - O 1 and in plant 2 is
=1,000 - O 1 and in plant 2 is
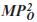 =1,200 - 2 O 2. What is the best assignment of oranges between two plants
=1,200 - 2 O 2. What is the best assignment of oranges between two plants
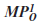 =1,000 - O 1 and in plant 2 is
=1,000 - O 1 and in plant 2 is 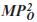 =1,200 - 2 O 2. What is the best assignment of oranges between two plants
=1,200 - 2 O 2. What is the best assignment of oranges between two plants
Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
19
Suppose that a firm uses both labor ( L ) and capital ( K ) as inputs and has the long-run production function Q = F ( L, K ) =
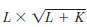 . If its capital is fixed at K = 10 in the short run, what is its short-run production function How much does it produce in the short run (using efficient production methods) if it hires one worker Two workers Three
. If its capital is fixed at K = 10 in the short run, what is its short-run production function How much does it produce in the short run (using efficient production methods) if it hires one worker Two workers Three
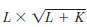 . If its capital is fixed at K = 10 in the short run, what is its short-run production function How much does it produce in the short run (using efficient production methods) if it hires one worker Two workers Three
. If its capital is fixed at K = 10 in the short run, what is its short-run production function How much does it produce in the short run (using efficient production methods) if it hires one worker Two workers Three
Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
20
Beta Inc. has two production plants that use finely divisible labor to produce its output. The production function in plant 1 is F 1 ( L 1 ) = 100 ln( L 1 ), where L 1 is the amount of labor assigned to plant 1 and ln(X ) is the natural logarithm function. The production function in plant 2 is F 2 ( L 1 ) = 50 ln( L 2 ), where L 2 is the amount of labor assigned to plant 2. Beta Inc. wants to find the best assignment of 90 workers between the two plants. What is it

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
21
Suppose that Noah and Naomi's weekly production function is F( L, K ) = 10 L + K, where L is the number of assembly workers (labor) and K is garage space (capital). Derive a formula for their isoquant for producing 10 garden benches per week. What is the general formula for their family of isoquants

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
22
Suppose that a firm's production function is Q = F ( L ) = L 3 -200 L 2 + 10,000 L. Its marginal product of labor is MP L = 3 L 2 - 400 L + 10,000. At what amount of labor input are the firm's average and marginal product of labor equal Confirm that the average and marginal product curves satisfy the relationship discussed in the text.

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
23
Alpha Corporation has two production plants that use labor and capital as inputs. The production function at each plant is F ( L , K ) = L 0.5 K 0.5. Alpha has 50 workers.
a. Initially the two plants each have 100 units of capital. What is the best assignment of workers to the two plants
b. Suppose that Alpha installs an additional 300 units of capital at plant 1. How does the best assignment of workers to the two plants change Why does the increase in capital at plant 1 lead to this change
a. Initially the two plants each have 100 units of capital. What is the best assignment of workers to the two plants
b. Suppose that Alpha installs an additional 300 units of capital at plant 1. How does the best assignment of workers to the two plants change Why does the increase in capital at plant 1 lead to this change

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
24
How does Noah and Naomi's marginal product of labor with three workers change in Table 7.4 on page 227 as the amount of garage space increases from 1,000 to 1,500 square feet (assuming workers must be hired by the week, so that L = 1) To 2,000 What is Noah and Naomi's marginal product of capital with 1,500 square feet of garage space and four workers if garage space can be changed in increments no smaller than 500 square feet (so that K = 500)

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
25
Suppose that John, April, and Tristan have two production plants for producing orange juice. They have a total of 850 crates of oranges and the marginal product of oranges in plant 1 is
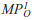 = 1,000 - O 1 and in plant 2 is
= 1,000 - O 1 and in plant 2 is
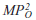 = 1,200 - 2 O 2. What is the best assignment of oranges between the two plants
= 1,200 - 2 O 2. What is the best assignment of oranges between the two plants
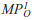 = 1,000 - O 1 and in plant 2 is
= 1,000 - O 1 and in plant 2 is 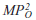 = 1,200 - 2 O 2. What is the best assignment of oranges between the two plants
= 1,200 - 2 O 2. What is the best assignment of oranges between the two plants
Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
26
There are two production plants for producing widgets out of labor and capital. Plant 1's weekly production function is F 1 ( L , K ) = 12( LK ) 1/4 and plant 2's weekly production function is F 2 ( L , K ) = ( LK ) 1/2. There is a total of 100 workers available and 25 units of capital. Suppose that initially plant 1 is using 4 units of capital and 64 units of labor, with plant 2 using the rest.
a. Is there a way to reallocate the inputs to increase the output of both plants
b. What allocation of labor and capital would get the most output from plant 2 without changing plant 1's output level
c. What allocation of labor and capital would maximize the total amount of output that the two plants produce
a. Is there a way to reallocate the inputs to increase the output of both plants
b. What allocation of labor and capital would get the most output from plant 2 without changing plant 1's output level
c. What allocation of labor and capital would maximize the total amount of output that the two plants produce

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
27
Suppose that Robert's production function is instead F ( L, K ) = ( L + K ) 2. Does his production function have constant, increasing, or decreasing returns to scale

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
28
You have an economics test in two weeks. It will cover both micro and macroeconomics. Each part will be worth 50 points. You have a total of 100 hours that you can spend studying for this exam. For the first 25 hours you spend studying microeconomics, you will get 1 point more for each hour of studying. After that, you will get 1 point more for each 3 hours you spend studying, up to the maximum possible of 50 points. Macroeconomics is different. For your first 15 hours of studying you will get 1 point for each 45 minutes you spend studying. After that, you will get 1 point for each 1.5 hours you spend up to the maximum of 50 points. How should you allocate your studying time to get the best grade Why

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
29
A firm has two plants that operate with capital and labor as inputs. The first plant has production function F 1 ( L , K ) = LK The second plant has production function F 2 ( L , K ) = ALK , where A is a technological parameter ( A as strictly positive number: A 0).
a. What are the marginal products of capital and has labor in each of the plants as a function of the amount of labor and capital being used in the plant
b. Suppose the firm has 100 units of capital and hired 50 units of labor. What is the allocation of capital and labor among the two plants that maximizes total output (Your answer should be in terms of A. )
a. What are the marginal products of capital and has labor in each of the plants as a function of the amount of labor and capital being used in the plant
b. Suppose the firm has 100 units of capital and hired 50 units of labor. What is the allocation of capital and labor among the two plants that maximizes total output (Your answer should be in terms of A. )

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
30
Beta Inc. has two production plants that use finely divisible labor to produce its output. The marginal product of labor at plant 1 is
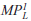 = 100/ L 1 , and the marginal product of labor at plant 2 is
= 100/ L 1 , and the marginal product of labor at plant 2 is
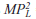 = 50/ L 2. Beta Inc. wants to find the best assignment of 90 workers between the two plants. What is it
= 50/ L 2. Beta Inc. wants to find the best assignment of 90 workers between the two plants. What is it
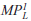 = 100/ L 1 , and the marginal product of labor at plant 2 is
= 100/ L 1 , and the marginal product of labor at plant 2 is 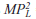 = 50/ L 2. Beta Inc. wants to find the best assignment of 90 workers between the two plants. What is it
= 50/ L 2. Beta Inc. wants to find the best assignment of 90 workers between the two plants. What is it
Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
31
A supermarket is considering adding self-scan checkout machines. Currently the supermarket checks out 200 customers an hour using 10 checkout clerks. Customers differ in their abilities to use these machines. Market research suggests that 20 percent of the store's customers can check themselves out using these machines in three minutes (on average), 30 percent can check themselves out in five minutes, and 50 percent will refuse to use the new machines. One employee can monitor up to four self-scan machines. If there are fewer than four machines, this employee can use her extra time in other productive ways. Draw an isoquant for checking out 200 customers an hour where the variable inputs are checkout clerks and self-scan checkout machines.

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
32
Jason has a boat-building firm. It uses labor L, capital K , and materials M, to build its boats. Its production function is Q = F ( L, K, M ) = AL K M . For what values of , , and does Jason's technology have constant returns to scale Increasing returns to scale Decreasing returns to scale

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
33
Suppose that because of a technological breakthrough a firm that uses labor and capital to produce its output is able to double its production for any given amounts of inputs. What happens to the firm's MRTS at any given input combination

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
34
Suppose that because of a technological breakthrough a firm that uses a single input to produce its output is able to double its production for any given amount of the input. What happens to the firm's average product of labor What about its marginal product of labor

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
35
You are the manager of a new firm that can choose between two technologies for producing output using only labor. Technology A can produce two units of output for each hour of labor input. Technology B can produce three units of output for each hour of labor input up to 100 hours, and units of output for each hour of labor above 100 hours. For what values of is technology B more productive than technology A

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck
36
A firm that uses L, K, and Z as inputs has a technology with production function Q = F ( L,K,Z ) = Z + min { L , K }. Which of the following statements is true
a. The firm's technology satisfies the Productive Inputs Principle and has decreasing returns to scale.
b. The firm's technology satisfies the Productive Inputs Principle and has constant returns to scale.
c. The firm's technology does not satisfy the Productive Inputs Principle but has decreasing returns to scale.
a. The firm's technology satisfies the Productive Inputs Principle and has decreasing returns to scale.
b. The firm's technology satisfies the Productive Inputs Principle and has constant returns to scale.
c. The firm's technology does not satisfy the Productive Inputs Principle but has decreasing returns to scale.

Unlock Deck
Unlock for access to all 36 flashcards in this deck.
Unlock Deck
k this deck



