Deck 10: Special Pricing Practices
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/25
Play
Full screen (f)
Deck 10: Special Pricing Practices
1
There are only two firms in the widget industry. The total demand for widgets is Q = 30 2 P. The two firms have identical cost functions, TC = 3 + 10 Q. The two firms agree to collude and act as though the industry were a monopoly. At what price and quantity will this cartel maximize its profit
There are only two firms in the Widget industry. The total demand for widgets is
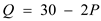 . The two firms have identical cost functions,
. The two firms have identical cost functions,
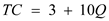 . The two firms agree to collude and act as though the industry were a monopoly.
. The two firms agree to collude and act as though the industry were a monopoly.
The Price and Quantity at which this cartel would maximize its profit can be calculated by inverting the demand function for P:
Given:
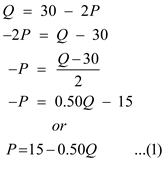 Total Revenue for both firms can be calculated by
Total Revenue for both firms can be calculated by
 Total Cost of both firms
Total Cost of both firms
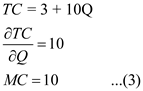 For Maximum Profit MC = MR
For Maximum Profit MC = MR
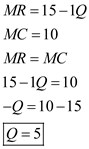 From (1) we can find equilibrium P
From (1) we can find equilibrium P
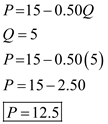 Thus, we can say that Profit Maximizing price is P=12.5 and Profit Maximizing quantity is Q = 5.
Thus, we can say that Profit Maximizing price is P=12.5 and Profit Maximizing quantity is Q = 5.
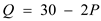 . The two firms have identical cost functions,
. The two firms have identical cost functions, 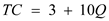 . The two firms agree to collude and act as though the industry were a monopoly.
. The two firms agree to collude and act as though the industry were a monopoly.The Price and Quantity at which this cartel would maximize its profit can be calculated by inverting the demand function for P:
Given:
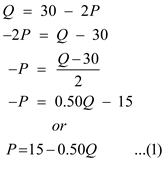 Total Revenue for both firms can be calculated by
Total Revenue for both firms can be calculated by  Total Cost of both firms
Total Cost of both firms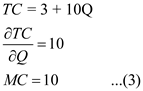 For Maximum Profit MC = MR
For Maximum Profit MC = MR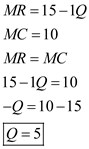 From (1) we can find equilibrium P
From (1) we can find equilibrium P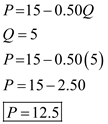 Thus, we can say that Profit Maximizing price is P=12.5 and Profit Maximizing quantity is Q = 5.
Thus, we can say that Profit Maximizing price is P=12.5 and Profit Maximizing quantity is Q = 5. 2
"If a company sets its prices on the basis of a cost-plus calculation, it cannot possibly suffer a loss on its products." True or false Comment.
The given statement state that "If a company sets its price on the basis of a cost-plus calculation, it cannot possibly suffer a loss on its products.",
This statement is false because company profit depends on the market price. If the market price is lower than the company's price then company have to bear the losses.
Thus, it is False , that setting prices on the basic of cost-plus calculation cannot leads to loss.
This statement is false because company profit depends on the market price. If the market price is lower than the company's price then company have to bear the losses.
Thus, it is False , that setting prices on the basic of cost-plus calculation cannot leads to loss.
3
The Bramwell Corporation has estimated its demand function and total cost function to be as follows:
Q = 25 - 0.05 P
TC = 700 + 200 Q
Answer the following questions either by developing demand and cost schedules ( Hint: Use quantities from 1 to 14) or by solving the equations.
a. What will be the price and quantity if Bramwell wants to
1. Maximize profits
2. Maximize revenue
3. Maximize revenue but require the profit to be a minimum of $300
b. Now assume the cost function is TC = 780 + 200 Q, while the demand function remains the same. What will the price and quantity be if Bramwell wants to
1. Maximize profits
2. Maximize revenue
3. Maximize revenue but require the profit to be a minimum of $300
c. Why are the answers the same in a (1) and b (1) but different in a (3) and b (3)
Q = 25 - 0.05 P
TC = 700 + 200 Q
Answer the following questions either by developing demand and cost schedules ( Hint: Use quantities from 1 to 14) or by solving the equations.
a. What will be the price and quantity if Bramwell wants to
1. Maximize profits
2. Maximize revenue
3. Maximize revenue but require the profit to be a minimum of $300
b. Now assume the cost function is TC = 780 + 200 Q, while the demand function remains the same. What will the price and quantity be if Bramwell wants to
1. Maximize profits
2. Maximize revenue
3. Maximize revenue but require the profit to be a minimum of $300
c. Why are the answers the same in a (1) and b (1) but different in a (3) and b (3)
The demand of a good is the quantity necessary of that good by the persons of an economy. The prerequisite turn out to be a demand when individuals are ready and able to pay for the good.
Supply of a good is the amount of a good that the manufacturers are ready to sell in a market.
Equilibrium price is the price at which demand and supply are equal. Similarly, equilibrium quantity is the quantity of a good exchanged in the market after arbitration.
a.
The demand function and the cost function is given as:
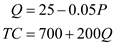 1. The price and quantity if B well maximize profits is calculated as follows:
1. The price and quantity if B well maximize profits is calculated as follows:
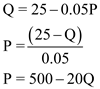 First, calculate total revenue (TR) by multiplying price and quantity and calculate marginal revenue (MR) as follows:
First, calculate total revenue (TR) by multiplying price and quantity and calculate marginal revenue (MR) as follows:
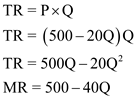 Calculate marginal cost (MC) as follows:
Calculate marginal cost (MC) as follows:
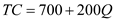
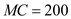 Now, using the profit maximizing condition calculate Q as follows:
Now, using the profit maximizing condition calculate Q as follows:
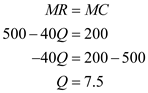 Now substitute the values of Q in the given demand function and solve for P as follows:
Now substitute the values of Q in the given demand function and solve for P as follows:
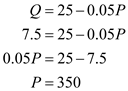 Hence, if B well maximize profits then the price would be
Hence, if B well maximize profits then the price would be
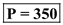 and the quantity would be
and the quantity would be
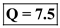 .
.
2. In order to find price and quantity when revenue is maximized, first find the average revenue (AR) by dividing the TR by Q as follows:
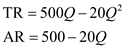 Now, calculate average cost (AC) by dividing TC by Q as follows:
Now, calculate average cost (AC) by dividing TC by Q as follows:
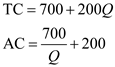 Now, using the revenue maximizing condition, the price and quantity is calculated as follows:
Now, using the revenue maximizing condition, the price and quantity is calculated as follows:

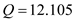 Substitute the value of Q in the demand function and solve for P as follows:
Substitute the value of Q in the demand function and solve for P as follows:
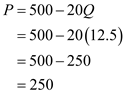 Hence, the revenue maximizing price is
Hence, the revenue maximizing price is
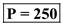 and quantity is
and quantity is
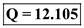 .
.
3. In this case, minimum profit is required to be $300, before maximizing revenue. So when P = 350 and Q = 7.5 (calculated in part 1.) the profit will be calculated as:

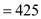 Since, profit estimated here is $425, thus satisfies the minimum profit condition. Now consider the revenue maximization (calculated in part 2.). Hence,
Since, profit estimated here is $425, thus satisfies the minimum profit condition. Now consider the revenue maximization (calculated in part 2.). Hence,
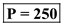 and
and
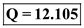 b.
b.
The demand function and the cost function is given as:
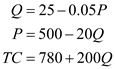 1. The profit maximizing price and quantity is calculated as follows:
1. The profit maximizing price and quantity is calculated as follows:
Since, the demand function is same as given in part (a), therefore the TR and MR will be same. Now calculate MC as follows:
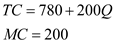 It shows that MC is also same as calculated in part (a). Thus, the price and quantity will be same as calculated in part (a). Hence, the profit maximizing price is
It shows that MC is also same as calculated in part (a). Thus, the price and quantity will be same as calculated in part (a). Hence, the profit maximizing price is
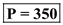 and quantity is
and quantity is
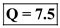 .
.
2. In order to find price and quantity when revenue is maximized, first find the AR and AC. Since, TR is same as calculated in part (a), so AR will also be same. Now calculate average cost (AC) by dividing TC by Q as follows:
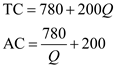 Now using the revenue maximizing condition, the price and quantity is calculated as follows:
Now using the revenue maximizing condition, the price and quantity is calculated as follows:

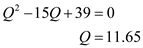 Substitute the value of Q in the demand function and solve for P as follows:
Substitute the value of Q in the demand function and solve for P as follows:
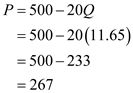 Hence, the revenue maximizing price is
Hence, the revenue maximizing price is
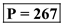 and quantity is
and quantity is
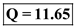 .
.
3. In this case, minimum profit is required to be $300, before maximizing revenue. So when P = 350 and Q = 7.5 (calculated in part a (1)) the profit will be calculated as:

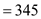 Since, profit estimated here is $345, thus satisfies the minimum profit condition. Now consider the revenue maximization (calculated in b (2)). Hence, the
Since, profit estimated here is $345, thus satisfies the minimum profit condition. Now consider the revenue maximization (calculated in b (2)). Hence, the
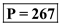 and
and
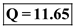 c.
c.
The answer is same in a(1) and b(1) because the MR and MC are same and thus, the profit maximizing price and quantity are same. On the other hand, answer is not same in a(3) and b(3) because AC is different in both cases.
Supply of a good is the amount of a good that the manufacturers are ready to sell in a market.
Equilibrium price is the price at which demand and supply are equal. Similarly, equilibrium quantity is the quantity of a good exchanged in the market after arbitration.
a.
The demand function and the cost function is given as:
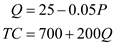 1. The price and quantity if B well maximize profits is calculated as follows:
1. The price and quantity if B well maximize profits is calculated as follows: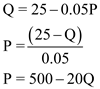 First, calculate total revenue (TR) by multiplying price and quantity and calculate marginal revenue (MR) as follows:
First, calculate total revenue (TR) by multiplying price and quantity and calculate marginal revenue (MR) as follows: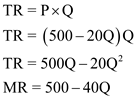 Calculate marginal cost (MC) as follows:
Calculate marginal cost (MC) as follows: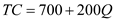
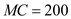 Now, using the profit maximizing condition calculate Q as follows:
Now, using the profit maximizing condition calculate Q as follows: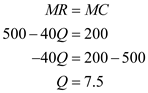 Now substitute the values of Q in the given demand function and solve for P as follows:
Now substitute the values of Q in the given demand function and solve for P as follows: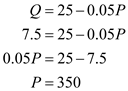 Hence, if B well maximize profits then the price would be
Hence, if B well maximize profits then the price would be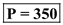 and the quantity would be
and the quantity would be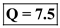 .
. 2. In order to find price and quantity when revenue is maximized, first find the average revenue (AR) by dividing the TR by Q as follows:
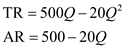 Now, calculate average cost (AC) by dividing TC by Q as follows:
Now, calculate average cost (AC) by dividing TC by Q as follows: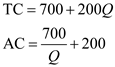 Now, using the revenue maximizing condition, the price and quantity is calculated as follows:
Now, using the revenue maximizing condition, the price and quantity is calculated as follows:
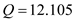 Substitute the value of Q in the demand function and solve for P as follows:
Substitute the value of Q in the demand function and solve for P as follows: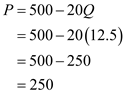 Hence, the revenue maximizing price is
Hence, the revenue maximizing price is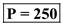 and quantity is
and quantity is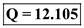 .
.3. In this case, minimum profit is required to be $300, before maximizing revenue. So when P = 350 and Q = 7.5 (calculated in part 1.) the profit will be calculated as:

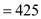 Since, profit estimated here is $425, thus satisfies the minimum profit condition. Now consider the revenue maximization (calculated in part 2.). Hence,
Since, profit estimated here is $425, thus satisfies the minimum profit condition. Now consider the revenue maximization (calculated in part 2.). Hence,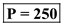 and
and 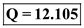 b.
b.The demand function and the cost function is given as:
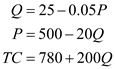 1. The profit maximizing price and quantity is calculated as follows:
1. The profit maximizing price and quantity is calculated as follows:Since, the demand function is same as given in part (a), therefore the TR and MR will be same. Now calculate MC as follows:
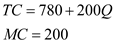 It shows that MC is also same as calculated in part (a). Thus, the price and quantity will be same as calculated in part (a). Hence, the profit maximizing price is
It shows that MC is also same as calculated in part (a). Thus, the price and quantity will be same as calculated in part (a). Hence, the profit maximizing price is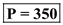 and quantity is
and quantity is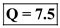 .
.2. In order to find price and quantity when revenue is maximized, first find the AR and AC. Since, TR is same as calculated in part (a), so AR will also be same. Now calculate average cost (AC) by dividing TC by Q as follows:
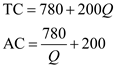 Now using the revenue maximizing condition, the price and quantity is calculated as follows:
Now using the revenue maximizing condition, the price and quantity is calculated as follows:
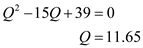 Substitute the value of Q in the demand function and solve for P as follows:
Substitute the value of Q in the demand function and solve for P as follows: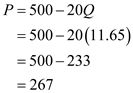 Hence, the revenue maximizing price is
Hence, the revenue maximizing price is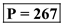 and quantity is
and quantity is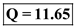 .
.3. In this case, minimum profit is required to be $300, before maximizing revenue. So when P = 350 and Q = 7.5 (calculated in part a (1)) the profit will be calculated as:

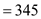 Since, profit estimated here is $345, thus satisfies the minimum profit condition. Now consider the revenue maximization (calculated in b (2)). Hence, the
Since, profit estimated here is $345, thus satisfies the minimum profit condition. Now consider the revenue maximization (calculated in b (2)). Hence, the 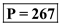 and
and 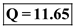 c.
c.The answer is same in a(1) and b(1) because the MR and MC are same and thus, the profit maximizing price and quantity are same. On the other hand, answer is not same in a(3) and b(3) because AC is different in both cases.
4
The Great Southern Paper Company has the following marginal cost schedule for producing pulp:
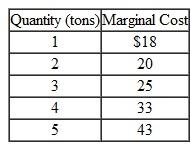
Pulp can be bought in the open market for $25 per ton. The marginal cost of converting pulp into paper is MC = 5 + 5 Q, and the demand for paper is P = 135 15 Q. Calculate the marginal cost of paper if the company produces its own pulp. What is the profit-maximizing quantity Should the company purchase pulp from the outside or produce it in-house

Pulp can be bought in the open market for $25 per ton. The marginal cost of converting pulp into paper is MC = 5 + 5 Q, and the demand for paper is P = 135 15 Q. Calculate the marginal cost of paper if the company produces its own pulp. What is the profit-maximizing quantity Should the company purchase pulp from the outside or produce it in-house

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
5
Many years ago, a neighborhood lunch counter charged 15 cents for a cup of coffee and 15 cents for a buttered hard roll. One day, a customer ordered the two items and was told that the total price was 35 cents. When the customer asked which of the two items had been raised by 5 cents, the owner's condescending reply was, "Which do you think" In your opinion, which of the two items was affected and why

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
6
Each pair of Fancy Shoes costs Bradbury Footwear Stores $30. Bradbury's economist has estimated the point elasticity to be 1.8. What price should Bradbury charge if it wants to maximize profits

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
7
Differentiate barometric price leadership and dominant price leadership

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
8
An airplane manufacturer has annual fixed costs of $50 million. Its variable costs are expected to be $2 million per plane. If the manufacturer wants to earn a 10 percent rate of return on its investment of $400 million and expects to produce 100 aircraft this year, what will its markup on total cost have to be If it expects to produce 150 aircraft, what will its markup have to be

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
9
Is there a similarity between cartel pricing and monopoly pricing

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
10
What conditions are favorable to the formation and maintenance of a cartel

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
11
The Prestige Office Equipment Company produces and sells different types of office furniture. One of the important items it sells is a high-quality desk. During the past year, Prestige sold 5,000 of these at a price of $500 each. The contribution profit for this line of furniture last year was $700,000.
A consultant suggests that Prestige decrease the price of each desk by $30. In his opinion, another 500 desks could then be sold, and the total profit would be maintained. A trade publication that employs an economist has estimated price elasticity of office furniture (including desks) to be about 1.8.
Assume the variable unit cost per desk in the coming year will remain the same. Evaluate the consultant's proposal. Be sure to include in your answer the price elasticity assumed by the consultant, as well as the published elasticity estimate.
A consultant suggests that Prestige decrease the price of each desk by $30. In his opinion, another 500 desks could then be sold, and the total profit would be maintained. A trade publication that employs an economist has estimated price elasticity of office furniture (including desks) to be about 1.8.
Assume the variable unit cost per desk in the coming year will remain the same. Evaluate the consultant's proposal. Be sure to include in your answer the price elasticity assumed by the consultant, as well as the published elasticity estimate.

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
12
Can government be a potent force in the establishment and maintenance of monopolistic conditions Name and describe such occurrences.

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
13
Describe the properties of the Baumol revenue maximization model. Do you consider this to be a good alternative to the profit maximization model

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
14
The Prime Company produces two products, X and Y. They are produced jointly so for each X manufactured a unit of Y is also produced. The joint cost function is
TC = 50 + 2 Q +.5 Q 2
Q represents the number of joint units produced. The demand equations for the two products are the following:
Q x = 100 - P x
Q y = 60 - 2 P y
a. How many units should the company produce per period
b. What price should it charge for each of the joint products
c. What will be the company's profit per period
Assume the company is a profit maximizer.
TC = 50 + 2 Q +.5 Q 2
Q represents the number of joint units produced. The demand equations for the two products are the following:
Q x = 100 - P x
Q y = 60 - 2 P y
a. How many units should the company produce per period
b. What price should it charge for each of the joint products
c. What will be the company's profit per period
Assume the company is a profit maximizer.

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
15
Telephone companies charge different rates for calls during the day, in the evening, and at night or weekends. Do you consider this to be price discrimination

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
16
George's Pants Store sells a particular brand of slacks. It recently decreased the price of a popular brand by 5 percent. This decrease was followed by a 20 percent increase in sales. The marginal cost of producing these slacks is $100.
a. What is the point price elasticity of demand for these slacks
b. What would be the optimal markup and price of these items
a. What is the point price elasticity of demand for these slacks
b. What would be the optimal markup and price of these items

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
17
Is cost-plus pricing necessarily inconsistent with marginal pricing

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
18
A firm makes two products, x and y. Inverse demand for each shows that pricing in one market depends on sales in the other according to the equations:
P x = 1000 - 20 x + 3 y and P y = 500 - 5 y + x.
The firm faces joint fixed cost of $12,000 and constant marginal cost of production in each product segment, MC x = $200, and MC y = $100.
a. What bundle of products ( x *, y *) should the firm produce
b. What prices will the firm be able to charge for each product given production at ( x *, y *)
c. What profits result in this instance
d. At ( x *, y *), what are the values of
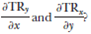 Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
e. Check your work by determining the profits that result if x and y are one unit more or less than optimal and fill in the following table.

Hints: This problem may be solved using the structure described in footnote 46 in this chapter. All answers are whole numbers. Part e is easily accomplished using Excel by setting up a test area where you can try different values of x and y and determine profit.
P x = 1000 - 20 x + 3 y and P y = 500 - 5 y + x.
The firm faces joint fixed cost of $12,000 and constant marginal cost of production in each product segment, MC x = $200, and MC y = $100.
a. What bundle of products ( x *, y *) should the firm produce
b. What prices will the firm be able to charge for each product given production at ( x *, y *)
c. What profits result in this instance
d. At ( x *, y *), what are the values of
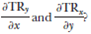 Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.e. Check your work by determining the profits that result if x and y are one unit more or less than optimal and fill in the following table.

Hints: This problem may be solved using the structure described in footnote 46 in this chapter. All answers are whole numbers. Part e is easily accomplished using Excel by setting up a test area where you can try different values of x and y and determine profit.

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
19
Airline ticket prices may differ with respect to when the ticket is bought, how long a passenger remains on the trip (e.g., over a weekend) and other variables. Are these differences a case of price discrimination

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
20
Redo Problem 12 given the following inverse demand functions:
P x = 1000 - 20 x - 3 y and P y = 500 - 5 y - 2 x
Hint: All answers to this problem are whole numbers, just like the answers to Problem.
A firm makes two products, x and y. Inverse demand for each shows that pricing in one market depends on sales in the other according to the equations:
P x = 1000 - 20 x + 3 y and P y = 500 - 5 y + x.
The firm faces joint fixed cost of $12,000 and constant marginal cost of production in each product segment, MC x = $200, and MC y = $100.
a. What bundle of products ( x *, y *) should the firm produce
b. What prices will the firm be able to charge for each product given production at ( x *, y *)
c. What profits result in this instance
d. At ( x *, y *), what are the values of
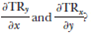 Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
e. Check your work by determining the profits that result if x and y are one unit more or less than optimal and fill in the following table.

Hints: This problem may be solved using the structure described in footnote 46 in this chapter. All answers are whole numbers. Part e is easily accomplished using Excel by setting up a test area where you can try different values of x and y and determine profit.
P x = 1000 - 20 x - 3 y and P y = 500 - 5 y - 2 x
Hint: All answers to this problem are whole numbers, just like the answers to Problem.
A firm makes two products, x and y. Inverse demand for each shows that pricing in one market depends on sales in the other according to the equations:
P x = 1000 - 20 x + 3 y and P y = 500 - 5 y + x.
The firm faces joint fixed cost of $12,000 and constant marginal cost of production in each product segment, MC x = $200, and MC y = $100.
a. What bundle of products ( x *, y *) should the firm produce
b. What prices will the firm be able to charge for each product given production at ( x *, y *)
c. What profits result in this instance
d. At ( x *, y *), what are the values of
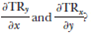 Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.e. Check your work by determining the profits that result if x and y are one unit more or less than optimal and fill in the following table.

Hints: This problem may be solved using the structure described in footnote 46 in this chapter. All answers are whole numbers. Part e is easily accomplished using Excel by setting up a test area where you can try different values of x and y and determine profit.

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
21
Does cost-plus pricing necessarily ignore the demand curve

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
22
Redo Problem given the following inverse demand functions:
P x = 1000 - 20 x and P y = 500 - 5 y
Hint: All answers to this problem are whole numbers, just like the answers to Problems
Problem
A firm makes two products, x and y. Inverse demand for each shows that pricing in one market depends on sales in the other according to the equations:
P x = 1000 - 20 x + 3 y and P y = 500 - 5 y + x.
The firm faces joint fixed cost of $12,000 and constant marginal cost of production in each product segment, MC x = $200, and MC y = $100.
a. What bundle of products ( x *, y *) should the firm produce
b. What prices will the firm be able to charge for each product given production at ( x *, y *)
c. What profits result in this instance
d. At ( x *, y *), what are the values of
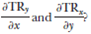 Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
e. Check your work by determining the profits that result if x and y are one unit more or less than optimal and fill in the following table.

Hints: This problem may be solved using the structure described in footnote 46 in this chapter. All answers are whole numbers. Part e is easily accomplished using Excel by setting up a test area where you can try different values of x and y and determine profit.
Problem
Redo Problem 12 given the following inverse demand functions:
P x = 1000 - 20 x - 3 y and P y = 500 - 5 y - 2 x
P x = 1000 - 20 x and P y = 500 - 5 y
Hint: All answers to this problem are whole numbers, just like the answers to Problems
Problem
A firm makes two products, x and y. Inverse demand for each shows that pricing in one market depends on sales in the other according to the equations:
P x = 1000 - 20 x + 3 y and P y = 500 - 5 y + x.
The firm faces joint fixed cost of $12,000 and constant marginal cost of production in each product segment, MC x = $200, and MC y = $100.
a. What bundle of products ( x *, y *) should the firm produce
b. What prices will the firm be able to charge for each product given production at ( x *, y *)
c. What profits result in this instance
d. At ( x *, y *), what are the values of
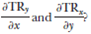 Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.e. Check your work by determining the profits that result if x and y are one unit more or less than optimal and fill in the following table.

Hints: This problem may be solved using the structure described in footnote 46 in this chapter. All answers are whole numbers. Part e is easily accomplished using Excel by setting up a test area where you can try different values of x and y and determine profit.
Problem
Redo Problem 12 given the following inverse demand functions:
P x = 1000 - 20 x - 3 y and P y = 500 - 5 y - 2 x

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
23
Define and describe (giving examples):
a. Transfer pricing
b. Psychological pricing
c. Price skimming
d. Penetration pricing.
a. Transfer pricing
b. Psychological pricing
c. Price skimming
d. Penetration pricing.

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
24
This problem requires completion of Problems 12-14. Can you explain what happened to the optimal x and y in the three problems It may be easiest to explain what happened in moving from the model with negative cross-good coefficients in Problem 12, to zero in Problem 14, and to positive in Problem 13.
A firm makes two products, x and y. Inverse demand for each shows that pricing in one market depends on sales in the other according to the equations:
P x = 1000 - 20 x + 3 y and P y = 500 - 5 y + x.
The firm faces joint fixed cost of $12,000 and constant marginal cost of production in each product segment, MC x = $200, and MC y = $100
.
a. What bundle of products ( x *, y *) should the firm produce
b. What prices will the firm be able to charge for each product given production at ( x *, y *)
c. What profits result in this instance
d. At ( x *, y *), what are the values of
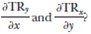 Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
e. Check your work by determining the profits that result if x and y are one unit more or less than optimal and fill in the following table.

Hints: This problem may be solved using the structure described in footnote 46 in this chapter. All answers are whole numbers. Part e is easily accomplished using Excel by setting up a test area where you can try different values of x and y and determine profit.
Redo Problem 12 given the following inverse demand functions:
P x = 1000 - 20 x - 3 y and P y = 500 - 5 y - 2 x
Redo Problem 12 given the following inverse demand functions:
P x = 1000 - 20 x and P y = 500 - 5 y
A firm makes two products, x and y. Inverse demand for each shows that pricing in one market depends on sales in the other according to the equations:
P x = 1000 - 20 x + 3 y and P y = 500 - 5 y + x.
The firm faces joint fixed cost of $12,000 and constant marginal cost of production in each product segment, MC x = $200, and MC y = $100
.
a. What bundle of products ( x *, y *) should the firm produce
b. What prices will the firm be able to charge for each product given production at ( x *, y *)
c. What profits result in this instance
d. At ( x *, y *), what are the values of
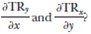 Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.
Provide a short (one- or two-sentence) explanation for each value. That is, explain to your less mathematically sophisticated boss the economic significance of each value.e. Check your work by determining the profits that result if x and y are one unit more or less than optimal and fill in the following table.

Hints: This problem may be solved using the structure described in footnote 46 in this chapter. All answers are whole numbers. Part e is easily accomplished using Excel by setting up a test area where you can try different values of x and y and determine profit.
Redo Problem 12 given the following inverse demand functions:
P x = 1000 - 20 x - 3 y and P y = 500 - 5 y - 2 x
Redo Problem 12 given the following inverse demand functions:
P x = 1000 - 20 x and P y = 500 - 5 y

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
25
Under what circumstances would a discriminating monopolist produce a more socially optimal quantity than a nondiscriminating monopolist Is there any situation under which a discriminating monopolist could produce the quantity that would be produced under competition

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck



