Deck 15: Revenue Management With Capacity Controls
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/10
Play
Full screen (f)
Deck 15: Revenue Management With Capacity Controls
1
(The Inn at Penn) The Inn at Penn hotel has 150 rooms with standard queen-size beds and two rates: a full price of $200 and a discount price of $120. To receive the discount price, a customer must purchase the room at least two weeks in advance (this helps to distinguish between leisure travelers, who tend to book early, and business travelers, who value the flexibility of booking late). For a particular Tuesday night, the hotel estimates that the demand from leisure travelers could fill the whole hotel while the demand from business travelers is distributed normally with a mean of 70 rooms and a standard deviation of 29.
a. Suppose 50 rooms are protected for full-price rooms. What is the booking limit for the discount rooms
b. Find the optimal protection level for full-price rooms (the number of rooms to be protected from sale at a discount price).
c. The Sheraton declared a fare war by slashing business travelers' prices down to $150. The Inn at Penn had to match that fare to keep demand at the same level. Does the optimal protection level increase, decrease, or remain the same Explain your answer.
d. What number of rooms (on average) remain unfilled if we establish a protection level of 61 for the full-priced rooms
e. If The Inn were able to ensure that every full-price customer would receive a room, what would The Inn's expected revenue be
f. If The Inn did not choose to protect any rooms for the full price and leisure travelers book before business travelers, then what would The Inn's expected revenue be
g. Taking the assumptions in part f and assuming now that The Inn protects 50 rooms for the full price, what is The Inn's expected revenue
a. Suppose 50 rooms are protected for full-price rooms. What is the booking limit for the discount rooms
b. Find the optimal protection level for full-price rooms (the number of rooms to be protected from sale at a discount price).
c. The Sheraton declared a fare war by slashing business travelers' prices down to $150. The Inn at Penn had to match that fare to keep demand at the same level. Does the optimal protection level increase, decrease, or remain the same Explain your answer.
d. What number of rooms (on average) remain unfilled if we establish a protection level of 61 for the full-priced rooms
e. If The Inn were able to ensure that every full-price customer would receive a room, what would The Inn's expected revenue be
f. If The Inn did not choose to protect any rooms for the full price and leisure travelers book before business travelers, then what would The Inn's expected revenue be
g. Taking the assumptions in part f and assuming now that The Inn protects 50 rooms for the full price, what is The Inn's expected revenue
Revenue management is concerned with maximizing the revenue by making optimal choice of capacity and pricing strategy given the supply or demand is fairly constant. In case of protection level/ booking limit is a capacity control tool. In this case, generally, two types of reservations are used to cater two types of customer arrivals. The arrival occurs in a staggered manner i.e. the low fare or discounted price offers are given the first priority is arrival. The concept from a newsvendor model from single period inventory model is borrowed in computing the optimal choice of capacity reserved for the full-priced offers.
a.
The available total capacity is 150 rooms.
Since 50 rooms are reserved for full-price rooms, the available capacity for the discounted rooms
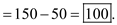 b.
b.
Compute the optimal reserved capacity for the full-price rooms as follows:
Full price,
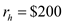 Half price,
Half price,
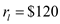
 For optimal reserved capacity of high-fare rooms, the probability of high-fare demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal reserved capacity of high-fare rooms, the probability of high-fare demand being less than or equal to reserved quantity equals the critical ratio. In other words,
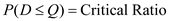 Or,
Or,
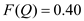 Compute the value of the standard normal variable z using the following Excel function:
Compute the value of the standard normal variable z using the following Excel function:
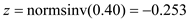 With the given full-price demand distribution, compute the value of Q using the following formula:
With the given full-price demand distribution, compute the value of Q using the following formula:
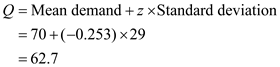 Rounding off the value of Q , the optimal level of full-priced rooms is
Rounding off the value of Q , the optimal level of full-priced rooms is
 c.
c.
After responding to the competitor's move the prices are as following:
Full price,
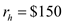 Half price,
Half price,
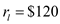
 Since the critical ratio reduces, the protection level for the full-price will also reduce.
Since the critical ratio reduces, the protection level for the full-price will also reduce.
d.
Given the protection level, compute the left-over inventory level as follows:
Protection level, Q = 61
 The corresponding loss function,
The corresponding loss function,
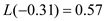 Expected lost sales,
Expected lost sales,
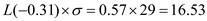 So,
So,
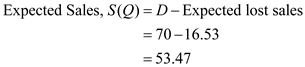
 The expected left-over inventory is
The expected left-over inventory is
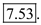 e.
e.
Note that on an average, 70 full-price rooms will be booked. Compute the expected revenue using the following equation:
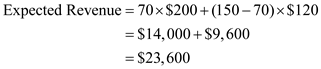 So, the expected revenue will be
So, the expected revenue will be
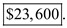 f.
f.
If there is no protection for full-price rooms, then all rooms will be sold as discounted-priced.
So, the expected revenue
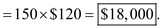 g.
g.
Given the protection level, compute the expected revenue as follows:
Protection level, Q = 50
 The corresponding loss function from the loss function table,
The corresponding loss function from the loss function table,
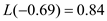 Expected lost sales,
Expected lost sales,
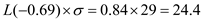 So,
So,
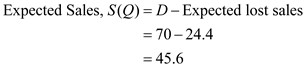
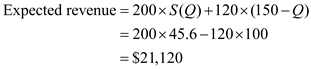 So, the expected revenue is
So, the expected revenue is
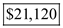 .
.
a.
The available total capacity is 150 rooms.
Since 50 rooms are reserved for full-price rooms, the available capacity for the discounted rooms
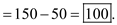 b.
b.Compute the optimal reserved capacity for the full-price rooms as follows:
Full price,
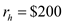 Half price,
Half price, 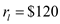
 For optimal reserved capacity of high-fare rooms, the probability of high-fare demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal reserved capacity of high-fare rooms, the probability of high-fare demand being less than or equal to reserved quantity equals the critical ratio. In other words,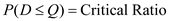 Or,
Or, 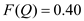 Compute the value of the standard normal variable z using the following Excel function:
Compute the value of the standard normal variable z using the following Excel function: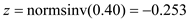 With the given full-price demand distribution, compute the value of Q using the following formula:
With the given full-price demand distribution, compute the value of Q using the following formula: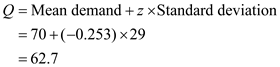 Rounding off the value of Q , the optimal level of full-priced rooms is
Rounding off the value of Q , the optimal level of full-priced rooms is  c.
c.After responding to the competitor's move the prices are as following:
Full price,
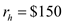 Half price,
Half price, 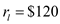
 Since the critical ratio reduces, the protection level for the full-price will also reduce.
Since the critical ratio reduces, the protection level for the full-price will also reduce.d.
Given the protection level, compute the left-over inventory level as follows:
Protection level, Q = 61
 The corresponding loss function,
The corresponding loss function, 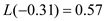 Expected lost sales,
Expected lost sales, 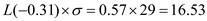 So,
So,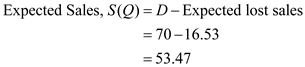
 The expected left-over inventory is
The expected left-over inventory is 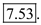 e.
e.Note that on an average, 70 full-price rooms will be booked. Compute the expected revenue using the following equation:
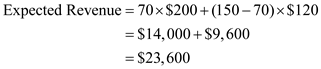 So, the expected revenue will be
So, the expected revenue will be 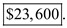 f.
f.If there is no protection for full-price rooms, then all rooms will be sold as discounted-priced.
So, the expected revenue
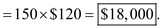 g.
g.Given the protection level, compute the expected revenue as follows:
Protection level, Q = 50
 The corresponding loss function from the loss function table,
The corresponding loss function from the loss function table, 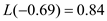 Expected lost sales,
Expected lost sales, 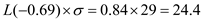 So,
So,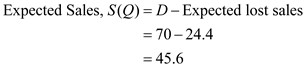
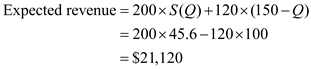 So, the expected revenue is
So, the expected revenue is .
. 2
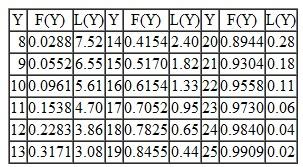
(Overbooking The Inn at Penn) Due to customer no-shows, The Inn at Penn hotel is considering implementing overbooking. Recall from Q16.1 that The Inn at Penn has 150 rooms, the full fare is $200, and the discount fare is $120. The forecast of no-shows is Poisson with a mean of 15.5. The distribution and loss functions of that distribution are as follows:
 The Inn is sensitive about the quality of service it provides alumni, so it estimates the cost of failing to honor a reservation is $325 in lost goodwill and explicit expenses.
The Inn is sensitive about the quality of service it provides alumni, so it estimates the cost of failing to honor a reservation is $325 in lost goodwill and explicit expenses.
a. What is the optimal overbooking limit, that is, the maximum reservations above the available 150 rooms that The Inn should accept
b. If The Inn accepts 160 reservations, what is the probability The Inn will not be able to honor a reservation
c. If The Inn accepts 165 reservations, what is the probability The Inn will be fully occupied
d. If The Inn accepts 170 reservations, what is the expected total cost incurred due to bumped customers
 The Inn is sensitive about the quality of service it provides alumni, so it estimates the cost of failing to honor a reservation is $325 in lost goodwill and explicit expenses.
The Inn is sensitive about the quality of service it provides alumni, so it estimates the cost of failing to honor a reservation is $325 in lost goodwill and explicit expenses.a. What is the optimal overbooking limit, that is, the maximum reservations above the available 150 rooms that The Inn should accept
b. If The Inn accepts 160 reservations, what is the probability The Inn will not be able to honor a reservation
c. If The Inn accepts 165 reservations, what is the probability The Inn will be fully occupied
d. If The Inn accepts 170 reservations, what is the expected total cost incurred due to bumped customers
Revenue management is concerned with maximizing the revenue by making optimal choice of capacity and pricing strategy given the supply or demand is fairly constant. In case of overbooking strategy, the overbooking is done for regular-price reservations. Overbooking is deployed only when the customer reservation is not deterministic in nature and as a result, a portion of the customer do not show up for their reserved seats.
a.
Compute the optimum overbooking limit using the following formula:
 Where,
Where,
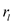 is the price of the regular-fare rooms.
is the price of the regular-fare rooms.
For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,
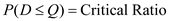 Or,
Or,
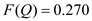 From the given Poisson table, F (13) = 0.3171 0.270
From the given Poisson table, F (13) = 0.3171 0.270
So, the optimal overbooking limit is
 b.
b.
The booked number of rooms is 160. So, if 9 or more no-shows happens, the hotel will not be able to completely accommodate the demand.
From the Poisson table, F (9) = 0.0552.
So, there is a
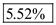 chance that the demand cannot be fulfilled.
chance that the demand cannot be fulfilled.
c.
The booked number of rooms is 165. So, if 15 or fewer no-shows happen, the hotel will be fully occupied and not able to completely accommodate the demand.
From the Poisson table, F (15) = 0.5170.
So, the probability that the Inn is fully occupied is
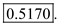 d.
d.
The overbooking limit is
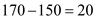 .
.
Corresponding to this value, the loss function is
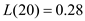 So,
So,
 So, the expected cost of bumping =
So, the expected cost of bumping =
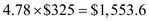 Therefore, the expected cost of bumping is
Therefore, the expected cost of bumping is
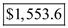 .
.
a.
Compute the optimum overbooking limit using the following formula:
 Where,
Where, 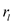 is the price of the regular-fare rooms.
is the price of the regular-fare rooms.For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,
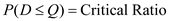 Or,
Or, 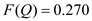 From the given Poisson table, F (13) = 0.3171 0.270
From the given Poisson table, F (13) = 0.3171 0.270So, the optimal overbooking limit is
 b.
b.The booked number of rooms is 160. So, if 9 or more no-shows happens, the hotel will not be able to completely accommodate the demand.
From the Poisson table, F (9) = 0.0552.
So, there is a
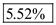 chance that the demand cannot be fulfilled.
chance that the demand cannot be fulfilled.c.
The booked number of rooms is 165. So, if 15 or fewer no-shows happen, the hotel will be fully occupied and not able to completely accommodate the demand.
From the Poisson table, F (15) = 0.5170.
So, the probability that the Inn is fully occupied is
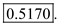 d.
d.The overbooking limit is
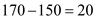 .
.Corresponding to this value, the loss function is
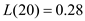 So,
So, So, the expected cost of bumping =
So, the expected cost of bumping = 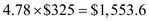 Therefore, the expected cost of bumping is
Therefore, the expected cost of bumping is 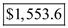 .
. 3
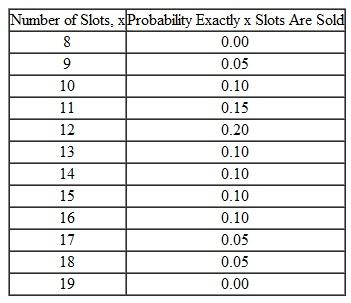
(WAMB) WAMB is a television station that has 25 thirty-second advertising slots during each evening. It is early January and the station is selling advertising for Sunday, March 24. They could sell all of the slots right now for $4,000 each, but, because on this particular Sunday the station is televising the Oscar ceremonies, there will be an opportunity to sell slots during the week right before March 24 for a price of $10,000. For now, assume that a slot not sold in advance and not sold during the last week is worthless to WAMB. To help make this decision, the salesforce has created the following probability distribution for last-minute sales:
 a. How many slots should WAMB sell in advance
a. How many slots should WAMB sell in advance
b. In practice, there are companies willing to place standby advertising messages: if there is an empty slot available (i.e., this slot was not sold either in advance or during the last week), the standby message is placed into this slot. Since there is no guarantee that such a slot will be available, standby messages can be placed at a much lower cost. Now suppose that if a slot is not sold in advance and not sold during the last week, it will be used for a standby promotional message that costs advertisers $2,500. Now how many slots should WAMB sell in advance
c. Suppose WAMB chooses a booking limit of 10 slots on advanced sales. In this case, what is the probability there will be slots left over for stand-by messages
d. One problem with booking for March 24 in early January is that advertisers often withdraw their commitment to place the ad (typically this is a result of changes in promotional strategies; for example, a product may be found to be inferior or an ad may turn out to be ineffective). Because of such opportunistic behavior by advertisers, media companies often overbook advertising slots. WAMB estimates that in the past the number of withdrawn ads has a Poisson distribution with mean 9. Assume each withdrawn ad slot can still be sold at a standby price of $2,500 although the company misses an opportunity to sell these slots at $4,000 a piece. Any ad that was accepted by WAMB but cannot be accommodated (because there isn't a free slot) costs the company $10,000 in penalties. How many slots (at most) should be sold
e. Over time, WAMB saw a steady increase in the number of withdrawn ads and decided to institute a penalty of $1,000 for withdrawals. (Actually, the company now requires a $1,000 deposit on any slot. It is refunded only if WAMB is unable to provide a slot due to overbooking.) The expected number of withdrawn ads is expected to be cut in half (to only 4.5 slots). Now how many slots (at most) should be sold
 a. How many slots should WAMB sell in advance
a. How many slots should WAMB sell in advance b. In practice, there are companies willing to place standby advertising messages: if there is an empty slot available (i.e., this slot was not sold either in advance or during the last week), the standby message is placed into this slot. Since there is no guarantee that such a slot will be available, standby messages can be placed at a much lower cost. Now suppose that if a slot is not sold in advance and not sold during the last week, it will be used for a standby promotional message that costs advertisers $2,500. Now how many slots should WAMB sell in advance
c. Suppose WAMB chooses a booking limit of 10 slots on advanced sales. In this case, what is the probability there will be slots left over for stand-by messages
d. One problem with booking for March 24 in early January is that advertisers often withdraw their commitment to place the ad (typically this is a result of changes in promotional strategies; for example, a product may be found to be inferior or an ad may turn out to be ineffective). Because of such opportunistic behavior by advertisers, media companies often overbook advertising slots. WAMB estimates that in the past the number of withdrawn ads has a Poisson distribution with mean 9. Assume each withdrawn ad slot can still be sold at a standby price of $2,500 although the company misses an opportunity to sell these slots at $4,000 a piece. Any ad that was accepted by WAMB but cannot be accommodated (because there isn't a free slot) costs the company $10,000 in penalties. How many slots (at most) should be sold
e. Over time, WAMB saw a steady increase in the number of withdrawn ads and decided to institute a penalty of $1,000 for withdrawals. (Actually, the company now requires a $1,000 deposit on any slot. It is refunded only if WAMB is unable to provide a slot due to overbooking.) The expected number of withdrawn ads is expected to be cut in half (to only 4.5 slots). Now how many slots (at most) should be sold
Revenue management is concerned with maximizing the revenue by making optimal choice of capacity and pricing strategy given the supply or demand is fairly constant. In case of protection level/ booking limit is a capacity control tool. In this case, generally, two types of reservations are used to cater two types of customer arrivals. The arrival occurs in a staggered manner i.e. the low fare or discounted price offers are given the first priority is arrival. The concept from a newsvendor model from single period inventory model is borrowed in computing the optimal choice of capacity reserved for the full-priced offers.
a.
Compute the optimal quantity sold in advance as follows:
High price,
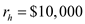 Regular price,
Regular price,
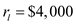
 For optimal reserved capacity of high price, the probability of high-price demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal reserved capacity of high price, the probability of high-price demand being less than or equal to reserved quantity equals the critical ratio. In other words,
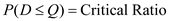 Or,
Or,
 Compute the cumulative probability from the given discrete distribution of number of slots using the Excel formulation as follows:
Compute the cumulative probability from the given discrete distribution of number of slots using the Excel formulation as follows:
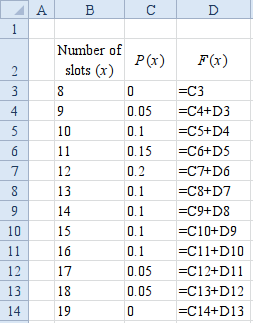 Result
Result
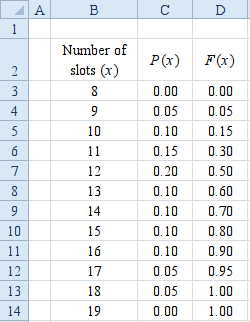 With the given high-price demand cumulative distribution as shown above, F(13) = 0.60
With the given high-price demand cumulative distribution as shown above, F(13) = 0.60
So, the optimal level of high-priced booking is 13. So, the optimal quantity that is to be sold in advance
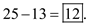 b.
b.
Compute the optimal quantity sold in advance after altering the critical ratio based on the given salvage value of the slots.
High price,
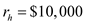 Regular price,
Regular price,
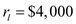
 For optimal reserved capacity of high price, the probability of high-price demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal reserved capacity of high price, the probability of high-price demand being less than or equal to reserved quantity equals the critical ratio. In other words,
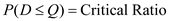 Or,
Or,
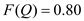 With the given high-price demand cumulative distribution, F(15) = 0.60
With the given high-price demand cumulative distribution, F(15) = 0.60
So, the optimal level of high-priced booking is 15. So, the optimal quantity that is to be sold in advance
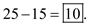 c.
c.
Given the number of slots booked in advance equal to 10, compute the probability of stock left-over for the stand-by customers as follows:
So, high-price booking
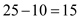 .
.
There will be stand-by customers if 14 or less slots are booked.
From the cumulative distribution table, F(14) = 0.70
So, the probability of stock left-over for the stand-by customers is
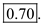 d.
d.
Compute the optimum overbooking limit using the following formula:
Cost of underage,
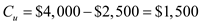 Cost of underage,
Cost of underage,
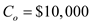
 For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,
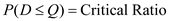 Or,
Or,
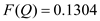 From the given Poisson table for the mean of 9, F(6) = 0.2068 0.1304
From the given Poisson table for the mean of 9, F(6) = 0.2068 0.1304
So, the optimal overbooking limit is 6 slots. So, the number of slots to be booked
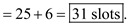 e.
e.
Compute the optimum overbooking limit using the following formula:
Cost of underage,
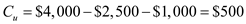 Cost of underage,
Cost of underage,
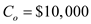
 For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,
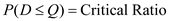 Or,
Or,
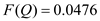 From the given Poisson table for the mean of 4.5, F(6) = 0.0611 0.0476
From the given Poisson table for the mean of 4.5, F(6) = 0.0611 0.0476
So, the optimal overbooking limit is 1 slot only. So, the number of slots to be booked
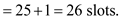 Therefore, the optimal overbooking quantity is
Therefore, the optimal overbooking quantity is
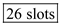 .
.
a.
Compute the optimal quantity sold in advance as follows:
High price,
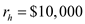 Regular price,
Regular price, 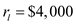
 For optimal reserved capacity of high price, the probability of high-price demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal reserved capacity of high price, the probability of high-price demand being less than or equal to reserved quantity equals the critical ratio. In other words,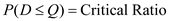 Or,
Or, 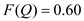 Compute the cumulative probability from the given discrete distribution of number of slots using the Excel formulation as follows:
Compute the cumulative probability from the given discrete distribution of number of slots using the Excel formulation as follows: Result
Result With the given high-price demand cumulative distribution as shown above, F(13) = 0.60
With the given high-price demand cumulative distribution as shown above, F(13) = 0.60So, the optimal level of high-priced booking is 13. So, the optimal quantity that is to be sold in advance
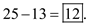 b.
b.Compute the optimal quantity sold in advance after altering the critical ratio based on the given salvage value of the slots.
High price,
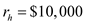 Regular price,
Regular price, 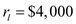
 For optimal reserved capacity of high price, the probability of high-price demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal reserved capacity of high price, the probability of high-price demand being less than or equal to reserved quantity equals the critical ratio. In other words,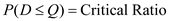 Or,
Or, 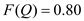 With the given high-price demand cumulative distribution, F(15) = 0.60
With the given high-price demand cumulative distribution, F(15) = 0.60So, the optimal level of high-priced booking is 15. So, the optimal quantity that is to be sold in advance
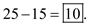 c.
c.Given the number of slots booked in advance equal to 10, compute the probability of stock left-over for the stand-by customers as follows:
So, high-price booking
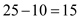 .
.There will be stand-by customers if 14 or less slots are booked.
From the cumulative distribution table, F(14) = 0.70
So, the probability of stock left-over for the stand-by customers is
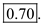 d.
d.Compute the optimum overbooking limit using the following formula:
Cost of underage,
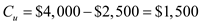 Cost of underage,
Cost of underage, 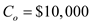
 For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,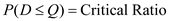 Or,
Or, 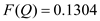 From the given Poisson table for the mean of 9, F(6) = 0.2068 0.1304
From the given Poisson table for the mean of 9, F(6) = 0.2068 0.1304So, the optimal overbooking limit is 6 slots. So, the number of slots to be booked
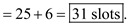 e.
e.Compute the optimum overbooking limit using the following formula:
Cost of underage,
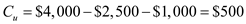 Cost of underage,
Cost of underage, 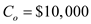
 For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,
For optimal overbooking limit, the probability of demand being less than or equal to reserved quantity equals the critical ratio. In other words,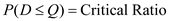 Or,
Or, 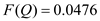 From the given Poisson table for the mean of 4.5, F(6) = 0.0611 0.0476
From the given Poisson table for the mean of 4.5, F(6) = 0.0611 0.0476So, the optimal overbooking limit is 1 slot only. So, the number of slots to be booked
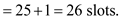 Therefore, the optimal overbooking quantity is
Therefore, the optimal overbooking quantity is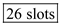 .
. 4
(Designer Dress) A fashion retailer in Santa Barbara, California, presents a new designer dress at one of the "by invitation only" fashion shows. After the show, the dress will be sold at the company's boutique store for $10,000 apiece. Demand at the boutique is limited due to the short time the dress remains fashionable and is estimated to be normal with mean 70 and standard deviation 40. There were only 100 dresses produced to maintain exclusivity and high price. It is the company's policy that all unsold merchandise is destroyed.
a. How many dresses remain unsold on average at the end of the season
b. What is the retailer's expected revenue
c. Fashion companies often sell a portion of new merchandise at exhibitions for a discount while the product is still "fresh" in the minds of the viewers. The company decides to increase revenues by selling a certain number of dresses at a greatly discounted price of $6,000 during the show. Later, remaining dresses will be available at the boutique store for a normal price of $10,000. Typically, all dresses offered at the show get sold, which, of course, decreases demand at the store: it is now normal with mean 40 and standard deviation 25. How many dresses should be sold at the show
d. Given your decision in part c, what is expected revenue
e. Given your decision in part c, how many dresses are expected to remain unsold
a. How many dresses remain unsold on average at the end of the season
b. What is the retailer's expected revenue
c. Fashion companies often sell a portion of new merchandise at exhibitions for a discount while the product is still "fresh" in the minds of the viewers. The company decides to increase revenues by selling a certain number of dresses at a greatly discounted price of $6,000 during the show. Later, remaining dresses will be available at the boutique store for a normal price of $10,000. Typically, all dresses offered at the show get sold, which, of course, decreases demand at the store: it is now normal with mean 40 and standard deviation 25. How many dresses should be sold at the show
d. Given your decision in part c, what is expected revenue
e. Given your decision in part c, how many dresses are expected to remain unsold

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
5
(Overbooking PHL-LAX) On a given Philadelphia-Los Angeles flight, there are 200 seats. Suppose the ticket price is $475 on average and the number of passengers who reserve a seat but do not show up for departure is normally distributed with mean 30 and standard deviation 15. You decide to overbook the flight and estimate that the average loss from a passenger who will have to be bumped (if the number of passengers exceeds the number of seats) is $800.
a. What is the maximum number of reservations that should be accepted
b. Suppose you allow 220 reservations. How much money do you expect to pay out in compensation to bumped passengers
c. Suppose you allow 220 reservations. What is the probability that you will have to deal with bumped passengers
a. What is the maximum number of reservations that should be accepted
b. Suppose you allow 220 reservations. How much money do you expect to pay out in compensation to bumped passengers
c. Suppose you allow 220 reservations. What is the probability that you will have to deal with bumped passengers

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
6
(PHL-LAX) Consider the Philadelphia-Los Angeles flight discussed in Q16.5. Assume the available capacity is 200 seats and there is no overbooking. The high fare is $675 and the low fare is $375. Demand for the low fare is abundant while demand for the high fare is normally distributed with a mean of 80 and standard deviation 35.
a. What is the probability of selling 200 reservations if you set an optimal protection level for the full fare
b. Suppose a protection level of 85 is established. What is the average number of lost high-fare passengers
c. Continue to assume a protection level of 85 is established. What is the expected number of unoccupied seats
d. Again assume a protection level of 85 is established. What is the expected revenue from the flight
a. What is the probability of selling 200 reservations if you set an optimal protection level for the full fare
b. Suppose a protection level of 85 is established. What is the average number of lost high-fare passengers
c. Continue to assume a protection level of 85 is established. What is the expected number of unoccupied seats
d. Again assume a protection level of 85 is established. What is the expected revenue from the flight

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
7
(Annenberg) Ron, the director at the Annenberg Center, is planning his pricing strategy for a musical to be held in a 100-seat theater. He sets the full price at $80 and estimates demand at this price to be normally distributed with mean 40 and standard deviation 30. Ron also decides to offer student-only advance sale tickets discounted 50 percent off the full price. Demand for the discounted student-only tickets is usually abundant and occurs well before full price ticket sales.
a. Suppose Ron sets a 50-seat booking limit for the student-only tickets. What is the number of full-price tickets that Ron expects to sell
b. Based on a review of the show in another city, Ron updates his demand forecast for full-price tickets to be normal with mean 60 and standard deviation 40, but he does not change the prices. What is the optimal protection level for full-price seats
c. Ron realizes that having many empty seats negatively affects the attendees' value from the show. Hence, he decides to change the discount given on student-only tickets from 50 percent off the full price to 55 percent off the full price and he continues to set his protection level optimally. (The demand forecast for full-price tickets remains as in b, normal with mean 60 and standard deviation 40.) How will this change in the student-only discount price affect the expected number of empty seats (Will they increase, decrease, or remain the same or it is not possible to determine what will happen )
d. Ron knows that on average eight seats (Poisson distributed) remain empty due to no-shows. Ron also estimates that it is 10 times more costly for him to have one more attendee than seats relative to having one empty seat in the theater. What is the maximum number of seats to sell in excess of capacity
a. Suppose Ron sets a 50-seat booking limit for the student-only tickets. What is the number of full-price tickets that Ron expects to sell
b. Based on a review of the show in another city, Ron updates his demand forecast for full-price tickets to be normal with mean 60 and standard deviation 40, but he does not change the prices. What is the optimal protection level for full-price seats
c. Ron realizes that having many empty seats negatively affects the attendees' value from the show. Hence, he decides to change the discount given on student-only tickets from 50 percent off the full price to 55 percent off the full price and he continues to set his protection level optimally. (The demand forecast for full-price tickets remains as in b, normal with mean 60 and standard deviation 40.) How will this change in the student-only discount price affect the expected number of empty seats (Will they increase, decrease, or remain the same or it is not possible to determine what will happen )
d. Ron knows that on average eight seats (Poisson distributed) remain empty due to no-shows. Ron also estimates that it is 10 times more costly for him to have one more attendee than seats relative to having one empty seat in the theater. What is the maximum number of seats to sell in excess of capacity

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
8
(Park Hyatt) Consider the example of the Park Hyatt Philadelphia discussed in the text. Recall that the full fare is $225, the expected full-fare demand is Poisson with mean 27.3, the discount fare is $159, and there are 118 king/queen rooms. Now suppose the cost of an occupied room is $45 per night. That cost includes the labor associated with prepping and cleaning a room, the additional utilities used, and the wear and tear on the furniture and fixtures. Suppose the Park Hyatt wishes to maximize expected profit rather than expected revenue. What is the optimal protection level for the full fare

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
9
(MBA Admissions) Each year the admissions committee at a top business school receives a large number of applications for admission to the MBA program and they have to decide on the number of offers to make. Since some of the admitted students may decide to pursue other opportunities, the committee typically admits more students than the ideal class size of 720 students. You were asked to help the admissions committee estimate the appropriate number of people who should be offered admission. It is estimated that in the coming year the number of people who will not accept the admission offer is normally distributed with mean 50 and standard deviation 21. Suppose for now that the school does not maintain a waiting list, that is, all students are accepted or rejected.
a. Suppose 750 students are admitted. What is the probability that the class size will be at least 720 students
b. It is hard to associate a monetary value with admitting too many students or admitting too few. However, there is a mutual agreement that it is about two times more expensive to have a student in excess of the ideal 720 than to have fewer students in the class. What is the appropriate number of students to admit
c. A waiting list mitigates the problem of having too few students since at the very last moment there is an opportunity to admit some students from the waiting list. Hence, the admissions committee revises its estimate: It claims that it is five times more expensive to have a student in excess of 720 than to have fewer students accept among the initial group of admitted students. What is your revised suggestion
a. Suppose 750 students are admitted. What is the probability that the class size will be at least 720 students
b. It is hard to associate a monetary value with admitting too many students or admitting too few. However, there is a mutual agreement that it is about two times more expensive to have a student in excess of the ideal 720 than to have fewer students in the class. What is the appropriate number of students to admit
c. A waiting list mitigates the problem of having too few students since at the very last moment there is an opportunity to admit some students from the waiting list. Hence, the admissions committee revises its estimate: It claims that it is five times more expensive to have a student in excess of 720 than to have fewer students accept among the initial group of admitted students. What is your revised suggestion

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
10
(Air Cargo) An air cargo company must decide how to sell its capacity. It could sell a portion of its capacity with long-term contracts. A long-term contract specifies that the buyer (the air cargo company's customer) will purchase a certain amount of cargo space at a certain price. The long-term contract rate is currently $1,875 per standard unit of space. If long-term contracts are not signed, then the company can sell its space on the spot market. The spot market price is volatile, but the expected future spot price is around $2,100. In addition, spot market demand is volatile: sometimes the company can find customers; other times it cannot on a short-term basis. Let's consider a specific flight on a specific date. The company's capacity is 58 units. Furthermore, the company expects that spot market demand is normally distributed with mean 65 and standard deviation 45. On average, it costs the company $330 in fuel, handling, and maintenance to fly a unit of cargo.
a. Suppose the company relied exclusively on the spot market, that is, it signed no long-term contracts. What would be the company's expected profit
b. Suppose the company relied exclusively on long-term contracts. What would be the company's expected profit
c. Suppose the company is willing to use both the long-term and the spot markets. How many units of capacity should the company sell with long-term contracts to maximize revenue
d. Suppose the company is willing to use both the long-term and the spot markets. How many units of capacity should the company sell with long-term contracts to maximize profit
a. Suppose the company relied exclusively on the spot market, that is, it signed no long-term contracts. What would be the company's expected profit
b. Suppose the company relied exclusively on long-term contracts. What would be the company's expected profit
c. Suppose the company is willing to use both the long-term and the spot markets. How many units of capacity should the company sell with long-term contracts to maximize revenue
d. Suppose the company is willing to use both the long-term and the spot markets. How many units of capacity should the company sell with long-term contracts to maximize profit

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck



