Deck 3: Current and Resistance
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/77
Play
Full screen (f)
Deck 3: Current and Resistance
1
If charges flow very slowly through a metal, why does it not require several hours for a light to come on when you throw a switch
Free electron theory states that the free electrons in wire are moving in random fashion, so, the average velocity of the electrons in the wire is less.
If we connect a battery to the given wire, some potential difference is set up between the ends of the wire. This potential difference is responsible for electric filed everywhere inside the wire. This electric filed drift the random electrons to single direction with a high speed, the light come on almost immediately after you throw the switch.
If we connect a battery to the given wire, some potential difference is set up between the ends of the wire. This potential difference is responsible for electric filed everywhere inside the wire. This electric filed drift the random electrons to single direction with a high speed, the light come on almost immediately after you throw the switch.
2
A person notices a mild shock if the current along a path through the thumb and index finger exceeds 80 µ A. Compare the maximum possible voltage without shock across the thumb and index finger with a dry-skin resistance of 4.0 × 10 5 and a wet-skin resistance of 2 000 V
Calculate the maximum possible voltage across the dry and wet skin, using the following equation.
Voltage across any conductor is the product of current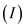 through the conductor and resistance
through the conductor and resistance 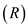 of the conductor.
of the conductor. 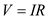 Substitute
Substitute 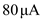 for
for 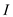 and
and 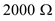 for
for 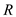 in the equation
in the equation 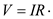
 Therefore, maximum possible voltage across the wet skin is
Therefore, maximum possible voltage across the wet skin is 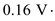 Substitute
Substitute 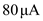 for
for 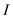 and
and 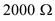 for
for 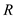 in the equation
in the equation 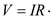
 Therefore, maximum possible voltage across the dry skin is
Therefore, maximum possible voltage across the dry skin is 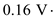 Now dividing
Now dividing 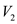 with
with 
 Therefore, voltage across the dry skin is
Therefore, voltage across the dry skin is 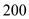 times the voltage across the wet skin.
times the voltage across the wet skin.
Voltage across any conductor is the product of current
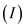 through the conductor and resistance
through the conductor and resistance 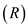 of the conductor.
of the conductor. 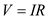 Substitute
Substitute 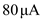 for
for 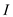 and
and 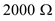 for
for 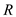 in the equation
in the equation 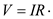
 Therefore, maximum possible voltage across the wet skin is
Therefore, maximum possible voltage across the wet skin is 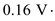 Substitute
Substitute 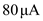 for
for 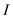 and
and 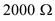 for
for 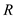 in the equation
in the equation 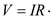
 Therefore, maximum possible voltage across the dry skin is
Therefore, maximum possible voltage across the dry skin is 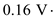 Now dividing
Now dividing 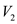 with
with 
 Therefore, voltage across the dry skin is
Therefore, voltage across the dry skin is 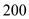 times the voltage across the wet skin.
times the voltage across the wet skin. 3
At 20°C, the carbon resistor in an electric circuit connected to a 5.0-V battery has a resistance of 2.0 × 10 2 . What is the current in the circuit when the temperature of the carbon rises to 80°C
Ohm's law states that the potential difference across the resistor is directly proportional to the current in the resistor. 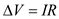 Here, I is the current and R is the resistance.
Here, I is the current and R is the resistance.
Rewrite the equation for I.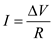 The resistance
The resistance 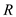 of a resistor is related to its initial resistance
of a resistor is related to its initial resistance  , temperature coefficient of resistivity
, temperature coefficient of resistivity 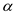 , and temperature deference
, and temperature deference 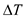 .The resistance is written as,
.The resistance is written as,  Substitute
Substitute  for
for 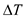 .
. 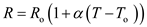 Substitute
Substitute 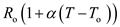 for
for 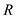 in the equation
in the equation 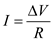 .
. 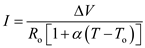 Substitute
Substitute 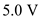 for
for 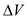 ,
, 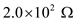 for
for  ,
, 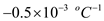 for
for 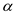 ,
,  for T and
for T and  for
for  .
.  Therefore, the current in the circuit is
Therefore, the current in the circuit is 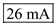 .
.
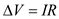 Here, I is the current and R is the resistance.
Here, I is the current and R is the resistance.Rewrite the equation for I.
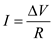 The resistance
The resistance 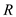 of a resistor is related to its initial resistance
of a resistor is related to its initial resistance  , temperature coefficient of resistivity
, temperature coefficient of resistivity 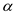 , and temperature deference
, and temperature deference 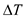 .The resistance is written as,
.The resistance is written as,  Substitute
Substitute  for
for 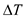 .
. 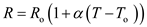 Substitute
Substitute 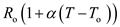 for
for 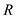 in the equation
in the equation 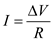 .
. 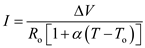 Substitute
Substitute 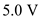 for
for 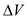 ,
, 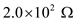 for
for  ,
, 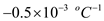 for
for 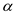 ,
,  for T and
for T and  for
for  .
.  Therefore, the current in the circuit is
Therefore, the current in the circuit is 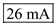 .
. 4
The potential difference across a resting neuron in the human body is about 75.0 mV and carries a current of about 0.200 mA. How much power does the neuron release

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
5
A 120-V motor has mechanical power output of 2.50 hp. It is 90.0% efficient in converting power that it takes in by electrical transmission into mechanical power. (a) Find the current in the motor. (b) Find the energy delivered to the motor by electrical transmission in 3.00 h of operation. (c) If the electric company charges $0.110/kWh, what does it cost to run the motor for 3.00 h

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
6
In the Bohr model of the hydrogen atom, an electron in the lowest energy state moves at a speed equal to 2.19 × 10 6 m/s in a circular path having a radius of 5.29 × 10 11 m. What is the effective current associated with this orbiting electron

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
7
Suppose you wish to fabricate a uniform wire out of 1.00 g of copper. If the wire is to have a resistance R = 0.500 , and if all the copper is to be used, what will be (a) the length and (b) the diameter of the wire

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
8
A wire 3.00 m long and 0.450 mm 2 in cross-sectional area has a resistance of 41.0 at 20°C. If its resistance increases to 41.4 at 29.0°C, what is the temperature coefficient of resistivity

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
9
The cost of electricity varies widely throughout the United States; $0.120/kWh is a typical value. At this unit price, calculate the cost of (a) leaving a 40.0-W porch light on for 2 weeks while you are on vacation, (b) making a piece of dark toast in 3.00 min with a 970-W toaster, and (c) drying a load of clothes in 40.0 min in a 5 200-W dryer.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
10
A Nichrome heating element in an oven has a resistance of 8.0 V at 20°C. (a) What is its resistance at 350°C (b) What assumption did you have to make to obtain your answer to part (a)

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
11
In an analogy between traffic flow and electrical current, (a) what would correspond to the charge Q (b) What would correspond to the current I

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
12
The current supplied by a battery in a portable device is typically 0.15 A. Find the number of electrons passing through the device in one hour

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
13
Nichrome wire of cross-sectional radius 0.791 mm is to be used in winding a heating coil. If the coil must carry a current of 9.25 A when a voltage of 1.20 × 10 2 is applied across its ends, find (a) the required resistance of the coil and (b) the length of wire you must use to wind the coil

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
14
(a) A 34.5-m length of copper wire at 20.0°C has a radius of 0.25 mm. If a potential difference of 9.0 V is applied across the length of the wire, determine the current in the wire. (b) If the wire is heated to 30.0°C while the 9.0-V potential difference is maintained, what is the resulting current in the wire

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
15
An electric utility company supplies a customer's house from the main power lines (120 V) with two copper wires, each of which is 50.0 m long and has a resistance of 0.108 per 300 m. (a) Find the potential difference at the customer's house for a load current of 110 A. For this load current, find (b) the power delivered to the customer.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
16
A length of metal wire has a radius of 5.00 × 10 3 m and a resistance of 0.100 . When the potential difference across the wire is 15.0 V, the electron drift speed is found to be 3.17 × 10 4 m/s. On the basis of these data, calculate the density of free electrons in the wire.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
17
When the voltage across a certain conductor is doubled, the current is observed to triple. What can you conclude about the conductor

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
18
A wire of diameter 0.800 mm and length 25.0 m has a measured resistance of 1.60 . What is the resistivity of the wire

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
19
An engineer needs a resistor with a zero overall temperature coefficient of resistance at 20.0°C. She designs a pair of circular cylinders, one of carbon and one of Nichrome as shown in Figure P17.30. The device must have an overall resistance of R 1 + R 2 = 10.0 independent of temperature and a uniform radius of r = 1.50 mm. Ignore thermal expansion of the cylinders and assume both are always at the same temperature. (a) Can she meet the design goal with this method (b) If so, state what you can determine about the lengths L 1 and L 2 of each segment. If not, explain.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
20
An office worker uses an immersion heater to warm 250 g of water in a light, covered, insulated cup from 20°C to 100°C in 4.00 minutes. The heater is a Nichrome resistance wire connected to a 120-V power supply. Assume the wire is at 100°C throughout the 4.00-min time interval. (a) Calculate the average power required to warm the water to 100°C in 4.00 min. (b) Calculate the required resistance in the heating element at 100°C. (c) Calculate the resistance of the heating element at 20.0°C. (d) Derive a relationship between the diameter of the wire, the resistivity at 20.0°C, 0 , the resistance at 20.0°C, R 0 , and the length L. (e) If L = 3.00 m, what is the diameter of the wire

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
21
In a certain stereo system, each speaker has a resistance of 4.00 . The system is rated at 60.0 W in each channel. Each speaker circuit includes a fuse rated at a maximum current of 4.00 A. Is this system adequately protected against overload

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
22
A certain laboratory experiment requires an aluminum wire of length of 32.0 m and a resistance of 2.50 at 20.0°C. What diameter wire must be used

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
23
A potential difference of 12 V is found to produce a current of 0.40 A in a 3.2-m length of wire with a uniform radius of 0.40 cm. What is (a) the resistance of the wire (b) The resistivity of the wire

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
24
In one form of plethysmograph (a device for measuring volume), a rubber capillary tube with an inside diameter of 1.00 mm is filled with mercury at 20°C. The resistance of the mercury is measured with the aid of electrodes sealed into the ends of the tube. If 100.00 cm of the tube is wound in a spiral around a patient's upper arm, the blood flow during a heartbeat causes the arm to expand, stretching the tube to a length of 100.04 cm. From this observation, and assuming cylindrical symmetry, you can find the change in volume of the arm, which gives an indication of blood flow. (a) Calculate the resistance of the mercury. (b) Calculate the fractional change in resistance during the heartbeat. Take H g = 9.4 × 10 7 m. Hint: Because the cylindrical volume is constant, V = A i L i = A f L f and A f = A i ( L i / L f )

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
25
Two wires A and B made of the same material and having the same lengths are connected across the same voltage source. If the power supplied to wire A is three times the power supplied to wire B , what is the ratio of their diameters

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
26
A resistor is constructed by forming a material of resistivity 3.5 × 10 5 · m into the shape of a hollow cylinder of length 4.0 cm and inner and outer radii 0.50 cm and 1.2 cm, respectively. In use, a potential difference is applied between the ends of the cylinder, producing a current parallel to the length of the cylinder. Find the resistance of the cylinder.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
27
Two lightbulbs are each connected to a voltage of 120 V. One has a power of 25 W, the other 100 W. (a) Which lightbulb has the higher resistance (b) Which lightbulb carries more current

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
28
Aluminum and copper wires of equal length are found to have the same resistance. What is the ratio of their radii

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
29
A platinum resistance thermometer has resistances of 200.0 when placed in a 0°C ice bath and 253.8 when immersed in a crucible containing melting potassium. What is the melting point of potassium Hint: First determine the resistance of the platinum resistance thermometer at room temperature, 20°C.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
30
A tungsten wire in a vacuum has length 15.0 cm and radius 1.00 mm. A potential difference is applied across it. (a) What is the resistance of the wire at 293 K (b) Suppose the wire reaches an equilibrium temperature such that it emits 75.0 W in the form of radiation. Neglecting absorption of any radiation from its environment, what is the temperature of the wire ( Note: = 0.320 for tungsten.) (c) What is the resistance of the wire at the temperature found in part (b) Assume the temperature changes linearly over this temperature range. (d) What voltage drop is required across the wire (e) Why are tungsten lightbulbs energetically inefficient as light sources

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
31
When a straight wire is heated, its resistance changes according to the equation 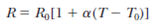
(Eq. 17.7), where a is the temperature coefficient of resistivity. (a) Show that a more precise result, which includes the length and area of a wire change when it is heated, is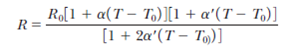
where is the coefficient of linear expansion. (See Chapter 10.) (b) Compare the two results for a 2.00-m-long copper wire of radius 0.100 mm, starting at 20.0°C and heated to 100.0°C.
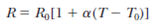
(Eq. 17.7), where a is the temperature coefficient of resistivity. (a) Show that a more precise result, which includes the length and area of a wire change when it is heated, is
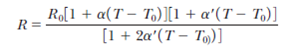
where is the coefficient of linear expansion. (See Chapter 10.) (b) Compare the two results for a 2.00-m-long copper wire of radius 0.100 mm, starting at 20.0°C and heated to 100.0°C.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
32
If 3.25 × 10 3 kg of gold is deposited on the negative electrode of an electrolytic cell in a period of 2.78 h, what is the current in the cell during that period Assume the gold ions carry one elementary unit of positive charge

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
33
A wire 50.0 m long and 2.00 mm in diameter is connected to a source with a potential difference of 9.11 V, and the current is found to be 36.0 A. Assume a temperature of 20°C and, using Table 17.1, identify the metal out of which the wire is made

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
34
Suppose your waffle iron is rated at 1.00 kW when connected to a 1.20 × 10 2 source. (a) What current does the waffle iron carry (b) What is its resistance

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
35
If a battery is rated at 60.0 A · h, how much total charge can it deliver before it goes "dead"

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
36
An x-ray tube used for cancer therapy operates at 4.0 MV, with a beam current of 25 mA striking the metal target. Nearly all the power in the beam is transferred to a stream of water flowing through holes drilled in the target. What rate of flow, in kilograms per second, is needed if the rise in temperature ( T ) of the water is not to exceed 50°C

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
37
Newspaper articles often have statements such as "10 000 volts of electricity surged through the victim's body." What is wrong with this statement

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
38
A rectangular block of copper has sides of length 10 cm, 20 cm, and 40 cm. If the block is connected to a 6.0-V source across two of its opposite faces, what are (a) the maximum current and (b) the minimum current the block can carry

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
39
If electrical energy costs $0.12 per kilowatt-hour, how much does it cost to (a) burn a 100-W lightbulb for 24 h (b) Operate an electric oven for 5.0 h if it carries a current of 20.0 A at 220 V

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
40
A car owner forgets to turn off the headlights of his car while it is parked in his garage. If the 12.0-V battery in his car is rated at 90.0 A · h and each headlight requires 36.0 W of power, how long will it take the battery to completely discharge

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
41
A man wishes to vacuum his car with a canister vacuum cleaner marked 535 W at 120 V. The car is parked far from the building, so he uses an extension cord 15.0 m long to plug the cleaner into a 120-V source. Assume the cleaner has constant resistance. (a) If the resistance of each of the two conductors of the extension cord is 0.900 V, what is the actual power delivered to the cleaner (b) If, instead, the power is to be at least 525 W, what must be the diameter of each of two identical copper conductors in the cord the young man buys (c) Repeat part (b) if the power is to be at least 532 W. Suggestion: A symbolic solution can simplify the calculations.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
42
A 200-km-long high-voltage transmission line 2.0 cm in diameter carries a steady current of 1 000 A. If the conductor is copper with a free charge density of 8.5 × 10 8 electrons per cubic meter, how many years does it take one electron to travel the full length of the cable

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
43
A wire of initial length L 0 and radius r 0 has a measured resistance of 1.0 . The wire is drawn under tensile stress to a new uniform radius of r = 0.25 r 0. What is the new resistance of the wire

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
44
Residential building codes typically require the use of 12-gauge copper wire (diameter 0.205 cm) for wiring receptacles. Such circuits carry currents as large as 20.0 A. If a wire of smaller diameter (with a higher gauge number) carried that much current, the wire could rise to a high temperature and cause a fire. (a) Calculate the rate at which internal energy is produced in 1.00 m of 12-gauge copper wire carrying 20.0 A. (b) Repeat the calculation for a 12-gauge aluminum wire. (c) Explain whether a 12-gauge aluminum wire would be as safe as a copper wire.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
45
Consider an aluminum wire of diameter 0.600 mm and length 15.0 m. The resistivity of aluminum at 20.0°C is 2.82 × 10 8 · m. (a) Find the resistance of this wire at 20.0°C. (b) If a 9.00 V battery is connected across the ends of the wire, find the current in the wire.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
46
There is an old admonition given to experimenters to "keep one hand in the pocket" when working around high voltages. Why is this warning a good idea

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
47
The human body can exhibit a wide range of resistances to current depending on the path of the current, contact area, and sweatiness of the skin. Suppose the resistance across the chest from the left hand to the right hand is 1.0 × 10 6 . (a) How much voltage is required to cause possible heart fibrillation in a man, which corresponds to 500 mA of direct current (b) Why should rubber-soled shoes and rubber gloves be worn when working around electricity

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
48
A high-voltage transmission line with a resistance of 0.31 /km carries a current of 1 000 A. The line is at a potential of 700 kV at the power station and carries the current to a city located 160 km from the station. (a) What is the power loss due to resistance in the line (b) What fraction of the transmitted power does this loss represent

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
49
A given copper wire has a resistance of 5.00 at 20.0°C while a tungsten wire of the same diameter has a resistance of 4.75 at 20.0°C. At what temperature will the two wires have the same resistance

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
50
An aluminum wire having a cross-sectional area of 4.00 × 10 6 m 2 carries a current of 5.00 A. The density of aluminum is 2.70 g/cm 3. Assume each aluminum atom supplies one conduction electron per atom. Find the drift speed of the electrons in the wire

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
51
Starting from Ohm's law, show that E = J , where E is the magnitude of the electric field (assumed constant) and J = I / A is called the current density. The result is in fact true in general.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
52
The heating element of a coffeemaker operates at 120 V and carries a current of 2.00 A. Assuming the water absorbs all the energy converted by the resistor, calculate how long it takes to heat 0.500 kg of water from room temperature (23.0°C) to the boiling point.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
53
A particular wire has a resistivity of 3.0 × 10 8 V · m and a cross-sectional area of 4.0 × 10 6 m 2. A length of this wire is to be used as a resistor that will develop 48 W of power when connected across a 20-V battery. What length of wire is required

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
54
What could happen to the drift velocity of the electrons in a wire and to the current in the wire if the electrons could move through it freely without resistance

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
55
If a certain silver wire has a resistance of 6.00 at 20.0°C, what resistance will it have at 34.0°C

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
56
The power supplied to a typical black-and-white television set is 90 W when the set is connected to 120 V. (a) How much electrical energy does this set consume in 1 hour (b) A color television set draws about 2.5 A when connected to 120 V. How much time is required for it to consume the same energy as the black-andwhite model consumes in 1 hour

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
57
Birds resting on high-voltage power lines are a common sight. The copper wire on which a bird stands is2.2 cm in diameter and carries a current of 50 A. If the bird's feet are 4.0 cm apart, calculate the potential difference between its feet.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
58
We have seen that an electric field must exist inside a conductor that carries a current. How is that possible in view of the fact that in electrostatics we concluded that the electric field must be zero inside a conductor

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
59
An iron wire has a cross-sectional area of 5.00 × 10 6 m 2. Carry out steps (a) through (e) to compute the drift speed of the conduction electrons in the wire. (a) How many kilograms are there in 1 mole of iron (b) Starting with the density of iron and the result of part (a), compute the molar density of iron (the number of moles of iron per cubic meter). (c) Calculate the number density of iron atoms using Avogadro's number. (d) Obtain the number density of conduction electrons given that there are two conduction electrons per iron atom. (e) If the wire carries a current of 30.0 A, calculate the drift speed of conduction electrons.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
60
While taking photographs in Death Valley on a day when the temperature is 58.0°C, Bill Hiker finds that a certain voltage applied to a copper wire produces a current of 1.000 A. Bill then travels to Antarctica and applies the same voltage to the same wire. What current does he register there if the temperature is 88.0°C Assume no change occurs in the wire's shape and size

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
61
Lightbulb A is marked "25.0 W 120 V," and lightbulb B is marked "100 W 120 V." These labels mean that each lightbulb has its respective power delivered to it when it is connected to a constant 120-V source. (a) Find the resistance of each lightbulb. (b) During what time interval does 1.00 C pass into lightbulb A (c) Is this charge different upon its exit versus its entry into the lightbulb Explain. (d) In what time interval does 1.00 J pass into lightbulb A (e) By what mechanisms does this energy enter and exit the lightbulb Explain. (f) Find the cost of running lightbulb A continuously for 30.0 days, assuming the electric company sells its product at $0.110 per kWh.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
62
An experiment is conducted to measure the electrical resistivity of Nichrome in the form of wires with different lengths and cross-sectional areas. For one set of measurements, a student uses 30-gauge wire, which has a cross-sectional area of 7.30 3 × m 2. The student measures the potential difference across the wire and the current in the wire with a voltmeter and an ammeter, respectively. For each of the measurements given in the following table taken on wires of three different lengths, calculate the resistance of the wires and the corresponding value of the resistivity. 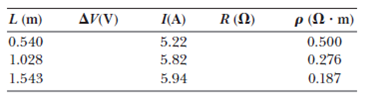
What is the average value of the resistivity, and how does this value compare with the value given in Table 17.1

What is the average value of the resistivity, and how does this value compare with the value given in Table 17.1

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
63
If a current of 80.0 mA exists in a metal wire, (a) how many electrons flow past a given cross section of the wire in 10.0 min (b) In what direction do the electrons travel with respect to the current

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
64
Some homes have light dimmers that are operated by rotating a knob. What is being changed in the electric circuit when the knob is rotated

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
65
A length of aluminum wire has a resistance of 30.0 at 20.0°C. When the wire is warmed in an oven and reaches thermal equilibrium, the resistance of the wire increases to 46.2 . (a) Neglecting thermal expansion, find the temperature of the oven. (b) Qualitatively, how would thermal expansion be expected to affect the answer

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
66
A certain toaster has a heating element made of Nichrome resistance wire. When the toaster is first connected to a 120-V source of potential difference (and the wire is at a temperature of 20.0°C), the initial current is 1.80 A but the current begins to decrease as the resistive element warms up. When the toaster reaches its final operating temperature, the current has dropped to 1.53 A. (a) Find the power the toaster converts when it is at its operating temperature. (b) What is the final temperature of the heating element

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
67
A 50.0-g sample of a conducting material is all that is available. The resistivity of the material is measured to be 11 × 10 8 · m, and the density is 7.86 g/cm 3. The material is to be shaped into a solid cylindrical wire that has a total resistance of 1.5 . (a) What length of wire is required (b) What must be the diameter of the wire

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
68
Use the atomic theory of matter to explain why the resistance of a material should increase as its temperature increases.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
69
An electric heater carries a current of 13.5 A when operating at a voltage of 1.20 × 10 2 V. What is the resistance of the heater

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
70
Gold is the most ductile of all metals. For example, one gram of gold can be drawn into a wire 2.40 km long. The density of gold is 19.3 × 10 3 kg/m 3 , and its resistivity is 2.44 × 10 8 m. What is the resistance of such a wire at 20.0°C

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
71
A copper cable is designed to carry a current of 300 A with a power loss of 2.00 W/m. What is the required radius of this cable

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
72
You are cooking breakfast for yourself and a friend using a 1 200-W waffle iron and a 500-W coffeepot. Usually, you operate these appliances from a 110-V outlet for 0.500 h each day. (a) At 12 cents per kWh, how much do you spend to cook breakfast during a 30.0-day period (b) You find yourself addicted to waffles and would like to upgrade to a 2 400-W waffle iron that will enable you to cook twice as many waffles during a half-hour period, but you know that the circuit breaker in your kitchen is a 20-A breaker. Can you do the upgrade

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
73
A copper wire has a circular cross section with a radius of 1.25 mm. (a) If the wire carries a current of 3.70 A, find the drift speed of the electrons in the wire. (See Example 17.2 for relevant data on copper.) (b) All other things being equal, what happens to the drift speed in wires made of metal having a larger number of conduction electrons per atom than copper Explain

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
74
When is more power delivered to a lightbulb, immediately after it is turned on and the glow of the filament is increasing or after it has been on for a few seconds and the glow is steady

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
75
At what temperature will aluminum have a resistivity that is three times the resistivity of copper at room temperature

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
76
Batteries are rated in terms of ampere-hours (A · h). For example, a battery that can deliver a current of 3.0 A for 5.0 h is rated at 15 A · h. (a) What is the total energy, in kilowatt-hours, stored in a 12-V battery rated at 55 A · h (b) At $0.12 per kilowatt-hour, what is the value of the electricity that can be produced by this battery

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
77
The current in a conductor varies in time as shown in Figure P17.58. (a) How many coulombs of charge pass through a cross section of the conductor in the interval from t = 0 to t = 5.0 s (b) What constant current would transport the same total charge during the 5.0-s interval as does the actual current 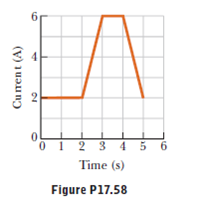


Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck



