Deck 15: Nonparametric Statistics
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/66
Play
Full screen (f)
Deck 15: Nonparametric Statistics
1
The runs test is appropriate for which type of data?
A) two or more independent samples from continuous distributions
B) a single sample in which each observation is classified into one of two mutually exclusive categories
C) a single sample in which each observation is classified into one of several (two or more) mutually exclusive categories
D) a single random and independent sample from a continuous non-normal distribution
A) two or more independent samples from continuous distributions
B) a single sample in which each observation is classified into one of two mutually exclusive categories
C) a single sample in which each observation is classified into one of several (two or more) mutually exclusive categories
D) a single random and independent sample from a continuous non-normal distribution
a single sample in which each observation is classified into one of two mutually exclusive categories
2
A Kruskal-Wallis test has the following characteristics: 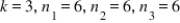 What would be the correct chi-square critical value for this test if α = 0.01?
What would be the correct chi-square critical value for this test if α = 0.01?
A) 11.3449
B) 33.4087
C) 9.2103
D) 10.5966
 What would be the correct chi-square critical value for this test if α = 0.01?
What would be the correct chi-square critical value for this test if α = 0.01?A) 11.3449
B) 33.4087
C) 9.2103
D) 10.5966
9.2103
3
A two-sample t test with independent samples is the parametric version of a:
A) Wilcoxon signed-rank test for paired samples.
B) Wilcoxon rank-sum test.
C) Wilcoxon signed-rank test for one sample.
D) runs test.
A) Wilcoxon signed-rank test for paired samples.
B) Wilcoxon rank-sum test.
C) Wilcoxon signed-rank test for one sample.
D) runs test.
Wilcoxon rank-sum test.
4
In a Kruskal-Wallis test for comparing three populations, the test statistic is calculated as H = 2.80.If the test is conducted at the 5% significance level, then:
A) the null hypothesis will not be rejected.
B) the null hypothesis will be rejected.
C) the test results are inconclusive.
D) the t test for matched pairs must be used.
A) the null hypothesis will not be rejected.
B) the null hypothesis will be rejected.
C) the test results are inconclusive.
D) the t test for matched pairs must be used.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
5
Maintenance is done to increase the printing speed of an office printer.The amount of time (in seconds) for each of the six employees to print a standardized report using this printer before the maintenance is recorded in the table.The amount of time for the same employees to print these same reports after the maintenance is also recorded. 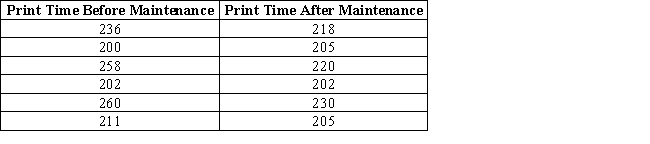
Assuming the distributions are symmetric and continuous, conduct a Wilcoxon signed-rank test to see if it appears that the median print time decreased after the maintenance.Select the correct critical value (CV), test statistic (TS), and decision.(Use α = 0.10.)
A) CV = 6, TS = 18, reject H₀
B) CV = 17, TS = 14, fail to reject H₀
C) CV = 2, TS = 10, reject H₀
D) CV = 13, TS = 14, reject H₀

Assuming the distributions are symmetric and continuous, conduct a Wilcoxon signed-rank test to see if it appears that the median print time decreased after the maintenance.Select the correct critical value (CV), test statistic (TS), and decision.(Use α = 0.10.)
A) CV = 6, TS = 18, reject H₀
B) CV = 17, TS = 14, fail to reject H₀
C) CV = 2, TS = 10, reject H₀
D) CV = 13, TS = 14, reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
6
Calculate the Spearman's rank correlation coefficient for the following data set:  .
.
A) 0.6569
B) 0.2211
C) -0.1125
D) 0.8750
 .
.A) 0.6569
B) 0.2211
C) -0.1125
D) 0.8750

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
7
The amount of time spent waiting for the bus at a city bus stop is symmetrically distributed.Eight randomly sampled people who catch the bus at this stop are asked how long they had to wait.Their wait times (in minutes) are: 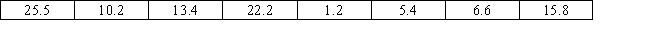
Conduct a Wilcoxon signed-rank test to see if the median wait time is more than 20 minutes.Select the correct critical value (CV), test statistic (TS), and decision.(Use α = 0.05.)
A) CV = 6, TS = 4, reject H₀
B) CV = 30, TS = 4, fail to reject H₀
C) CV = 30, TS = 3, reject H₀
D) CV = 6, TS = 4, fail to reject H₀
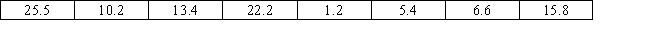
Conduct a Wilcoxon signed-rank test to see if the median wait time is more than 20 minutes.Select the correct critical value (CV), test statistic (TS), and decision.(Use α = 0.05.)
A) CV = 6, TS = 4, reject H₀
B) CV = 30, TS = 4, fail to reject H₀
C) CV = 30, TS = 3, reject H₀
D) CV = 6, TS = 4, fail to reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
8
An athletic trainer is concerned that his athletes are not drinking enough water during games.He randomly samples 11 previous games with comparable temperature and humidity and records the amount of water consumed by the team in liters (shown in the table).What is the null hypothesis?
Amount of Water Consumed per Game (in liters)
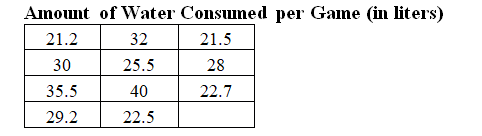
A) H₀: The median amount of water consumed by the team is equal to 30 L.
B) H₀: The mean amount of water consumed by the team is equal to 30 L.
C) H₀: The median amount of water consumed by the team is less than 30 L.
D) H₀: The mean amount of water consumed by the team is less than 30 L.
Amount of Water Consumed per Game (in liters)

A) H₀: The median amount of water consumed by the team is equal to 30 L.
B) H₀: The mean amount of water consumed by the team is equal to 30 L.
C) H₀: The median amount of water consumed by the team is less than 30 L.
D) H₀: The mean amount of water consumed by the team is less than 30 L.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
9
The first 10 final exams turned in for a particular class are classified as either pass (earned a grade of "C" or higher) or fail (earned a grade lower than "C").The sequence of exam submission and the results are shown here: 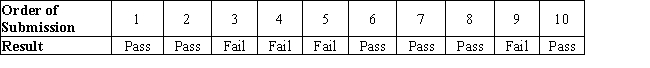
A runs test with α = 0.05 is used to determine whether there is any evidence that the order in which the exams were submitted was not random.What is the alternative hypothesis in this case?
A) The statistics will conform that the distribution is random.
B) The distribution can be modeled as a normal curve.
C) There is not alternative hypothesis; there are too few data points to analyze correctly.
D) The distribution is not random.

A runs test with α = 0.05 is used to determine whether there is any evidence that the order in which the exams were submitted was not random.What is the alternative hypothesis in this case?
A) The statistics will conform that the distribution is random.
B) The distribution can be modeled as a normal curve.
C) There is not alternative hypothesis; there are too few data points to analyze correctly.
D) The distribution is not random.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
10
Which of the following is a disadvantage of the sign test?
A) Tied pairs are not considered in the analysis.
B) Only the signs of the differences, not the actual values, are used in the analysis.
C) The sign test cannot be used when the sample sizes are small.
D) The results from a sign test have little practical value.
A) Tied pairs are not considered in the analysis.
B) Only the signs of the differences, not the actual values, are used in the analysis.
C) The sign test cannot be used when the sample sizes are small.
D) The results from a sign test have little practical value.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
11
The sign test is a nonparametric procedure for testing:
A) the amount of skewness in a population.
B) whether two populations have identical probability distributions.
C) whether two populations have identical means.
D) whether two populations have identical medians.
A) the amount of skewness in a population.
B) whether two populations have identical probability distributions.
C) whether two populations have identical means.
D) whether two populations have identical medians.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
12
In a Kruskal-Wallis test, the null hypothesis is always that:
A)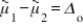 .
.
B)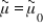 .
.
C) the sequence of observations is random.
D) the k samples are from identical populations.
A)
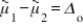 .
.B)
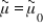 .
.C) the sequence of observations is random.
D) the k samples are from identical populations.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
13
In the sign test on a population median, what is done with values equal to the hypothesized median?
A) Every other one is given a positive sign.
B) They are excluded from the analysis.
C) They are all averaged, and if the average is greater than the median a positive sign is assigned to them.
D) In a continuous distribution, the probability of a value being exactly equal to the median is zero, so this will never occur.
A) Every other one is given a positive sign.
B) They are excluded from the analysis.
C) They are all averaged, and if the average is greater than the median a positive sign is assigned to them.
D) In a continuous distribution, the probability of a value being exactly equal to the median is zero, so this will never occur.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
14
The Wilcoxon rank-sum test is an appropriate test when:
A) comparing two population proportions, assuming the underlying distributions are normal.
B) comparing two population medians, assuming both populations are mound-shaped symmetric.
C) comparing two population medians when the underlying distributions are non-normal or when the data are ranks.
D) testing whether the median value from a single population is different from an assumed value when the distribution is normal.
A) comparing two population proportions, assuming the underlying distributions are normal.
B) comparing two population medians, assuming both populations are mound-shaped symmetric.
C) comparing two population medians when the underlying distributions are non-normal or when the data are ranks.
D) testing whether the median value from a single population is different from an assumed value when the distribution is normal.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
15
Consider the following statements: 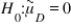
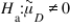
Where The null hypothesis can be interpreted as:
The null hypothesis can be interpreted as:
A) population median 1 is larger than population median 2.
B) population median 1 is different than population median 2.
C) population median 1 is smaller than population median 2.
D) population median 1 is the same as population median 2.
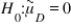
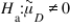
Where
 The null hypothesis can be interpreted as:
The null hypothesis can be interpreted as:A) population median 1 is larger than population median 2.
B) population median 1 is different than population median 2.
C) population median 1 is smaller than population median 2.
D) population median 1 is the same as population median 2.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
16
In determining the Spearman's rank correlation coefficient, what is done if multiple observations within either variable have the same value?
A) As the data are assumed to be from continuous distributions, this will never happen.
B) The observations are ignored and the sample size adjusted accordingly.
C) The observations are given the mean rank of their positions in the ordered list.
D) The observations are arbitrarily assigned a rank in sequence.
A) As the data are assumed to be from continuous distributions, this will never happen.
B) The observations are ignored and the sample size adjusted accordingly.
C) The observations are given the mean rank of their positions in the ordered list.
D) The observations are arbitrarily assigned a rank in sequence.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
17
The Wilcoxon signed-rank test:
A) is concerned with the analysis of a single population.
B) cannot be used with ordinal data.
C) makes use of the sign and magnitude of the rank of the differences between pairs of measurements.
D) makes use of the sign, but not the magnitude, of the differences between pairs of measurements.
A) is concerned with the analysis of a single population.
B) cannot be used with ordinal data.
C) makes use of the sign and magnitude of the rank of the differences between pairs of measurements.
D) makes use of the sign, but not the magnitude, of the differences between pairs of measurements.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
18
For the Kruskal-Wallis test, if the decision from the test is "reject H₀," then:
A) none of the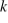 populations under consideration is different (i.e., the samples are from identical populations).
populations under consideration is different (i.e., the samples are from identical populations).
B) at least two of the populations under consideration are different.
C) at least two of the population means are different, whereas the other characteristics are assumed to be the same.
D) there is no need for further analysis, as this test fully describes the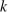 populations.
populations.
A) none of the
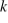 populations under consideration is different (i.e., the samples are from identical populations).
populations under consideration is different (i.e., the samples are from identical populations).B) at least two of the populations under consideration are different.
C) at least two of the population means are different, whereas the other characteristics are assumed to be the same.
D) there is no need for further analysis, as this test fully describes the
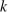 populations.
populations.
Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
19
The _________________ test is appropriate for testing whether a sequence of observations is not random.
A) random
B) runs
C) Mann-Whitney
D) Kruskal-Wallis
A) random
B) runs
C) Mann-Whitney
D) Kruskal-Wallis

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
20
Perform a Wilcoxon rank-sum test on the two independent samples shown here to see if it supports a conclusion that median 1 is larger than median 2.Select the correct critical value (CV), test statistic (TS), and decision.(Use α = 0.01.)  .
.
A) CV = 54, TS = 39, reject H₀
B) CV = 24, TS = 22, fail to reject H₀
C) CV = 54, TS = 39, fail to reject H₀
D) CV = 24, TS = 29, reject H₀
 .
.A) CV = 54, TS = 39, reject H₀
B) CV = 24, TS = 22, fail to reject H₀
C) CV = 54, TS = 39, fail to reject H₀
D) CV = 24, TS = 29, reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
21
A normal approximation for the Wilcoxon rank-sum test is appropriate when:
A)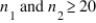 .
.
B)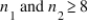 .
.
C)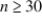 .
.
D)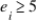 for all categories.
for all categories.
A)
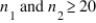 .
.B)
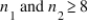 .
.C)
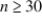 .
.D)
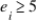 for all categories.
for all categories.
Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
22
The runs test is a nonparametric procedure for testing:
A) the amount of skewness in a population.
B) whether a population is normally distributed.
C) whether data are ordered.
D) whether data are from a random process.
A) the amount of skewness in a population.
B) whether a population is normally distributed.
C) whether data are ordered.
D) whether data are from a random process.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
23
What is the nearest critical value for a Wilcoxon sign-ranked test with 15 nonzero differences, Ha: 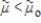 , and α = 0.05?
, and α = 0.05?
A) 30
B) 90
C) 25
D) 95
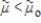 , and α = 0.05?
, and α = 0.05?A) 30
B) 90
C) 25
D) 95

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
24
A normal approximation can be used in the Wilcoxon signed-rank test if:
A)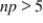 .
.
B)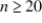 .
.
C)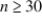 .
.
D)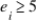 for all categories.
for all categories.
A)
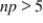 .
.B)
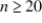 .
.C)
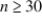 .
.D)
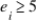 for all categories.
for all categories.
Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
25
A manufacturer of ballpoint pens wishes to know if a machine designed to fill each individual pen with ink is operating properly.The median amount of ink should be 0.05 oz.Suppose 58 pens are randomly sampled from this particular machine, and none of the pens contains exactly 0.05 oz.Assuming the amount of ink in each pen is symmetrically distributed, conduct a Wilcoxon signed-rank test to see if the median amount of ink differs from 0.05 oz.The sum of positive differences (t+) from the sample is 502.Use a normal approximation and select the correct p-value and decision.(Use α = 0.05.)
A) p = 0.0183, reject H₀
B) p = 0.9817, fail to reject H₀
C) p = 0.0049, reject H₀
D) p = 0.1195, fail to reject H₀
A) p = 0.0183, reject H₀
B) p = 0.9817, fail to reject H₀
C) p = 0.0049, reject H₀
D) p = 0.1195, fail to reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
26
Researchers wish to test the effectiveness of a drug designed to treat depression.Seven clinically depressed patients are given a standardized depression test before taking the drug and are evaluated 4 weeks later using the same test.It is known that the scores from this particular test are not normally distributed.The scores are shown here. 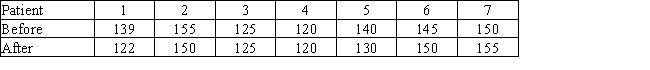
Use a sign test to conclude whether the drug can be associated with a median decrease in depression level.Choose the correct p-value and conclusion.(Use α = 0.05.)
A) p = 0.5000; there appears to be no median decrease in depression.
B) p = 0.8125; there appears to be no median decrease in depression.
C) p = 0.1875; there appears to be no median decrease in depression.
D) p = 0.1875; there appears to be a median decrease in depression.

Use a sign test to conclude whether the drug can be associated with a median decrease in depression level.Choose the correct p-value and conclusion.(Use α = 0.05.)
A) p = 0.5000; there appears to be no median decrease in depression.
B) p = 0.8125; there appears to be no median decrease in depression.
C) p = 0.1875; there appears to be no median decrease in depression.
D) p = 0.1875; there appears to be a median decrease in depression.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
27
A recent survey of practicing Jews, Christians, and Muslims asked these participants' their opinion concerning prayer in public schools.The possible responses were "Strongly For," "No Opinion," or "Strongly Against." The survey also asked several additional questions, which were used to create a continuous "religiosity" variable designed to indicate the amount of influence an individual's religion has on his or her daily life. 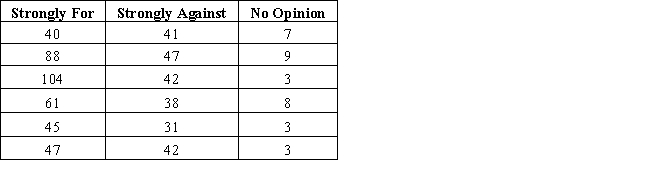
Conduct a Kruskal-Wallis test to see if there is a significant difference in the religiosity of the response groups.Use a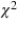 approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.01.)
approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.01.)
A) CV = 9.2103, TS = 13.20, reject H₀
B) CV = 9.2103, TS = 12.22, reject H₀
C) CV = 11.3449, TS = 13.20, reject H₀
D) CV = 10.5966, TS = 9.22, fail to reject H₀

Conduct a Kruskal-Wallis test to see if there is a significant difference in the religiosity of the response groups.Use a
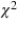 approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.01.)
approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.01.)A) CV = 9.2103, TS = 13.20, reject H₀
B) CV = 9.2103, TS = 12.22, reject H₀
C) CV = 11.3449, TS = 13.20, reject H₀
D) CV = 10.5966, TS = 9.22, fail to reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
28
Calculate the Spearman's rank correlation coefficient for the following data: 
A) -0.560
B) 0.520
C) 0.531
D) 0.588

A) -0.560
B) 0.520
C) 0.531
D) 0.588

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
29
A runs test was performed on 200 measurements of beam deflections and resulted in a test statistic value of 2.486.Using a 5% significance level, what would you conclude about whether the sequence was produced in a random manner?
A) 2.486 > 1.96, so reject the null hypothesis and conclude the sequence of observations is not random.
B) 2.4868 > 1.96, so do not reject the null hypothesis and conclude the sequence of observations may well be random.
C) 2.486 < 2.575, so reject the null hypothesis and conclude the sequence of observations is not random.
D) 2.486 < 2.575, so do not reject the null hypothesis and conclude the sequence of observations may well be random.
A) 2.486 > 1.96, so reject the null hypothesis and conclude the sequence of observations is not random.
B) 2.4868 > 1.96, so do not reject the null hypothesis and conclude the sequence of observations may well be random.
C) 2.486 < 2.575, so reject the null hypothesis and conclude the sequence of observations is not random.
D) 2.486 < 2.575, so do not reject the null hypothesis and conclude the sequence of observations may well be random.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
30
In the Wilcoxon signed-rank test, if two or more measurements have the same nonzero difference, then:
A) the scores are removed from the analysis.
B) the scores are assigned the lowest rank of the tied values.
C) the scores are assigned the highest rank of the tied values.
D) the scores are assigned the mean of the ranks of the tied values.
A) the scores are removed from the analysis.
B) the scores are assigned the lowest rank of the tied values.
C) the scores are assigned the highest rank of the tied values.
D) the scores are assigned the mean of the ranks of the tied values.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
31
Nonparametric tests can be appropriate for quantitative data when:
A) one or more of the assumptions underlying a particular parametric statistical test has been violated.
B) the sample size is very large.
C) the underlying population can be assumed to be normally distributed.
D) all of the assumptions for a particular parametric statistical test have been met.
A) one or more of the assumptions underlying a particular parametric statistical test has been violated.
B) the sample size is very large.
C) the underlying population can be assumed to be normally distributed.
D) all of the assumptions for a particular parametric statistical test have been met.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
32
Conduct a sign test on the following sample data to see if the median differs from 122. 
Choose the correct p-value and conclusion.(Use α = 0.10).
A) p = 0.0592; there is sufficient statistical evidence to reject the null hypothesis and state that the median appears to be different than 122.
B) p = 0.3018; there is insufficient evidence to state that the median differs from 122.
C) p = 0.1509; there is sufficient statistical evidence to reject the null hypothesis and state that the median appears to be different than 122.
D) p = 0.8491; there is insufficient evidence to reject the null hypothesis and state that the median differs from 122.

Choose the correct p-value and conclusion.(Use α = 0.10).
A) p = 0.0592; there is sufficient statistical evidence to reject the null hypothesis and state that the median appears to be different than 122.
B) p = 0.3018; there is insufficient evidence to state that the median differs from 122.
C) p = 0.1509; there is sufficient statistical evidence to reject the null hypothesis and state that the median appears to be different than 122.
D) p = 0.8491; there is insufficient evidence to reject the null hypothesis and state that the median differs from 122.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
33
The Kruskal-Wallis test is the nonparametric version of the:
A) t test on two proportions.
B) ANOVA F test.
C) multiple regression t test for a significant predictor.
D) paired t test.
A) t test on two proportions.
B) ANOVA F test.
C) multiple regression t test for a significant predictor.
D) paired t test.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
34
If the underlying distributions are known to be symmetric, we can use the Wilcoxon rank-sum test to compare two independent population means.This is so because:
A) the rank-sum test is designed as a test of two independent means.
B) the mean and the median have the same value for all symmetric distributions.
C) the mean is the parameter that is usually evaluated when using nonparametric procedures.
D) the difference in two population means will always be normally distributed and the Wilcoxon rank-sum test is used to test normally distributed variables.
A) the rank-sum test is designed as a test of two independent means.
B) the mean and the median have the same value for all symmetric distributions.
C) the mean is the parameter that is usually evaluated when using nonparametric procedures.
D) the difference in two population means will always be normally distributed and the Wilcoxon rank-sum test is used to test normally distributed variables.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
35
With the Wilcoxon sign-ranked test, what is done in the case of equal values?
A) As the data are from a continuous distribution, this will never happen.
B) The observations are assigned the mean rank for their positions in the ordered list.
C) The observations are thrown out and not considered in the analysis.
D) The equal values are arbitrarily assigned an appropriate rank in sequence.
A) As the data are from a continuous distribution, this will never happen.
B) The observations are assigned the mean rank for their positions in the ordered list.
C) The observations are thrown out and not considered in the analysis.
D) The equal values are arbitrarily assigned an appropriate rank in sequence.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
36
A corporation wishes to evaluate the effectiveness of a new employee training program.Workers on two independent assembly lines are monitored, and the amount of time (in minutes) to complete a standardized assembly procedure is recorded for each.The distribution of assembly times is known to be heavily left-skewed.Based on the given sample information, does it appear that the training program is associated with a reduced median assembly time? Use the Wilcoxon rank-sum test to make your decision.Select the correct critical value (CV), test statistic (TS), and decision.(Use α = 0.05.) 
A) CV = 41, TS = 57, fail to reject H₀
B) CV = 41, TS = 28, reject H₀
C) CV = 71, TS = 57, fail to reject H₀
D) CV = 71, TS = 82, reject H₀

A) CV = 41, TS = 57, fail to reject H₀
B) CV = 41, TS = 28, reject H₀
C) CV = 71, TS = 57, fail to reject H₀
D) CV = 71, TS = 82, reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
37
An athletic trainer is concerned that his athletes are not drinking enough water during games.He randomly samples 11 previous games with comparable temperature and humidity and records the amount of water consumed by the team in liters (shown in the table).Conduct a sign test to see if the median amount of water consumed exceeds 30 L.(Assume that the amount of water consumed per game is a continuous variable.)
Amount of Water Consumed per Game (in liters)
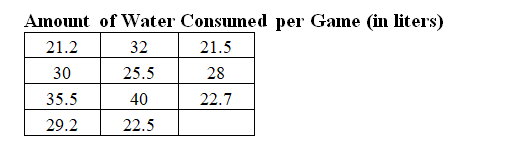
Select the correct critical value (CV), test statistic (TS), and decision.(Use α = 0.10.)
A) CV = 7, TS = 3, fail to reject H₀
B) CV = 8, TS = 4, fail to reject H₀
C) CV = 8, TS = 3, fail to reject H₀
D) CV = 7, TS = 4, fail to reject H₀
Amount of Water Consumed per Game (in liters)

Select the correct critical value (CV), test statistic (TS), and decision.(Use α = 0.10.)
A) CV = 7, TS = 3, fail to reject H₀
B) CV = 8, TS = 4, fail to reject H₀
C) CV = 8, TS = 3, fail to reject H₀
D) CV = 7, TS = 4, fail to reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
38
Which of the following tests uses matched-pairs sampling?
A) Wilcoxon signed-rank test
B) Wilcoxon rank-sum test
C) Mann-Whitney test
D) Kruskal-Wallis test
A) Wilcoxon signed-rank test
B) Wilcoxon rank-sum test
C) Mann-Whitney test
D) Kruskal-Wallis test

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
39
With the Kruskal-Wallis test, the data from all k samples are combined and each observation is assigned a rank.What is done in the case of equal values?
A) As the data are all from continuous distributions, this will never happen.
B) The observations are assigned the mean rank for their positions in the ordered list.
C) The observations are thrown out and not considered in the analysis.
D) The equal values are arbitrarily assigned an appropriate rank in sequence.
A) As the data are all from continuous distributions, this will never happen.
B) The observations are assigned the mean rank for their positions in the ordered list.
C) The observations are thrown out and not considered in the analysis.
D) The equal values are arbitrarily assigned an appropriate rank in sequence.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
40
Two variables have a Spearman's rank correlation coefficient of -0.9872.We can conclude from this that:
A) the two variables are strongly correlated.
B) there is a causal connection between the two variables.
C) the two variables are not correlated at all.
D) the two samples are random.
A) the two variables are strongly correlated.
B) there is a causal connection between the two variables.
C) the two variables are not correlated at all.
D) the two samples are random.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
41
When comparing the output of strawberries grown on plots using fertilizer A with that grown on otherwise identical plots using fertilizer B, making a general assessment of relative fertilizer effectiveness may well call for a:
A) sign test.
B) Kruskal-Wallis test.
C) Wilcoxon signed-rank test for paired samples.
D) Wilcoxon rank-sum test.
A) sign test.
B) Kruskal-Wallis test.
C) Wilcoxon signed-rank test for paired samples.
D) Wilcoxon rank-sum test.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
42
A meteorologist has measured the amount of rain in four cities for the last six months.She wants to know if there are different amounts of rain in the four cities.The table shows the data. 
Conduct a Kruskal-Wallis test to see if there is a significant difference in the amount of rain in the four cities.Use a χ2 approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.025.)
A) CV = 7.3778, TS = 14.04, reject H₀
B) CV = 9.2103, TS = 15.98, reject H₀
C) CV = 9.3484, TS = 15.98, reject H₀
D) CV = 9.3484, TS = 9.22, fail to reject H₀

Conduct a Kruskal-Wallis test to see if there is a significant difference in the amount of rain in the four cities.Use a χ2 approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.025.)
A) CV = 7.3778, TS = 14.04, reject H₀
B) CV = 9.2103, TS = 15.98, reject H₀
C) CV = 9.3484, TS = 15.98, reject H₀
D) CV = 9.3484, TS = 9.22, fail to reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
43
When performing a Wilcoxon rank-sum test on the two independent samples shown here at α = 0.01, what is the alternative hypothesis?  .
.
A) The samples have statistically different means.
B) The samples have the same median.
C) The samples have statistically different medians.
D) The samples have the same mean.
 .
.A) The samples have statistically different means.
B) The samples have the same median.
C) The samples have statistically different medians.
D) The samples have the same mean.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
44
The total number of words per page is recorded from a random sample of 17 pages printed using a standard font and from 20 pages using a new font.A Wilcoxon rank-sum test is performed, and the sum of the ranks of the standard font sample (sample 1) is 301 .Using a normal approximation, does the new font appear to increase the median number of words per page? Assume the number of words per page can be modeled as a continuous variable.Select the correct p-value and decision.(Use α = 0.05.)
A) p = 0.9920, fail to reject H₀
B) p = 0.0080, reject H₀
C) p = 0.2514, fail to reject H₀
D) p = 0.7486, fail to reject H₀
A) p = 0.9920, fail to reject H₀
B) p = 0.0080, reject H₀
C) p = 0.2514, fail to reject H₀
D) p = 0.7486, fail to reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
45
Suppose a pair of dice is rolled 50 times to determine if "doubles" occur randomly.The following results were obtained (N = not a double, Y = double): N N Y N Y Y N N N N N N Y N Y N N N N N N Y N N N N N N Y N Y N N Y N N N N N Y N N N N N N N N N N
How many runs are there in these data?
A) 10
B) 17
C) 19
D) 40
How many runs are there in these data?
A) 10
B) 17
C) 19
D) 40

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
46
A Kruskal-Wallis test is always:
A) used with only one sample.
B) used with exactly two samples.
C) a test that rejects H₀ for large values of the test statistic.
D) a test that rejects H₀ for very large or very small values of the test statistic.
A) used with only one sample.
B) used with exactly two samples.
C) a test that rejects H₀ for large values of the test statistic.
D) a test that rejects H₀ for very large or very small values of the test statistic.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
47
Which of the following statements about Spearman's rank correlation coefficient is false?
A) It is not exact when there are tied observations.
B) It indicates the correlation between the ranks of two variables.
C) It can take on only values between -1 and +1.
D) It can take on only values between 0 and + 1.
A) It is not exact when there are tied observations.
B) It indicates the correlation between the ranks of two variables.
C) It can take on only values between -1 and +1.
D) It can take on only values between 0 and + 1.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
48
Calculate the Spearman's rank correlation coefficient for the following rankings by x and y: 
A) 0.30
B) 0.70
C) 0.75
D) 1.00

A) 0.30
B) 0.70
C) 0.75
D) 1.00

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
49
A manufacturer has three machine lines (Red, White, and Blue) that produce the same product.The product can be scrutinized for eccentricity based on an evaluation process that returns a raw score indicating the product's departure from the standard (a higher score indicates more eccentricity).Six products are chosen at random from each line. 
Conduct a Kruskal-Wallis test to see if the production lines appear to differ.Use a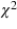 approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.05.)
approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.05.)
A) CV = 6.47, TS = 5.9915, fail to reject H₀
B) CV = 27.5871, TS = 10.56, fail to reject H₀
C) CV = 7.8147, TS = 3.22, reject H₀
D) CV = 5.9915, TS = 6.47, reject H₀

Conduct a Kruskal-Wallis test to see if the production lines appear to differ.Use a
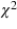 approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.05.)
approximation.Select the appropriate critical value (CV), test statistic (TS), and decision.(Use α = 0.05.)A) CV = 6.47, TS = 5.9915, fail to reject H₀
B) CV = 27.5871, TS = 10.56, fail to reject H₀
C) CV = 7.8147, TS = 3.22, reject H₀
D) CV = 5.9915, TS = 6.47, reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
50
Concerning the runs test, a normal approximation is appropriate when:
A) n ≥ 30.
B) the number of observations in both categories is more than 10 each.
C) the number of categories of the variable (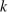 ) is at least 3.
) is at least 3.
D) the expected value of each category is at least 5.
A) n ≥ 30.
B) the number of observations in both categories is more than 10 each.
C) the number of categories of the variable (
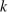 ) is at least 3.
) is at least 3.D) the expected value of each category is at least 5.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
51
Concerning the Kruskal-Wallis test, the test statistic follows a chi-square distribution with 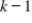 degrees of freedom if H₀ is true and:
degrees of freedom if H₀ is true and:
A)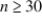 .
.
B)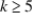 .
.
C)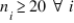 .
.
D) .and
.and 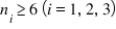
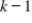 degrees of freedom if H₀ is true and:
degrees of freedom if H₀ is true and:A)
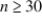 .
.B)
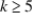 .
.C)
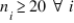 .
.D)
 .and
.and 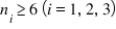

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
52
The first step in a Wilcoxon rank-sum test is to order the data and assign a rank of 1 to the:
A) largest observation.
B) smallest observation.
C) observation that occurs most frequently.
D) observation that occurs least frequently.
A) largest observation.
B) smallest observation.
C) observation that occurs most frequently.
D) observation that occurs least frequently.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
53
A study looked at x = distance of convenience stores from a large city's Contemporary Art Museum, and y = price of a 50-mL bottle of water at the stores.The Spearman's rank correlation coefficient was calculated to be -0.73.What does this tell you about the variables?
A) There is no association between distance from the museum and the price of bottled water.
B) There is an association between these variables: On average, the farther a convenience store is from the museum, the less expensive a bottle of water is at the store.
C) There is an association between these variables: On average, the farther a convenience store is from the museum, the more expensive a bottle of water is at the store.
D) The distance of a convenience store from the museum determines the price of a bottle of water at the store.
A) There is no association between distance from the museum and the price of bottled water.
B) There is an association between these variables: On average, the farther a convenience store is from the museum, the less expensive a bottle of water is at the store.
C) There is an association between these variables: On average, the farther a convenience store is from the museum, the more expensive a bottle of water is at the store.
D) The distance of a convenience store from the museum determines the price of a bottle of water at the store.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
54
Which of the following statements is true?
A) Unlike parametric methods, nonparametric methods cannot be applied to nominal data that lack numeric values.
B) Nonparametric methods can be applied to a wider variety of problems because they have more rigid requirements compared to parametric methods.
C) Nonparametric methods can be applied to a wider variety of problems because they have less rigid requirements compared to parametric methods.
D) Nonparametric methods can be applied to a wider variety of problems because they have less rigid requirements compared to parametric methods; also, unlike parametric methods, nonparametric methods cannot be applied to nominal data that lack numeric values.
A) Unlike parametric methods, nonparametric methods cannot be applied to nominal data that lack numeric values.
B) Nonparametric methods can be applied to a wider variety of problems because they have more rigid requirements compared to parametric methods.
C) Nonparametric methods can be applied to a wider variety of problems because they have less rigid requirements compared to parametric methods.
D) Nonparametric methods can be applied to a wider variety of problems because they have less rigid requirements compared to parametric methods; also, unlike parametric methods, nonparametric methods cannot be applied to nominal data that lack numeric values.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
55
As used in the runs test, a run is:
A) a series, or subsequence, of one or more identical observations.
B) a series, or subsequence, of two or more identical observations.
C) a series, or subsequence, of one or more unusual observations.
D) a series, or subsequence, of two or more unusual observations.
A) a series, or subsequence, of one or more identical observations.
B) a series, or subsequence, of two or more identical observations.
C) a series, or subsequence, of one or more unusual observations.
D) a series, or subsequence, of two or more unusual observations.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
56
For the Kruskal-Wallis test, the test statistic follows a chi-square distribution with 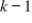 degrees of freedom if H₀ is true and:
degrees of freedom if H₀ is true and:
A)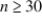 .
.
B)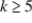 .
.
C)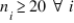 .
.
D)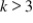 .and
.and 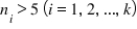
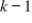 degrees of freedom if H₀ is true and:
degrees of freedom if H₀ is true and:A)
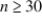 .
.B)
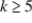 .
.C)
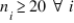 .
.D)
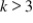 .and
.and 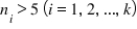

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
57
The smallest possible number of runs in any sample is:
A) 0.
B) 1.
C) dependent on the number of samples.
D) dependent on the number of observations in each category.
A) 0.
B) 1.
C) dependent on the number of samples.
D) dependent on the number of observations in each category.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
58
The runs test is a nonparametric test.This means that:
A) the underlying population is assumed to be normal.
B) the underlying population is assumed to be nonskewed.
C) there are no assumptions made concerning the underlying population.
D) the underlying population is assumed to be mound-shaped symmetric.
A) the underlying population is assumed to be normal.
B) the underlying population is assumed to be nonskewed.
C) there are no assumptions made concerning the underlying population.
D) the underlying population is assumed to be mound-shaped symmetric.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
59
In general, a primary reason for choosing a nonparametric procedure over a parametric one is:
A) nonparametric procedures do not require parameters that limit the scope of an experiment.
B) nonparametric procedures generally require fewer assumptions than parametric procedures.
C) parametric procedures make inferences on parameters, whereas nonparametric procedures do not.
D) the assumption of normality, which most parametric procedures require, is rarely valid.
A) nonparametric procedures do not require parameters that limit the scope of an experiment.
B) nonparametric procedures generally require fewer assumptions than parametric procedures.
C) parametric procedures make inferences on parameters, whereas nonparametric procedures do not.
D) the assumption of normality, which most parametric procedures require, is rarely valid.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
60
If 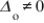 , then the value
, then the value 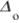 is subtracted from each observation in sample 1.This will:
is subtracted from each observation in sample 1.This will:
A) "shift" sample 1, effectively making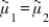 if H₀ is true.
if H₀ is true.
B) correct for non-normality of both populations.
C) effectively do nothing, because subtracting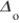 from the values will not change the median.
from the values will not change the median.
D) help smooth out discontinuities that occur when attempting to perform the test on discrete data.
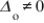 , then the value
, then the value 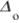 is subtracted from each observation in sample 1.This will:
is subtracted from each observation in sample 1.This will:A) "shift" sample 1, effectively making
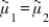 if H₀ is true.
if H₀ is true.B) correct for non-normality of both populations.
C) effectively do nothing, because subtracting
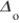 from the values will not change the median.
from the values will not change the median.D) help smooth out discontinuities that occur when attempting to perform the test on discrete data.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
61
What is the primary difference between parametric and nonparametric tests of significance?
A) Nonparametric tests are involved with populations that have no parameters.
B) Nonparametric tests involve only interval data, whereas parametric tests involve only ordinal data.
C) Nonparametric tests do not have hypotheses based on population parameters or assumptions based on the distributions of populations from which the samples are drawn.
D) Nonparametric tests do not make any distributional assumptions throughout the entire process.
A) Nonparametric tests are involved with populations that have no parameters.
B) Nonparametric tests involve only interval data, whereas parametric tests involve only ordinal data.
C) Nonparametric tests do not have hypotheses based on population parameters or assumptions based on the distributions of populations from which the samples are drawn.
D) Nonparametric tests do not make any distributional assumptions throughout the entire process.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
62
Suppose you have data that you wish to use for the Wilcoxon rank-sum test.The 12th, 13th, and 14th values in your ordered data are all $50.The 11th value is less than $50, and the 15th value is more than $50.What are the appropriate ranks for the three $50 values?
A) 12, 13, 14
B) 12, 12, 12
C) 13, 13, 13
D) 14, 14, 14
A) 12, 13, 14
B) 12, 12, 12
C) 13, 13, 13
D) 14, 14, 14

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
63
With the Wilcoxon rank-sum test, what is done in the case of equal values?
A) As the data are from a continuous distribution, this will never happen.
B) The observations are assigned the mean rank for their positions in the ordered list.
C) The observations are thrown out and not considered in the analysis.
D) The equal values are arbitrarily assigned an appropriate rank in sequence.
A) As the data are from a continuous distribution, this will never happen.
B) The observations are assigned the mean rank for their positions in the ordered list.
C) The observations are thrown out and not considered in the analysis.
D) The equal values are arbitrarily assigned an appropriate rank in sequence.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
64
In the Wilcoxon rank-sum test, the null hypothesis may be expressed as: 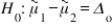
If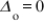 , then:
, then:
A) the test is not valid.
B) we can conclude that the null hypothesis is true and there is no difference in the two population medians only if the sample provides sufficient support for this assertion.
C) there is insufficient evidence to reject the null hypothesis, but nothing is said about the validity of the null hypothesis.
D) the median of population 1 is equal to the mean of population 2, provided both populations are heavily skewed.
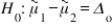
If
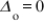 , then:
, then:A) the test is not valid.
B) we can conclude that the null hypothesis is true and there is no difference in the two population medians only if the sample provides sufficient support for this assertion.
C) there is insufficient evidence to reject the null hypothesis, but nothing is said about the validity of the null hypothesis.
D) the median of population 1 is equal to the mean of population 2, provided both populations are heavily skewed.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
65
The first 10 final exams turned in for a particular class are classified as either pass (earned a grade of "C" or higher) or fail (earned a grade lower than "C").The sequence of exam submission and the results are shown here: 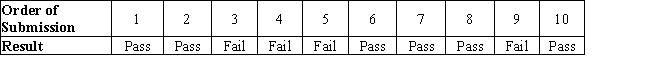
Use the runs test with α = 0.05 to determine whether there is any evidence that the order in which the exams were submitted was not random.Select the appropriate critical values (CV), test statistic (TS), and decision.
A) CV = 3 and 9, TS = 5, fail to reject H₀
B) CV = 3 and 8, TS = 5, fail to reject H₀
C) CV = 3 and 9, TS = 3, reject H₀
D) CV = 2 and 8, TS = 2, reject H₀

Use the runs test with α = 0.05 to determine whether there is any evidence that the order in which the exams were submitted was not random.Select the appropriate critical values (CV), test statistic (TS), and decision.
A) CV = 3 and 9, TS = 5, fail to reject H₀
B) CV = 3 and 8, TS = 5, fail to reject H₀
C) CV = 3 and 9, TS = 3, reject H₀
D) CV = 2 and 8, TS = 2, reject H₀

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck
66
The Spearman's rank correlation coefficient is a nonparametric indicator of:
A) the similarity between two independent samples.
B) the correlation between the ranks of two variables.
C) any relationship between the ranks of two or more variables.
D) departure from normality.
A) the similarity between two independent samples.
B) the correlation between the ranks of two variables.
C) any relationship between the ranks of two or more variables.
D) departure from normality.

Unlock Deck
Unlock for access to all 66 flashcards in this deck.
Unlock Deck
k this deck



