Deck 8: Sampling Distributions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/50
Play
Full screen (f)
Deck 8: Sampling Distributions
1
What effect does increasing the sample size have on the sampling distribution of 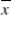 ?
?
A) The mean of the distribution gets smaller.
B) Sample size has no effect on the sampling distribution of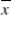 .
.
C) The variability of the sampling distribution gets smaller.
D) The mean of the distribution gets larger.
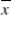 ?
?A) The mean of the distribution gets smaller.
B) Sample size has no effect on the sampling distribution of
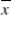 .
.C) The variability of the sampling distribution gets smaller.
D) The mean of the distribution gets larger.
The variability of the sampling distribution gets smaller.
2
A department store claims that its average customer satisfaction rating is 28.5.We believe that this rating is too high, so we randomly sample 40 customers and find a sample average of 25.2.If the standard deviation of customer satisfaction for this store is 13.1, what is the probability of observing a sample at least this far below the claimed mean, assuming the claim is true?
A) 0.9441
B) 0.4013
C) 0.0559
D) 0.2500
A) 0.9441
B) 0.4013
C) 0.0559
D) 0.2500
0.0559
3
In the natural world, the number of measurable variables that follow a normal distribution is quite remarkable.Many of these measurable variables are actually the sum of other independent variables.The Central Limit Theorem helps explain this phenomenon because:
A) the Central Limit Theorem states that all measurable variables follow a normal distribution.
B) the Central Limit Theorem states that for a sufficiently large number of variables, the sum of these variables will be approximately normally distributed.
C) the Central Limit Theorem states that for large sample sizes, all measurable variables will follow a normal distribution.
D) the Central Limit Theorem states that because nature is normative, variables found in nature will be at least approximately normal.
A) the Central Limit Theorem states that all measurable variables follow a normal distribution.
B) the Central Limit Theorem states that for a sufficiently large number of variables, the sum of these variables will be approximately normally distributed.
C) the Central Limit Theorem states that for large sample sizes, all measurable variables will follow a normal distribution.
D) the Central Limit Theorem states that because nature is normative, variables found in nature will be at least approximately normal.
the Central Limit Theorem states that for a sufficiently large number of variables, the sum of these variables will be approximately normally distributed.
4
A simple random sample (SRS) of size n is best defined as:
A) a sample selected in such a way that every possible sample of size n will be selected.
B) a sample selected in such a way that every possible sample of size n has the same chance of being selected.
C) a sample selected in such a way that every possible sample of size n has a randomly assigned chance of being selected.
D) a sample selected in such a way that every possible sample of size n has some chance of being selected.
A) a sample selected in such a way that every possible sample of size n will be selected.
B) a sample selected in such a way that every possible sample of size n has the same chance of being selected.
C) a sample selected in such a way that every possible sample of size n has a randomly assigned chance of being selected.
D) a sample selected in such a way that every possible sample of size n has some chance of being selected.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
5
Suppose you are interested in determining the average word length in Lincoln's Gettysburg Address.You decide to estimate this average word length by using a sample of 10 randomly selected words.Which of the following methods should result in a simple random sample of 10 words from the Address?
A) Have a student select 10 "representative" words from a copy of the Address.
B) Obtain a copy of the Address on a page of paper.Close your eyes and point the tip of your pencil on the page five times, each time selecting the word that your pencil lands on or nearest.
C) Obtain a list of the 268 words in the Address.Number the words.Use a random number generator to determine which 10 words to select.
D) Select every 27th word in the Address.
A) Have a student select 10 "representative" words from a copy of the Address.
B) Obtain a copy of the Address on a page of paper.Close your eyes and point the tip of your pencil on the page five times, each time selecting the word that your pencil lands on or nearest.
C) Obtain a list of the 268 words in the Address.Number the words.Use a random number generator to determine which 10 words to select.
D) Select every 27th word in the Address.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
6
If the monthly rents of all one-bedroom apartments in a small town are known to be normally distributed with a mean of $175 and a standard deviation of $35, which of the following would be the least likely individual rent that you might expect to find?
A) $135
B) $140
C) $210
D) $225
A) $135
B) $140
C) $210
D) $225

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
7
Let X be the fuel efficiency, in miles per gallon, of a particular vehicle. Suppose X ~ N(μ = 18.5, σ = 2.2).Find P(18.2 ≤ 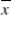 ≤ 19) if n = 5.
≤ 19) if n = 5.
A) 0.6950
B) 0.9839
C) 0.1467
D) 0.3129
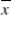 ≤ 19) if n = 5.
≤ 19) if n = 5.A) 0.6950
B) 0.9839
C) 0.1467
D) 0.3129

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
8
The ______________ of a statistic is the probability distribution of the statistic.
A) limiting distribution
B) exponential distribution
C) normal distribution
D) sampling distribution
A) limiting distribution
B) exponential distribution
C) normal distribution
D) sampling distribution

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
9
Homeowners who recently bought their houses from a local developer allege that 30% of the houses this developer constructs have some major defect that will require substantial repairs.To test this allegation, we randomly sample 20 houses constructed by the developer and find that two of the homes do, indeed, have some major defect.If the allegation is correct, what is the probability of observing at most two defective homes out of a random sample of 20?
A) 0.0256
B) 0.1000
C) 0.0300
D) 0.9744
A) 0.0256
B) 0.1000
C) 0.0300
D) 0.9744

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
10
An industrial fan is designed to move 2500 ft3of air per minute, on average.Under normal operating conditions, the standard deviation of air volume moved by this fan is 300 ft3/min.If the fan is operating as designed, what is the probability that the average of 30 randomly selected measurements of fan capacity will be less than 2000 ft3/min?
A) 0.0475
B) 0.0000
C) 0.9525
D) 0.913
A) 0.0475
B) 0.0000
C) 0.9525
D) 0.913

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
11
Consider the following discrete population distribution: 
We randomly draw samples of size 5 from this population using the simple random sampling technique.If we know that μx = 5.4, σx= 2.069, what are the mean and the standard deviation of the sampling distribution of sample means? Assume the Central Limit Theorem applies.
A) μ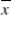 = 5.4, σ
= 5.4, σ 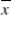 = 2.069
= 2.069
B) μ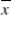 = 1.08, σ
= 1.08, σ 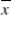 = 0.4138
= 0.4138
C) μ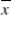 = 5.4, σ
= 5.4, σ 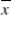 = 0.9253
= 0.9253
D) μ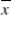 = 5.4, σ
= 5.4, σ 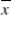 = 0.4138
= 0.4138

We randomly draw samples of size 5 from this population using the simple random sampling technique.If we know that μx = 5.4, σx= 2.069, what are the mean and the standard deviation of the sampling distribution of sample means? Assume the Central Limit Theorem applies.
A) μ
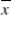 = 5.4, σ
= 5.4, σ 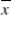 = 2.069
= 2.069B) μ
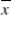 = 1.08, σ
= 1.08, σ 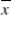 = 0.4138
= 0.4138C) μ
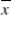 = 5.4, σ
= 5.4, σ 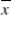 = 0.9253
= 0.9253D) μ
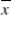 = 5.4, σ
= 5.4, σ 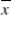 = 0.4138
= 0.4138
Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
12
A psychologist randomly samples 28 clinically depressed patients.She subjects each patient to a standardized battery of tests.The average battery test score for the sample is 128.In this example, the number 128 is a:
A) parameter.
B) sampling distribution.
C) statistic.
D) random variable.
A) parameter.
B) sampling distribution.
C) statistic.
D) random variable.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
13
A _________ is any quantity computed from values in a sample.
A) sampling distribution
B) parameter
C) statistic
D) parametric statistic
A) sampling distribution
B) parameter
C) statistic
D) parametric statistic

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
14
Concerning the sampling distribution of 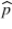 , what is the significance of the conditions np ≥ 5 and n(1 - p) ≥ 5?
, what is the significance of the conditions np ≥ 5 and n(1 - p) ≥ 5?
A) It is impossible to make any inference on p using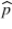 unless both these conditions are met.
unless both these conditions are met.
B) The sampling distribution of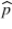 will be approximately normal if both these conditions are met.
will be approximately normal if both these conditions are met.
C) It is possible to use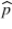 to estimate p only if one of these conditions is met.
to estimate p only if one of these conditions is met.
D) The value of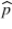 will be very close to p if and only if the sample size is large enough for both these conditions to be met.
will be very close to p if and only if the sample size is large enough for both these conditions to be met.
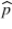 , what is the significance of the conditions np ≥ 5 and n(1 - p) ≥ 5?
, what is the significance of the conditions np ≥ 5 and n(1 - p) ≥ 5?A) It is impossible to make any inference on p using
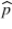 unless both these conditions are met.
unless both these conditions are met.B) The sampling distribution of
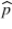 will be approximately normal if both these conditions are met.
will be approximately normal if both these conditions are met.C) It is possible to use
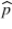 to estimate p only if one of these conditions is met.
to estimate p only if one of these conditions is met.D) The value of
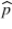 will be very close to p if and only if the sample size is large enough for both these conditions to be met.
will be very close to p if and only if the sample size is large enough for both these conditions to be met.
Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
15
An exponential population has parameter λ = 0.012 (hence, μ= 83.33, σ2= 6944.44).What is the probability that a random sample of 41 from this population will average between 81 and 84?
A) 0.5199
B) 0.0160
C) 0.0000
D) 0.0913
A) 0.5199
B) 0.0160
C) 0.0000
D) 0.0913

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
16
If the sample size is large (n ≥ 30), then, regardless of the population distribution, which of the following summarizes the sampling distribution of random variable 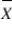 ?
?
A)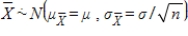
B)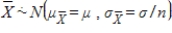
C)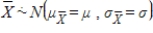
D)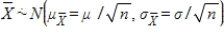
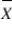 ?
?A)
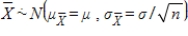
B)
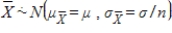
C)
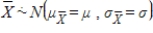
D)
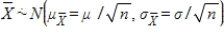

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
17
Because 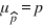
A)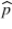 will always be the same as p.
will always be the same as p.
B)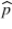 is unbiased for p.
is unbiased for p.
C)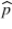 must always be ≤ p.
must always be ≤ p.
D)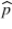 will always be ≥ p.
will always be ≥ p.
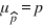
A)
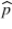 will always be the same as p.
will always be the same as p.B)
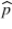 is unbiased for p.
is unbiased for p.C)
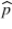 must always be ≤ p.
must always be ≤ p.D)
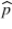 will always be ≥ p.
will always be ≥ p. 
Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
18
A heavily right-skewed population has a mean of 28 and a standard deviation of 2.What is the probability that a random sample of 5 from this population will average less than 27?
A) 0.1314
B) 0.3085
C) 0.6915
D) We cannot answer this question because the sample size is small and the population is non-normal; the distribution of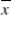 cannot be assumed to be normal.
cannot be assumed to be normal.
A) 0.1314
B) 0.3085
C) 0.6915
D) We cannot answer this question because the sample size is small and the population is non-normal; the distribution of
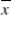 cannot be assumed to be normal.
cannot be assumed to be normal.
Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
19
It is believed that 15% of people who fly on commercial airliners are "very concerned" about the safety of the carrier they have chosen.If this statement is accurate, what is the probability that out of 150 randomly selected people who fly on commercial airliners, between 20 and 25 of them are "very concerned" about the safety of the carrier that they have chosen?
A) 0.1333
B) 0.0333
C) 0.4314
D) 0.7157
A) 0.1333
B) 0.0333
C) 0.4314
D) 0.7157

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
20
If a population has a variance of 64 and we take a simple random sample of size 25, what will the standard deviation of the sampling distribution of 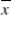 be, assuming the Central Limit Theorem holds in this case?
be, assuming the Central Limit Theorem holds in this case?
A) 0.32
B) 0.625
C) 1.60
D) 3.125
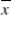 be, assuming the Central Limit Theorem holds in this case?
be, assuming the Central Limit Theorem holds in this case?A) 0.32
B) 0.625
C) 1.60
D) 3.125

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
21
If a population proportion is 0.8 and a sample of size n = 100 is randomly selected from this population, what is the standard deviation of the proportion 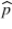 ?
?
A) 0.0258
B) 0.0355
C) 0.0400
D) 0.0538
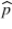 ?
?A) 0.0258
B) 0.0355
C) 0.0400
D) 0.0538

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
22
Heights of children entering kindergarten are normally distributed with a mean of 103 cm and a standard deviation of 1.27 cm.What is the probability that a random sample of 8 children will have an average height more than 103.5 cm?
A) 0.8665
B) 0.6517
C) 0.3483
D) 0.1335
A) 0.8665
B) 0.6517
C) 0.3483
D) 0.1335

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
23
A population of sports teams consists of 26 individual teams.We wish to randomly sample 4 teams for observation.If each team has an equal likelihood of being selected, what is the probability that any given sample of size 4 will be selected?
A) 1/14,950
B) 4/14,950
C) 1/358,800
D) 4/358,800
A) 1/14,950
B) 4/14,950
C) 1/358,800
D) 4/358,800

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
24
For after-hours emergencies, doctors often use a messaging/paging service to allow patients to contact them.If it is known that 30% of the patients who call the messaging service fail to leave a message for the doctor, what is the probability that out of 80 randomly sampled calls, more than 30 will fail to leave a message?
A) 0.0512
B) 0.9279
C) 0.3750
D) 0.0721
A) 0.0512
B) 0.9279
C) 0.3750
D) 0.0721

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
25
In a recent study, it was reported that the proportion of employees who miss work on Fridays is 0.15, and that the standard deviation of the sample proportion is 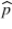 .However, the report did not indicate the sample size n.What was the sample size, assuming the Central Limit Theorem holds in this case?
.However, the report did not indicate the sample size n.What was the sample size, assuming the Central Limit Theorem holds in this case?
A) 26
B) 58
C) 102
D) 204
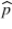 .However, the report did not indicate the sample size n.What was the sample size, assuming the Central Limit Theorem holds in this case?
.However, the report did not indicate the sample size n.What was the sample size, assuming the Central Limit Theorem holds in this case?A) 26
B) 58
C) 102
D) 204

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
26
To better understand the student body, a college administrator takes a census of all students currently enrolled at the college.From the census, he finds that the range of ages at this school is 41 years.In this example, 41 years is a:
A) parameter.
B) statistic.
C) sampling distribution.
D) random variable.
A) parameter.
B) statistic.
C) sampling distribution.
D) random variable.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
27
Which of the following statements is true about the sampling distribution of the sample proportion 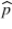 ?
?
A) The mean of the sampling distribution will equal the population proportion p.
B) An increase in the sample size n will result in a decrease in the standard error of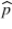 .
.
C) The sampling distribution will be approximately normal, provided that np ≥ 5 and n(1-p) ≥ 5.
D) All of the above are true.
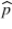 ?
?A) The mean of the sampling distribution will equal the population proportion p.
B) An increase in the sample size n will result in a decrease in the standard error of
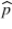 .
.C) The sampling distribution will be approximately normal, provided that np ≥ 5 and n(1-p) ≥ 5.
D) All of the above are true.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
28
Which of the following statements about the Central Limit Theorem is true?
A) The Central Limit Theorem states that the sample mean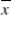 is always equal to the population mean μ.
is always equal to the population mean μ.
B) The Central Limit Theorem states that the sampling distribution of the population mean μ is approximately normal provided that n ≥ 30.
C) The Central Limit Theorem states that the sampling distribution of the sample mean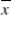 is approximately normal for large sample sizes (n ≥ 30).
is approximately normal for large sample sizes (n ≥ 30).
D) The Central Limit Theorem states that the sample mean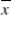 is equal to the population mean μ provided that n ≥ 30.
is equal to the population mean μ provided that n ≥ 30.
A) The Central Limit Theorem states that the sample mean
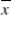 is always equal to the population mean μ.
is always equal to the population mean μ.B) The Central Limit Theorem states that the sampling distribution of the population mean μ is approximately normal provided that n ≥ 30.
C) The Central Limit Theorem states that the sampling distribution of the sample mean
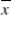 is approximately normal for large sample sizes (n ≥ 30).
is approximately normal for large sample sizes (n ≥ 30).D) The Central Limit Theorem states that the sample mean
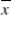 is equal to the population mean μ provided that n ≥ 30.
is equal to the population mean μ provided that n ≥ 30.
Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
29
A math professor once claimed that 90% of students pass a particular class of hers.To check this claim, a random sample of 150 students who had taken this class was obtained.It was found that 129 passed the class.If the professor is correct, what is the probability that 129 or fewer of the students in such a sample would have passed the class?
A) 0.9484
B) 0.0516
C) 0.5516
D) 0.4484
A) 0.9484
B) 0.0516
C) 0.5516
D) 0.4484

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
30
We wish to record the results from two six-sided die rolls.How many unique outcomes are possible?
A) 15
B) 36
C) 30
D) 64
A) 15
B) 36
C) 30
D) 64

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
31
In the normal process of simple random sampling (SRS), samples are almost always drawn without replacement.Why is this a problem for the assumptions involved with a true SRS?
A) Because the samples are drawn without replacement, there will never be enough samples to accurately calculate a meaningful statistic.
B) Because the samples are drawn without replacement, the process is not really random.
C) Because the samples are drawn without replacement, the samples are not truly independent, as the probability of one sample is affected by previous selections.
D) Because the samples are drawn without replacement, this creates complexity, which violates the assumption of simplicity.
A) Because the samples are drawn without replacement, there will never be enough samples to accurately calculate a meaningful statistic.
B) Because the samples are drawn without replacement, the process is not really random.
C) Because the samples are drawn without replacement, the samples are not truly independent, as the probability of one sample is affected by previous selections.
D) Because the samples are drawn without replacement, this creates complexity, which violates the assumption of simplicity.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
32
Consider a large population with a mean of 150 and a standard deviation of 27.A random sample of size 36 is taken from this population.What is the standard error of the sampling distribution of the sample mean?
A) 4.17
B) 4.50
C) 5.20
D) 5.56
A) 4.17
B) 4.50
C) 5.20
D) 5.56

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
33
If the standard error of the sampling distribution of a sample proportion is 0.02049 for samples of size 500, then the population proportion must be either:
A) 0.2 or 0.8.
B) 0.3 or 0.7.
C) 0.6 or 0.4.
D) 0.15 or 0.85.
A) 0.2 or 0.8.
B) 0.3 or 0.7.
C) 0.6 or 0.4.
D) 0.15 or 0.85.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
34
Suppose we know that the proportion of objects in a population that have a particular characteristic of interest is 0.10.Are there any concerns if we want to calculate probabilities for the sampling distribution of 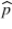 n = 40 using a normal approximation?
n = 40 using a normal approximation?
A) Because nis large, our only concern with using a normal approximation will be the small amount of variability in the sampling distribution of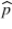 .
.
B) Because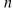 is small here, the sampling distribution of
is small here, the sampling distribution of 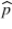 should not be assumed to be approximately normal.
should not be assumed to be approximately normal.
C) Because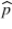 ≤ 0.5, the sampling distribution of
≤ 0.5, the sampling distribution of 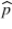 should not be assumed to be approximately normal.
should not be assumed to be approximately normal.
D) If n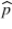 < 5, then a normal approximation is not appropriate.
< 5, then a normal approximation is not appropriate.
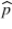 n = 40 using a normal approximation?
n = 40 using a normal approximation?A) Because nis large, our only concern with using a normal approximation will be the small amount of variability in the sampling distribution of
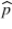 .
.B) Because
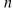 is small here, the sampling distribution of
is small here, the sampling distribution of 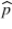 should not be assumed to be approximately normal.
should not be assumed to be approximately normal.C) Because
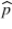 ≤ 0.5, the sampling distribution of
≤ 0.5, the sampling distribution of 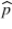 should not be assumed to be approximately normal.
should not be assumed to be approximately normal.D) If n
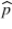 < 5, then a normal approximation is not appropriate.
< 5, then a normal approximation is not appropriate.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
35
If the population proportion is 0.38, what is 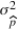 if we calculate
if we calculate 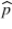 using 20 samples?
using 20 samples?
A) 0.0118
B) 0.1085
C) 0.0190
D) Because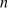 is small, we cannot calculate
is small, we cannot calculate 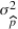 accurately.
accurately.
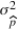 if we calculate
if we calculate 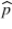 using 20 samples?
using 20 samples?A) 0.0118
B) 0.1085
C) 0.0190
D) Because
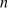 is small, we cannot calculate
is small, we cannot calculate 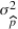 accurately.
accurately.
Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
36
In a major city in the northwest United States, 28% of the days experience some period of rain.What is the probability that more than 12 days out of a random sample of 31 days will experience some period of rain?
A) 0.0918
B) 0.0806
C) 0.9082
D) 0.3871
A) 0.0918
B) 0.0806
C) 0.9082
D) 0.3871

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
37
The standard error of a statistic used as an estimator of a population parameter is:
A) the standard deviation of the sampling distribution of the statistic.
B) the variance of the sampling distribution of the statistic.
C) the same value as the population standard deviation.
D) the square root of the population variance.
A) the standard deviation of the sampling distribution of the statistic.
B) the variance of the sampling distribution of the statistic.
C) the same value as the population standard deviation.
D) the square root of the population variance.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
38
The survival times of adult mosquitoes exposed to a commonly used synthetic pesticide (Phenothrin) follow a gamma distribution, with a mean time until extermination of 48 sec and a standard deviation of 15.18 sec.What is the probability that a random sample of 40 mosquitoes will have an average survival time between 47.5 sec and 48 sec?
A) 0.0832
B) 0.4207
C) 0.4880
D) 0.0120
A) 0.0832
B) 0.4207
C) 0.4880
D) 0.0120

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
39
In a study whose results were published in the British Medical Journal (Willis et al., 2004), researchers tested whether dogs can smell cancer in humans.Each dog used in the study was presented with seven urine samples to smell, with only one of the urine samples coming from a patient who had bladder cancer.Taken as a group, the dogs were tested on 54 trials and correctly identified the urine from the cancer patient in 22 trials.If the dogs were just guessing and so had a probability of success p = 1/7, what is the probability they would be correct in 22 or more of the 54 trials?
A) 0.000
B) 0.042
C) 0.087
D) 0.168
A) 0.000
B) 0.042
C) 0.087
D) 0.168

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
40
The speed at which an automated assembly line produces a product follows a normal distribution, with a mean production time of 32.20 sec and a standard deviation of 1.05 sec.A full production run from this line consists of 20 completed products.What is the probability that a full production run will take less than 32 sec, on average, to finish?
A) 0.4247
B) 0.1977
C) 0.1900
D) 0.5753
A) 0.4247
B) 0.1977
C) 0.1900
D) 0.5753

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
41
Suppose 45% of voters favor candidate A.We can be 67% sure that out of 200 randomly selected voters, at least how many will favor candidate A?
A) 90
B) 87
C) 134
D) 93
A) 90
B) 87
C) 134
D) 93

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
42
If the population is perfectly normal, the sampling distribution of 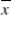 will be:
will be:
A) exactly normal for any sample size.
B) approximately normal for any sample size.
C) exactly normal only if the sample size is large enough.
D) approximately normal only if the sample size is large enough.
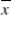 will be:
will be:A) exactly normal for any sample size.
B) approximately normal for any sample size.
C) exactly normal only if the sample size is large enough.
D) approximately normal only if the sample size is large enough.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
43
If the sample size (n) is large (say, n ≥ 30), then it is safe to model the sampling distribution of 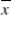 as a:
as a:
A) discrete distribution.
B) continuous distribution.
C) log-normal distribution.
D) normal distribution.
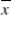 as a:
as a:A) discrete distribution.
B) continuous distribution.
C) log-normal distribution.
D) normal distribution.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
44
The problems associated with sampling without replacement in the simple random sample process are negligible provided:
A) the sample is large relative to the population size.
B) the population is small relative to the sample size.
C) the sample and the population are of roughly the same size.
D) the population is large relative to the sample size.
A) the sample is large relative to the population size.
B) the population is small relative to the sample size.
C) the sample and the population are of roughly the same size.
D) the population is large relative to the sample size.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
45
We wish to survey high schools in a particular city.If the city has 22 high schools and we want to select 5 of them, how many unique samples of size 5 are possible?
A) 3,160,080
B) 5,153,632
C) 26,334
D) 2.384 × 1015
A) 3,160,080
B) 5,153,632
C) 26,334
D) 2.384 × 1015

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
46
A sample of 82 mice is observed from birth until they are one year old.The total amount of growth experienced by each mouse is recorded.The median amount of growth of these mice is 4.5 cm.In this example, 4.5 cm is a:
A) parameter.
B) sampling distribution.
C) statistic.
D) random variable.
A) parameter.
B) sampling distribution.
C) statistic.
D) random variable.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
47
All statistics may be treated as:
A) characteristics of a population.
B) fixed quantities.
C) round variables.
D) random variables.
A) characteristics of a population.
B) fixed quantities.
C) round variables.
D) random variables.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
48
If n ≥ 30, we know that the sampling distribution of 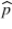 is:
is:
A) approximately normal.
B) exactly normal.
C) suitable for estimating the unknown parameter p.
D) unknown, because n ≥ 30 alone tells us nothing substantial concerning the sampling distribution of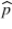 .
.
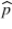 is:
is:A) approximately normal.
B) exactly normal.
C) suitable for estimating the unknown parameter p.
D) unknown, because n ≥ 30 alone tells us nothing substantial concerning the sampling distribution of
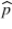 .
.
Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
49
According to the Central Limit Theorem, as the sample size (n) from a non-normal population increases:
A) the mean of the sampling distribution of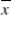 becomes closer to μ, the population mean.
becomes closer to μ, the population mean.
B) the sample standard deviation decreases.
C) the sampling distribution of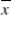 increasingly approximates a normal distribution.
increasingly approximates a normal distribution.
D) there is less of a problem with nonrepresentative sample bias.
A) the mean of the sampling distribution of
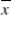 becomes closer to μ, the population mean.
becomes closer to μ, the population mean.B) the sample standard deviation decreases.
C) the sampling distribution of
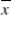 increasingly approximates a normal distribution.
increasingly approximates a normal distribution.D) there is less of a problem with nonrepresentative sample bias.

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
50
If a population is skewed, based on the Central Limit Theorem, what is a good rule of thumb for assuming the sampling distribution of 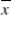 is approximately normal?
is approximately normal?
A) np ≥ 5
B) n ≥ 30
C) μ ≥ 30
D) n ≤ 30
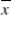 is approximately normal?
is approximately normal?A) np ≥ 5
B) n ≥ 30
C) μ ≥ 30
D) n ≤ 30

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck



