Deck 5: Static Magnetic Fields
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/64
Play
Full screen (f)
Deck 5: Static Magnetic Fields
1
Refer to Problem P.5-4 and Fig. 5-25. Find the magnetic flux density B 2. at point P 2 ( w + d 2 , 0).
Write the expression for magnetic flux density at a point located at a distance
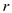 from the wire in the bisecting plane:
from the wire in the bisecting plane:
 When
When
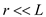 Consider that a small element of conducting wire
Consider that a small element of conducting wire
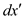 along x -axis leads to corresponding change in the magnetic flux density
along x -axis leads to corresponding change in the magnetic flux density
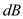 , as shown in Figure 1.
, as shown in Figure 1.
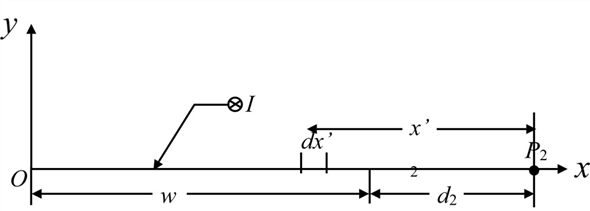 Figure 1
Figure 1
The vector form of the magnetic field due to small element of conducting wire
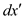 is:
is:
 If we align the conductor along the z-axis, the magnetic flux density
If we align the conductor along the z-axis, the magnetic flux density
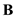 will be
will be
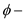 directed in negative direction. Thus,
directed in negative direction. Thus,

 …… (1)
…… (1)
Integrate equation (1) with limits from
 to
to
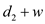 on both sides,
on both sides,

 Therefore magnetic flux density
Therefore magnetic flux density
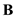 at point
at point
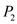 is
is
 .
.
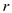 from the wire in the bisecting plane:
from the wire in the bisecting plane:  When
When 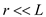 Consider that a small element of conducting wire
Consider that a small element of conducting wire 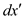 along x -axis leads to corresponding change in the magnetic flux density
along x -axis leads to corresponding change in the magnetic flux density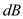 , as shown in Figure 1.
, as shown in Figure 1. Figure 1
Figure 1The vector form of the magnetic field due to small element of conducting wire
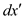 is:
is: If we align the conductor along the z-axis, the magnetic flux density
If we align the conductor along the z-axis, the magnetic flux density 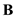 will be
will be 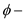 directed in negative direction. Thus,
directed in negative direction. Thus,
 …… (1)
…… (1)Integrate equation (1) with limits from
 to
to 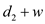 on both sides,
on both sides,
 Therefore magnetic flux density
Therefore magnetic flux density 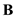 at point
at point 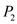 is
is .
. 2
What is the relation between vector magnetic potential A and the magnetic flux through a given area
The relation between the vector magnetic potential
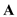 and magnetic flux
and magnetic flux
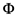 through a given area is stated by an equation:
through a given area is stated by an equation:
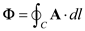 Clearly from this expression, the circulation of the vector magnetic potential
Clearly from this expression, the circulation of the vector magnetic potential
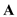 around in any closed area is equal to the total magnetic flux which is passing through the enclosed area path.
around in any closed area is equal to the total magnetic flux which is passing through the enclosed area path.
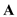 and magnetic flux
and magnetic flux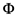 through a given area is stated by an equation:
through a given area is stated by an equation: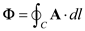 Clearly from this expression, the circulation of the vector magnetic potential
Clearly from this expression, the circulation of the vector magnetic potential 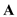 around in any closed area is equal to the total magnetic flux which is passing through the enclosed area path.
around in any closed area is equal to the total magnetic flux which is passing through the enclosed area path. 3
Does the magnetic field intensity due to a current distribution depend on the properties of the medium Does the magnetic flux density
The equation of the magnetic flux density(B) is,
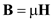 (Wb/m²)
(Wb/m²)
The magnetic flux density is
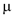 times the magnetic field intensity.
times the magnetic field intensity.
The equation of the magnetic field intensity (H) is,
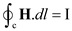 (A)
(A)
The magnetic field intensity due to current distribution depends on the property of the medium because the magnetic field intensity is mainly based on the distribution of the current in the circuit.
The magnetic flux density is
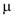 times the magnetic field intensity,
times the magnetic field intensity,
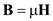 As, the magnetic field intensity due to current distribution depends on the medium, hence magnetic flux density also depends on the medium.
As, the magnetic field intensity due to current distribution depends on the medium, hence magnetic flux density also depends on the medium.
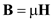 (Wb/m²)
(Wb/m²)The magnetic flux density is
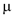 times the magnetic field intensity.
times the magnetic field intensity.The equation of the magnetic field intensity (H) is,
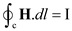 (A)
(A)The magnetic field intensity due to current distribution depends on the property of the medium because the magnetic field intensity is mainly based on the distribution of the current in the circuit.
The magnetic flux density is
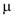 times the magnetic field intensity,
times the magnetic field intensity, 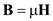 As, the magnetic field intensity due to current distribution depends on the medium, hence magnetic flux density also depends on the medium.
As, the magnetic field intensity due to current distribution depends on the medium, hence magnetic flux density also depends on the medium. 4
Write the formula expressing the torque on a current-carrying circuit in a magnetic field.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
5
Which postulate of magnetostatics denies the existence of isolated magnetic charges

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
6
A very large slab of material of thickness d lies perpendicularly to a uniform magnetic field of intensity H 0 = a z H 0. Ignoring edge effect, determine the magnetic field intensity in the slab:
a) if the slab material has a permeability , and
b) if the slab is a permanent magnet having a magnetization vector M i = a z M i.
a) if the slab material has a permeability , and
b) if the slab is a permanent magnet having a magnetization vector M i = a z M i.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
7
The cross section of a long thin metal strip and a parallel wire is shown in Fig. 5-30. Equal and opposite currents I flow in the conductors. Find the force per unit length on the conductors.
Fig. Cross section of parallel strip and wire conductor (Problem P.5-19)
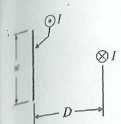
Fig. Cross section of parallel strip and wire conductor (Problem P.5-19)


Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
8
Explain the principle of operation of d-c motors.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
9
A cylindrical magnet of radius 5 (cm) and length 12 (cm) has an axial magnetization a z 130 (A/cm). Find B at
a) the center of the top face,
b) the center of the bottom face, and
c) the center of the magnet.
a) the center of the top face,
b) the center of the bottom face, and
c) the center of the magnet.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
10
State Biot-Savart law.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
11
Define diamagnetic, paramagnetic, and ferromagnetic materials.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
12
What is the relation between the force and the stored magnetic energy in a system of current-carrying circuits under the condition of constant flux linkages

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
13
An infinitely long, very thin cylindrical conducting tube of radius b carries a uniform surface current J s = a z J s (A/m). Find B everywhere.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
14
A current I flows in the inner conductor of an infinitely long coaxial line and returns via the outer conductor. The radius of the inner conductor is a and the inner and outer radii of the outer conductor are b and c, respectively Find the magnetic flux density B for all regions and plot |B| versus r.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
15
A circular rod of magnetic material with permeability is inserted coaxially in a very long air solenoid. The radius of the rod, a, is less than the inner radius, b, of the solenoid. The solenoid's winding has n turns per unit length and carries a current I.
a) Find the values of B, H, and M inside the solenoid for r and for a
b) What are the equivalent magnetization current densities J mv and J ms for the magnetized rod
a) Find the values of B, H, and M inside the solenoid for r and for a
b) What are the equivalent magnetization current densities J mv and J ms for the magnetized rod

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
16
The bar AA in Fig. 5-31 serves as a conducting path (such as the blade of a circuit breaker) for the current I in two very long parallel lines. The lines have a radius b and are spaced at a distance d apart. Find the direction and the magnitude of the magnetic force on the bar.
Fig Force on conducting bar (Problem P5-20)
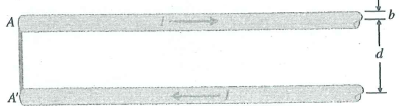
Fig Force on conducting bar (Problem P5-20)


Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
17
A point charge Q with a velocity u = a x u 0 enters a region having a uniform magnetic field B = a x B x + a y B y + a z B z. What E field should exist in the region so that the charge proceeds without a change of velocity

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
18
State the law of conservation of magnetic flux.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
19
What is a magnetic dipole Define magnetic dipole moment. What is its SI unit

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
20
What is a hysteresis loop

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
21
What is the expression for the force on a test charge q that moves with velociy u in a magnetic field of flux density B

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
22
The magnetic field intensity H 1 in medium 1 having a permeability 1 makes an angle 1 with the normal at an interface with medium 2 having a permeability 2. Find the relation between the angle 2 (that H 2 makes with the normal) and 1.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
23
A ferromagnetic sphere of radius b is magnetized uniformly with a magnetization M = a z M 0.
a) Determine the equivalent magnetization current densities J mv and J ms.
b) Determine the magnetic flux density at the center of the sphere.
a) Determine the equivalent magnetization current densities J mv and J ms.
b) Determine the magnetic flux density at the center of the sphere.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
24
A d-c current I = 10 (A) flows in a triangular loop in the xy -plane as in Fig. 5-32. Assuming a uniform magnetic flux density B = a y 6(mT) in the region, find the forces and torque on the loop. The dimensions are in (cm).
Fig. A triangular loop in a uniform magnetic field (Problem P.5-21)
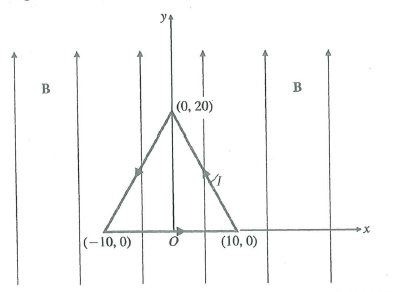
Fig. A triangular loop in a uniform magnetic field (Problem P.5-21)


Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
25
Find the magnetic flux density inside a very long cylindrical solenoid with an air core having n turns per meter and carrying a current I.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
26
A thin conducting wire of length 3 w forms a planar equilateral triangle A direct current I flows in the wire. Find the magnetic flux density at the center of the triangle.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
27
Define magnetization vector. What is its SI unit

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
28
Define remanent flux density and coercive field intensity.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
29
Find the total magnetic flux through a circular toroid with a rectangular cross section of height h. The inner and outer radii of the toroid are a and b, respectively. A current I flows in N turns of closely wound wire around the toroid. Determine the percentage of error if the flux is found by multiplying the cross-sectional area by the flux density at the mean radius. What is the error if b/a = 5

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
30
State Ampère's circuital law.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
31
Consider a plane boundary ( y = 0) between air (region 1, r 1 = 1) and iron (region 2, r 2 = 5000).
a) Assuming B 1 = a x 2 a y 10(mT), find B 2 and the angle that B 2 makes with the interface.
b) Assuming B 2 = a x 10 + a y 2 (mT), find B 1 and the angle that B 1 makes with the normal to the interface.
a) Assuming B 1 = a x 2 a y 10(mT), find B 2 and the angle that B 2 makes with the interface.
b) Assuming B 2 = a x 10 + a y 2 (mT), find B 1 and the angle that B 1 makes with the normal to the interface.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
32
A small circular turn of wire of radius r 1 that carries a steady current I 1 is placed at the center of a much larger turn of wire of radius r 2 ( r 2 r 1 that carries a steady current I 2 in the same direction. The angle between the normals of the two circuits is and the small circular wire is free to turn about its diameter. Determine the magnitude and the direction of the torque on the small circular wire.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
33
Verify that tesla (T), the unit for magnetic flux density, is the same as Volt second per square meter (V · s/m 2 ).

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
34
Express the stored magnetic energy in terms of flux linkage and current I in an inductor having an inductance L

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
35
What is meant by "equivalent magnetization current densities" What are the SI units for × M and M × a n

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
36
Discuss the difference between soft and hard ferromagnetic materials.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
37
Verify Eq. (5-17) in Cartesian coordinates.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
38
Refer to Fig. 5-26; Determine the magnetic flux density at a point P on the axis of a solenoid with radius b and length L, and with a current I in its N turns of closely wound coil. Show that the result reduces to that given in Eq (5-82) when L approaches infinity. Hint: Use Eq. (5-37).

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
39
Determine the self-inductance of a toroidal coil of N turns of wife wound on an air frame with mean radius r o and a circular cross section of radius b. Obtain an approximate expression assuming b r o.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
40
What is curie temperature

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
41
direct current I flows in a straight filamentary conductor P 1 P 2.
a) Prove that B at a point P , whose location is specified by the perpendicular distance r and the two angles 1 and 2 shown in Fig 5-24 is
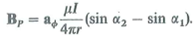 (5-135).
(5-135).
b) Verify that Eq. (5-135) reduces to Eq. (5-35) when the wire is infinitely long.
a) Prove that B at a point P , whose location is specified by the perpendicular distance r and the two angles 1 and 2 shown in Fig 5-24 is
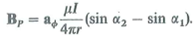 (5-135).
(5-135).b) Verify that Eq. (5-135) reduces to Eq. (5-35) when the wire is infinitely long.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
42
In what manner does the B -field of an infinitely long straight filament carrying a direct current I vary with distance

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
43
Define magnetic field intensity vector. What is its SI unit

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
44
What are the boundary conditions for magnetostatic fields at an interface between two different magnetic media

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
45
Write Lorentz's force equation.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
46
A current I flows in the N -turn toroidal coil in Fig. 5-15.
a) Obtain an expression for the stored magnetic energy.
b) Use Eq. (5-109) to determine its self-inductance and check your result with Eq. (5-81).
a) Obtain an expression for the stored magnetic energy.
b) Use Eq. (5-109) to determine its self-inductance and check your result with Eq. (5-81).

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
47
Determine the mutual inductance between a very long, straight Wire and a conducting equilateral triangular loop, as shown in Fig. 5-27.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
48
Define (a) the mutual inductance between two circuits, and (b) the self inductance of a single coil.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
49
An 8 (cm) × 6 (cm) rectangular conducting loop lies in the xy -plane. A direct current of 5 (A) flows in a clockwise direction viewing from the top. Find B at the center of the loop.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
50
A direct current I flows in an infinitely long wire of a radius 2( mm ) along the z -axis.
a) Obtain the vector magnetic potential A at r 2(mm) from the expression of B in Eq. (5-12). Choose the reference zero potential at wire surface.
b) If I = 10(A), determine from A the total amount of magnetic flux passing through a square loop specified by z = ±0.3(m) and y = 0.1 (m) and 0.7 (m).
a) Obtain the vector magnetic potential A at r 2(mm) from the expression of B in Eq. (5-12). Choose the reference zero potential at wire surface.
b) If I = 10(A), determine from A the total amount of magnetic flux passing through a square loop specified by z = ±0.3(m) and y = 0.1 (m) and 0.7 (m).

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
51
Write the two fundamental governing differential equations magnetostatics.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
52
What is meant by the internal inductance of a conductor

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
53
A current I flows lengthwise in a very long, thin conducting sheet of width w, as shown in Fig. 5-25. Assuming that the current flows into the paper, determine the magnetic flux density B 1 at point P 1 (0, d ).

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
54
Define vector magnetic potential A. What is its SI unit

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
55
Find the mutual inductance between two coplanar rectangular loops with parallel sides, as shown in Fig. 5-28. Assume that h 1 h 2 ( h 2 w 2 d ).

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
56
Write the expression for the stored magnetic energy of two coupled current carrying loops.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
57
What are the two fundamental postulates of magnetostatics

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
58
Assume that a current I 2 flows in the rectangular loop in Fig. 5-18 in the clockwise direction. Determine the net force on the loop.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
59
Define magnetic susceptibility and relative permeability. What are their units

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
60
Write the expression for stored magnetic energy in terms of field quantities.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
61
Refer to Fig. 5-6. Find B :
a) at the center of a circular loop of radius 5 (cm) carrying a direct current 2(A) and
b) at the center of a semi-circular loop of radius 8 (cm) carrying a direct current 4(A).
a) at the center of a circular loop of radius 5 (cm) carrying a direct current 2(A) and
b) at the center of a semi-circular loop of radius 8 (cm) carrying a direct current 4(A).

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
62
A d-c surface current with density a x J s 0 flows in an infinite conducting sheet coinciding with the xy -plane.
a) Determine the magnetic flux density B at (0, 0, z) and at (0, 0, z ).
b) Find the vector magnetic potential A at (0, 0, z ) from B. Choose the reference zero potential at an arbitrary point z = z 0.
a) Determine the magnetic flux density B at (0, 0, z) and at (0, 0, z ).
b) Find the vector magnetic potential A at (0, 0, z ) from B. Choose the reference zero potential at an arbitrary point z = z 0.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
63
Calculate the force per unit length on each of three equidistant, infinitely long, parallel wires 10 (cm) apart, each carrying a current of 25(A) in the same direction. A cross section of the arrangement is shown in Fig. 5.29 Specify the direction of the force.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
64
Give the integral expression for the force on a closed circuit that carries. a current I in a magnetic field B.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck



