Deck 11: CHI-Squared Tests of Fit
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/56
Play
Full screen (f)
Deck 11: CHI-Squared Tests of Fit
1
Parametric tests work by assuming ______.
A) that a meaningful statistic can be set and then testing a parameter again that statistic
B) that a meaningful parameter can be set and then testing a sample against that parameter
C) that a parameter can be used to reasonably summarize data and then measuring that parameter
D) none of these
A) that a meaningful statistic can be set and then testing a parameter again that statistic
B) that a meaningful parameter can be set and then testing a sample against that parameter
C) that a parameter can be used to reasonably summarize data and then measuring that parameter
D) none of these
B
2
A non-parametric test ______.
A) makes fewer assumptions about the parameters it measures
B) does not assume that a meaningful parameter exists
C) does not make assumptions about scale of measurement
D) none of these
A) makes fewer assumptions about the parameters it measures
B) does not assume that a meaningful parameter exists
C) does not make assumptions about scale of measurement
D) none of these
B
3
Because they do not rely upon means and standard deviations, non-parametric tests can often be conducted when the scale of measurement of the outcome variable is ______.
A) nominal or ordinal
B) ordinal or interval
C) interval or ratio
D) ratio only
A) nominal or ordinal
B) ordinal or interval
C) interval or ratio
D) ratio only
A
4
Non-parametric tests examine the ______ of a(n) ______ .
A) goodness, independence
B) independence, goodness
C) fit, model
D) model, fit
A) goodness, independence
B) independence, goodness
C) fit, model
D) model, fit

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
5
A goodness of fit test is used when we want to see how likely data is given a model of likely ______.
A) counts of a single nominal variable
B) interactions between two nominal variables
C) values for a parameter
D) none of these
A) counts of a single nominal variable
B) interactions between two nominal variables
C) values for a parameter
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
6
Which of these is a research question describing a goodness of fit test?
A) Is this preference for our product likely given a population mean of 8?
B) Are there differences in preferences for our product between groups?
C) Between our three products, is one chosen more often by men?
D) When sampling each of our products, which is most often chosen as best?
A) Is this preference for our product likely given a population mean of 8?
B) Are there differences in preferences for our product between groups?
C) Between our three products, is one chosen more often by men?
D) When sampling each of our products, which is most often chosen as best?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
7
Which of these is a research question describing a test of independence?
A) Is this preference for our product likely given a population mean of 8?
B) Are there differences in preferences for our product between groups?
C) Between our three products, is one chosen more often by men?
D) When sampling each of our products, which is most often chosen as best?
A) Is this preference for our product likely given a population mean of 8?
B) Are there differences in preferences for our product between groups?
C) Between our three products, is one chosen more often by men?
D) When sampling each of our products, which is most often chosen as best?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
8
Which of these is a valid null hypothesis for a χ2 goodness of fit test?
A) Product preferences are independent of gender.
B) Product preferences are not independence of gender.
C) There is no difference between observed and expected frequencies of product preference.
D) There is a difference between observed and expected frequencies of product preference.
A) Product preferences are independent of gender.
B) Product preferences are not independence of gender.
C) There is no difference between observed and expected frequencies of product preference.
D) There is a difference between observed and expected frequencies of product preference.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
9
Which of these is a valid alternative hypothesis for a χ2 test of independence?
A) Product preferences are independent of gender.
B) Product preferences are not independence of gender.
C) There is no difference between observed and expected frequencies of product preference.
D) There is a difference between observed and expected frequencies of product preference.
A) Product preferences are independent of gender.
B) Product preferences are not independence of gender.
C) There is no difference between observed and expected frequencies of product preference.
D) There is a difference between observed and expected frequencies of product preference.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
10
The shape of the χ2 distribution is dependent on ______.
A) between-groups degrees of freedom
B) within-groups degrees of freedom
C) total degrees of freedom
D) none of these
A) between-groups degrees of freedom
B) within-groups degrees of freedom
C) total degrees of freedom
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
11
Degrees of freedom for a χ2 test are dependent upon the number of ______.
A) subjects
B) categories
C) types
D) none of these
A) subjects
B) categories
C) types
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
12
When = .05 at 6 degrees of freedom, the critical χ2 is ______.
A) 12.592
B) 24.725
C) 11.070
D) none of these
A) 12.592
B) 24.725
C) 11.070
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
13
In a goodness of fit test, when = .05 and k = 9, degrees of freedom are ______.
A) 5
B) 9
C) 8
D) none of these
A) 5
B) 9
C) 8
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
14
In a test of independence, when = .01, k1 = 7 and k2 = 4, degrees of freedom are ______.
A) 6
B) 3
C) 27
D) 18
A) 6
B) 3
C) 27
D) 18

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
15
In a goodness of fit test, when = .01 and n = 20, the critical χ2 is ______.
A) 52.191
B) 50.892
C) 31.410
D) none of these
A) 52.191
B) 50.892
C) 31.410
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
16
In a goodness of fit test, when k = 5 and = .05, the critical χ2 is ______.
A) 9.488
B) 11.070
C) 24.725
D) none of these
A) 9.488
B) 11.070
C) 24.725
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
17
In a test of independence, when = .01 and k1 = 5, the critical χ2 is ______.
A) 21.666
B) 24.725
C) 9.488
D) none of these
A) 21.666
B) 24.725
C) 9.488
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
18
In a test of independence, when = .05, k1 = 6 and k2 = 3, the critical χ2 is ______.
A) 28.869
B) 12.592
C) 18.307
D) 7.815
A) 28.869
B) 12.592
C) 18.307
D) 7.815

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
19
The χ2 statistic examines ______.
A) the difference between observed and expected counts as a proportion of expected counts
B) the squared difference between observed and expected counts as a proportion of expected counts
C) the difference between observed and expected counts as a proportion of observed counts
D) the squared difference between observed and expected counts as a proportion of observed counts
A) the difference between observed and expected counts as a proportion of expected counts
B) the squared difference between observed and expected counts as a proportion of expected counts
C) the difference between observed and expected counts as a proportion of observed counts
D) the squared difference between observed and expected counts as a proportion of observed counts

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
20
In a goodness-of-fit test, when n = 30 and k = 3, what do we expect to occur in each cell?
A) 3
B) 30
C) 10
D) 90
A) 3
B) 30
C) 10
D) 90

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
21
A ______ table is used to illustrate the counts at each intersection of two variables in a test of independence.
A) contingency
B) cross
C) computational
D) none of these
A) contingency
B) cross
C) computational
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
22
In a test of independence, a unique effect created by a particular combination of levels in two variables is called a(n) ______.
A) interaction
B) blend
C) combination
D) none of these
A) interaction
B) blend
C) combination
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
23
χ2 tests are sensitive to small cell sizes. You should try to have a sufficient sample size to have at least ______ observed or expected cases in every cell.
A) 3
B) 5
C) 10
D) 15
A) 3
B) 5
C) 10
D) 15

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
24
If 2(3) = 8.1, then you should ______.
A) Reject the null
B) Retain the null
C) Retain the alternative
D) Reject the alternative
A) Reject the null
B) Retain the null
C) Retain the alternative
D) Reject the alternative

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
25
In a test of independence, if 2 = 9.0, k1 = 3 and k2 = 3, you should ______.
A) Reject the null
B) Retain the null
C) Retain the alternative
D) Reject the alternative
A) Reject the null
B) Retain the null
C) Retain the alternative
D) Reject the alternative

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
26
If 2 = 2, n = 10 and k = 4, what is the value of Cramer's V?
A) 0.26
B) 0.07
C) 2
D) none of these
A) 0.26
B) 0.07
C) 2
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
27
If 2 = 4, n = 20, k1 = 5 and k2 = 7, what is the value of Cramer's V?
A) 0.05
B) 0.26
C) 5
D) none of these
A) 0.05
B) 0.26
C) 5
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
28
Which of the following is considered a "strong" Cramer's V?
A) .2
B) .8
C) -.8
D) none of these
A) .2
B) .8
C) -.8
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
29
When a χ2 is statistically significant, for supplemental analyses, we ______.
A) calculate Cramer's V
B) calculate Cohen's d
C) calculate a phi coefficient
D) none of these
A) calculate Cramer's V
B) calculate Cohen's d
C) calculate a phi coefficient
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
30
A Cramer's V calculated on a 2 × 2 contingency table is also called a ______.
A) Cohen's d
B) phi coefficient
C) eta-squared
D) none of these
A) Cohen's d
B) phi coefficient
C) eta-squared
D) none of these

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
31
With one degree of freedom, the χ2 distribution looks similar to a normal distribution.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
32
In a χ2 goodness of fit test, the expected values are always equal.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
33
Non-parametric tests are used when we cannot make assumptions about parameters.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
34
With a non-parametric test, we examine how particular data fit a particular model.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
35
In a goodness of fit test, we test a model assuming that two variables are independent from one another.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
36
χ2 tests are always non-directional.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
37
Counts occurring at all possible combinations of two variables can be expressed using a contingency table.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
38
The following is a valid null hypothesis: There is a difference between observed and expected frequencies of gum preference.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
39
The following is a valid alternative hypothesis: : Gum preference is not independent of gender.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
40
All χ2 distributions are similar to the normal distribution.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
41
The region of rejection in χ2 is always in the left tail.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
42
When conducting a goodness-of-fit test on data with 6 groups, there are 5 degrees of freedom.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
43
When conducting a goodness-of-fit test on data with 7 groups, the critical value is 12.592.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
44
When conducting a χ2 test of independence with 8 groups in the first variable and 5 groups in the second variable, there are 28 degrees of freedom.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
45
When conducting a χ2 test of independence when k1 = 3 and k2 = 4, the critical value is 21.026.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
46
χ2 is calculated as the sum of all squared differences between observed and expected counts, divided by observed counts.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
47
Because χ2 is sensitive to small cell counts, you should always collect a large enough sample so that observed and expected cell sizes are all above 5.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
48
If 2(2) = +1.133, the null should be rejected.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
49
If 2 = 20 and k = 12, the null should be retained.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
50
Cramer's V can be interpreted as the difference between cells in standard deviation units.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
51
When is a non-parametric test more appropriate than a parametric test?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
52
How do you determine the expected model for a goodness-of-fit test? Provide an example.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
53
When is a χ2 test of independence used? Provide an example of significant interaction in a business context.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
54
Cramer's V is not easily interpretable on its own. But under what conditions is it more useful?

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
55
You run a small business with four employees: Susan, Jimmy, Albert, and Camilla. Because you need three employees at work at any given time, only one employee ever has the day off. Unfortunately, everyone always wants Saturday off. One of your employees has confronted you and said that you favour some employees over others in providing Saturdays off. To investigate this, you pulled up a list of who has recently had Saturdays off each week. Over the past 24 weeks, Jimmy has had 10 Saturdays off, Susan has had 2 Saturdays off, Albert has had 12 Saturdays off, and Camilla has had 16 Saturdays off.
1) What is the research question?
2) What are the hypotheses?
3) What are the degrees of freedom?
4) What is the critical value for this test?
5) State the formal results of the test.
6) Should you compute a measure of the overall effect size? How do you know? If so, calculate this effect size.
7) State all appropriate conclusions.
1) What is the research question?
2) What are the hypotheses?
3) What are the degrees of freedom?
4) What is the critical value for this test?
5) State the formal results of the test.
6) Should you compute a measure of the overall effect size? How do you know? If so, calculate this effect size.
7) State all appropriate conclusions.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
56
You run a small business with three retail clothing outlets. At each outlet, you sell three types of products: budget items, mid-range, and high fashion. You have decided to investigate if sales of these types differ by store. To do this, you retrieved a list of sales at all three stores and created a dataset also recording which store sold which item. Conduct a test of independence to investigate this. The contingency table from your records appears below.
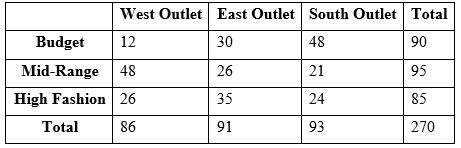 1) What is the research question?
1) What is the research question?
2) What are the hypotheses?
3) What are the degrees of freedom?
4) What is the critical value for this test?
5) State the formal results of the test.
6) Should you compute a measure of the overall effect size? How do you know? If so, calculate this effect size.
7) State all appropriate conclusions.
 1) What is the research question?
1) What is the research question?2) What are the hypotheses?
3) What are the degrees of freedom?
4) What is the critical value for this test?
5) State the formal results of the test.
6) Should you compute a measure of the overall effect size? How do you know? If so, calculate this effect size.
7) State all appropriate conclusions.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck



