Deck 5: Mechanistic Analysis
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/53
Play
Full screen (f)
Deck 5: Mechanistic Analysis
1
Consider an elementary reaction
where M is a metal and
is metal ion in solution. F denotes faraday constant. The net faradaic current density due to metal deposition is given by
A)
B)
C)
D)
where M is a metal and
is metal ion in solution. F denotes faraday constant. The net faradaic current density due to metal deposition is given by
A)
B)
C)
D)
2
Consider a reaction given by .
-In the standard notation, the fractional surface coverage ( ) of the adsorbed intermediate
is described by
A)
B)
C)
D)
-In the standard notation, the fractional surface coverage ( ) of the adsorbed intermediate
is described by
A)
B)
C)
D)
3
Consider a reaction given by .
-In the same mechanism, the steady-state fractional surface coverage of the intermediate is given by
A)
B)
C)
D)
-In the same mechanism, the steady-state fractional surface coverage of the intermediate is given by
A)
B)
C)
D)
4
Consider a reaction given by .
-In the same mechanism, the faradaic current is given by
A)
B)
C)
D)
-In the same mechanism, the faradaic current is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
5
Consider a reaction given by .
-In the same mechanism, the value of
is given by
A)
B)
C)
D)
-In the same mechanism, the value of
is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
6
Consider a reaction given by .
-In the same mechanism, the faradaic impedance (ZF) is given by
A)
B)
C)
D)
-In the same mechanism, the faradaic impedance (ZF) is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
7
Consider a reaction given by 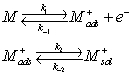 .
.
-In the above reaction, the rate constants are given by
where E is measured wrt equilibrium potential. Given that k10 = 10-7 mol cm-2 s-1, b1 = 20 V-1, b-1 = -18 V-1 , k2 = 10-5 mol cm-2 s-1, k-2 = 100 cm s-1 and the concentration of
as 25 mM, determine (i) k-10 = ___________ mol cm-2 s-1 . At E = 0.3 V vs. "Equilibrium. potential", (ii) value of k1dc = ___________mol cm-2 s-1, (iii) k-1dc = ___________mol cm-2 s-1, (iv) steady state fractional surface coverage of the intermediate is _______
Remember that 1 M = 1 mol/lit = 10-3 mol/ cm3.
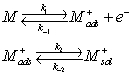 .
.-In the above reaction, the rate constants are given by
where E is measured wrt equilibrium potential. Given that k10 = 10-7 mol cm-2 s-1, b1 = 20 V-1, b-1 = -18 V-1 , k2 = 10-5 mol cm-2 s-1, k-2 = 100 cm s-1 and the concentration of
as 25 mM, determine (i) k-10 = ___________ mol cm-2 s-1 . At E = 0.3 V vs. "Equilibrium. potential", (ii) value of k1dc = ___________mol cm-2 s-1, (iii) k-1dc = ___________mol cm-2 s-1, (iv) steady state fractional surface coverage of the intermediate is _______
Remember that 1 M = 1 mol/lit = 10-3 mol/ cm3.

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
8
When kinetics is rate-limiting, the faradaic impedance of a simple electron transfer reaction can be modeled as
A) a simple resistor
B) a simple capacitor
C) a capacitor in parallel with a resistor
D) none of the above
A) a simple resistor
B) a simple capacitor
C) a capacitor in parallel with a resistor
D) none of the above

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
9
Consider the reaction
The kinetic parameters are given by k10 = 10-5 cm s-1, b1 = 20 V-1, k20 = 10-6 cm s-1, b2 = 20 V-1, k-20 =10-6 mol cm-2 s-1 , b-2 = -18 V-1 and = 10-8 mol cm-2. Solution species concentrations are given by [Psol] = [Qsol] =[
] = 100 mM.
-The equation for fractional surface coverage of Aads is given by
A)
B)
C)
D)
The kinetic parameters are given by k10 = 10-5 cm s-1, b1 = 20 V-1, k20 = 10-6 cm s-1, b2 = 20 V-1, k-20 =10-6 mol cm-2 s-1 , b-2 = -18 V-1 and = 10-8 mol cm-2. Solution species concentrations are given by [Psol] = [Qsol] =[
] = 100 mM.
-The equation for fractional surface coverage of Aads is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
10
Consider the reaction
The kinetic parameters are given by k10 = 10-5 cm s-1, b1 = 20 V-1, k20 = 10-6 cm s-1, b2 = 20 V-1, k-20 =10-6 mol cm-2 s-1 , b-2 = -18 V-1 and = 10-8 mol cm-2. Solution species concentrations are given by [Psol] = [Qsol] =[
] = 100 mM.
-At Edc = 0.25 V vs. OCP, the value of fractional surface coverage of Aads is given by,
ss = _________
The kinetic parameters are given by k10 = 10-5 cm s-1, b1 = 20 V-1, k20 = 10-6 cm s-1, b2 = 20 V-1, k-20 =10-6 mol cm-2 s-1 , b-2 = -18 V-1 and = 10-8 mol cm-2. Solution species concentrations are given by [Psol] = [Qsol] =[
] = 100 mM.
-At Edc = 0.25 V vs. OCP, the value of fractional surface coverage of Aads is given by,
ss = _________

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
11
Consider the reaction
The kinetic parameters are given by k10 = 10-5 cm s-1, b1 = 20 V-1, k20 = 10-6 cm s-1, b2 = 20 V-1, k-20 =10-6 mol cm-2 s-1 , b-2 = -18 V-1 and = 10-8 mol cm-2. Solution species concentrations are given by [Psol] = [Qsol] =[
] = 100 mM.
-At the same potential (Edc = 0.25 V vs. OCP), the total Faradaic current is given by,
iF-dc = ______________ mA cm-2
The kinetic parameters are given by k10 = 10-5 cm s-1, b1 = 20 V-1, k20 = 10-6 cm s-1, b2 = 20 V-1, k-20 =10-6 mol cm-2 s-1 , b-2 = -18 V-1 and = 10-8 mol cm-2. Solution species concentrations are given by [Psol] = [Qsol] =[
] = 100 mM.
-At the same potential (Edc = 0.25 V vs. OCP), the total Faradaic current is given by,
iF-dc = ______________ mA cm-2

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
12
The polarization plot of an electrochemical system is given below.
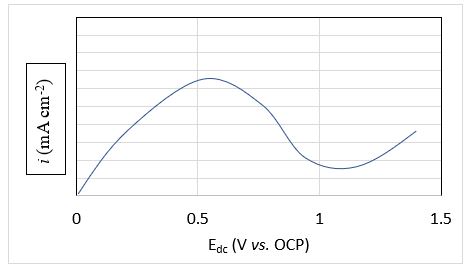
-If an impedance spectrum is acquired at Edc = 0.7 V vs. OCP, then the polarization resistance is expected to be.
A) negative
B) positive
C) can be either negative or positive
D) zero.

-If an impedance spectrum is acquired at Edc = 0.7 V vs. OCP, then the polarization resistance is expected to be.
A) negative
B) positive
C) can be either negative or positive
D) zero.

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
13
The polarization plot of an electrochemical system is given below.

-In the same system, if an impedance spectrum is acquired at Edc = 1.2 V vs. OCP, then the polarization resistance is expected to be.
A) negative
B) positive
C) can be either negative or positive
D) zero.

-In the same system, if an impedance spectrum is acquired at Edc = 1.2 V vs. OCP, then the polarization resistance is expected to be.
A) negative
B) positive
C) can be either negative or positive
D) zero.

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
14
Consider the reaction
The kinetic parameters are given by k10 = 10-9 mol cm-2 s-1, b1 = 10 V-1, k-10 = 10-8 mol cm-2 s-1, b-1 = -10 V-1, k20 = 10-8 mol cm-2 s-1, b2 = 10 V-1 and = 10-8 mol cm-2.
-At Edc = 0.1 V vs. OCP, the fractional surface coverage of Mads is given by,
ss = _________
The kinetic parameters are given by k10 = 10-9 mol cm-2 s-1, b1 = 10 V-1, k-10 = 10-8 mol cm-2 s-1, b-1 = -10 V-1, k20 = 10-8 mol cm-2 s-1, b2 = 10 V-1 and = 10-8 mol cm-2.
-At Edc = 0.1 V vs. OCP, the fractional surface coverage of Mads is given by,
ss = _________

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
15
Consider the reaction
The kinetic parameters are given by k10 = 10-9 mol cm-2 s-1, b1 = 10 V-1, k-10 = 10-8 mol cm-2 s-1, b-1 = -10 V-1, k20 = 10-8 mol cm-2 s-1, b2 = 10 V-1 and = 10-8 mol cm-2.
-To model a system with the above mechanism, the minimum number of dc potentials at which EIS data should be taken to avoid an infinite number of solutions is __________
The kinetic parameters are given by k10 = 10-9 mol cm-2 s-1, b1 = 10 V-1, k-10 = 10-8 mol cm-2 s-1, b-1 = -10 V-1, k20 = 10-8 mol cm-2 s-1, b2 = 10 V-1 and = 10-8 mol cm-2.
-To model a system with the above mechanism, the minimum number of dc potentials at which EIS data should be taken to avoid an infinite number of solutions is __________

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
16
In a kinetic limited electrochemical reaction, the number of loops appearing in the complex plane plot of EIS can be related to the
A) number of steps in the mechanism
B) the number of reacting species
C) the number of product species
D) the number of adsorbed intermediates
A) number of steps in the mechanism
B) the number of reacting species
C) the number of product species
D) the number of adsorbed intermediates

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
17
Consider the reaction
. Frumkin isotherm is used to describe the adsorption. The kinetic parameter values are k10 = 10-9 mol cm-2 s-1, b1 = 10 V-1, g = 15, 1 = -0.5 , k20 = 10-8 mol cm-2 s-1, 2 = +0.5 and = 10-8 mol cm-2.
-The maximum number of 'loops' that can appear in complex plane plots is _____________
. Frumkin isotherm is used to describe the adsorption. The kinetic parameter values are k10 = 10-9 mol cm-2 s-1, b1 = 10 V-1, g = 15, 1 = -0.5 , k20 = 10-8 mol cm-2 s-1, 2 = +0.5 and = 10-8 mol cm-2.
-The maximum number of 'loops' that can appear in complex plane plots is _____________

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
18
Consider the reaction
. Frumkin isotherm is used to describe the adsorption. The kinetic parameter values are k10 = 10-9 mol cm-2 s-1, b1 = 10 V-1, g = 15, 1 = -0.5 , k20 = 10-8 mol cm-2 s-1, 2 = +0.5 and = 10-8 mol cm-2.
-In the above question, the total number of electrical elements required to model the reaction (i.e., only the Faradaic part, without considering the double-layer capacitor or solution resistance) is ____
. Frumkin isotherm is used to describe the adsorption. The kinetic parameter values are k10 = 10-9 mol cm-2 s-1, b1 = 10 V-1, g = 15, 1 = -0.5 , k20 = 10-8 mol cm-2 s-1, 2 = +0.5 and = 10-8 mol cm-2.
-In the above question, the total number of electrical elements required to model the reaction (i.e., only the Faradaic part, without considering the double-layer capacitor or solution resistance) is ____

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
19
An impedance spectrum of an electrochemical reaction could be modeled well by the equivalent circuit given below. The solution resistance was negligible, mass transfer was rapid, and there was not film formation on the surface.
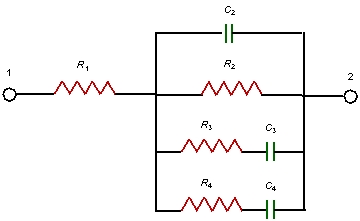 If a mechanism is proposed to explain the results, what is the minimum number of adsorbed intermediate species necessary to model the data? __________
If a mechanism is proposed to explain the results, what is the minimum number of adsorbed intermediate species necessary to model the data? __________
 If a mechanism is proposed to explain the results, what is the minimum number of adsorbed intermediate species necessary to model the data? __________
If a mechanism is proposed to explain the results, what is the minimum number of adsorbed intermediate species necessary to model the data? __________
Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
20
Choose the mechanism(s), which, for appropriate values of kinetic parameters and dc potentials, can yield negative differential impedance.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
21
Consider the following mechanism.
The faradaic impedance of this system is to be modeled using the Maxwell type circuit with resistances and capacitances. Apart from polarization resistance, how many Maxwell pairs are necessary to adequately model an impedance spectrum from this system? ________
At how many potentials should EIS be acquired to eliminate the possibility of an infinite number of solutions _________
The faradaic impedance of this system is to be modeled using the Maxwell type circuit with resistances and capacitances. Apart from polarization resistance, how many Maxwell pairs are necessary to adequately model an impedance spectrum from this system? ________
At how many potentials should EIS be acquired to eliminate the possibility of an infinite number of solutions _________

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
22
A Frumkin isotherm model will reduce to Langmuir isotherm when parameter "g" is _____

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
23
Temkin model describes the relationship between _______ and fractional surface coverage
A) rate constant
B) equilibrium constant
C) potential
D) current
A) rate constant
B) equilibrium constant
C) potential
D) current

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
24
Consider the reaction
The net reaction rate is given by
A)
B)
C)
D) none of the above
The net reaction rate is given by
A)
B)
C)
D) none of the above

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
25
The following impedance spectrum was obtained for an electrochemical system.
 Which of the following mechanisms can be considered to describe the electrochemical system?
Which of the following mechanisms can be considered to describe the electrochemical system?
A)
B)
C)
D)
 Which of the following mechanisms can be considered to describe the electrochemical system?
Which of the following mechanisms can be considered to describe the electrochemical system?A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
26
A unique method of representing impedance spectrum is
A) Voigt representation
B) Maxwell representation
C) Ladder representation
D) Zero-pole representation
A) Voigt representation
B) Maxwell representation
C) Ladder representation
D) Zero-pole representation

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
27
Consider the following elementary reaction
where M is metal and
is metal ion in solution, F denotes Faraday constant. The net faradaic current density due to metal dissolution is
A)
B)
C)
D)
where M is metal and
is metal ion in solution, F denotes Faraday constant. The net faradaic current density due to metal dissolution is
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
28
Consider the reaction given by .
-In standard notation, the fractional surface coverage ( ) of the adsorbed intermediate
is given by
A)
B)
C)
D)
-In standard notation, the fractional surface coverage ( ) of the adsorbed intermediate
is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
29
Consider the reaction given by .
-In the same mechanism, the steady-state fractional surface coverage of the intermediate is given by
A)
B)
C)
D)
-In the same mechanism, the steady-state fractional surface coverage of the intermediate is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
30
Consider the reaction given by .
-In the same mechanism, the faradaic current is given by
A)
B)
C)
D)
-In the same mechanism, the faradaic current is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
31
Consider the reaction given by .
-In the same mechanism, the value of
is given by
A)
B)
C)
D)
-In the same mechanism, the value of
is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
32
Consider the reaction given by .
-In the same mechanism, the faradaic impedance (ZF) is given by
A)
B)
C)
D)
-In the same mechanism, the faradaic impedance (ZF) is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
33
Consider the reaction given by .
-The complex plane plot of impedance spectra of the above reaction can exhibit at the most ______ loops
-The complex plane plot of impedance spectra of the above reaction can exhibit at the most ______ loops

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
34
Consider the reaction given by .
-For the above mechanism, the mid and low-frequency data, in the complex plane plot can exhibit
A) capacitive loop
B) inductive loop
C) Warburg behavior
D) none of the above
-For the above mechanism, the mid and low-frequency data, in the complex plane plot can exhibit
A) capacitive loop
B) inductive loop
C) Warburg behavior
D) none of the above

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
35
Negative real values of impedance indicate that
A) there is a problem in the measurement
B) when a potential is applied, current flows in the opposite direction
C) when potential is increased, current decreases
D) the phase difference between the potential and current is 180 °
A) there is a problem in the measurement
B) when a potential is applied, current flows in the opposite direction
C) when potential is increased, current decreases
D) the phase difference between the potential and current is 180 °

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
36
The polarization plot of an electrochemical system is given below. An impedance spectrum is acquired at some dc bias and shows hidden negative impedance. 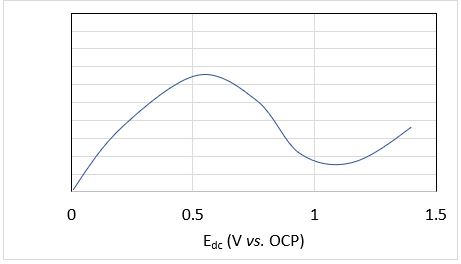 The dc bias at which the spectrum was acquired could be
The dc bias at which the spectrum was acquired could be
A) 0.4 V
B) 0.8 V
C) 1.2 V
D) none of the above
 The dc bias at which the spectrum was acquired could be
The dc bias at which the spectrum was acquired could beA) 0.4 V
B) 0.8 V
C) 1.2 V
D) none of the above

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
37
Consider the reaction
-The mass balance equation for fractional surface coverage of Aads is given by
A)
B)
C)
D)
-The mass balance equation for fractional surface coverage of Aads is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
38
Consider the reaction
-In the same reaction, the equation for fractional surface coverage of Aads is given by
A)
B)
C)
D)
-In the same reaction, the equation for fractional surface coverage of Aads is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
39
Consider the reaction
-In the same reaction, the faradaic current is given by
A)
B)
C)
D)
-In the same reaction, the faradaic current is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
40
Consider the reaction
-In the same reaction, the expression for
is given by
A)
B)
C)
D)
-In the same reaction, the expression for
is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
41
Consider the reaction
-In the same mechanism, the charge transfer resistance (Rt) is given by
A)
B)
C)
D)
-In the same mechanism, the charge transfer resistance (Rt) is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
42
Consider the reaction 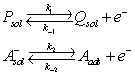
-If EIS is acquired for the above mechanism, the maximum number of loops that can manifest in a complex plane plot is _____

-If EIS is acquired for the above mechanism, the maximum number of loops that can manifest in a complex plane plot is _____

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
43
Consider the reaction 
-For the above mechanism, the number of electrical elements (including polarization resistance) required to model the Faradaic impedance is _____

-For the above mechanism, the number of electrical elements (including polarization resistance) required to model the Faradaic impedance is _____

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
44
Consider the reaction 
-The minimum number of dc potentials at which EIS must be acquired to avoid an infinite number of solutions is ______

-The minimum number of dc potentials at which EIS must be acquired to avoid an infinite number of solutions is ______

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
45
Consider the reaction
-The mass balance equation for fractional surface coverage of
is given by
A)
B)
C)
D)
-The mass balance equation for fractional surface coverage of
is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
46
Consider the reaction
-In the same reaction, the equation for fractional surface coverage of Aads is given by
A)
B)
C)
D)
-In the same reaction, the equation for fractional surface coverage of Aads is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
47
Consider the reaction
-In the same reaction, the faradaic current is given by
A)
B)
C)
D)
-In the same reaction, the faradaic current is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
48
Consider the reaction
-In the same reaction, the expression for
is given by
A)
B)
C)
D)
-In the same reaction, the expression for
is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
49
Consider the reaction
-In the same mechanism, the charge transfer resistance (Rt) is given by
A)
B)
C)
D)
-In the same mechanism, the charge transfer resistance (Rt) is given by
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
50
Consider the reaction 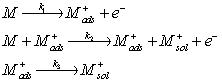
-If EIS is acquired for the above mechanism, the maximum number of loops that can manifest in a complex plane plot is _____
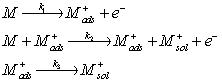
-If EIS is acquired for the above mechanism, the maximum number of loops that can manifest in a complex plane plot is _____

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
51
Consider the reaction 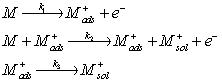
-For the above mechanism, the number of electrical elements (including polarization resistance) required to model the Faradaic impedance is _____
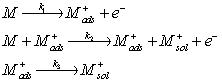
-For the above mechanism, the number of electrical elements (including polarization resistance) required to model the Faradaic impedance is _____

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
52
Consider the reaction 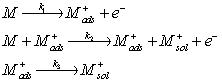
-The minimum number of dc potentials at which EIS must be acquired to avoid an infinite number of solutions is ______
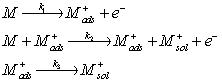
-The minimum number of dc potentials at which EIS must be acquired to avoid an infinite number of solutions is ______

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck
53
Consider the reaction
-Choose the mechanism(s), which, for appropriate values of kinetic parameters and dc potentials, can yield negative differential impedance.
A)
B)
C)
D)
-Choose the mechanism(s), which, for appropriate values of kinetic parameters and dc potentials, can yield negative differential impedance.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 53 flashcards in this deck.
Unlock Deck
k this deck



