Deck 3: Oscillations, Fluids, Waves, Temperature, Heat, and the First Law of Thermodynamics
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/13
Play
Full screen (f)
Deck 3: Oscillations, Fluids, Waves, Temperature, Heat, and the First Law of Thermodynamics
1
When the temperature of a copper penny is increased by 100 C its diameter increases by 0.17%. The area of one of its faces increases by:
A) 0.17%
B) 0.34%
C) 0.51%
D) 0.13%
E) 0.27%
A) 0.17%
B) 0.34%
C) 0.51%
D) 0.13%
E) 0.27%
0.34%
2
Object A, with heat capacity CA and initially at temperature TA, is placed in thermal contact with object B, with heat capacity CB and initially at temperature TB. The combination is thermally isolated. If the heat capacities are independent of the temperature and no phase changes occur, the final temperature of both objects is:
A) (CATA - CBTB)/(CA + CB)
B) (CATA + CBTB)/(CA + CB)
C) (CATA - CBTB)/(CA - CB)
D) (CA - CB)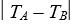
E) (CA + CB)11ef181b_aacf_e68f_b5ce_19b6b0f164d3_TB10137_11
A) (CATA - CBTB)/(CA + CB)
B) (CATA + CBTB)/(CA + CB)
C) (CATA - CBTB)/(CA - CB)
D) (CA - CB)
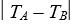
E) (CA + CB)11ef181b_aacf_e68f_b5ce_19b6b0f164d3_TB10137_11
(CATA + CBTB)/(CA + CB)
3
Two notes are an "octave" apart.The ratio of their frequencies is:
A) 8
B) 10
C)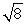
D) 2
E)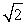
A) 8
B) 10
C)
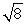
D) 2
E)
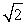
2
4
The Doppler shift formula for the frequency detected is
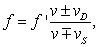
Where ƒ ' is the frequency emitted, v is the speed of sound, vD is the speed of the detector, and vs is the speed of the source. Suppose the source is traveling at 5 m/s away from the detector, the detector is traveling at 7 m/s toward the source, and there is a 3 m/s wind blowing from the source toward the detector. The values that should be substituted into the Doppler shift equation are:
A) vD = 7 m/s and vs = 5 m/s
B) vD = 10 m/s and vs = 8 m/s
C) vD = 4 m/s and vs = 2 m/s
D) vD = 10 m/s and vs = 2 m/s
E) vD = 4 m/s and vs = 8 m/s
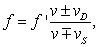
Where ƒ ' is the frequency emitted, v is the speed of sound, vD is the speed of the detector, and vs is the speed of the source. Suppose the source is traveling at 5 m/s away from the detector, the detector is traveling at 7 m/s toward the source, and there is a 3 m/s wind blowing from the source toward the detector. The values that should be substituted into the Doppler shift equation are:
A) vD = 7 m/s and vs = 5 m/s
B) vD = 10 m/s and vs = 8 m/s
C) vD = 4 m/s and vs = 2 m/s
D) vD = 10 m/s and vs = 2 m/s
E) vD = 4 m/s and vs = 8 m/s

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
5
Here are the equations for the three waves traveling on separate strings. Rank them according to the maxium transverse speed, least to greatest.
Wave 1: y(x,t) = (2.0 mm) sin [(4.0 m-1)x - (3.0 s-1)t]
Wave 2: y(x,t) = (1.0 mm) sin [(8.0 m-1)x - (4.0 s-1)t]
Wave 3: y(x,t) = (1.0 mm) sin [(4.0 m-1)x - (8.0 s-1)t]
A) 1, 2, 3
B) 1, 3, 2
C) 2, 1, 3
D) 2, 3, 1
E) 3, 1, 2
Wave 1: y(x,t) = (2.0 mm) sin [(4.0 m-1)x - (3.0 s-1)t]
Wave 2: y(x,t) = (1.0 mm) sin [(8.0 m-1)x - (4.0 s-1)t]
Wave 3: y(x,t) = (1.0 mm) sin [(4.0 m-1)x - (8.0 s-1)t]
A) 1, 2, 3
B) 1, 3, 2
C) 2, 1, 3
D) 2, 3, 1
E) 3, 1, 2

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
6
The mathematical forms for the three sinusoidal traveling waves are gives by
Wave 1: y(x,t) = (2 cm) sin (3x - 6t)
Wave 2: y(x,t) = (3 cm) sin (4x - 12t)
Wave 3: y(x,t) = (4 cm) sin (5x - 11t)
Where x is in meters and t is in seconds. Of these waves:
A) wave 1 has the greatest wave speed and the greatest maximum transverse string speed
B) wave 2 has the greatest wave speed and wave 1 has the greatest maxmium transverse string speed
C) wave 3 has the greatest wave speed and the greatest maximum transverse string speed
D) wave 2 has the greatest wave speed and wave 3 has the greatest maximum transverse string speed
E) wave 3 has the greatest wave speed and wave 2 has the greatest maximum transverse string speed
Wave 1: y(x,t) = (2 cm) sin (3x - 6t)
Wave 2: y(x,t) = (3 cm) sin (4x - 12t)
Wave 3: y(x,t) = (4 cm) sin (5x - 11t)
Where x is in meters and t is in seconds. Of these waves:
A) wave 1 has the greatest wave speed and the greatest maximum transverse string speed
B) wave 2 has the greatest wave speed and wave 1 has the greatest maxmium transverse string speed
C) wave 3 has the greatest wave speed and the greatest maximum transverse string speed
D) wave 2 has the greatest wave speed and wave 3 has the greatest maximum transverse string speed
E) wave 3 has the greatest wave speed and wave 2 has the greatest maximum transverse string speed

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
7
Two traveling sinusoidal waves interfere to produce a wave with the mathematical form
Y(x,t) = ym sin(kx +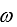 t + ).
t + ).
If the value of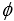 is appropriately chosen, the two waves might be:
is appropriately chosen, the two waves might be:
A)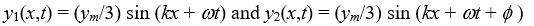
B)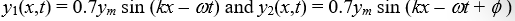
C)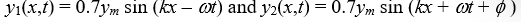
D)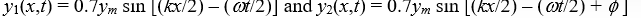
E)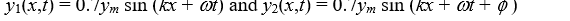
Y(x,t) = ym sin(kx +
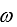 t + ).
t + ).If the value of
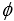 is appropriately chosen, the two waves might be:
is appropriately chosen, the two waves might be:A)
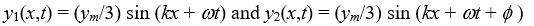
B)
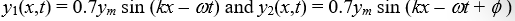
C)
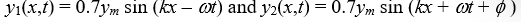
D)
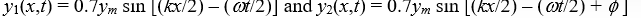
E)
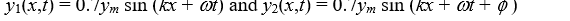

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
8
Which of the following represents a standing wave?
A) y = (6.0 mm)sin[(3.0 m-1)x + (2.0 s-1)t] - (6.0 mm)cos[(3.0 m-1)x + 2.0]
B) y = (6.0 mm)cos[(3.0 m-1)x - (2.0 s-1)t] + (6.0 mm)cos[(2.0 s-1)t + 3.0 m-1)x]
C) y = (6.0 mm)cos[(3.0 m-1)x - (2.0 s-1)t] - (6.0 mm)sin[(2.0 s-1)t - 3.0]
D) y = (6.0 mm)sin[(3.0 m-1)x - (2.0 s-1)t] - (6.0 mm)cos[(2.0 s-1)t + 3.0 m-1)x]
E) y = (6.0 mm)sin[(3.0 m-1)x] + (6.0 mm)cos[(2.0 s-1)t]
A) y = (6.0 mm)sin[(3.0 m-1)x + (2.0 s-1)t] - (6.0 mm)cos[(3.0 m-1)x + 2.0]
B) y = (6.0 mm)cos[(3.0 m-1)x - (2.0 s-1)t] + (6.0 mm)cos[(2.0 s-1)t + 3.0 m-1)x]
C) y = (6.0 mm)cos[(3.0 m-1)x - (2.0 s-1)t] - (6.0 mm)sin[(2.0 s-1)t - 3.0]
D) y = (6.0 mm)sin[(3.0 m-1)x - (2.0 s-1)t] - (6.0 mm)cos[(2.0 s-1)t + 3.0 m-1)x]
E) y = (6.0 mm)sin[(3.0 m-1)x] + (6.0 mm)cos[(2.0 s-1)t]

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
9
If the length of a simple pendulum is doubled, its period will:
A) halve
B)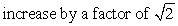
C)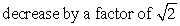
D) double
E) remain the same
A) halve
B)
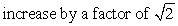
C)
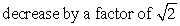
D) double
E) remain the same

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
10
Five hoops are each pivoted at a point on the rim and allowed to swing as physical pendulums. The masses and radii are
Hoop 1: M = 150g and R = 50 cm
Hoop 2: M = 200g and R = 40 cm
Hoop 3: M = 250g and R = 30 cm
Hoop 4: M = 300g and R = 20 cm
Hoop 5: M = 350g and R = 10 cm
Order the hoops according to the periods of their motions, smallest to largest.
A) 1, 2, 3, 4, 5
B) 5, 4, 3, 2, 1
C) 1, 2, 3, 5, 4
D) 1, 2, 5, 4, 3
E) 5, 4, 1, 2, 3
Hoop 1: M = 150g and R = 50 cm
Hoop 2: M = 200g and R = 40 cm
Hoop 3: M = 250g and R = 30 cm
Hoop 4: M = 300g and R = 20 cm
Hoop 5: M = 350g and R = 10 cm
Order the hoops according to the periods of their motions, smallest to largest.
A) 1, 2, 3, 4, 5
B) 5, 4, 3, 2, 1
C) 1, 2, 3, 5, 4
D) 1, 2, 5, 4, 3
E) 5, 4, 1, 2, 3

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
11
The density of water is 1.0 g/cm3. The density of the oil in the left column of the U-tube shown below is:
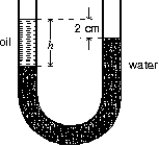
A) 0.20 g/cm3
B) 0.80 g/cm3
C) 1.0 g/cm3
D) 1.3 g/cm3
E) 5.0 g/cm3

A) 0.20 g/cm3
B) 0.80 g/cm3
C) 1.0 g/cm3
D) 1.3 g/cm3
E) 5.0 g/cm3

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
12
An incompressible liquid flows along the pipe as shown. The ratio of the speeds v2/v1 is:
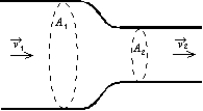
A) A1/A2
B) A2/A1
C)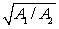
D)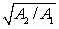
E) v1/v2

A) A1/A2
B) A2/A1
C)
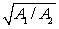
D)
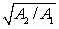
E) v1/v2

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
13
A water line enters a house 2.0 m below ground. A smaller diameter pipe carries water to a faucet 5.0 m above ground, on the second floor. Water flows at 2.0 m/s in the main line and at 7.0 m/s on the second floor. Take the density of water to be 1.0 x103 kg/m3. The diffenernce in pressure in the main line is 2.0 x 105 Pa, then the pressure on the second floor is:
A) 7.5 x 104 Pa with the main line at the higher pressure
B) 2.65 x104 Pa with the main line at the higher pressure
C) 7.5 x 104 Pa with the main line at the lower pressure
D) 2.65 x 104 Pa with the main line at the lower pressure
E) 9.4 x 104 Pa with the main line at the higher pressure
A) 7.5 x 104 Pa with the main line at the higher pressure
B) 2.65 x104 Pa with the main line at the higher pressure
C) 7.5 x 104 Pa with the main line at the lower pressure
D) 2.65 x 104 Pa with the main line at the lower pressure
E) 9.4 x 104 Pa with the main line at the higher pressure

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck



