Deck 6: Some Processes Are Irreversible Thermal Physics
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/151
Play
Full screen (f)
Deck 6: Some Processes Are Irreversible Thermal Physics
1
Consider a rectangular book. The book is (approximately) symmetric for rotations around an axis that goes diagonally from one corner to the other
T
2
Consider a system consisting of two Einstein solids and in thermal contact. Assume that we know the number of atoms in each solid and . What do we know about the system if we also know the total energy in each of the two objects?
A) Its macrostate
B) Its microstate
C) Its macropartition
D) Its microstate and macropartition
E) Its macrostate and macropartition
F) Its macrostate and microstate
G) Its macrostate, microstate, and macropartition
A) Its macrostate
B) Its microstate
C) Its macropartition
D) Its microstate and macropartition
E) Its macrostate and macropartition
F) Its macrostate and microstate
G) Its macrostate, microstate, and macropartition
Its macrostate and macropartition
3
Consider a system consisting of two Einstein solids and in thermal contact. Assume that we know the number of atoms in each solid and . What do we know about the system if we also know the total energy of the combined system?
A) Its macrostate
B) Its microstate
C) Its macropartition
D) Its microstate and macropartition
E) Its macrostate and macropartition
F) Its macrostate and microstate
G) Its macrostate, microstate, and macropartition
A) Its macrostate
B) Its microstate
C) Its macropartition
D) Its microstate and macropartition
E) Its macrostate and macropartition
F) Its macrostate and microstate
G) Its macrostate, microstate, and macropartition
Its macrostate
4
What is the crucial characteristic of an Einstein solid that makes it easier to analyze in the context of this chapter than most other kinds of thermodynamic systems?
A) The atoms are arranged in a regular, cubic lattice.
B) The atoms are identical.
C) Its microstates are comparatively easy to count.
D) Each atom's energy levels are equally spaced.
E) is always reasonably small.
F) Other (specify).
A) The atoms are arranged in a regular, cubic lattice.
B) The atoms are identical.
C) Its microstates are comparatively easy to count.
D) Each atom's energy levels are equally spaced.
E) is always reasonably small.
F) Other (specify).

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
5
We can ignore an Einstein solid's zero-point energy because
A) it is zero.
B) it never changes in any thermal interaction.
C) it is insignificant compared to the solid's total energy.
D) it is just a quantum-mechanical effect.
E) other (specify).
A) it is zero.
B) it never changes in any thermal interaction.
C) it is insignificant compared to the solid's total energy.
D) it is just a quantum-mechanical effect.
E) other (specify).

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
6
Which of the following statements is true?
A) There are always many microstates in a macrostate.
B) All accessible macrostates are equally probable.
C) All microstates of a system are equally probable.
D) All accessible macropartitions are equally probable.
E) When two objects in thermal contact are isolated from everything else, their macrostates cannot change.
F) None of the above.
A) There are always many microstates in a macrostate.
B) All accessible macrostates are equally probable.
C) All microstates of a system are equally probable.
D) All accessible macropartitions are equally probable.
E) When two objects in thermal contact are isolated from everything else, their macrostates cannot change.
F) None of the above.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
7
Suppose that we increase an -atom Einstein solid's value of from to . By what factor does the value of its multiplicity increase? (Choose whichever result is the closest, assuming that and .)
A)
B)
C)
D)
E)
F)
A)
B)
C)
D)
E)
F)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
8
In the situation shown in figure T2.5c, the width of the bell curve at half its peak value is . If we multiply , , and by a factor of 100 , then the peak's width (as you can check) is about . Suppose that we increase each solid's and the system's total energy by another factor of to create solids containing of a mole of atoms (still pretty small objects by everyday standards). Assuming that the trend continues, what will be the approximate width of the combined system's probability bell curve as a fraction of ?
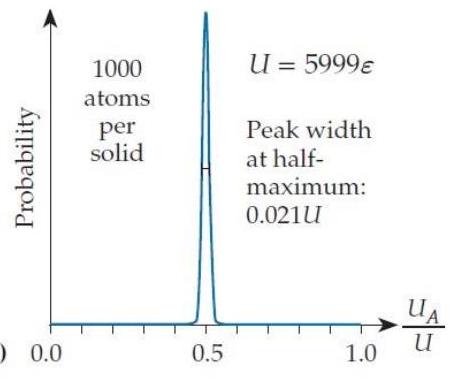
A)
(c) 0.0
B)
Figure T2.5
C)
D)
E)
F)
. Some other factor (specify).

A)
(c) 0.0
B)
Figure T2.5
C)
D)
E)
F)
. Some other factor (specify).

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
9
Suppose that you use a super-fast computer to measure a system's macropartition a billion times a second. What is the approximate probability of the least-probable macropartition that you might plausibly see in your lifetime? Select the closest response.
A) 1 chance in a million
B) 1 chance in a billion
C) 1 chance in a trillion
D) 1 chance in
E) 1 chance in
F) 1 chance in
A) 1 chance in a million
B) 1 chance in a billion
C) 1 chance in a trillion
D) 1 chance in
E) 1 chance in
F) 1 chance in

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
10
Consider the system where (see figure T2.6). Suppose that we can measure each object's energy to within (the size of one bin). Once the system has reached a macropartition in the most probable bin, it will never spontaneously move to a macropartition outside that bin.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
11
Which of the systems listed below is the largest, having and for which there is a better than 1 in a billion chance of seeing one or the other solid having zero energy? (Hint: Using StatMech to check cases is probably the easiest approach.)
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
12
A hot object is placed in contact with a cold object. We observe that heat flows spontaneously from the hot object to the cold object, but not in the other direction. According to the argument in this chapter, this is so because
A) this increases the entropies of both objects.
B) this decreases the entropy of the combined system.
C) the system will tend to evolve toward macropartitions that have more microstates.
D) and
E) and
A) this increases the entropies of both objects.
B) this decreases the entropy of the combined system.
C) the system will tend to evolve toward macropartitions that have more microstates.
D) and
E) and

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
13
Consider an object (object ) whose multiplicity is always 1 , no matter how much energy you put into it. If you put a very large amount of energy into such an object, and place it into thermal contact with an Einstein solid (object ) having the same number of atoms but much less energy, what will happen? (Hint: Imagine a macropartition table for such a pair of objects.)
A) Energy flows from to until has very little energy.
B) Energy flows from to until has very little energy.
C) Energy flows from to until both objects have the same energy.
D) No energy will flow between and at all.
E) Something else happens (describe).
A) Energy flows from to until has very little energy.
B) Energy flows from to until has very little energy.
C) Energy flows from to until both objects have the same energy.
D) No energy will flow between and at all.
E) Something else happens (describe).

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
14
In a given macropartition, the total entropy of a system comprised of two or more parts is always equal to the sum of the entropies of those parts.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
15
. What is In ? (Select the closest response.)
A) 100
B) 10
C) 4.6
D)
A) 100
B) 10
C) 4.6
D)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
16
The entropy of a certain macropartition of a combined system is . The entropy of another macropartition is . How much more likely is the system to be in the second macropartition than the first? (Select the closest response.)
A) 2 times
B) times
C) times
D) times
E) ; so the two macropartitions have basically the same probability
A) 2 times
B) times
C) times
D) times
E) ; so the two macropartitions have basically the same probability

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
17
Which system has the greater entropy, a puddle of water sitting on a table next to a chaotic scattering of salt crystals or the same amount of salt dissolved in an evenly distributed way in the puddle? Be prepared to describe your reasoning.
A) The puddle and the scattered crystals.
B) The puddle with the dissolved salt.
C) Both have the same entropy.
D) One needs detailed models of water and salt to say.
A) The puddle and the scattered crystals.
B) The puddle with the dissolved salt.
C) Both have the same entropy.
D) One needs detailed models of water and salt to say.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
18
Object A's entropy increases quite a bit when we give it a certain tiny amount of energy, whereas object B's entropy increases by a much smaller amount with the same gift. Which has the higher temperature?
A) Object
B) Object
C) One cannot say without more information.
A) Object
B) Object
C) One cannot say without more information.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
19
As a normal object's thermal energy goes to zero, the slope of a graph of its entropy versus
A) approaches infinity.
B) approaches some positive value less than infinity.
C) approaches zero.
D) approaches some finite negative value.
E) approaches negative infinity.
F) depends entirely on the object's properties.
A) approaches infinity.
B) approaches some positive value less than infinity.
C) approaches zero.
D) approaches some finite negative value.
E) approaches negative infinity.
F) depends entirely on the object's properties.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
20
Consider a system whose multiplicity is 1 , no matter how much energy one puts into it. What is such a system's temperature?
A) Zero
B) Positive
C) Negative
D) Undefined
A) Zero
B) Positive
C) Negative
D) Undefined

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
21
Suppose that an object's entropy increases from zero when its thermal energy is to some maximum value when , and then goes back to zero when (as shown in the drawing below). The greatest thermal energy that such an object could have when in thermal equilibrium with a normal object is closest to which of the following?
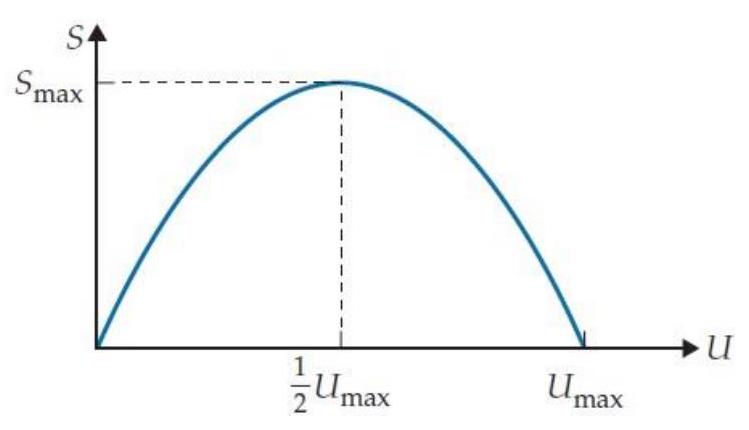
A) 0
B)
C)
D)
E)
F) Other (specify)

A) 0
B)
C)
D)
E)
F) Other (specify)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
22
If an object has a multiplicity that decreases as its thermal energy increases, its temperature would
A) be always zero.
B) be always positive (and nonzero).
C) be negative (and nonzero).
D) increase as increases.
E) decrease as increases.
A) be always zero.
B) be always positive (and nonzero).
C) be negative (and nonzero).
D) increase as increases.
E) decrease as increases.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
23
Suppose that an atom has two energy levels separated by an energy difference . When such atoms are in thermal contact with a reservoir at temperature , there is roughly 1 atom in the higher state for every 4 in the lower state. What is the approximate ratio of to ?
A)
B) 4
C) In 4
D)
E)
F)
G) Other (specify)
A)
B) 4
C) In 4
D)
E)
F)
G) Other (specify)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
24
Suppose that an atom has exactly two energy levels separated by an energy difference . When such atoms are in contact with a reservoir at a temperature , the ratio of the number of atoms in the higher level to the number in the lower level is . If we increase the temperature to , the numerical value of this ratio
A) increases.
B) decreases.
C) remains the same.
A) increases.
B) decreases.
C) remains the same.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
25
The quantity in the expression depends on the temperature of the reservoir.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
26
The quantity in the expression must be
A) always greater than 1 .
B) always less than 1 .
C) It could be either greater than or less than 1.
A) always greater than 1 .
B) always less than 1 .
C) It could be either greater than or less than 1.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
27
As temperatures become large, the denominator in equation becomes
Equation
A) very large.
B) very small.
C) approximately 1.
D) approximately -1 .
Equation
A) very large.
B) very small.
C) approximately 1.
D) approximately -1 .

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
28
Suppose that the energy difference between adjacent energy levels for an oscillator in an Einstein solid is . At room temperature, the value of is
A) quite a bit smaller than 1
B) about equal to 0.5 .
C) about equal to 1 .
D) about equal to 2 .
E) quite a bit larger than 2.
F) (One doesn't have enough information to tell.)
A) quite a bit smaller than 1
B) about equal to 0.5 .
C) about equal to 1 .
D) about equal to 2 .
E) quite a bit larger than 2.
F) (One doesn't have enough information to tell.)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
29
Suppose that the energy difference between adjacent energy levels for an oscillator in an Einstein solid is . We would expect the average energy of this oscillator when it is in contact with a reservoir at room temperature to be
A) negligible.
B) smaller than .
C) about equal to .
D) about equal to .
E) significantly larger than .
F) One doesn't have enough information to tell.
A) negligible.
B) smaller than .
C) about equal to .
D) about equal to .
E) significantly larger than .
F) One doesn't have enough information to tell.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
30
To calculate an object's heat capacity, we take its specific heat and
A) multiply by the object's mass .
B) divide by the object's mass .
C) multiply by the molar mass of the object's molecules.
D) divide by the molar mass of the object's molecules.
E) multiply by the mass of one molecule in the object.
F) divide by the mass of one molecule in the object.
G) do something else (specify).
A) multiply by the object's mass .
B) divide by the object's mass .
C) multiply by the molar mass of the object's molecules.
D) divide by the molar mass of the object's molecules.
E) multiply by the mass of one molecule in the object.
F) divide by the mass of one molecule in the object.
G) do something else (specify).

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
31
Suppose that the temperature is about for a certain Einstein solid. The probability that an oscillator in that solid will have one unit of energy is closest to
(Hint: See equation for how to calculate .)
Equation T4.32:
A) 1
B)
C)
D)
E)
(Hint: See equation for how to calculate .)
Equation T4.32:
A) 1
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
32
is much smaller for lead than for iron. Which therefore has the greater heat capacity per atom at
-(a) ?
A) Lead
B) Iron
C) Both should be about the same.
-(a) ?
A) Lead
B) Iron
C) Both should be about the same.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
33
is much smaller for lead than for iron. Which therefore has the greater heat capacity per atom at
-(b) ?
A) Lead
B) Iron
C) Both should be about the same.
-(b) ?
A) Lead
B) Iron
C) Both should be about the same.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
34
At a sufficiently high temperature, an oscillator in an Einstein solid is more likely to have one unit of energy than no energy at all.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
35
Suppose that the forces holding an atom in its position in a crystal are the same for two different monatomic solids, but the mass of an atom is larger for solid than for solid . Which has the larger value of ?
A) Solid
B) Solid
C) Both have about the same value of .
A) Solid
B) Solid
C) Both have about the same value of .

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
36
If we increase a one-dimensional container's length by a factor of 100 , by what factor does the value of change at room temperature?
A) 10
B) 100
C) 10,000
D)
E)
F)
A) 10
B) 100
C) 10,000
D)
E)
F)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
37
Using an integral to calculate a sum over (where is an integer) is a good approximation when
A) the summed values are individually small.
B) the value of varies only very slowly with .
C) the value of goes to zero as goes to infinity.
D) the value of goes to zero as goes to zero.
E) All of the conditions above are true.
A) the summed values are individually small.
B) the value of varies only very slowly with .
C) the value of goes to zero as goes to infinity.
D) the value of goes to zero as goes to zero.
E) All of the conditions above are true.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
38
Which of the following energy storage modes for a gas molecule "switch on" fully at
-(a) the lowest temperature?
A) The molecule's kinetic energy
B) The molecule's rotational energy
C) The molecule's vibrational energy
-(a) the lowest temperature?
A) The molecule's kinetic energy
B) The molecule's rotational energy
C) The molecule's vibrational energy

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
39
Which of the following energy storage modes for a gas molecule "switch on" fully at
-(b) the highest temperature?
A) The molecule's kinetic energy
B) The molecule's rotational energy
C) The molecule's vibrational energy
-(b) the highest temperature?
A) The molecule's kinetic energy
B) The molecule's rotational energy
C) The molecule's vibrational energy

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
40
The heat capacity of any energy storage mode with quantized energy levels goes to zero as the temperature goes to zero.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
41
An atom of helium can store energy by bumping an electron from its lowest orbital energy state to a higher orbital energy level. In particular, moving an electron from the lowest state to the next-lowest state would store an energy of . Why can we ignore this energy storage mode when calculating the heat capacity of helium gas?
A) This mode is "frozen" out at normal temperatures.
B) Collisions between atoms can't influence the energy levels of electrons inside the atoms.
C) This storage mode is completely independent of the kinetic and/or rotational energy modes.
D) Only modes involving helium molecules count.
E) We have ignored this mode only for simplicity's sake.
F) Some other reason (specify).
A) This mode is "frozen" out at normal temperatures.
B) Collisions between atoms can't influence the energy levels of electrons inside the atoms.
C) This storage mode is completely independent of the kinetic and/or rotational energy modes.
D) Only modes involving helium molecules count.
E) We have ignored this mode only for simplicity's sake.
F) Some other reason (specify).

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
42
(a) The average -velocity of molecules in a container of gas at rest is zero.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
43
(b) The average molecular speed is zero.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
44
If the speed of a molecule in a container doubles (other things remaining the same), the average pressure that this molecule exerts on the container wall
A) remains the same.
B) doubles.
C) quadruples.
D) One does not have enough information to say.
A) remains the same.
B) doubles.
C) quadruples.
D) One does not have enough information to say.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
45
Suppose that when we add to 1 mol of a certain gas, its temperature is observed to increase by . Which of the following statements is true?
A) This gas is monatomic.
B) This gas is diatomic.
C) This gas is polyatomic.
D) One cannot determine the type of gas without information that we are not given.
E) The results described are impossible.
A) This gas is monatomic.
B) This gas is diatomic.
C) This gas is polyatomic.
D) One cannot determine the type of gas without information that we are not given.
E) The results described are impossible.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
46
The ideal gas model assumes that molecules have infinitesimal size. Consider one molecule bouncing around in the container. As the molecule's size increases relative to the size of the container, how will the average pressure that it exerts change, other things being held constant?
A) increases.
B) does not change.
C) decreases.
D) One does not have enough information to say.
A) increases.
B) does not change.
C) decreases.
D) One does not have enough information to say.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
47
Two identical rooms, and , are sealed except for an open doorway between them. Room is warmer than . Which room has the greater number of molecules? (Hint: How will the pressures in the two rooms compare?)
A) Room A
B) Room B
C) Both have the same number of molecules.
A) Room A
B) Room B
C) Both have the same number of molecules.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
48
One mole of hydrogen gas and mole of helium gas are mixed in a container and maintained at a fixed temperature . How does the total pressure exerted by the hydrogen molecules compare to the total pressure exerted by the helium molecules? Note that a molecule (atom) of helium has twice the mass of an molecule.
A)
B)
C)
D)
E)
F) Other (specify)
A)
B)
C)
D)
E)
F) Other (specify)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
49
molecules of a monatomic gas are mixed with the same number of molecules of a diatomic gas in a container, and the mixture is held at a constant temperature (which is about equal to room temperature). The molecules of gas have mass , and the molecules of gas have mass Which gas has In each case, choose from one of the four answers below.
-(a) the greater value of ,
A) The quantity is greater for gas .
B) The quantity is greater for gas .
C) The quantity is the same for both gases.
D) One does not know enough to compare the quantities.
-(a) the greater value of ,
A) The quantity is greater for gas .
B) The quantity is greater for gas .
C) The quantity is the same for both gases.
D) One does not know enough to compare the quantities.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
50
molecules of a monatomic gas are mixed with the same number of molecules of a diatomic gas in a container, and the mixture is held at a constant temperature (which is about equal to room temperature). The molecules of gas have mass , and the molecules of gas have mass Which gas has In each case, choose from one of the four answers below.
-(b) the greater average kinetic energy per molecule,
A) The quantity is greater for gas .
B) The quantity is greater for gas .
C) The quantity is the same for both gases.
D) One does not know enough to compare the quantities.
-(b) the greater average kinetic energy per molecule,
A) The quantity is greater for gas .
B) The quantity is greater for gas .
C) The quantity is the same for both gases.
D) One does not know enough to compare the quantities.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
51
molecules of a monatomic gas are mixed with the same number of molecules of a diatomic gas in a container, and the mixture is held at a constant temperature (which is about equal to room temperature). The molecules of gas have mass , and the molecules of gas have mass Which gas has In each case, choose from one of the four answers below.
-(c) the greater total thermal energy , and
A) The quantity is greater for gas .
B) The quantity is greater for gas .
C) The quantity is the same for both gases.
D) One does not know enough to compare the quantities.
-(c) the greater total thermal energy , and
A) The quantity is greater for gas .
B) The quantity is greater for gas .
C) The quantity is the same for both gases.
D) One does not know enough to compare the quantities.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
52
molecules of a monatomic gas are mixed with the same number of molecules of a diatomic gas in a container, and the mixture is held at a constant temperature (which is about equal to room temperature). The molecules of gas have mass , and the molecules of gas have mass Which gas has In each case, choose from one of the four answers below.
-(d) the greater pressure ?
A) The quantity is greater for gas .
B) The quantity is greater for gas .
C) The quantity is the same for both gases.
D) One does not know enough to compare the quantities.
-(d) the greater pressure ?
A) The quantity is greater for gas .
B) The quantity is greater for gas .
C) The quantity is the same for both gases.
D) One does not know enough to compare the quantities.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
53
When counting particle-in-a-box quantum states in three dimensions, why do we divide the "volume" of only one eighth of a full spherical shell by the "volume" per quantum state? Why not the full shell's volume?
A) Molecules in a gas can only have positive momentum components.
B) A single quantum energy state embraces both positive and negative values for each momentum component.
C) A particle's momentum magnitude must be positive.
D) The factor of 2 in the expression already counts both possible momentum signs.
E) We really do include the full shell: the diagram only shows of the shell to make it easier to draw.
F) Some other reason (specify).
A) Molecules in a gas can only have positive momentum components.
B) A single quantum energy state embraces both positive and negative values for each momentum component.
C) A particle's momentum magnitude must be positive.
D) The factor of 2 in the expression already counts both possible momentum signs.
E) We really do include the full shell: the diagram only shows of the shell to make it easier to draw.
F) Some other reason (specify).

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
54
Physically, why is a gas molecule's speed more likely to be near than near zero?
A) There are fewer quantum states with smaller speeds than larger speeds.
B) The Boltzmann factor has a larger value near than near .
C) We know that , and is closer to than zero is.
A) There are fewer quantum states with smaller speeds than larger speeds.
B) The Boltzmann factor has a larger value near than near .
C) We know that , and is closer to than zero is.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
55
Physically, why is a gas molecule's speed more likely to be near than near ?
A) There are fewer quantum states for larger speeds than there are at speeds near .
B) The Boltzmann factor is larger at than at .
C) We know that , and is closer to than is.
A) There are fewer quantum states for larger speeds than there are at speeds near .
B) The Boltzmann factor is larger at than at .
C) We know that , and is closer to than is.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
56
How should compare to for an ideal gas?
A)
B)
C)
D) One cannot tell without careful calculation.
A)
B)
C)
D) One cannot tell without careful calculation.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
57
Which of the speeds listed below is the lowest speed where the probability of a gas molecule having that speed is negligible compared to that for ("negligible" meaning less than of its value at ).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
58
How would the values of for carbon dioxide gas compare to that of nitrogen gas at a given temperature? (Hint: Carbon dioxide gas has a molar mass of ; nitrogen has a molar mass of .)
A) will be larger for carbon dioxide.
B) will be larger for nitrogen.
C) will be equal in both cases.
D) One does not have enough information to tell for sure.
A) will be larger for carbon dioxide.
B) will be larger for nitrogen.
C) will be equal in both cases.
D) One does not have enough information to tell for sure.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
59
How does the number of molecules with speed compare to the number having speed ?
A) Fewer molecules have .
B) The same number of molecules are in each range.
C) More molecules have .
D) The answer depends on the temperature.
A) Fewer molecules have .
B) The same number of molecules are in each range.
C) More molecules have .
D) The answer depends on the temperature.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
60
The number of photons per second radiated by a blackbody is proportional to what power of ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
61
Doubling a blackbody's temperature doubles the number of photons it emits per second.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
62
Will a blackbody at temperature emit more photons per second with energy or with energy ? (Note: The amount of energy emitted per time is not the same as the number of photons emitted per time, though these quantities are related.)
A) It will emit more photons with the lower energy.
B) It will emit more photons with the higher energy.
C) The number of photons emitted will be the same.
D) One has no way of telling.
A) It will emit more photons with the lower energy.
B) It will emit more photons with the higher energy.
C) The number of photons emitted will be the same.
D) One has no way of telling.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
63
Will a blackbody at temperature emit more photons-perBsecond with energy or with energy ? (Note: The amount of energy emitted per time is not the same as the number of photons emitted per time, though these quantities are related.)
A) It will emit more photons with the lower energy.
B) It will emit more photons with the higher energy.
C) The number of photons emitted will be the same.
D) One has no way of telling.
A) It will emit more photons with the lower energy.
B) It will emit more photons with the higher energy.
C) The number of photons emitted will be the same.
D) One has no way of telling.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
64
A perfectly reflective piece of metal at room temperature would not radiate any photons,

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
65
An object strongly reflects red light, but readily absorbs all other colors of light. Relative to the number of thermal photons of red light emitted by a black body at the same temperature , our object's thermal emission rate of photons of red light will be
A) enhanced.
B) Suppressed.
C) unchanged.
D) The answer depends on the value of .
A) enhanced.
B) Suppressed.
C) unchanged.
D) The answer depends on the value of .

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
66
Suppose that a star has twice the surface temperature of the sun and also twice the radius. How many times larger is the rate at which this star emits energy compared to the rate at which the sun emits energy?
A) The star radiates at the same rate as the sun.
B) 2 times
C) 4 times
D) 8 times
E) 16 times
F) 32 times
G) 64 times
A) The star radiates at the same rate as the sun.
B) 2 times
C) 4 times
D) 8 times
E) 16 times
F) 32 times
G) 64 times

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
67
Suppose that we double the electrical power flowing into the filament of an incandescent bulb. By what factor will the most probable photon energy increase?
A)
B)
C)
D) 2
E) 4
F) 8
G) 16
A)
B)
C)
D) 2
E) 4
F) 8
G) 16

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
68
A gas with a pressure of is in a container that is a cube measuring on a side. If we move one wall in 1 millimeter ( ), does work flow into (A) or out of (B) the gas? What is the magnitude of W?
A)
B)
C)
D)
E)
F) Other (specify)
A)
B)
C)
D)
E)
F) Other (specify)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
69
Which of the following triplets of macroscopic variables would not adequately specify a gas's macrostate?
A)
B)
C)
D)
E)
F)
A)
B)
C)
D)
E)
F)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
70
Problems T7T. 3 through T7T. 7 refer to the following cyclic gas process:
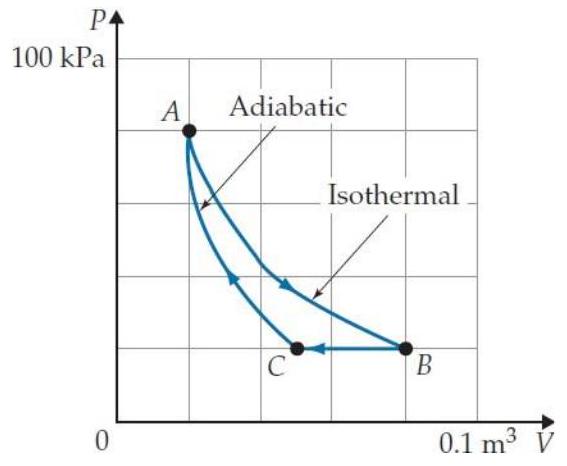
-The process shown is
A) an isochoric process.
B) an isothermal process.
C) an isobaric process.
D) an adiabatic process.
E) an isometric process.
F) none of the above.

-The process shown is
A) an isochoric process.
B) an isothermal process.
C) an isobaric process.
D) an adiabatic process.
E) an isometric process.
F) none of the above.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
71
Problems T7T. 3 through T7T. 7 refer to the following cyclic gas process:

-What are the signs of , and for the process ?
A)
B)
C),,+- 0
D),,++ 0
E),,-+ 0
F) Other (specify)

-What are the signs of , and for the process ?
A)
B)
C),,+- 0
D),,++ 0
E),,-+ 0
F) Other (specify)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
72
Problems T7T. 3 through T7T. 7 refer to the following cyclic gas process:

-What are the signs of , and for the process ?
A)
B)
C),,+- 0
D),,++ 0
E),,-+ 0
F) Other (specify)

-What are the signs of , and for the process ?
A)
B)
C),,+- 0
D),,++ 0
E),,-+ 0
F) Other (specify)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
73
Problems T7T. 3 through T7T. 7 refer to the following cyclic gas process:

-What are the signs of , and for the process ?
A)
B)
C),,+- 0
D),,++ 0
E),,-+ 0
F) Other (specify)

-What are the signs of , and for the process ?
A)
B)
C),,+- 0
D),,++ 0
E),,-+ 0
F) Other (specify)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
74
Problems T7T. 3 through T7T. 7 refer to the following cyclic gas process:

-In static equilibrium, any excess charge on a conductor is uniformly spread on its surface.

-In static equilibrium, any excess charge on a conductor is uniformly spread on its surface.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
75
Problems T7T. 3 through T7T. 7 refer to the following cyclic gas process:

-When an object slides down a frictionless incline, The interaction does not change the object's momentum

-When an object slides down a frictionless incline, The interaction does not change the object's momentum

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
76
Suppose that a bubble of helium (a monatomic gas) rising from the bottom of the ocean expands in volume by a factor of 8 by the time it reaches the surface [where the pressure is 1 atmosphere (atm)]. Assume that the bubble rises so fast that it expands essentially adiabatically. What was the pressure on the gas at the depth where it formed (in atmospheres)?
A)
B)
C)
D)
E) 32 atm
F) Other (specify)
A)
B)
C)
D)
E) 32 atm
F) Other (specify)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
77
If the temperature of the bubble described in problem T7T. 8 was when it formed, what is its approximate final temperature when it reaches the surface?
A)
B)
C)
D)
E)
F) Other (specify)
A)
B)
C)
D)
E)
F) Other (specify)

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
78
During an adiabatic compression, does a gas's temperature increase, decrease, or remain the same?
A) It increases.
B) It decreases.
C) It remains the same.
A) It increases.
B) It decreases.
C) It remains the same.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
79
Does the pressure of a gas decrease more rapidly or less rapidly when expanding adiabatically than it would when expanding isothermally?
A) More rapidly.
B) Less rapidly.
C) The pressure decreases at the same rate in both cases.
D) The pressure increases in an adiabatic expansion.
E) The pressure increases in an isothermal expansion.
A) More rapidly.
B) Less rapidly.
C) The pressure decreases at the same rate in both cases.
D) The pressure increases in an adiabatic expansion.
E) The pressure increases in an isothermal expansion.

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck
80
Water actually contracts when it is warmed near its freezing point. In this range, is water's heat capacity at constant volume (A) greater than, (B) less than, or (C) equal to its heat capacity at constant pressure?
A) greater than
B) less than
C) equal to
A) greater than
B) less than
C) equal to

Unlock Deck
Unlock for access to all 151 flashcards in this deck.
Unlock Deck
k this deck



