Deck 3: Utility
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/57
Play
Full screen (f)
Deck 3: Utility
1
If someone has a utility function U = 2 min{x, y}, then x and y are perfect complements for that person.
True
2
A person with the utility function U(x1, x2) = min{x1 + 2x2, 2x1 + x2} has convex but not strictly convex preferences.
False
3
If one utility function is a monotonic transformation of another, then the former must assign a higher utility number to every bundle than the latter.
False
4
Ike's utility function is U(x, y) = 25xy. He has 12 units of good x and 8 units of good y. Ben's utility function for the same two goods is U(x, y) = 4x + 4y. Ben has 9 units of x and 13 units of y.
A) Ike prefers Ben's bundle to his own bundle, but Ben prefers his own bundle to Ike's.
B) Ben prefers Ike's bundle to his own, but Ike prefers his own bundle to Ben's.
C) Each prefers the other's bundle to his own.
D) Neither prefers the other's bundle to his own.
E) Since they have different preferences, there is not enough information to determine who envies whom.
A) Ike prefers Ben's bundle to his own bundle, but Ben prefers his own bundle to Ike's.
B) Ben prefers Ike's bundle to his own, but Ike prefers his own bundle to Ben's.
C) Each prefers the other's bundle to his own.
D) Neither prefers the other's bundle to his own.
E) Since they have different preferences, there is not enough information to determine who envies whom.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
5
Fiery Demon is a rotgut whisky made in Kentucky. Smoothy is an unblended malt whisky imported from Scotland. Ed regards these brands as perfect substitutes. When he goes into a bar, he sometimes buys only Fiery Demon. Other times he buys only Smoothy. This shows that Ed has unstable preferences.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
6
Mr. Surly consumes only two goods and hates them both. His utility function is U(x, y) = 2max{x, y}. Mr. Surly has (weakly) convex preferences.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
7
A person with the utility function U(x, y) = 10 + y2 + x has convex preferences.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
8
A person with the utility function U(x, y) = y + x2 has convex preferences.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
9
Alice's utility function is U(x, y) = x2y. Steve's utility function is U(x, y) = x2y + 2x. Alice and Steve have the same preferences since Steve's utility function is a monotonic transformation of Alice's.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
10
Angela's utility function is U(x1, x2) = (x1 + x2)3. Her indifference curves are downward-sloping, parallel straight lines.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
11
Quasilinear preferences are homothetic when the optimal amount of good 1 is not affordable.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
12
A person with utility function U(x, y) = 5 + y2 + 2x has nonconvex preferences.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
13
Jeans utility function is U(x, y) = x + y 2 - y. If we draw her indifference curves with x on the horizontal axis and y on the vertical axis, then these indifference curves are everywhere downward sloping and get flatter as one moves from left to right.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
14
A consumer has preferences represented by the utility function U(x1, x2) = 10(x21 + 2x1x2 + x22) - 50. For this consumer, goods 1 and 2 are perfect substitutes.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
15
The utility function U(x1, x2) = 2lnx1 + 3lnx2 represents Cobb-Douglas preferences.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
16
With quasilinear preferences, the slope of indifference curves is constant along all rays through the origin.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
17
Wanda Lott has the utility function U(x, y) = max{x, y}. Wanda's preferences are convex.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
18
Henrietta's utility function is U(x1, x2) = x1x2. She has diminishing marginal rate of substitution between goods 1 and 2.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
19
Mark strictly prefers consumption bundle A to consumption bundle B and weakly prefers bundle B to bundle A. These preferences can be represented by a utility function.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
20
Maximilian consumes two goods, x and y. His utility function is U(x, y) = max{x, y}. Therefore x and y are perfect substitutes for Max.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
21
Jean-Pierre has preferences represented by the utility function U(x, y) = min{ 2x + y, x + 6y}. If x is on the horizontal axis and y is on the vertical axis, what is the slope of his indifference curve at the point (7, 7)?
A) -1/2
B) -6/2
C) -1/6
D) -2
E) -7/7
A) -1/2
B) -6/2
C) -1/6
D) -2
E) -7/7

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
22
Angela has preferences represented by the utility function U(x, y) = 2x + 2y. She consumes 10 units of good x and 6 units of good y. If her consumption of good x is lowered to 4, how many units of y must she have in order to be exactly as well off as before?
A) 14
B) 13
C) 12
D) 15
E) None of the above.
A) 14
B) 13
C) 12
D) 15
E) None of the above.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
23
Harmon's utility function is U(x1, x2) = x1x2. His income is $100. The price of good 2 is p2 = 4. Good 1 is priced as follows. The first 15 units cost $4 per unit and any additional units cost $2 per unit. What consumption bundle does Harmon choose?
A) (12.5, 12.5)
B) (25, 12.5)
C) (12.5, 25)
D) (15, 10)
E) None of the above.
A) (12.5, 12.5)
B) (25, 12.5)
C) (12.5, 25)
D) (15, 10)
E) None of the above.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
24
Doreen has preferences represented by the utility function U(x, y) = 10x + 5y. She consumes 10 units of good x and 9 units of good y. If her consumption of good x is lowered to 1, how many units of y must she have in order to be exactly as well off as before?
A) 30
B) 30
C) 27
D) 18
E) None of the above.
A) 30
B) 30
C) 27
D) 18
E) None of the above.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
25
Emily's utility function is U(x, y) = 3min{x, y} + y. If we draw her indifference curves with x on the horizontal axis and y on the vertical axis, these indifference curves are
A) made up of two line segments that meet where x = 4y. One of these line segments is vertical and the other has slope -1.
B) L-shaped with kinks where x = 4y.
C) L-shaped with kinks where x = y.
D) made up of two line segments that meet where x = y. One of these line segments is horizontal and the other has slope -3.
E) V-shaped with kinks where x = 3y.
A) made up of two line segments that meet where x = 4y. One of these line segments is vertical and the other has slope -1.
B) L-shaped with kinks where x = 4y.
C) L-shaped with kinks where x = y.
D) made up of two line segments that meet where x = y. One of these line segments is horizontal and the other has slope -3.
E) V-shaped with kinks where x = 3y.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
26
Charles's utility function is U(x, y) = xy. Anne's utility function is U(x, y) = 1,000xy. Diana's utility function is -xy. Elizabeth's utility function is 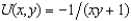 . Fergie's utility function is xy - 10,000. Margaret's utility function is
. Fergie's utility function is xy - 10,000. Margaret's utility function is 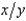 . Philip's utility function is x(y + 1). (The goods x and y are two very expensive goods. We leave you to speculate about what they are.) Which of these persons have the same preferences as Charles?
. Philip's utility function is x(y + 1). (The goods x and y are two very expensive goods. We leave you to speculate about what they are.) Which of these persons have the same preferences as Charles?
A) Everybody except Diana
B) Anne and Fergie
C) Anne, Fergie, and Elizabeth
D) None of them
E) All of them
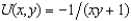 . Fergie's utility function is xy - 10,000. Margaret's utility function is
. Fergie's utility function is xy - 10,000. Margaret's utility function is 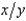 . Philip's utility function is x(y + 1). (The goods x and y are two very expensive goods. We leave you to speculate about what they are.) Which of these persons have the same preferences as Charles?
. Philip's utility function is x(y + 1). (The goods x and y are two very expensive goods. We leave you to speculate about what they are.) Which of these persons have the same preferences as Charles?A) Everybody except Diana
B) Anne and Fergie
C) Anne, Fergie, and Elizabeth
D) None of them
E) All of them

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
27
The absolute value of Mar's MRS at his current consumption bundle is greater than 3. (That is, 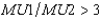 ). Mars has convex preferences and is currently consuming positive amounts of both goods.
). Mars has convex preferences and is currently consuming positive amounts of both goods.
A) Taking away some of good 1 and giving Mars 3 units of good 2 for each unit of good 1 taken away will necessarily make him worse off.
B) Taking away some of good 1 and giving Mars 3 units of good 2 for each unit of good 1 taken away will necessarily make him better off.
C) Giving Mars some of good 1 and taking away 3 units of good 2 for each unit of good 1 he is given will necessarily make him worse off.
D) Giving Mars some of good 1 and taking away 3 units of good 2 for each unit of good 1 he is given will necessarily make him better off.
E) More than one of the above is true.
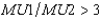 ). Mars has convex preferences and is currently consuming positive amounts of both goods.
). Mars has convex preferences and is currently consuming positive amounts of both goods.A) Taking away some of good 1 and giving Mars 3 units of good 2 for each unit of good 1 taken away will necessarily make him worse off.
B) Taking away some of good 1 and giving Mars 3 units of good 2 for each unit of good 1 taken away will necessarily make him better off.
C) Giving Mars some of good 1 and taking away 3 units of good 2 for each unit of good 1 he is given will necessarily make him worse off.
D) Giving Mars some of good 1 and taking away 3 units of good 2 for each unit of good 1 he is given will necessarily make him better off.
E) More than one of the above is true.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
28
Mac's utility function is U(x, y) = max{ 2x - y, 2y - x}.
A) Mac's preferences are quasilinear.
B) If Mac has more x than y, any increase in his consumption of y would lower his utility.
C) If Mac has more x than y, a decrease in his consumption of y would raise his utility.
D) Mac always prefers more of each good to less.
E) Goods x and y are perfect substitutes.
A) Mac's preferences are quasilinear.
B) If Mac has more x than y, any increase in his consumption of y would lower his utility.
C) If Mac has more x than y, a decrease in his consumption of y would raise his utility.
D) Mac always prefers more of each good to less.
E) Goods x and y are perfect substitutes.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
29
Henry's utility function is x2 + 16xw + 64w2, where x is his consumption of x and w is his consumption of w.
A) Henry's preferences are nonconvex.
B) Henry's indifference curves are straight lines.
C) Henry has a bliss point.
D) Henry's indifference curves are hyperbolas.
E) None of the above.
A) Henry's preferences are nonconvex.
B) Henry's indifference curves are straight lines.
C) Henry has a bliss point.
D) Henry's indifference curves are hyperbolas.
E) None of the above.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
30
Raymond's preferences are represented by the utility function U(x, y) = 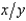 if y > 0 and U(x, y) = 0 if y = 0.
if y > 0 and U(x, y) = 0 if y = 0.
A) Raymond has indifference curves that are rectangular hyperbolas.
B) Raymond prefers more of each good to less.
C) Raymond has quasilinear preferences.
D) Raymond has a bliss point.
E) Raymond has indifference curves that are upward-sloping straight lines if y > 0.
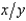 if y > 0 and U(x, y) = 0 if y = 0.
if y > 0 and U(x, y) = 0 if y = 0.A) Raymond has indifference curves that are rectangular hyperbolas.
B) Raymond prefers more of each good to less.
C) Raymond has quasilinear preferences.
D) Raymond has a bliss point.
E) Raymond has indifference curves that are upward-sloping straight lines if y > 0.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
31
Jim's utility function is U(x, y) = xy. Jerry's utility function is U(x, y) = 1,000xy + 2,000. Tammy's utility function is U(x, y) = xy(1 - xy). Oral's utility function is - 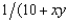 . Billy's utility function is
. Billy's utility function is 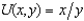 . Pat's utility function is U(x, y) = -xy.
. Pat's utility function is U(x, y) = -xy.
A) No two of these people have the same preferences.
B) They all have the same preferences except for Billy.
C) Jim, Jerry, and Pat all have the same indifference curves, but Jerry and Oral are the only ones with the same preferences as Jim.
D) Jim, Tammy, and Oral all have the same preferences.
E) None of the above.
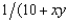 . Billy's utility function is
. Billy's utility function is 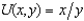 . Pat's utility function is U(x, y) = -xy.
. Pat's utility function is U(x, y) = -xy.A) No two of these people have the same preferences.
B) They all have the same preferences except for Billy.
C) Jim, Jerry, and Pat all have the same indifference curves, but Jerry and Oral are the only ones with the same preferences as Jim.
D) Jim, Tammy, and Oral all have the same preferences.
E) None of the above.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
32
Isabella's utility function is U(x, y) = 4min{x, y} + y. If we draw her indifference curves with x on the horizontal axis and y on the vertical axis, these indifference curves are
A) L-shaped with kinks where x = y.
B) made up of two line segments that meet where x = y. One of these line segments is horizontal and the other has slope -4.
C) L-shaped with kinks where x = 5y.
D) made up of two line segments that meet where x = 5y. One of these line segments is vertical and the other has slope -1.
E) V-shaped with kinks where x = 4y.
A) L-shaped with kinks where x = y.
B) made up of two line segments that meet where x = y. One of these line segments is horizontal and the other has slope -4.
C) L-shaped with kinks where x = 5y.
D) made up of two line segments that meet where x = 5y. One of these line segments is vertical and the other has slope -1.
E) V-shaped with kinks where x = 4y.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
33
Ike's utility function is U(x, y) = xy. Ike consumes 2 units of x and 8 units of y.
A) Ike would be willing to make small exchanges of x for y in which he would give up 4 units of x for every unit of y he got.
B) Ike would be willing to trade away all of his x for y as long as he got more than 4 units of y for every unit of x he gave up.
C) Ike will always be willing to make trades at any price if he does not have equal amounts of the two goods.
D) Ike likes x and y equally well so he is always willing to exchange 1 unit of either good for more than 1 unit of the other.
E) None of the above.
A) Ike would be willing to make small exchanges of x for y in which he would give up 4 units of x for every unit of y he got.
B) Ike would be willing to trade away all of his x for y as long as he got more than 4 units of y for every unit of x he gave up.
C) Ike will always be willing to make trades at any price if he does not have equal amounts of the two goods.
D) Ike likes x and y equally well so he is always willing to exchange 1 unit of either good for more than 1 unit of the other.
E) None of the above.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
34
Tim has preferences represented by the utility function U(x, y) = min{ 6x + y, x + 2y}. If x is on the horizontal axis and y is on the vertical axis, what is the slope of his indifference curve at the point (8, 9)?
A) -6
B) -2/6
C) -1/2
D) -1/6.
E) -8/9
A) -6
B) -2/6
C) -1/2
D) -1/6.
E) -8/9

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
35
Waldo's utility function is U(x, y) = xy. Waldo consumes 5 units of x and 25 units of y.
A) Waldo would be willing to make small exchanges of x for y in which he would give up 5 units of x for every unit of y he got.
B) Waldo would be willing to trade away all of his x for y as long as he got more than 5 units of y for every unit of x he gave up.
C) Waldo likes x and y equally well so he is always willing to exchange 1 unit of either good for more than 1 unit of the other.
D) Waldo will always be willing to make trades at any price if he does not have equal amounts of the two goods.
E) None of the above.
A) Waldo would be willing to make small exchanges of x for y in which he would give up 5 units of x for every unit of y he got.
B) Waldo would be willing to trade away all of his x for y as long as he got more than 5 units of y for every unit of x he gave up.
C) Waldo likes x and y equally well so he is always willing to exchange 1 unit of either good for more than 1 unit of the other.
D) Waldo will always be willing to make trades at any price if he does not have equal amounts of the two goods.
E) None of the above.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
36
Josephine's utility function is U(x, y) = y + 5x.5. She has 1 unit of x and 2 units of y. If her consumption of x is reduced to zero, how much y must she have in order to be exactly as well off as before?
A) 14 units
B) 9 units
C) 11 units
D) 7 units
E) None of the above.
A) 14 units
B) 9 units
C) 11 units
D) 7 units
E) None of the above.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
37
Ernie's utility function is U(x, y) = 32xy. He has 10 units of good x and 8 units of good y. Waldo's utility function for the same two goods is U(x, y) = 3x + 5y. Waldo has 9 units of x and 13 units of y.
A) Waldo prefers Ernie's bundle to his own, but Ernie prefers his own bundle to Waldo's.
B) Each prefers the other's bundle to his own.
C) Neither prefers the other's bundle to his own.
D) Ernie prefers Waldo's bundle to his own bundle, but Waldo prefers his own bundle to Ernie's.
E) Since they have different preferences, there is not enough information to determine who envies whom.
A) Waldo prefers Ernie's bundle to his own, but Ernie prefers his own bundle to Waldo's.
B) Each prefers the other's bundle to his own.
C) Neither prefers the other's bundle to his own.
D) Ernie prefers Waldo's bundle to his own bundle, but Waldo prefers his own bundle to Ernie's.
E) Since they have different preferences, there is not enough information to determine who envies whom.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
38
Oswald Odd consumes only goods 1 and 2. His utility function is U(x1, x2) = x1 + x2 + min{x1, x2}. Each of Oswald's indifference curves is
A) L-shaped.
B) made up of three line segments with slopes -2, -1, and -.
C) made up of two line segments with slopes -2 and -.
D) is smooth and has no kinks.
E) is a diamond-shaped figure consisting of four line segments.
A) L-shaped.
B) made up of three line segments with slopes -2, -1, and -.
C) made up of two line segments with slopes -2 and -.
D) is smooth and has no kinks.
E) is a diamond-shaped figure consisting of four line segments.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
39
Janet consumes x1 and x2 together in fixed proportions. She always consumes 2 units of x1 for every unit x2. One utility function that describes her preferences is
A) U(x1, x2) = 2x1x2.
B) U(x1, x2) = 2x1 + x2.
C) U(x1, x2) = x1 + 2x2.
D) U(x1, x2) = min{ 2x1, x2}.
E) U(x1, x2) = min{x1, 2x2}.
A) U(x1, x2) = 2x1x2.
B) U(x1, x2) = 2x1 + x2.
C) U(x1, x2) = x1 + 2x2.
D) U(x1, x2) = min{ 2x1, x2}.
E) U(x1, x2) = min{x1, 2x2}.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
40
Molly's utility function is U(x, y) = y + 4x.5. She has 25 units of x and 12 units of y. If her consumption of x is reduced to 0, how many units of y would she need in order to be exactly as well off as before?
A) 48
B) 37
C) 32
D) 112
E) None of the above.
A) 48
B) 37
C) 32
D) 112
E) None of the above.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
41
A consumer has a utility function of the form U(x, y) =x a + y b, where both a and b are nonnegative. What additional restrictions on the values of the parameters a and b are imposed by each of the following assumptions?
a. Preferences are quasilinear and convex, and x is a normal good.
b. Preferences are homothetic.
c. Preferences are homothetic and convex.
d. Goods x and y are perfect substitutes.
a. Preferences are quasilinear and convex, and x is a normal good.
b. Preferences are homothetic.
c. Preferences are homothetic and convex.
d. Goods x and y are perfect substitutes.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
42
Phil Rupp's sister Ethel has the utility function U(x, y) = min{ 4x + y, 5y}. Where x is measured on the horizontal axis and y on the vertical axis, her indifference curves consist of a
A) vertical line segment and a horizontal line segment which meet in a kink along the line y = 4x.
B) vertical line segment and a horizontal line segment which meet in a kink along the line x = 4y.
C) horizontal line segment and a negatively sloped line segment which meet in a kink along the line x = y.
D) positively sloped line segment and a negatively sloped line segment which meet along the line x = y.
E) horizontal line segment and a positively sloped line segment which meet in a kink along the line x = 4y.
A) vertical line segment and a horizontal line segment which meet in a kink along the line y = 4x.
B) vertical line segment and a horizontal line segment which meet in a kink along the line x = 4y.
C) horizontal line segment and a negatively sloped line segment which meet in a kink along the line x = y.
D) positively sloped line segment and a negatively sloped line segment which meet along the line x = y.
E) horizontal line segment and a positively sloped line segment which meet in a kink along the line x = 4y.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
43
Jim's utility function is U(x, y) = xy. Jerry's utility function is U(x, y) = 1,000xy + 2,000. Tammy's utility function is U(x, y) = xy(1 - xy). Oral's utility function is 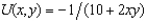 . Marjoe's utility function is U(x, y) = x(y + 1,000). Pat's utility function is U(x, y) = 0.5xy - 10,000. Billy's utility function is U(x, y) =
. Marjoe's utility function is U(x, y) = x(y + 1,000). Pat's utility function is U(x, y) = 0.5xy - 10,000. Billy's utility function is U(x, y) = 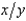 . Francis's utility function is U(x, y) = -xy.
. Francis's utility function is U(x, y) = -xy.
a. Who has the same preferences as Jim?
b. Who has the same indifference curves as Jim?
c. Explain why the answers to (a) and (b) differ.
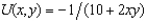 . Marjoe's utility function is U(x, y) = x(y + 1,000). Pat's utility function is U(x, y) = 0.5xy - 10,000. Billy's utility function is U(x, y) =
. Marjoe's utility function is U(x, y) = x(y + 1,000). Pat's utility function is U(x, y) = 0.5xy - 10,000. Billy's utility function is U(x, y) = 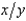 . Francis's utility function is U(x, y) = -xy.
. Francis's utility function is U(x, y) = -xy. a. Who has the same preferences as Jim?
b. Who has the same indifference curves as Jim?
c. Explain why the answers to (a) and (b) differ.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
44
Ambrose has the utility function 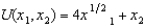 . If Ambrose is initially consuming 64 units of nuts and 10 units of berries, then what is the largest number of berries that he would be willing to give up in return for an additional 17 units of nuts?
. If Ambrose is initially consuming 64 units of nuts and 10 units of berries, then what is the largest number of berries that he would be willing to give up in return for an additional 17 units of nuts?
A) 9
B) 19
C) 4
D) 2
E) 1
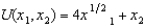 . If Ambrose is initially consuming 64 units of nuts and 10 units of berries, then what is the largest number of berries that he would be willing to give up in return for an additional 17 units of nuts?
. If Ambrose is initially consuming 64 units of nuts and 10 units of berries, then what is the largest number of berries that he would be willing to give up in return for an additional 17 units of nuts?A) 9
B) 19
C) 4
D) 2
E) 1

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
45
Joe Bob's cousin Leonard consumes goods 1 and 2. Leonard thinks that 2 units of good 1 is always a perfect substitute for 3 units of good 2. Which of the following utility functions is the only one that would not represent Leonard's preferences?
A) U(x1, x2) = 3x1 + 2x2 + 1,000.
B) U(x1, x2) = 9x21 + 12x1x2 + 4x22.
C) U(x1, x2) = min{ 3x1, 2x2}.
D) U(x1, x2) = 30x1 + 20x2 - 10,000.
E) More than one of the above does not represent Leonard's preferences.
A) U(x1, x2) = 3x1 + 2x2 + 1,000.
B) U(x1, x2) = 9x21 + 12x1x2 + 4x22.
C) U(x1, x2) = min{ 3x1, 2x2}.
D) U(x1, x2) = 30x1 + 20x2 - 10,000.
E) More than one of the above does not represent Leonard's preferences.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
46
Use separate graphs to draw indifference curves for each of the following utility functions:
a. U(x, y) = min{2x + y,2y + x}.
b. U(x, y) = max{2x + y,2y + x}.
c. U(x, y) = x + min{x, y}.
In which of these cases are preferences convex?
a. U(x, y) = min{2x + y,2y + x}.
b. U(x, y) = max{2x + y,2y + x}.
c. U(x, y) = x + min{x, y}.
In which of these cases are preferences convex?

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
47
Charlie's utility function is U(A, B) = AB, where A and B are the numbers of apples and bananas, respectively, that he consumes. When Charlie is consuming 15 apples and 90 bananas, if we put apples on the horizontal axis and bananas on the vertical axis, the slope of his indifference curve at his current consumption is
A)215
)b. -6.
C) -12.
D) -1/6.
E) -1/12.
A)215
)b. -6.
C) -12.
D) -1/6.
E) -1/12.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
48
Joe Bob's cousin Peter consumes goods 1 and 2. Peter thinks that 4 units of good 1 is always a perfect substitute for 2 units of good 2. Which of the following utility functions is the only one that would not represent Peter's preferences?
A) U(x1, x2) = min{ 2x1, 4x2}.
B) U(x1, x2) = 20x1 + 40x2 - 10,000.
C) U(x1, x2) = 2x1 + 4x2 + 1,000.
D) U(x1, x2) = 4x21 + 16x1x2 + 16x22.
E) More than one of the above does not represent Peter's preferences.
A) U(x1, x2) = min{ 2x1, 4x2}.
B) U(x1, x2) = 20x1 + 40x2 - 10,000.
C) U(x1, x2) = 2x1 + 4x2 + 1,000.
D) U(x1, x2) = 4x21 + 16x1x2 + 16x22.
E) More than one of the above does not represent Peter's preferences.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
49
Ambrose has the utility function 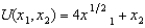 . If Ambrose is initially consuming 25 units of nuts and 17 units of berries, then what is the largest number of berries that he would be willing to give up in return for an additional 39 units of nuts?
. If Ambrose is initially consuming 25 units of nuts and 17 units of berries, then what is the largest number of berries that he would be willing to give up in return for an additional 39 units of nuts?
A) 8
B) 12
C) 25
D) 6
E) 3
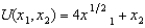 . If Ambrose is initially consuming 25 units of nuts and 17 units of berries, then what is the largest number of berries that he would be willing to give up in return for an additional 39 units of nuts?
. If Ambrose is initially consuming 25 units of nuts and 17 units of berries, then what is the largest number of berries that he would be willing to give up in return for an additional 39 units of nuts?A) 8
B) 12
C) 25
D) 6
E) 3

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
50
Charlie has the utility function U(xA, xB) = xAxB. His indifference curve passing through 35 apples and 18 bananas will also pass through the point where he consumes 5 apples and
A) 131 bananas.
B) 137 bananas.
C) 21 bananas.
D) 42 bananas.
E) 126 bananas.
A) 131 bananas.
B) 137 bananas.
C) 21 bananas.
D) 42 bananas.
E) 126 bananas.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
51
Victor Finick likes to have the same amount of x as he has of y. His utility function is U(x, y) = min{2x- y, 2y- x}.
a. Draw the indifference curve for Victor that passes through the bundle (0, 0) and the indifference curve that passes through (4, 4). (Hint: Each indifference curve is the intersection of two line segments.)
b. If Victor has a bundle that he likes better than (0, 0) and his consumption of both goods is doubled, is Victor better off?
c. Does Victor always prefer more of either good to less?
a. Draw the indifference curve for Victor that passes through the bundle (0, 0) and the indifference curve that passes through (4, 4). (Hint: Each indifference curve is the intersection of two line segments.)
b. If Victor has a bundle that he likes better than (0, 0) and his consumption of both goods is doubled, is Victor better off?
c. Does Victor always prefer more of either good to less?

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
52
Phil Rupp's sister Ethel has the utility function U(x, y) = min{ 5x + y, 6y}. Where x is measured on the horizontal axis and y on the vertical axis, her indifference curves consist of a
A) horizontal line segment and a negatively sloped line segment which meet in a kink along the line x = y.
B) vertical line segment and a horizontal line segment which meet in a kink along the line y = 5x.
C) positively sloped line segment and a negatively sloped line segment which meet along the line x = y.
D) vertical line segment and a horizontal line segment which meet in a kink along the line x = 5y.
E) horizontal line segment and a positively sloped line segment which meet in a kink along the line x = 5y.
A) horizontal line segment and a negatively sloped line segment which meet in a kink along the line x = y.
B) vertical line segment and a horizontal line segment which meet in a kink along the line y = 5x.
C) positively sloped line segment and a negatively sloped line segment which meet along the line x = y.
D) vertical line segment and a horizontal line segment which meet in a kink along the line x = 5y.
E) horizontal line segment and a positively sloped line segment which meet in a kink along the line x = 5y.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
53
Charlie has the utility function U(xA, xB) = xAxB. His indifference curve passing through 32 apples and 8 bananas will also pass through the point where he consumes 4 apples and
A) 16 bananas.
B) 32 bananas.
C) 68 bananas.
D) 72 bananas.
E) 64 bananas.
A) 16 bananas.
B) 32 bananas.
C) 68 bananas.
D) 72 bananas.
E) 64 bananas.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
54
Use separate graphs to sketch two indifference curves for people with each of the following utility functions:
a. U(x, y) = x + 2y.
b. U(x, y) = min{x,2y}.
c. U(x, y) = max{x,2y}.
a. U(x, y) = x + 2y.
b. U(x, y) = min{x,2y}.
c. U(x, y) = max{x,2y}.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
55
Harry Mazzola has the utility function U(x1, x2) = min{x1 + 2x2, 2x1 + x2}. He has $40 to spend on corn chips and french fries. If the price of corn chips is $1 per unit and the price of french fries is $4, then Harry will
A) consume at least as many french fries as corn chips but might consume both.
B) consume at least as many corn chips as french fries but might consume both.
C) definitely spend all of his income on corn chips.
D) definitely spend all of his income on french fries.
E) consume equal amounts of french fries and corn chips.
A) consume at least as many french fries as corn chips but might consume both.
B) consume at least as many corn chips as french fries but might consume both.
C) definitely spend all of his income on corn chips.
D) definitely spend all of his income on french fries.
E) consume equal amounts of french fries and corn chips.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
56
Charlie's utility function is U(A, B) = AB, where A and B are the numbers of apples and bananas, respectively, that he consumes. When Charlie is consuming 20 apples and 80 bananas, if we put apples on the horizontal axis and bananas on the vertical axis, the slope of his indifference curve at his current consumption is
A) -8.
B) -1/4.
C) -20.
D) -4.
E) -1/8.
A) -8.
B) -1/4.
C) -20.
D) -4.
E) -1/8.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck
57
Harry Mazzola has the utility function U(x1, x2) = min{x1 + 2x2, 2x1 + x2}. He has $40 to spend on corn chips and french fries. If the price of corn chips is $3 per unit and the price of french fries is $4, then Harry will
A) definitely spend all of his income on corn chips.
B) definitely spend all of his income on french fries.
C) consume at least as many corn chips as french fries but might consume both.
D) consume at least as many french fries as corn chips but might consume both.
E) consume equal amounts of french fries and corn chips.
A) definitely spend all of his income on corn chips.
B) definitely spend all of his income on french fries.
C) consume at least as many corn chips as french fries but might consume both.
D) consume at least as many french fries as corn chips but might consume both.
E) consume equal amounts of french fries and corn chips.

Unlock Deck
Unlock for access to all 57 flashcards in this deck.
Unlock Deck
k this deck



