
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Edition 3ISBN: 978-9352863501
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Edition 3ISBN: 978-9352863501 Exercise 35
Y i ,i = 1,..., n , are i.i.d. Bernoulli random variables with p = 0.4. Let
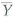 denote the sample mean.
denote the sample mean.
a. Use the central limit to compute approximations for
i. Pr(
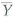 0.43) when n = 100.
0.43) when n = 100.
ii. Pr(
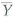 0.37) when n = 400.
0.37) when n = 400.
b. How large would n need to be to ensure that Pr(0.39
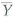 0.41) 0.95 (Use the central limit theorem to compute an approximate answer.)
0.41) 0.95 (Use the central limit theorem to compute an approximate answer.)
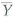 denote the sample mean.
denote the sample mean.a. Use the central limit to compute approximations for
i. Pr(
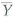 0.43) when n = 100.
0.43) when n = 100.ii. Pr(
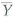 0.37) when n = 400.
0.37) when n = 400.b. How large would n need to be to ensure that Pr(0.39
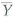 0.41) 0.95 (Use the central limit theorem to compute an approximate answer.)
0.41) 0.95 (Use the central limit theorem to compute an approximate answer.)Explanation
It is provided that
are i.i.d. Bernou...
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Why don’t you like this exercise?
Other Minimum 8 character and maximum 255 character
Character 255



