
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Edition 3ISBN: 978-9352863501
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Edition 3ISBN: 978-9352863501 Exercise 13
The moving average model of order q has the form
 ,
,
where e t is a serially uncorrelated random variable with mean 0 and variance
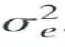 .
.
a. Show that E ( Y t ) = ß 0.
b. Show that the variance of Y t is var
 .
.
c. Show that for j q.
d. Suppose that q = 1. Derive the autocovariances for Y.
 ,
,where e t is a serially uncorrelated random variable with mean 0 and variance
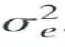 .
. a. Show that E ( Y t ) = ß 0.
b. Show that the variance of Y t is var
 .
.c. Show that for j q.
d. Suppose that q = 1. Derive the autocovariances for Y.
Explanation
a) The moving average model has the equa...
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Why don’t you like this exercise?
Other Minimum 8 character and maximum 255 character
Character 255



