Chat with AI
Introduction to Econometrics 3rd Edition by James Stock, James Stock
Edition 3ISBN: 978-9352863501Introduction to Econometrics 3rd Edition by James Stock, James Stock
Edition 3ISBN: 978-9352863501 Exercise 9
Consider the regression model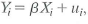 
where u i , and X i satisfy the assumptions in Key Concept 4.3. Let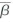
denote an estimator of that is constructed as 
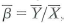where 
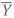and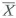 
are the sample means of Y i and X i , respectively.
a. Show that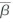
is a linear function of Y₁ , Y₂ ,..., Y n.
b. Show that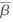
is conditionally unbiased. 
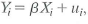
where u i , and X i satisfy the assumptions in Key Concept 4.3. Let
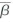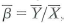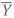denote an estimator of that is constructed as where and 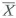are the sample means of Y i and X i , respectively.a. Show that
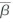is a linear function of Y₁ , Y₂ ,..., Y n. b. Show that
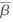is conditionally unbiased. Explanation
Verified
Verified
Regression analysis is a statistical ana...
Introduction to Econometrics 3rd Edition by James Stock, James Stock
Why don’t you like this exercise?
Other Minimum 8 character and maximum 255 character
Character 255
