Deck 7: A: Random Variables and Discrete Probability Distributions

Question 1

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
A table,formula,or graph that shows all possible values a random variable can assume,together with their associated probabilities,is referred to as probability distribution.

Answer 1

True

Question 2

The weighted average of the possible values that a random variable X can assume,where the weights are the probabilities of occurrence of those values,is referred to as the:

A) variance.
B) standard deviation.
C) expected value.
D) None of these choices.

Answer 2

C

Question 3

Which of the following are required conditions for the distribution of a discrete random variable X that can assume values x_i?

A) 0 $\le$ p(x_i) $\le$ 1 for all x_i
B) $$\sum _ { \text {all } x _ { 1 } } p \left( x _ { i } \right) = 1$$
C) Both a and b are required conditions.
D) Neither a nor b are required conditions.

Answer 3

Both a and b are required conditions.

Question 4

Another name for the mean of a probability distribution is its expected value.

Question 5

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
The mean of a discrete probability distribution for X is the sum of all possible values of X,divided by the number of possible values of X.

Question 6

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
The time required to drive from New York to New Mexico is a discrete random variable.

Question 7

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
The number of homeless people in Boston is an example of a discrete random variable.

Question 8

A table,formula,or graph that shows all possible values a random variable can assume,together with their associated probabilities,is called a(n):

A) probability distribution.
B) discrete random variable.
C) expected value of a discrete random variable.
D) None of these choices.

Question 9

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
Given that X is a discrete random variable,then the laws of expected value and variance can be applied to show that E(X + 5)= E(X)+ 5,and V(X + 5)= V(X)+ 25.

Question 10

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
The number of home insurance policy holders is an example of a discrete random variable

Question 11

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
The amount of milk consumed by a baby in a day is an example of a discrete random variable.

Question 12

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
The length of time for which an apartment in a large complex remains vacant is a discrete random variable.

Question 13

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
A random variable is a function or rule that assigns a number to each outcome of an experiment.

Question 14

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
For a random variable X,if V(cX)= 4V(X),where V refers to the variance,then c must be 2.

Question 15

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
A continuous variable may take on any value within its relevant range even though the measurement device may not be precise enough to record it.

Question 16

The number of accidents that occur annually on a busy stretch of highway is an example of:

A) a discrete random variable.
B) a continuous random variable.
C) expected value of a discrete random variable.
D) expected value of a continuous random variable.

Question 17

For a random variable X,E(X + 2)- 5 = E(X)-3,where E refers to the expected value.

Question 18

Unsafe Levels of Radioactivity
The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year.
Faculty rank (professor,associate professor,assistant professor,and lecturer)is an example of a discrete random variable.

Question 19

For a random variable X,V(X + 3)= V(X + 6),where V refers to the variance.

Question 20

A function or rule that assigns a numerical value to each outcome of an experiment is called:

A) a sample space.
B) a probability distribution.
C) a random variable.
D) None of these choices.

Question 21

A motorcycle insurance company evaluates many numerical variables about a person before deciding on an appropriate rate for motorcycle insurance.The number of tickets a person has received in the last 3 years is an example of a(n)____________________ random variable.

Question 22

A(n)____________________ random variable is one whose values are uncountable.

Question 23

A lab at the DeBakey Institute orders 150 rats a week for each of the 52 weeks in the year for experiments that the lab conducts.Suppose the mean cost of rats used in lab experiments turned out to be $20.00 per week.Interpret this value.

A) Most of the weeks resulted in rat costs of $20.00
B) The median cost for the distribution of rat costs is $20.00
C) The expected or average costs for all weekly rat purchases is $20.00
D) The rat cost that occurs more often than any other is $20.00

Question 24

A motorcycle insurance company evaluates many numerical variables about a person before deciding on an appropriate rate for motorcycle insurance.How long a person has been a licensed rider is an example of a(n)____________________ random variable.

Question 25

Number of Motorcycles
The probability distribution of a discrete random variable X is shown below,where X represents the number of motorcycles owned by a family.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } &0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.25 & 0.40 & 0.20 & 0.15 \\\hline\end{array}$$

-{Number of Motorcycles Narrative} Find the standard deviation of X.

Question 26

The dean of students conducted a survey on campus.Grade point average (GPA)is an example of a(n)____________________ random variable.

Question 27

Which of the following is a continuous random variable?

A) The number of employees of an automobile company.
B) The amount of milk produced by a cow in one 24-hour period.
C) The number of gallons of milk sold at Albertson's grocery store last week.
D) None of these choices.

Question 28

Number of Horses
The random variable X represents the number of horses per family in a rural area in Iowa,with the probability distribution: p(x)= 0.05x,x = 2,3,4,5,or 6.
{Number of Horses Narrative} Express the probability distribution in tabular form.

Question 29

A(n)____________________ random variable is one whose values are countable.

Question 30

A motorcycle insurance company evaluates many numerical variables about a person before deciding on an appropriate rate for motorcycle insurance.The distance a person rides in a year is an example of a(n)____________________ random variable.

Question 31

In the notation below,X is the random variable,c is a constant,and V refers to the variance.Which of the following laws of variance is not true?

A) V(c)= 0
B) V(X + c)= V(X)+ c
C) V(cX)= c² V(X)
D) None of these choices.

Question 32

The amount of time that a microcomputer is used per week is an example of a(n)____________________ random variable.

Question 33

Which of the following is not a required condition for the distribution of a discrete random variable X that can assume values x_i?

A) 0 $\le$ p(x_i) $\le$ 1 for all x_i
B) $$\sum _ { \text {all } x _ { 1 } } p \left( x _ { i } \right) = 1$$
C) p(x_i)> 1 for all x_i
D) All of these choices are true.

Question 34

Number of Motorcycles
The probability distribution of a discrete random variable X is shown below,where X represents the number of motorcycles owned by a family.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } &0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.25 & 0.40 & 0.20 & 0.15 \\\hline\end{array}$$

-{Number of Motorcycles Narrative} Find the following probabilities:
a.
P(X > 1)
b.
P(X $\le$ 2)
c.
P(1 $\le$ X $\le$ 2)
d.
P(0 < X < 1)
e.
P(1 $\le$ X < 3)

Question 35

An auto insurance company evaluates many numerical variables about a person before deciding on an appropriate rate for automobile insurance.A person's age is an example of a(n)____________________ random variable.

Question 36

Number of Motorcycles
The probability distribution of a discrete random variable X is shown below,where X represents the number of motorcycles owned by a family.

{Number of Motorcycles Narrative} Find the expected value of X.

Question 37

Which of the following is a discrete random variable?

A) The Dow Jones Industrial average.
B) The volume of water in Michigan Lakes.
C) The time it takes you to drive to school.
D) The number of employees of a soft drink company.

Question 38

The number of days that a microcomputer goes without a breakdown is an example of a(n)____________________ random variable.

Question 39

An auto insurance company evaluates many numerical variables about a person before deciding on an appropriate rate for automobile insurance.The number of claims a person has made in the last 3 years is an example of a(n)____________________ random variable.

Question 40

In the notation below,X is the random variable,E and V refer to the expected value and variance,respectively.Which of the following is false?

A) E(3X)= 3E(X)
B) V(2)= 0
C) E(X + 1)= E(X)+ 1
D) All of these choices are true.

Question 41

Number of Horses
The random variable X represents the number of horses per family in a rural area in Iowa,with the probability distribution: p(x)= 0.05x,x = 2,3,4,5,or 6.

-{Number of Horses Narrative} Find the variance and standard deviation of X.

Question 42

Number of Horses
The random variable X represents the number of horses per family in a rural area in Iowa,with the probability distribution: p(x)= 0.05x,x = 2,3,4,5,or 6.
{Number of Horses Narrative} Find the expected number of horses per family.

Question 43

Shopping Outlet
A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below.

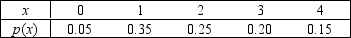
{Shopping Outlet Narrative} Find the expected value of the number of stores entered.

Question 44

Gym Visits
Let X represent the number of times a student visits a gym in a one month period.Assume that the probability distribution of X is as follows:
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.05 & 0.25 & 0.50 & 0.20 \\\hline\end{array}$$

-{Gym Visits Narrative} Find the mean $\mu$ and the standard deviation $\sigma$ of this distribution.

Question 45

Gym Visits
Let X represent the number of times a student visits a gym in a one month period.Assume that the probability distribution of X is as follows:
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.05 & 0.25 & 0.50 & 0.20 \\\hline\end{array}$$

-{Gym Visits Narrative} Find the mean and the standard deviation of Y = 2X - 1.

Question 46

Gym Visits
Let X represent the number of times a student visits a gym in a one month period.Assume that the probability distribution of X is as follows:
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.05 & 0.25 & 0.50 & 0.20 \\\hline\end{array}$$

-The monthly sales at a Gas Station have a mean of $50,000 and a standard deviation of $6,000.Profits are calculated by multiplying sales by 40% and subtracting fixed costs of $12,000.Find the mean and standard deviation of monthly profits.

Question 47

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.

{Retries Narrative} What is the variance for the number of retries?

Question 48

Gym Visits
Let X represent the number of times a student visits a gym in a one month period.Assume that the probability distribution of X is as follows:

{Gym Visits Narrative} What is the probability that the student visits the gym at most twice in a month?

Question 49

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.

{Retries Narrative} What is the mean or expected value for the number of retries?

Question 50

Shopping Outlet
A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below.

{Shopping Outlet Narrative} Calculate the variance and standard deviation of Y directly from the probability distribution of Y.

Question 51

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.

{Retries Narrative} What is the probability of a least one retry?

Question 52

Blackjack
The probability distribution of a random variable X is shown below,where X represents the amount of money (in $1,000s)gained or lost in a particular game of Blackjack.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & - 4 & 0 & 4 & 8 \\\hline p ( x ) & 0.15 & 0.25 & 0.20 & 0.40 \\\hline\end{array}$$

-{Blackjack Narrative} Find the following probabilities:
a.
P(X $\le$ 0)
b.
P(X > 3)
c.
P(0 $\le$ X $\le$ 4)
d.
P(X = 5)

Question 53

Shopping Outlet
A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below.

{Shopping Outlet Narrative} Use the laws of expected value to calculate the mean of Y from the probability distribution of X.

Question 54

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.

{Retries Narrative} What is the probability of no retries?

Question 55

Shopping Outlet
A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below.

{Shopping Outlet Narrative} Calculate the expected value of Y directly from the probability distribution of Y.

Question 56

Shopping Outlet
A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below.

{Shopping Outlet Narrative} What did you notice about the mean,variance,and standard deviation of Y = 2X + 1 in terms of the mean,variance,and standard deviation of X?

Question 57

Shopping Outlet
A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below.
$$\begin{array} { | c | c c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 & 4 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.05 & 0.35 & 0.25 &0 .20 & 0.15 \\\hline\end{array}$$

-{Shopping Outlet Narrative} Use the laws of variance to calculate the variance and standard deviation of Y from the probability distribution of X.

Question 58

Shopping Outlet
A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below.

{Shopping Outlet Narrative} Find the variance and standard deviation of the number of stores entered.

Question 59

Shopping Outlet
A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below.

{Shopping Outlet Narrative} Suppose Y = 2X + 1 for each value of X.What is the probability distribution of Y?

Question 60

Gym Visits
Let X represent the number of times a student visits a gym in a one month period.Assume that the probability distribution of X is as follows:

{Gym Visits Narrative} What is the probability that the student visits the gym at least once in a month?

Question 61

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are two variables with $\sigma _ { x } ^ { 2 } = 3.25$ , $\sigma _ { y } ^ { 2 } = 5.8$ ,and COV(X,Y)= 14.703,then the coefficient of correlation $\rho$ = 0.78.

Question 62

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are two variables with $\sigma$ _x = 3.8, $\sigma$ _y = 4.2,and COV(X,Y)= -0.25,then V(X + Y)= 31.58.

Question 63

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-The variance of X must be non-negative;the variance of Y must be non-negative;hence the covariance of X and Y must be non-negative.

Question 64

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are independent variables with V(X)= 23.48 and V(Y)= 36.52,then the standard deviation of W = X + Y is $\sigma$ _w = 7.746.

Question 65

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are random variables with E(X)= 6 and E(Y)= 9,then E(2X + 3Y)is:

A) 39
B) 15
C) 27
D) 12

Question 66

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are two variables with E(XY)= 10.56,E(X)= 4.22,and E(Y)= 5.34,then COV(X,Y)= 1.0.

Question 67

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-The covariance can be negative but the coefficient of correlation cannot.

Question 68

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-A statistical measure of the strength of the relationship between two random variables X and Y is referred to as the:

A) expected value
B) variance
C) covariance
D) standard deviation

Question 69

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are two variables with $$\sigma _ { x } ^ { 2 } = 12.25$$ , $\sigma _ { y } ^ { 2 } = 17.64$ ,and COV(X,Y)= 11.76,then the coefficient of correlation $\sigma$ = 0.8.

Question 70

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-{Retries Narrative} What is the standard deviation of the number of retries?

Question 71

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are independent variables,then COV(X,Y)> 0.

Question 72

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-Bivariate distributions provide probabilities of combinations of two variables.

Question 73

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-The sum of the expected values always equals the expected value of the sums.

Question 74

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are random variables,the sum of all the conditional probabilities of X given a specific value of Y will always be:

A) 0.0
B) 1.0
C) the average of the possible values of X.
D) the average of the possible values of Y.

Question 75

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are independent variables,then their coefficient of correlation $\rho$ = 0.

Question 76

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are any random variables with COV(X,Y)= 0.25, $\sigma _ { x } ^ { 2 } = 0.36$ ,and $\sigma _ { y } ^ { 2 } = 0.49$
,then the coefficient of correlation $\rho$ is

A) 1.417
B) 1.190
C) 0.595
D) 0.354

Question 77

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-The covariance of two variables X and Y:

A) must be between -1 and +1.
B) must be positive.
C) can be any real number.
D) None of these choices.

Question 78

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If X and Y are any random variables with E(X)= 5,E(Y)= 6,E(XY)= 21,V(X)= 9 and V(Y)= 10,then the relationship between X and Y is a:

A) strong positive relationship
B) strong negative relationship
C) weak positive relationship
D) weak negative relationship

Question 79

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-If you add two single probability distributions together you get a bivariate distribution.

Question 80

Retries
The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
$$\begin{array} { | c | c c c c | } \hline \boldsymbol { x } & 0 & 1 & 2 & 3 \\\hline \boldsymbol { p } ( \boldsymbol { x } ) & 0.35 & 0.35 & 0.25 & 0.05 \\\hline\end{array}$$

-The variance of the sum always equals the sum of the variances.