Deck 4: Probability and Probability Distributions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/114
Play
Full screen (f)
Deck 4: Probability and Probability Distributions
1
Probability is a number between 0 and 1, inclusive, which measures the likelihood that some event will occur.
True
2
If P(A) = 0.25 and P(B) = 0.65, then P(A and B) is:
A) 0.25
B) 0.40
C) 0.90
D) This cannot be determined from the information given.
A) 0.25
B) 0.40
C) 0.90
D) This cannot be determined from the information given.
This cannot be determined from the information given.
3
Which of the following best describes the concept of probability?
A) It is a measure of the likelihood that a particular event will occur.
B) It is a measure of the likelihood that a particular event will occur, given that another event has already occurred.
C) It is a measure of the likelihood of the simultaneous occurrence of two or more events.
D) None of these choices describe the concept of probability.
A) It is a measure of the likelihood that a particular event will occur.
B) It is a measure of the likelihood that a particular event will occur, given that another event has already occurred.
C) It is a measure of the likelihood of the simultaneous occurrence of two or more events.
D) None of these choices describe the concept of probability.
It is a measure of the likelihood that a particular event will occur.
4
If two events are mutually exclusive, what is the probability that both occur at the same time?
A) 0.00
B) 0.50
C) 1.00
D) This cannot be determined from the information given.
A) 0.00
B) 0.50
C) 1.00
D) This cannot be determined from the information given.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
5
The probabilities shown in a table with two rows, 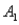 and
and 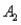 and two columns,
and two columns, 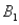 and
and 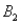 , are as follows: P(
, are as follows: P( 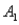 and
and 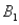 ) = .10, P(
) = .10, P( 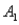 and
and 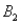 ) = .30, P(
) = .30, P( 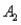 and
and 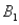 ) = .05, and P(
) = .05, and P( 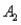 and
and 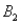 ) = .55. Then P(
) = .55. Then P( 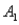 |
| 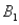 ), calculated up to two decimals, is:
), calculated up to two decimals, is:
A) .33
B) .35
C) .65
D) .67
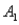 and
and 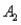 and two columns,
and two columns, 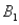 and
and 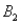 , are as follows: P(
, are as follows: P( 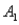 and
and 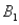 ) = .10, P(
) = .10, P( 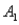 and
and 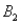 ) = .30, P(
) = .30, P( 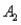 and
and 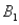 ) = .05, and P(
) = .05, and P( 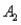 and
and 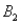 ) = .55. Then P(
) = .55. Then P( 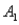 |
| 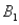 ), calculated up to two decimals, is:
), calculated up to two decimals, is:A) .33
B) .35
C) .65
D) .67

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
6
If events A and B have nonzero probabilities, then they can be both independent and mutually exclusive.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
7
The law of large numbers is relevant to the estimation of:
A) objective probabilities
B) subjective probabilities
C) both objective and subjective probabilities
D) neither objective nor subjective probabilities
A) objective probabilities
B) subjective probabilities
C) both objective and subjective probabilities
D) neither objective nor subjective probabilities

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
8
If events A and B are mutually exclusive, then the probability of both events occurring simultaneously is equal to:
A) 0.0
B) 0.5
C) 1.0
D) any value between 0.5 and 1.0
A) 0.0
B) 0.5
C) 1.0
D) any value between 0.5 and 1.0

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
9
Two or more events are said to be exhaustive if one of them must occur.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
10
If P(A and B) = 1, then A and B must be collectively exhaustive.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
11
Let A and B be the events of the FDA approving and rejecting a new drug to treat hypertension, respectively. The events A and B are:
A) independent
B) conditional
C) unilateral
D) mutually exclusive
A) independent
B) conditional
C) unilateral
D) mutually exclusive

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
12
The probabilities shown in a table with two rows, 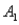 and
and 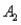 and two columns,
and two columns, 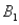 and
and 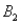 , are as follows: P(
, are as follows: P( 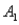 and
and 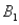 ) = .10, P(
) = .10, P( 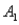 and
and  ) = .30, P(
) = .30, P( 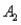 and
and 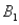 ) = .05, and P(
) = .05, and P( 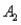 and
and 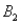 ) = .55. Then P(
) = .55. Then P( 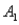 |
| 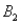 ), calculated up to two decimals, is:
), calculated up to two decimals, is:
A) .33
B) .35
C) .65
D) .67
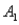 and
and 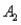 and two columns,
and two columns, 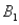 and
and 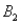 , are as follows: P(
, are as follows: P( 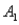 and
and 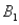 ) = .10, P(
) = .10, P( 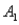 and
and  ) = .30, P(
) = .30, P( 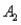 and
and 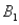 ) = .05, and P(
) = .05, and P( 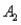 and
and 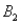 ) = .55. Then P(
) = .55. Then P( 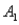 |
| 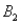 ), calculated up to two decimals, is:
), calculated up to two decimals, is:A) .33
B) .35
C) .65
D) .67

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
13
If A and B are independent events with P(A) = 0.40 and P(B) = 0.50, then P(A/B) is 0.50.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
14
If two events are collectively exhaustive, what is the probability that both occur at the same time?
A) 0.00
B) 0.50
C) 1.00
D) This cannot be determined from the information given.
A) 0.00
B) 0.50
C) 1.00
D) This cannot be determined from the information given.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
15
If P(A) = P(A|B), then events A and B are said to be:
A) mutually exclusive
B) independent
C) exhaustive
D) complementary
A) mutually exclusive
B) independent
C) exhaustive
D) complementary

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
16
A function that associates a numerical value with each possible outcome of an uncertain event is called a:
A) conditional variable
B) random variable
C) population variable
D) sample variable
A) conditional variable
B) random variable
C) population variable
D) sample variable

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
17
If A and B are mutually exclusive events with P(A) = 0.70, then P(B):
A) can be any value between 0 and 1
B) can be any value between 0 and 0.70
C) cannot be larger than 0.30
D) can be any value between 0.30 and 0.70
A) can be any value between 0 and 1
B) can be any value between 0 and 0.70
C) cannot be larger than 0.30
D) can be any value between 0.30 and 0.70

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
18
If two events are independent, what is the probability that they both occur?
A) 0
B) 0.50
C) 1.00
D) This cannot be determined from the information given.
A) 0
B) 0.50
C) 1.00
D) This cannot be determined from the information given.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
19
If A and B are any two events with P(A) = .8 and P(B|A) = .4, then the joint probability of A and B is:
A) .80
B) .40
C) .32
D) 1.20
A) .80
B) .40
C) .32
D) 1.20

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
20
You think you have a 90% chance of passing your statistics class. This is an example of subjective probability.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
21
Two events A and B are said to mutually be exclusive if P(A and B) = 0.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
22
Football teams toss a coin to see who will get their choice of kicking or receiving to begin a game. The probability that given team will win the toss three games in a row is 0.125.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
23
Two or more events are said to be mutually exclusive if at most one of them can occur.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
24
How many females were interviewed?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
25
The law of large numbers states that subjective probabilities can be estimated based on the long run relative frequencies of events.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
26
The multiplication rule for two events A and B is: P(A and B) = P(A|B)P(A).

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
27
What is the probability that a respondent chosen at random is a male?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
28
Two or more events are said to be exhaustive if at most one of them can occur.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
29
Two events are said to be independent when knowledge of one event is of no value when assessing the probability of the other.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
30
The relative frequency of an event is the number of times the event occurs out of the total number of times the random experiment is run.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
31
Suppose that after graduation, you will either buy a new car (event A) or take a trip to Europe (event B). In this case, events A and B are mutually exclusive.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
32
Conditional probability is the probability that an event will occur, with no other events taken into consideration.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
33
If A and B are two independent events with P(A) = 0.20 and P(B) = 0.60, then P(A and B) = 0.80.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
34
What is the probability that a respondent chosen at random enjoys shopping for clothing?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
35
Suppose A and B are mutually exclusive events where P(A) = 0.3 and P(B) = 0.4. Then, P(A and B) = 0.12.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
36
What is the probability that a household answered no?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
37
Suppose A and B are two events where P(A) = 0.5, P(B) = 0.4, and P(A and B) = 0.2, then P(B/A) = 0.5.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
38
Given that events A and B are independent and that P(A) = 0.8 and P(B/A) = 0.4, then P(A and B) = 0.32.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
39
What is the probability that a respondent was female?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
40
If P(A and B) = 0, then A and B must be collectively exhaustive.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
41
What is the probability that a respondent chosen at random is a male who enjoys shopping for clothing or a female who enjoys shopping for clothing?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
42
Are gender of patrons and drinking preference independent? Explain.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
43
What is the probability that a respondent chosen at random is a male and does not enjoy shopping for clothing?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
44
What is the probability that a respondent chosen at random enjoys or does not enjoy shopping for clothing?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
45
What is the probability that none of the oil wells will be successful?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
46
Suppose a randomly selected patron prefers beer. What is the probability the patron is a male?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
47
Suppose a randomly selected patron prefers wine. What is the probability the patron is a male?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
48
What is the probability that a respondent chosen at random is a female and does not enjoy shopping for clothing?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
49
Suppose a randomly selected patron is a female. What is the probability that the patron prefers wine?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
50
What is the probability that any well will not be successful?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
51
What is the probability that a respondent chosen at random does not enjoy shopping for clothing?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
52
Does consumer behavior depend on the gender of consumer? Explain using probabilities.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
53
What is the probability a randomly selected patron prefers wine?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
54
What is the probability a randomly selected patron is a female who prefers beer?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
55
Suppose a randomly selected patron is a female. What is the probability the patron prefers beer?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
56
What is the probability a randomly selected patron is a female who prefers wine?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
57
What is the probability that a respondent chosen at random is a male or a female?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
58
What is the probability that a randomly selected patron is not male?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
59
What is the probability a randomly selected patron is a female?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
60
What is the probability that a respondent chosen at random is a female and enjoys shopping for clothing?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
61
A probability tree is a graphical representation of how events occur through time, which is useful for calculating probabilities.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
62
Find the probability that three or fewer customers are in line.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
63
If it costs $200,000 to drill each well and a successful well will produce $1,000,000 worth of oil over its lifetime, what is the expected net value of this three-well program if no wells are successful?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
64
The time students spend in a computer lab during one day is an example of a continuous random variable.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
65
If it costs $200,000 to drill each well and a successful well will produce $1,000,000 worth of oil over its lifetime, what is the expected net value of this three-well program if all three wells are successful?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
66
There are two types of random variables, they are:
A) discrete and continuous
B) exhaustive and mutually exclusive
C) complementary and cumulative
D) real and unreal
A) discrete and continuous
B) exhaustive and mutually exclusive
C) complementary and cumulative
D) real and unreal

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
67
A discrete probability distribution:
A) is a set of possible values and a corresponding set of probabilities that sum to 1
B) is a modeling tool that can be used to incorporate uncertainty into models
C) can be estimated from long-run proportions
D) is the distribution of a single random variable
A) is a set of possible values and a corresponding set of probabilities that sum to 1
B) is a modeling tool that can be used to incorporate uncertainty into models
C) can be estimated from long-run proportions
D) is the distribution of a single random variable

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
68
(A) What is the expected completion time (in months) from now for this project?
(B) How much variability (in months) exists around the expected value found in (A)?
(B) How much variability (in months) exists around the expected value found in (A)?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
69
Find the probability distribution of X.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
70
What is the probability that this project will be completed in less than 4 months from now?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
71
A small grocery store is considering installing an express checkout line. Let X be the number of customers in the regular checkout line. Note that these numbers include the customers being served, if any. The probability distribution of X is given in the table below. 
Find the probability that no more than one customer is in line.

Find the probability that no more than one customer is in line.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
72
The number of cars produced by GM during a given quarter is a continuous random variable.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
73
A small grocery store is considering installing an express checkout line. Let X be the number of customers in the regular checkout line. Note that these numbers include the customers being served, if any. The probability distribution of X is given in the table below. 
Find the probability that one customer is in the regular checkout line.

Find the probability that one customer is in the regular checkout line.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
74
The number of car insurance policy holders is an example of a discrete random variable.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
75
What is the probability that this project will not be completed on time?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
76
The temperature of the room in which you are writing this test is a continuous random variable.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
77
The possible annual percentage return of the stocks of Gamma, Inc. and Delta, Inc. share a common probability distribution, given below. 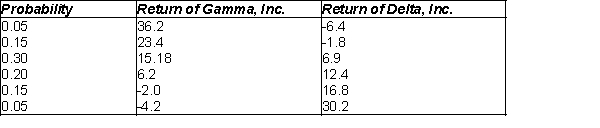
(A) What is the expected annual return of each stock?
(B) What is the standard deviation of the annual return of each stock?
(C) On the basis of your answers to (A) and (B), which of these stocks would you prefer to buy? Defend your choice.
(D) Are the annual returns of these two stocks positively or negatively associated with each other? How might the answer to this question influence your decision to purchase shares?

(A) What is the expected annual return of each stock?
(B) What is the standard deviation of the annual return of each stock?
(C) On the basis of your answers to (A) and (B), which of these stocks would you prefer to buy? Defend your choice.
(D) Are the annual returns of these two stocks positively or negatively associated with each other? How might the answer to this question influence your decision to purchase shares?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
78
If a new pipeline will be constructed in the event that all three wells are successful, what is the probability that the pipeline will be constructed?

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
79
A small grocery store is considering installing an express checkout line. Let X be the number of customers in the regular checkout line. Note that these numbers include the customers being served, if any. The probability distribution of X is given in the table below. 
Find the probability that at least two people are in line.

Find the probability that at least two people are in line.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
80
The number of people entering a shopping mall on a given day is an example of a discrete random variable.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck



