Deck 5: Understanding and Comparing Distributions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/103
Play
Full screen (f)
Deck 5: Understanding and Comparing Distributions
1
To plan the budget for next year a college needs to estimate what impact the current economic downturn might have on student requests for financial aid. Historically, this college has provided aid to 35% of its students. Officials look at a random sample of this year's applications to see what proportion indicate a need for financial aid. Based on these data they create a 90% confidence interval of (32%, 40%). Could this interval be used to test the hypothesis H0: p = 0.35 versus HA: p ×0.35 at the a = 0.10 level of significance?
A) No, because financial aid amounts may not be normally distributed.
B) Yes; since 35% is in the confidence interval they fail to reject the null hypothesis, concluding that there is not strong evidence of any change in financial aid requests.
C) No, because they only used a sample of the applicants instead of all of them.
D) Yes; since 35% is not at the center of the confidence interval they reject the null hypothesis, concluding that the percentage of students requiring aid will increase.
E) Yes; since 35% is in the confidence interval they accept the null hypothesis, concluding that the percentage of students requiring financial aid will stay the same.
A) No, because financial aid amounts may not be normally distributed.
B) Yes; since 35% is in the confidence interval they fail to reject the null hypothesis, concluding that there is not strong evidence of any change in financial aid requests.
C) No, because they only used a sample of the applicants instead of all of them.
D) Yes; since 35% is not at the center of the confidence interval they reject the null hypothesis, concluding that the percentage of students requiring aid will increase.
E) Yes; since 35% is in the confidence interval they accept the null hypothesis, concluding that the percentage of students requiring financial aid will stay the same.
Yes; since 35% is in the confidence interval they fail to reject the null hypothesis, concluding that there is not strong evidence of any change in financial aid requests.
2
Pew research reported in 2013 that 15% of American adults do not use the internet or e-mail. They report a margin of error of 2.3 percentage points. The meaning of that margin of error is:
A) They are 2.3% confident that their confidence interval captures the true proportion of American adults that don't use the internet or e-mail.
B) If they repeatedly sampled from the population, and constructed a confidence interval for each estimate, about 2.3% of those intervals would capture the proportion of American adults who don't use the internet or e-mail.
C) They are pretty sure that the poll result differs from the actual percentage of American adults who don't use the internet or e-mail by 2.3% or less.
D) There is a 2.3% probability that their estimate is incorrect.
E) They estimate that 2.3% of those surveyed answered incorrectly.
A) They are 2.3% confident that their confidence interval captures the true proportion of American adults that don't use the internet or e-mail.
B) If they repeatedly sampled from the population, and constructed a confidence interval for each estimate, about 2.3% of those intervals would capture the proportion of American adults who don't use the internet or e-mail.
C) They are pretty sure that the poll result differs from the actual percentage of American adults who don't use the internet or e-mail by 2.3% or less.
D) There is a 2.3% probability that their estimate is incorrect.
E) They estimate that 2.3% of those surveyed answered incorrectly.
C
3
A pharmaceutical company investigating whether drug stores are less likely than food stores to remove over-the-counter drugs from the shelves when the drugs are past the expiration date found a P-value of 2.8%. This means that:
A) 97.2% more drug stores remove over-the-counter drugs from the shelves when the drugs are past the expiration date than food stores.
B) There is a 97.2% chance the drug stores remove more expired over-the-counter drugs.
C) None of these.
D) 2.8% more drug stores remove over-the-counter drugs from the shelves when the drugs are past the expiration date.
E) There is a 2.8% chance the drug stores remove more expired over-the-counter drugs.
A) 97.2% more drug stores remove over-the-counter drugs from the shelves when the drugs are past the expiration date than food stores.
B) There is a 97.2% chance the drug stores remove more expired over-the-counter drugs.
C) None of these.
D) 2.8% more drug stores remove over-the-counter drugs from the shelves when the drugs are past the expiration date.
E) There is a 2.8% chance the drug stores remove more expired over-the-counter drugs.
C
4
Suppose that a conveyor used to sort packages by size does not work properly. We test the conveyor on several packages (with H₀: incorrect sort) and our data results in a P-value of 0.016. What probably happens as a result of our testing?
A) We reject H₀, making a Type I error.
B) We correctly fail to reject H₀.
C) We reject H₀, making a Type II error.
D) We fail to reject H₀, committing a Type II error.
E) We correctly reject H₀.
A) We reject H₀, making a Type I error.
B) We correctly fail to reject H₀.
C) We reject H₀, making a Type II error.
D) We fail to reject H₀, committing a Type II error.
E) We correctly reject H₀.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
5
Suppose that a manufacturer is testing one of its machines to make sure that the machine is producing more than 97% good parts (H? : p = 0.97 and HA : p > 0.97) . The test results in aP-value of 0.122. Unknown to the manufacturer, the machine is actually producing 99% good parts. What probably happens as a result of the testing?
A) They reject H0, making a Type I error.
B) They correctly reject H0.
C) They fail to reject H0, making a Type I error.
D) They fail to reject H0, making a Type II error.
E) They correctly fail to reject H0.
A) They reject H0, making a Type I error.
B) They correctly reject H0.
C) They fail to reject H0, making a Type I error.
D) They fail to reject H0, making a Type II error.
E) They correctly fail to reject H0.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
6
A pharmaceutical company has a drug they think is better at lowering blood pressure than the current medication, but it costs more money for consumers. They conduct a significance test on their clinical study (H₀: The new drug works the same as the old drug, Ha: The new drug works better than the old drug.) Which statement about errors is correct?
A) A Type I error means people pay more money for a drug that doesn't work better.
B) If they reject the null hypothesis, it is possible that they are rejecting a Type II error.
C) A Type I error means people keep using the old drug when a new drug works better.
D) If they fail to reject the null hypothesis, they might be committing a Type I error.
E) A Type II error would mean that they did not use randomization in their experiment.
A) A Type I error means people pay more money for a drug that doesn't work better.
B) If they reject the null hypothesis, it is possible that they are rejecting a Type II error.
C) A Type I error means people keep using the old drug when a new drug works better.
D) If they fail to reject the null hypothesis, they might be committing a Type I error.
E) A Type II error would mean that they did not use randomization in their experiment.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
7
An online catalog company wants on-time delivery for at least 90% of the orders they ship. They have been shipping orders via UPS and FedEx but will switch to a more expensive service (ShipFast) if there is evidence that this service can exceed the 90% on-time goal. As a test the company sends a random sample of orders via ShipFast, and then makes follow-up phone calls to see if these orders arrived on time. Which hypotheses should they test?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
8
Suppose that a device advertised to increase a car's gas mileage really does not work. We test it on a small fleet of cars (with H0: not effective), and our data results in a P-value of 0.004. What Probably happens as a result of our experiment?
A) We fail to reject H0, committing a Type II error.
B) We correctly reject H0.
C) We reject H0, making a Type I error.
D) We correctly fail to reject H0.
E) We reject H0, making a Type II error.
A) We fail to reject H0, committing a Type II error.
B) We correctly reject H0.
C) We reject H0, making a Type I error.
D) We correctly fail to reject H0.
E) We reject H0, making a Type II error.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
9
To plan the course offerings for the next year a university department dean needs to estimate what impact the "No Child Left Behind" legislation might have on the teacher credentialing program. Historically, 40% of this university's pre-service teachers have qualified for paid internship positions each year. The Dean of Education looks at a random sample of internship applications to see what proportion indicate the applicant has achieved the content-mastery that is required for the internship. Based on these data he creates a 90% confidence interval of (33%, 41%). Could this confidence interval be used to test the hypothesis H₀: p = 0.40 versus HA: p < 0.40 at the a = 0.05 level of significance?
A) Yes, since 40% is not the center of the confidence interval he rejects the null hypothesis, concluding that the percentage of qualified applicants will decrease.
B) No, because the dean should have used a 95% confidence interval.
C) No, because the dean only reviewed a sample of the applicants instead of all of them.
D) Yes, since 40% is in the confidence interval he accepts the null hypothesis, concluding that the percentage of applicants qualified for paid internship positions will stay the same.
E) Yes, since 40% is in the confidence interval he fails to reject the null hypothesis, concluding that there is not strong enough evidence of any change in the percent of qualified applicants.
A) Yes, since 40% is not the center of the confidence interval he rejects the null hypothesis, concluding that the percentage of qualified applicants will decrease.
B) No, because the dean should have used a 95% confidence interval.
C) No, because the dean only reviewed a sample of the applicants instead of all of them.
D) Yes, since 40% is in the confidence interval he accepts the null hypothesis, concluding that the percentage of applicants qualified for paid internship positions will stay the same.
E) Yes, since 40% is in the confidence interval he fails to reject the null hypothesis, concluding that there is not strong enough evidence of any change in the percent of qualified applicants.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
10
We will test the hypothesis that p = 60% versus p > 60%. We don't know it, but actually p is 70%.
With which sample size and significance level will our test have the greatest power?
A) a = 0.05, n = 200
B) a = 0.01, n = 200
C) The power will be the same so long as the true proportion p remains 70%.
D) a = 0.01, n = 500
E) a = 0.05, n = 500
With which sample size and significance level will our test have the greatest power?
A) a = 0.05, n = 200
B) a = 0.01, n = 200
C) The power will be the same so long as the true proportion p remains 70%.
D) a = 0.01, n = 500
E) a = 0.05, n = 500

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
11
A truck company wants on-time delivery for 98% of the parts they order from a metal manufacturing plant. They have been ordering from Hudson Manufacturing but will switch to a new, cheaper manufacturer (Steel-R-Us) unless there is evidence that this new manufacturer cannot meet the 98% on-time goal. As a test the truck company purchases a random sample of metal parts from Steel-R-Us, and then determines if these parts were delivered on-time. Which hypothesis should they test?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
12
We have calculated a confidence interval based on a sample of size n = 100. After looking over our work, we realized that the sample size was actually 400. How will the corrected interval compare to the original interval?
A) The corrected interval will be the same width.
B) The corrected interval will be one-fourth as wide.
C) The corrected interval will be four times as wide.
D) The corrected interval will be one-half as wide.
E) The corrected interval will be twice as wide.
A) The corrected interval will be the same width.
B) The corrected interval will be one-fourth as wide.
C) The corrected interval will be four times as wide.
D) The corrected interval will be one-half as wide.
E) The corrected interval will be twice as wide.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
13
A city planner wants to construct a confidence interval for the proportion of workers who would take public transportation to work if it were available. What sample size should he use to estimate the proportion to within 4 percentage points at a 90% level of confidence?
A) 30
B) 423
C) 218
D) 842
E) 601
A) 30
B) 423
C) 218
D) 842
E) 601

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
14
We conduct a significance test with H0: p = 0.43 and Ha: p × 0.43. We take a sample of size 250 and get a sample proportion of 0.40. The appropriate test statistic is:
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
15
We test the hypothesis that p = 35% versus p < 35%. We don't know it but actually p = 26%. With which sample size and significance level will our test have the greatest power?
A) a = 0.03, n = 250
B) a = 0.01, n = 250
C) The power will be the same as long as the true proportion p remains 26%
D) a = 0.01, n = 400
E) a = 0.03, n = 400
A) a = 0.03, n = 250
B) a = 0.01, n = 250
C) The power will be the same as long as the true proportion p remains 26%
D) a = 0.01, n = 400
E) a = 0.03, n = 400

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
16
A statistics professor wants to see if more than 80% of her students enjoyed taking her class. At the end of the term, she takes a random sample of students from her large class and asks, in an anonymous survey, if the students enjoyed taking her class. Which set of hypotheses should she test?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
17
A college alumni fund appeals for donations by phoning or emailing recent graduates. A random sample of 300 alumni shows that 40% of the 150 who were contacted by telephone actually made contributions compared to only 30% of the 150 who received email requests. Which formula calculates the 98% confidence interval for the difference in the proportions of alumni who may make donations if contacted by phone or by email?
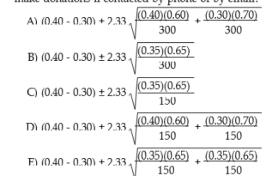


Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
18
A P-value indicates
A) the probability that the alternative hypothesis is true.
B) the probability the null is true given the observed statistic.
C) the probability that the null hypothesis is true.
D) the probability of the observed statistic given that the null hypothesis is true.
E) the probability of the observed statistic given that the alternative hypothesis is true.
A) the probability that the alternative hypothesis is true.
B) the probability the null is true given the observed statistic.
C) the probability that the null hypothesis is true.
D) the probability of the observed statistic given that the null hypothesis is true.
E) the probability of the observed statistic given that the alternative hypothesis is true.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
19
A statistician consulting for a state department of transportation conducts a study to see if the proportion of drivers who are intoxicated has decreased since last year.
H₀: The proportion is the same as last year
Ha: The proportion is less than last year.
The P-value of a significance test is greater than the significance level. She can conclude:
A) there is not enough evidence to reject the null hypothesis.
B) the test was too powerful.
C) the null hypothesis is true.
D) there is enough evidence to reject the null hypothesis.
E) the alternative hypothesis is true.
H₀: The proportion is the same as last year
Ha: The proportion is less than last year.
The P-value of a significance test is greater than the significance level. She can conclude:
A) there is not enough evidence to reject the null hypothesis.
B) the test was too powerful.
C) the null hypothesis is true.
D) there is enough evidence to reject the null hypothesis.
E) the alternative hypothesis is true.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
20
A researcher investigating whether joggers are less likely to get colds than people who do not jog found a P-value of 3%. This means that:
A) 3% of joggers get colds.
B) There's a 3% chance that joggers don't get fewer colds.
C) There's a 3% chance that joggers get fewer colds.
D) Joggers get 3% fewer colds than non-joggers.
E) None of these.
A) 3% of joggers get colds.
B) There's a 3% chance that joggers don't get fewer colds.
C) There's a 3% chance that joggers get fewer colds.
D) Joggers get 3% fewer colds than non-joggers.
E) None of these.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
21
Pew Research found that, in 2013, 50% of American adults favored allowing same-sex couples to marry legally. This is up from 48% in 2012. The 2013 estimate was based on a random sample of 1,501 adults. Assume the same sample size was used in 2012. ["Changing Attitudes on Gay Marriage," Pew Internet and American Life Project, June 2013
-What is meant by the phrase "95% confident" in this context?
-What is meant by the phrase "95% confident" in this context?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
22
Pew Research found that, in 2013, 50% of American adults favored allowing same-sex couples to marry legally. This is up from 48% in 2012. The 2013 estimate was based on a random sample of 1,501 adults. Assume the same sample size was used in 2012. ["Changing Attitudes on Gay Marriage," Pew Internet and American Life Project, June 2013
-Because it is known that support for allowing same-sex couples to marry has been rising, it would be reasonable to perform a one-sided hypothesis test with the alternative
hypothesis that the proportion of Americans who favor allowing such marriages is greater
in 2013 than 2012. Would such a test cause you to reach the same conclusion you reached in question 3?
-Because it is known that support for allowing same-sex couples to marry has been rising, it would be reasonable to perform a one-sided hypothesis test with the alternative
hypothesis that the proportion of Americans who favor allowing such marriages is greater
in 2013 than 2012. Would such a test cause you to reach the same conclusion you reached in question 3?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
23
Exercise A random sample of 150 men found that 88 of the men exercise regularly, while a random sample of 200 women found that 130 of the women exercise regularly.
a. Based on the results, construct and interpret a 95% confidence interval for the difference in the proportions of women and men who exercise regularly.
b. A friend says that she believes that a higher proportion of women than men exercise regularly. Does your confidence interval support this conclusion? Explain.
a. Based on the results, construct and interpret a 95% confidence interval for the difference in the proportions of women and men who exercise regularly.
b. A friend says that she believes that a higher proportion of women than men exercise regularly. Does your confidence interval support this conclusion? Explain.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
24
The countries of Europe report that 46% of the labor force is female. The United Nations wonders if the percentage of females in the labor force is the same in the United States. Representatives from the United States Department of Labor plan to check a random sample of over 10,000 employment records on file to estimate a percentage of females in the United States labor force.
-They actually select a random sample of 525 employment records, and find that 229 of the people are females. Create the confidence interval.
-They actually select a random sample of 525 employment records, and find that 229 of the people are females. Create the confidence interval.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
25
A statistics professor asked her students whether or not they were registered to vote. In a sample of 50 of her students
(randomly sampled from her 700 students), 35 said they were registered to vote.
-Find a 95% confidence interval for the true proportion of the professor's students who were registered to vote. (Make sure to check any necessary conditions and to state a conclusion
in the context of the problem.)
(randomly sampled from her 700 students), 35 said they were registered to vote.
-Find a 95% confidence interval for the true proportion of the professor's students who were registered to vote. (Make sure to check any necessary conditions and to state a conclusion
in the context of the problem.)

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
26
Researchers conduct a study to test a potential side effect of a new allergy medication. A random sample of 160 subjects with allergies was selected for the study. The new "improved" Brand I medication was randomly assigned to 80 subjects, and the current Brand C medication was randomly assigned to the other 80 subjects. 14 of the 80 patients with Brand I reported drowsiness, and 22 of the 80 patients with Brand C reported drowsiness.
-Would you make the same conclusion as question 2 if you conducted a hypothesis test?
Explain.
-Would you make the same conclusion as question 2 if you conducted a hypothesis test?
Explain.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
27
Gun ownership Concerned about recent incidence of gun violence, a public interest group conducts a poll of 850 randomly selected American adults and finds that 44% of those surveyed have a gun in their home.
a. Construct and interpret a 95% confidence interval for the proportion of all American adults who have a gun in their home.
b. Explain what is meant by 95% confidence in this context.
a. Construct and interpret a 95% confidence interval for the proportion of all American adults who have a gun in their home.
b. Explain what is meant by 95% confidence in this context.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
28
According to the 2010 census, 20.3% of the population of the United States (ages 5 and up) live in a home in which a language other than English is spoken. Advocates for providing government programs to assist non-English speakers are convinced that, with the increasing non-white population in the United States, this proportion has probably increased. They plan to conduct a survey, and if they find the proportion of people who live in such homes has increased, they will organize a campaign to increase government investment in these assistance programs.
-What alpha level did the group use?
-What alpha level did the group use?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
29
A company manufacturing computer chips finds that 8% of all chips manufactured are defective. Management is concerned that employee inattention is partially responsible for the high defect rate. In an effort to decrease the percentage of defective chips, management decides to offer incentives to employees who have lower defect rates on their shifts. The incentive program is instituted for one month. If successful, the company will continue with the incentive program.
-Based on the data they collected during the trial program, management found that a 95% confidence interval for the percentage of defective chips was (5.0%, 7.0%). What conclusion should management reach about the new incentive program? Explain.
-Based on the data they collected during the trial program, management found that a 95% confidence interval for the percentage of defective chips was (5.0%, 7.0%). What conclusion should management reach about the new incentive program? Explain.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
30
All 423 Wisconsin public schools were all given a rating by the Wisconsin Department of Public Instruction based on several variables. The mean rating reported was 71.5 and the standard deviation was 4.87. To do a follow-up study a random sample of 40 schools was selected. In this sample, the mean rating was 70.9. One of the researchers is alarmed, thinking the report may have been mistaken. Do you think this sample result is unusually low? Explain.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
31
Pew Research reports that 63% of the U.S. adult cell phone owners use their phone to go online. A company wants to target 16- to 24-year olds for advertising and they wonder if that age group has a similar pattern of phone use.
-The company wants to estimate the true percentage of 16- to 24-year old cell phone owners who use their phone to go online to within ±7.5%, with 95% confidence. How many cell phone owners in this age group should they sample?
-The company wants to estimate the true percentage of 16- to 24-year old cell phone owners who use their phone to go online to within ±7.5%, with 95% confidence. How many cell phone owners in this age group should they sample?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
32
A report on health care in the US said that 28% of Americans have experienced times when they haven't been able to afford medical care. A news organization randomly sampled 801 black Americans, of whom 38% reported that there had been times in the last year when they had not been able to afford medical care. Does this indicate that this problem is more severe among black Americans?
-Explain what your P-value means in this context.
-Explain what your P-value means in this context.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
33
In 2000, the United Nations claimed that there was a higher rate of illiteracy in men than in women from the country of
Qatar. A humanitarian organization went to Qatar to conduct a random sample. The results revealed that 45 out of 234
men and 42 out of 251 women were classified as illiterate on the same measurement test. Do these results indicate that the
United Nations findings were correct?
-Find a 95% confidence interval for the difference in the proportions of illiteracy in men and women from Qatar. Interpret your interval.
Qatar. A humanitarian organization went to Qatar to conduct a random sample. The results revealed that 45 out of 234
men and 42 out of 251 women were classified as illiterate on the same measurement test. Do these results indicate that the
United Nations findings were correct?
-Find a 95% confidence interval for the difference in the proportions of illiteracy in men and women from Qatar. Interpret your interval.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
34
Roadblocks From time to time police set up roadblocks to check cars to see if the safety inspection is up to date. At one such roadblock they issued tickets for expired inspection stickers to 22 of 628 cars they stopped.
a. Based on the results at this roadblock, construct and interpret a 95% confidence interval for the proportion of autos in that region whose safety inspections have expired.
b. Explain the meaning of "95% confidence" in part a.
a. Based on the results at this roadblock, construct and interpret a 95% confidence interval for the proportion of autos in that region whose safety inspections have expired.
b. Explain the meaning of "95% confidence" in part a.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
35
The owner of a small clothing store is concerned that only 28% of people who enter her store actually buy something. A marketing salesman suggests that she invest in a new line of celebrity mannequins (think Seth Rogan modeling the latest jeans…). He loans her several different "people" to scatter around the store for a two-week trial period. The owner carefully counts how many shoppers enter the store and how many buy something so that at the end of the trial she can decide if she'll purchase the mannequins. She'll buy the mannequins if there is evidence that the percentage of people that buy something increases.
-In this context describe a Type II error and the impact such an error would have on the store.
-In this context describe a Type II error and the impact such an error would have on the store.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
36
Health care Organizations like Gallup often track public opinion on many issues by asking the same question at different times. In October of 2013, Gallup reported that 52% of U.S. adults disapprove of the Affordable Care Act (known as Obamacare), which is up from
45% a year earlier. Assume these were both based on random samples of 450 people. Use a significance test to determine if this is evidence of a real change in public opinion.
45% a year earlier. Assume these were both based on random samples of 450 people. Use a significance test to determine if this is evidence of a real change in public opinion.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
37
Herpetologists (snake specialists) found that a certain species of reticulated python has an average length of 20.5 feet with a standard deviation of 2.3 feet. The scientists collect a random sample of 30 adult pythons and measure their lengths. In their sample the mean length was 19.5 feet long. One of the herpetologists fears that pollution might be affecting the natural growth of the pythons. Do you think this sample result is unusually small? Explain.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
38
In 2000, the United Nations claimed that there was a higher rate of illiteracy in men than in women from the country of
Qatar. A humanitarian organization went to Qatar to conduct a random sample. The results revealed that 45 out of 234
men and 42 out of 251 women were classified as illiterate on the same measurement test. Do these results indicate that the
United Nations findings were correct?
-Test an appropriate hypothesis and state your conclusion.
Qatar. A humanitarian organization went to Qatar to conduct a random sample. The results revealed that 45 out of 234
men and 42 out of 251 women were classified as illiterate on the same measurement test. Do these results indicate that the
United Nations findings were correct?
-Test an appropriate hypothesis and state your conclusion.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
39
The International Olympic Committee states that the female participation in the 2004 Summer Olympic Games was 42%, even with new sports such as weight lifting, hammer throw, and modern pentathlon being added to the Games. Broadcasting and clothing companies want to change their advertising and marketing strategies if the female participation increases at the next games. An independent sports expert arranged for a random sample of pre-Olympic
exhibitions. The sports expert reported that 202 of 454 athletes in the random sample were women. Is this strong evidence that the participation rate may increase?
-Test an appropriate hypothesis and state your conclusion.
exhibitions. The sports expert reported that 202 of 454 athletes in the random sample were women. Is this strong evidence that the participation rate may increase?
-Test an appropriate hypothesis and state your conclusion.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
40
Pew Research found that, in 2013, 50% of American adults favored allowing same-sex couples to marry legally. This is up from 48% in 2012. The 2013 estimate was based on a random sample of 1,501 adults. Assume the same sample size was used in 2012. ["Changing Attitudes on Gay Marriage," Pew Internet and American Life Project, June 2013.]
-Does this interval provide evidence that the proportion of people who favor allowing same-sex couples to marry has increased?
-Does this interval provide evidence that the proportion of people who favor allowing same-sex couples to marry has increased?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
41
According to the 2010 census, 20.3% of the population of the United States (ages 5 and up) live in a home in which a language other than English is spoken. Advocates for providing government programs to assist non-English speakers are convinced that, with the increasing non-white population in the United States, this proportion has probably increased. They plan to conduct a survey, and if they find the proportion of people who live in such homes has increased, they will organize a campaign to increase government investment in these assistance programs.
-In the larger sample the proportion of people living in a home in which a language other than English is spoken was 20.8%. The consultant decided this increase was statistically significant. Now that the group is convinced the proportion has increased, why might they still choose not to organize the campaign?
-In the larger sample the proportion of people living in a home in which a language other than English is spoken was 20.8%. The consultant decided this increase was statistically significant. Now that the group is convinced the proportion has increased, why might they still choose not to organize the campaign?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
42
Employment program A city council must decide whether to fund a new
"welfare-to-work" program to assist long-time unemployed people in finding jobs. This
program would help clients fill out job applications and give them advice about dealing with job interviews. A six-month trial has just ended. At the start of this trial a number of unemployed residents were randomly divided into two groups; one group went through the help program and the other group did not. Data about employment at the end of this
trial are shown in the table. Should the city council fund this program? Test an appropriate hypothesis and state your conclusion.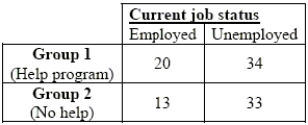
"welfare-to-work" program to assist long-time unemployed people in finding jobs. This
program would help clients fill out job applications and give them advice about dealing with job interviews. A six-month trial has just ended. At the start of this trial a number of unemployed residents were randomly divided into two groups; one group went through the help program and the other group did not. Data about employment at the end of this
trial are shown in the table. Should the city council fund this program? Test an appropriate hypothesis and state your conclusion.


Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
43
Baldness and heart attacks A recent medical study observed a higher frequency of heart attacks among a group of bald men than among another group of men who were not bald. Based on a P-value of 0.062 the researchers concluded there was some evidence that male baldness may be a risk factor for predicting heart attacks. Explain in context what their
P-value means.
P-value means.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
44
According to the 2010 census, 20.3% of the population of the United States (ages 5 and up) live in a home in which a language other than English is spoken. Advocates for providing government programs to assist non-English speakers are convinced that, with the increasing non-white population in the United States, this proportion has probably increased. They plan to conduct a survey, and if they find the proportion of people who live in such homes has increased, they will organize a campaign to increase government investment in these assistance programs.
-Write the advocates' null and alternative hypotheses.
-Write the advocates' null and alternative hypotheses.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
45
The International Olympic Committee states that the female participation in the 2004 Summer Olympic Games was 42%, even with new sports such as weight lifting, hammer throw, and modern pentathlon being added to the Games. Broadcasting and clothing companies want to change their advertising and marketing strategies if the female participation increases at the next games. An independent sports expert arranged for a random sample of pre-Olympic exhibitions. The sports expert reported that 202 of 454 athletes in the random sample were women. Is this strong evidence that the participation rate may increase?
-Was your test one-tail upper tail, lower tail, or two-tail? Explain why you choose that kind of test in this situation.
-Was your test one-tail upper tail, lower tail, or two-tail? Explain why you choose that kind of test in this situation.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
46
Tax advice Each year people who have income file income tax reports with the government. In some instances people seek advice from accountants and financial advisors regarding their income tax situations. This advice is meant to lower the percentage of taxes paid to the government each year. A random sample of people who filed tax reports resulted in the data in the table below. Does this data indicate that people should seek tax advice from an accountant or financial advisor? Test an appropriate hypothesis and state your conclusion. 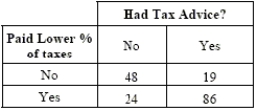


Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
47
Internet access A recent Gallup poll found that 28% of U.S. teens aged 13-17 have a computer with Internet access in their rooms. The poll was based on a random sample of
1028 teens and reported a margin of error of ±3%. What level of confidence did Gallup use for this poll?
1028 teens and reported a margin of error of ±3%. What level of confidence did Gallup use for this poll?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
48
The board of directors for Procter and Gamble is concerned that only 19.5% of the people who use toothpaste buy Crest toothpaste. A marketing director suggests that the company invest in a new marketing campaign which will include advertisements and new labeling for the toothpaste. The research department conducts product trials in test markets for one month to determine if the market share increases with new labels.
-Write the company's null and alternative hypotheses.
-Write the company's null and alternative hypotheses.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
49
The board of directors for Procter and Gamble is concerned that only 19.5% of the people who use toothpaste buy Crest toothpaste. A marketing director suggests that the company invest in a new marketing campaign which will include advertisements and new labeling for the toothpaste. The research department conducts product trials in test markets for one month to determine if the market share increases with new labels.
-The board of directors asked the research department to extend the trial period so that the decision can be made on two months worth of data. Will the power increase, decrease, or remain the same?
-The board of directors asked the research department to extend the trial period so that the decision can be made on two months worth of data. Will the power increase, decrease, or remain the same?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
50
Sleep Do more than 50% of U.S. adults feel they get enough sleep? According to Gallup's December 2004 Lifestyle poll, 55% of U.S. adults said that that they get enough sleep. The poll was based on a random sample of 1003 U.S. adults. Test an appropriate hypothesis and state your conclusion in the context of the problem.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
51
A report on health care in the US said that 28% of Americans have experienced times when they haven't been able to afford medical care. A news organization randomly sampled 801 black Americans, of whom 38% reported that there had been times in the last year when they had not been able to afford medical care. Does this indicate that this problem is more severe among black Americans?
-Was your test one-tail upper tail, one-tail lower tail, or two-tail? Explain why you chose that kind of test in this situation.
-Was your test one-tail upper tail, one-tail lower tail, or two-tail? Explain why you chose that kind of test in this situation.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
52
A state's Department of Education reports that 12% of the high school students in that state attend private high schools. The State University wonders if the percentage is the same in their applicant pool. Admissions officers plan to check a random sample of the over 10,000 applications on file to estimate the percentage of students applying for admission who attend private schools.
-Interpret the confidence interval in this context.
-Interpret the confidence interval in this context.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
53
A company manufacturing computer chips finds that 8% of all chips manufactured are defective. Management is concerned that employee inattention is partially responsible for the high defect rate. In an effort to decrease the percentage of defective chips, management decides to offer incentives to employees who have lower defect rates on their shifts. The incentive program is instituted for one month. If successful, the company will continue with the incentive program.
-In this context describe a Type I error and the impact such an error would have on the company.
-In this context describe a Type I error and the impact such an error would have on the company.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
54
A company manufacturing computer chips finds that 8% of all chips manufactured are defective. Management is concerned that employee inattention is partially responsible for the high defect rate. In an effort to decrease the percentage of defective chips, management decides to offer incentives to employees who have lower defect rates on their shifts. The incentive program is instituted for one month. If successful, the company will continue with the incentive program.
-Management decided to extend the incentive program so that the decision can be made on three months of data instead. Will the power increase, decrease, or remain the same?
-Management decided to extend the incentive program so that the decision can be made on three months of data instead. Will the power increase, decrease, or remain the same?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
55
Wildlife scientists studying a certain species of frogs know that past records indicate the adults should weigh an average of 118 grams with a standard deviation of 14 grams. The researchers collect a random sample of 50 adult frogs and weigh them. In their sample the mean weight was only 110 grams. One of the scientists is alarmed, fearing that environmental changes may be adversely affecting the frogs. Do you think this sample result is unusually low? Explain.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
56
Explain what your P-value means in this context.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
57
A company manufacturing computer chips finds that 8% of all chips manufactured are defective. Management is concerned that employee inattention is partially responsible for the high defect rate. In an effort to decrease the percentage of defective chips, management decides to offer incentives to employees who have lower defect rates on their shifts. The incentive program is instituted for one month. If successful, the company will continue with the incentive program.
-In this context describe a Type II error and the impact such an error would have on the company.
-In this context describe a Type II error and the impact such an error would have on the company.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
58
The owner of a small clothing store is concerned that only 28% of people who enter her store actually buy something. A marketing salesman suggests that she invest in a new line of celebrity mannequins (think Seth Rogan modeling the latest jeans…). He loans her several different "people" to scatter around the store for a two-week trial period. The owner carefully counts how many shoppers enter the store and how many buy something so that at the end of the trial she can decide if she'll purchase the mannequins. She'll buy the mannequins if there is evidence that the percentage of people that buy something increases.
-In this context describe a Type I error and the impact such an error would have on the store.
-In this context describe a Type I error and the impact such an error would have on the store.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
59
According to the 2010 census, 20.3% of the population of the United States (ages 5 and up) live in a home in which a language other than English is spoken. Advocates for providing government programs to assist non-English speakers are convinced that, with the increasing non-white population in the United States, this proportion has probably increased. They plan to conduct a survey, and if they find the proportion of people who live in such homes has increased, they will organize a campaign to increase government investment in these assistance programs.
-A consultant talked the group into gathering a larger sample. Will the power of the test increase, decrease, or remain the same?
-A consultant talked the group into gathering a larger sample. Will the power of the test increase, decrease, or remain the same?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
60
The board of directors for Procter and Gamble is concerned that only 19.5% of the people who use toothpaste buy Crest toothpaste. A marketing director suggests that the company invest in a new marketing campaign which will include advertisements and new labeling for the toothpaste. The research department conducts product trials in test markets for one month to determine if the market share increases with new labels.
-In this context describe a Type I error and the impact such an error would have on the company.
-In this context describe a Type I error and the impact such an error would have on the company.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
61
Approval rating The President's job approval rating is always a hot topic. Your local paper conducts a poll of 100 randomly selected adults to determine the President's job approval rating. A CNN/USA Today/Gallup poll conducts a poll of 1010 randomly selected adults. Which poll is more likely to report that the President's approval rating is below
50%, assuming that his actual approval rating is 54%? Explain.
50%, assuming that his actual approval rating is 54%? Explain.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
62
Researchers conduct a study to test a potential side effect of a new allergy medication. A random sample of 160 subjects with allergies was selected for the study. The new "improved" Brand I medication was randomly assigned to 80 subjects, and the current Brand C medication was randomly assigned to the other 80 subjects. 14 of the 80 patients with Brand I reported drowsiness, and 22 of the 80 patients with Brand C reported drowsiness.
-Does the interval in question 1 provide evidence that the side effect of drowsiness is different with the new medication?
-Does the interval in question 1 provide evidence that the side effect of drowsiness is different with the new medication?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
63
The countries of Europe report that 46% of the labor force is female. The United Nations wonders if the percentage of females in the labor force is the same in the United States. Representatives from the United States Department of Labor plan to check a random sample of over 10,000 employment records on file to estimate a percentage of females in the United States labor force.
-Should the representatives from the Department of Labor conclude that the percentage of females in their labor force is lower than Europe's rate of 46%? Explain.
-Should the representatives from the Department of Labor conclude that the percentage of females in their labor force is lower than Europe's rate of 46%? Explain.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
64
A report on health care in the US said that 28% of Americans have experienced times when they haven't been able to afford medical care. A news organization randomly sampled 801 black Americans, of whom 38% reported that there had been times in the last year when they had not been able to afford medical care. Does this indicate that this problem is more severe among black Americans?
-Test an appropriate hypothesis and state your conclusion. (Make sure to check any necessary conditions and to state a conclusion in the context of the problem.)
-Test an appropriate hypothesis and state your conclusion. (Make sure to check any necessary conditions and to state a conclusion in the context of the problem.)

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
65
The average composite ACT score for Ohio students who took the test in 2003 was 21.4.Assume that the standard deviation is 1.05. In a random sample of 25 students who took the exam in 2003, what is the probability that the average composite ACT score is 22 or more? (Make sure to identify the sampling distribution you use and check all necessary conditions.)

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
66
Pew Research reports that 63% of the U.S. adult cell phone owners use their phone to go online. A company wants to target 16- to 24-year olds for advertising and they wonder if that age group has a similar pattern of phone use
-They ignore your advice in Question 1 and just select a random sample of 300 cell phone users aged 16 to 24, and find that 206 of those surveyed use their phone to go online. Create the confidence interval.
-They ignore your advice in Question 1 and just select a random sample of 300 cell phone users aged 16 to 24, and find that 206 of those surveyed use their phone to go online. Create the confidence interval.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
67
According to the 2010 census, 20.3% of the population of the United States (ages 5 and up) live in a home in which a language other than English is spoken. Advocates for providing government programs to assist non-English speakers are convinced that, with the increasing non-white population in the United States, this proportion has probably increased. They plan to conduct a survey, and if they find the proportion of people who live in such homes has increased, they will organize a campaign to increase government investment in these assistance programs.
-In this context describe a Type I error and the impact such an error would have on this group's efforts.
-In this context describe a Type I error and the impact such an error would have on this group's efforts.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
68
According to the 2010 U.S. Census, 11.7% of the people in the state of Oregon were Hispanic or Latino. A political party wants to know how much impact the Hispanic and Latino vote will have, so they wonder if that percentage has changed since then. They take a random sample of 853 adults in Oregon and ask, among other things, their race. 113 of the people surveyed were Hispanic or Latino. Can the political party conclude that the Hispanic proportion of the population has changed since 2010?
-Explain what your P-value means in this context.
-Explain what your P-value means in this context.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
69
The countries of Europe report that 46% of the labor force is female. The United Nations wonders if the percentage of females in the labor force is the same in the United States. Representatives from the United States Department of Labor plan to check a random sample of over 10,000 employment records on file to estimate a percentage of females in the United States labor force.
-Interpret the confidence interval in this context.
-Interpret the confidence interval in this context.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
70
The countries of Europe report that 46% of the labor force is female. The United Nations wonders if the percentage of females in the labor force is the same in the United States. Representatives from the United States Department of Labor plan to check a random sample of over 10,000 employment records on file to estimate a percentage of females in the United States labor force.
-Explain what 90% confidence means in this context.
-Explain what 90% confidence means in this context.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
71
The owner of a small clothing store is concerned that only 28% of people who enter her store actually buy something. A marketing salesman suggests that she invest in a new line of celebrity mannequins (think Seth Rogan modeling the latest jeans…). He loans her several different "people" to scatter around the store for a two-week trial period. The owner carefully counts how many shoppers enter the store and how many buy something so that at the end of the trial she can decide if she'll purchase the mannequins. She'll buy the mannequins if there is evidence that the percentage of people that buy something increases.
-The owner talked the salesman into extending the trial period so that she can base her decision on data for a full month. Will the power of the test increase, decrease, or remain the same?
-The owner talked the salesman into extending the trial period so that she can base her decision on data for a full month. Will the power of the test increase, decrease, or remain the same?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
72
A company manufacturing computer chips finds that 8% of all chips manufactured are defective. Management is concerned that employee inattention is partially responsible for the high defect rate. In an effort to decrease the percentage of defective chips, management decides to offer incentives to employees who have lower defect rates on their shifts. The incentive program is instituted for one month. If successful, the company will continue with the incentive program.
-Write the company's null and alternative hypotheses.
-Write the company's null and alternative hypotheses.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
73
Births A city has two hospitals, with many more births recorded at the larger hospital than at the smaller one. Records indicate that in general babies are about equally likely to be boys or girls, but the actual gender ratio varies from week to week. Which hospital is more likely to report a week when over two-thirds of the babies born were girls? Explain.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
74
Pew Research reports that 63% of the U.S. adult cell phone owners use their phone to go online. A company wants to target 16- to 24-year olds for advertising and they wonder if that age group has a similar pattern of phone use.
-Explain what 95% confidence means in this context.
-Explain what 95% confidence means in this context.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
75
Hamsters You have ten hamsters. Their weights in grams are 134, 142, 148, 151, 152, 155,158, 160, 164, 167. Describe a procedure to create a simulated sampling distribution of the sample maximum weight for samples of three hamsters.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
76
Cereal A box of Raspberry Crunch cereal contains a mean of 13 ounces with a standard deviation of 0.5 ounce. The distribution of the contents of cereal boxes is approximately Normal. What is the probability that a case of 12 cereal boxes contains a total of more than
160 ounces?
160 ounces?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
77
A statistics professor asked her students whether or not they were registered to vote. In a sample of 50 of her students
(randomly sampled from her 700 students), 35 said they were registered to vote.
-What is the probability that the true proportion of the professor's students who were registered to vote is in your confidence interval?
(randomly sampled from her 700 students), 35 said they were registered to vote.
-What is the probability that the true proportion of the professor's students who were registered to vote is in your confidence interval?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
78
According to the Centers for Disease Control, about 36% of U.S. adults were obese in 2013.
To see if that has changed since the last study, a random sample of 250 U.S. adults will be checked.
a. Describe the sampling distribution model for the sample proportion (assuming no change in obesity rates) by naming the model and telling its mean and standard deviation. Justify your answer.
b. Sketch and clearly label the model.
c. What is the probability that in this group less than 25% of the adults will be found to be obese?
To see if that has changed since the last study, a random sample of 250 U.S. adults will be checked.
a. Describe the sampling distribution model for the sample proportion (assuming no change in obesity rates) by naming the model and telling its mean and standard deviation. Justify your answer.
b. Sketch and clearly label the model.
c. What is the probability that in this group less than 25% of the adults will be found to be obese?

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
79
According to Gallup, about 33% of Americans polled said they frequently experience stress in their daily lives. Suppose you are in a class of 45 students.
a. What is the probability that no more than 12 students in the class will say that they frequently experience stress in their daily lives? (Make sure to identify the sampling distribution you use and check all necessary conditions.)
b. If 20 students in the class said they frequently experience stress in their daily lives, would you be surprised? Explain, and use statistics to support your answer.
a. What is the probability that no more than 12 students in the class will say that they frequently experience stress in their daily lives? (Make sure to identify the sampling distribution you use and check all necessary conditions.)
b. If 20 students in the class said they frequently experience stress in their daily lives, would you be surprised? Explain, and use statistics to support your answer.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
80
The board of directors for Procter and Gamble is concerned that only 19.5% of the people who use toothpaste buy Crest toothpaste. A marketing director suggests that the company invest in a new marketing campaign which will include advertisements and new labeling for the toothpaste. The research department conducts product trials in test markets for one month to determine if the market share increases with new labels.
-Describe to the board of directors an advantage and a disadvantage of using a 5% alpha level of significance instead.
-Describe to the board of directors an advantage and a disadvantage of using a 5% alpha level of significance instead.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck



