Deck 16: Multiple Regression
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/122
Play
Full screen (f)
Deck 16: Multiple Regression
1
In a multiple regression model, the mean of the probability distribution of the error variable is assumed to be:
A)1.0.
B)0.0.
C)any value greater than 1.
D)k, where k is the number of independent variables included in the model.
A)1.0.
B)0.0.
C)any value greater than 1.
D)k, where k is the number of independent variables included in the model.
0.0.
2
When the independent variables are correlated with one another in a multiple regression analysis, this condition is called:
A)multicollinearity.
B)homoscedasticity.
C)heteroscedasticity.
D)linearity.
A)multicollinearity.
B)homoscedasticity.
C)heteroscedasticity.
D)linearity.
multicollinearity.
3
In a multiple regression analysis, when there is no linear relationship between each of the independent variables and the dependent variable, then:
A)multiple t-tests of the individual coefficients will likely show some are significant.
B)we will conclude erroneously that the model has some validity.
C)the chance of erroneously concluding that the model is useful is substantially less with the F-test than with multiple t-tests.
D)All of these choices are correct.
A)multiple t-tests of the individual coefficients will likely show some are significant.
B)we will conclude erroneously that the model has some validity.
C)the chance of erroneously concluding that the model is useful is substantially less with the F-test than with multiple t-tests.
D)All of these choices are correct.
All of these choices are correct.
4
A multiple regression model involves8 independent variables and 32 observations. If we want to test at the 5% significance level the parameter , the critical value will be:
A)1.714.
B)2.042.
C)2.064.
D)2.069.
A)1.714.
B)2.042.
C)2.064.
D)2.069.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
5
In a multiple regression model, the standard deviation of the error variable is assumed to be:
A)constant for all values of the independent variables.
B)constant for all values of the dependent variable.
C)1.0.
D)None of these choices are correct.
A)constant for all values of the independent variables.
B)constant for all values of the dependent variable.
C)1.0.
D)None of these choices are correct.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
6
In order to test the validity of a multiple regression model involving 4 independent variables and 35 observations, the numbers of degrees of freedom for the numerator and denominator, respectively, for the critical value of F are:
A)4 and 35.
B)3 and 32.
C)3 and 34.
D)4 and 30.
A)4 and 35.
B)3 and 32.
C)3 and 34.
D)4 and 30.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
7
In a multiple regression analysis involving 30 data points, the standard error of estimate squared is calculated as s 2 = 1.5 and the sum of squares for error as SSE = 36. The number of the independent variables must be:
A)6
B)5
C)4
D)3.
A)6
B)5
C)4
D)3.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
8
To test the validity of a multiple regression model involving 2 independent variables, the null hypothesis is that:
A) 0 = 1 = 2.
B) 0 = 1 = 2 = 0.
C) 1 = 2 = 0.
D) 1 = 2.
A) 0 = 1 = 2.
B) 0 = 1 = 2 = 0.
C) 1 = 2 = 0.
D) 1 = 2.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
9
An estimated multiple regression model has the form ŷ = 8 + 3x1 - 5x2 -4x3. As x1 increases by 1 unit, with x2 and x3 held constant, the value of y, on average, is estimated to:
A)decrease by 3 unit.
B)increase by 3 units.
C)decrease by 6 units.
D)increase by 11 units.
A)decrease by 3 unit.
B)increase by 3 units.
C)decrease by 6 units.
D)increase by 11 units.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
10
The problem of multicollinearity arises when the:
A)dependent variables are highly correlated with one another.
B)independent variables are highly correlated with one another.
C)independent variables are highly correlated with the dependent variable.
D)dependent variables are highly correlated with one another or independent variables are highly correlated with one another..
A)dependent variables are highly correlated with one another.
B)independent variables are highly correlated with one another.
C)independent variables are highly correlated with the dependent variable.
D)dependent variables are highly correlated with one another or independent variables are highly correlated with one another..

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
11
A multiple regression model involves 5 independent variables and the sample size is 30. If we want to test the validity of the model at the 5% significance level, the critical value is:
A)2.59.
B)2.53.
C)2.62.
D)2.56.
A)2.59.
B)2.53.
C)2.62.
D)2.56.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
12
In multiple regression models, the values of the error variable are assumed to be:
A)autocorrelated.
B)dependent on each other.
C)independent of each other.
D)always positive.
A)autocorrelated.
B)dependent on each other.
C)independent of each other.
D)always positive.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
13
Which of the following is used to test the significance of the overall regression equation?
A)t-test..
B)z-test.
C)χ2 test
D)F-test
A)t-test..
B)z-test.
C)χ2 test
D)F-test

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
14
In a multiple regression analysis involving k independent variables and n data points, the number of degrees of freedom associated with the sum of squares for regression is:
A)k.
B)n - k.
C)k - 1.
D)n - k - 1.
A)k.
B)n - k.
C)k - 1.
D)n - k - 1.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
15
The adjusted coefficient of determination is adjusted for the:
A)number of regression parameters including the y-intercept.
B)number of dependent variables and the sample size.
C)number of independent variables and the sample size.
D)coefficient of correlation and the significance level.
A)number of regression parameters including the y-intercept.
B)number of dependent variables and the sample size.
C)number of independent variables and the sample size.
D)coefficient of correlation and the significance level.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
16
In a multiple regression analysis, if the model provides a poor fit, this indicates that:
A)the sum of squares for error will be large.
B)the standard error of estimate will be large.
C)the coefficient of determination will be close to zero.
D)All of these choices are correct.
A)the sum of squares for error will be large.
B)the standard error of estimate will be large.
C)the coefficient of determination will be close to zero.
D)All of these choices are correct.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
17
Which of the following statements is not true?
A)Multicollinearity exists in virtually all multiple regression models.
B)Multicollinearity is also called collinearity and intercorrelation.
C)Multicollinearity is a condition that exists when the independent variables are highly correlated with the dependent variable.
D)Multicollinearity does not affect the F-test of the analysis of variance.
A)Multicollinearity exists in virtually all multiple regression models.
B)Multicollinearity is also called collinearity and intercorrelation.
C)Multicollinearity is a condition that exists when the independent variables are highly correlated with the dependent variable.
D)Multicollinearity does not affect the F-test of the analysis of variance.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
18
Which of the following best describes the ratio MSR/MSE in a multiple linear regression model?
A)Sum of squares of the residuals.
B)t-test statistic to test the individual regression coefficients of the independent variables.
C)F-test to test the overall significance of the regression model.
D)Adjusted coefficient of determination.
A)Sum of squares of the residuals.
B)t-test statistic to test the individual regression coefficients of the independent variables.
C)F-test to test the overall significance of the regression model.
D)Adjusted coefficient of determination.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
19
In a multiple regression analysis involving 6 independent variables and a sample of 19 data points the total variation in y is SST = 900 and the amount of variation in y that is explained by the variations in the independent variables is SSR = 600. The value of the F-test statistic for this model is:
A)4.0.
B)4.3.
C)4.8.
D)6.3.
A)4.0.
B)4.3.
C)4.8.
D)6.3.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
20
Which of the following best explains a small F-statistic when testing the validity of a multiple regression model?
A)Most of the variation in x is unexplained by the regression equation.
B)Most of the variation in y is explained by the regression equation.
C)The model provides a good fit.
D)Most of the variation in y is unexplained by the regression equation.
A)Most of the variation in x is unexplained by the regression equation.
B)Most of the variation in y is explained by the regression equation.
C)The model provides a good fit.
D)Most of the variation in y is unexplained by the regression equation.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
21
An estimated multiple regression model has the form Which of the following best describes ?
A)We estimate for each one unit increase in x2, that y will increase by units, on average.
B)Whilst holding x1 constant, we estimate for each one unit increase in x2, that y will increase by units, on average.
C)Whilst holding x2 constant, we estimate for each one unit increase in x1, that y will increase by units, on average
D)Whilst holding x1 constant, we estimate for each one unit increase in x2, that y will increase by units, on average .
A)We estimate for each one unit increase in x2, that y will increase by units, on average.
B)Whilst holding x1 constant, we estimate for each one unit increase in x2, that y will increase by units, on average.
C)Whilst holding x2 constant, we estimate for each one unit increase in x1, that y will increase by units, on average
D)Whilst holding x1 constant, we estimate for each one unit increase in x2, that y will increase by units, on average .

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
22
An estimated multiple regression model has the form ŷ = 100 − 2x1 + 9x2. As x1 increases by 1 unit while holding x2 constant, which of the following best describes the change in y?
A)y will increase by 2 units, estimated, on average.
B)y will decrease by 98 units, estimated, on average.
C)y will increase by 98 units, estimated, on average.
D)y will decrease by 2 units, estimated, on average.
A)y will increase by 2 units, estimated, on average.
B)y will decrease by 98 units, estimated, on average.
C)y will increase by 98 units, estimated, on average.
D)y will decrease by 2 units, estimated, on average.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
23
If none of the data points for a multiple regression model with two independent variables were on the regression plane, then the coefficient of determination would be:
A)-1.0.
B)1.0.
C)any number between -1 and 1, inclusive.
D)any number greater than or equal to zero but smaller than 1.
A)-1.0.
B)1.0.
C)any number between -1 and 1, inclusive.
D)any number greater than or equal to zero but smaller than 1.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
24
Which of the following best describes a multiple linear regression model?
A)A multiple linear regression model has more than one dependent variable.
B)A multiple linear regression model has more than one independent variable.
C)A multiple linear regression model must have more than one independent variable and more than one independent variable.
D)A multiple linear regression model has one independent variable.
A)A multiple linear regression model has more than one dependent variable.
B)A multiple linear regression model has more than one independent variable.
C)A multiple linear regression model must have more than one independent variable and more than one independent variable.
D)A multiple linear regression model has one independent variable.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
25
If multicollinearity exists among the independent variables included in a multiple regression model, then:
A)regression coefficients will be difficult to interpret.
B)the standard errors of the regression coefficients for the correlated independent variables will increase.
C)coefficient of determination will assume a value close to zero.
D)the standard errors of the regression coefficients for the correlated independent variables will increase and coefficient of determination will assume a value close to zero.
A)regression coefficients will be difficult to interpret.
B)the standard errors of the regression coefficients for the correlated independent variables will increase.
C)coefficient of determination will assume a value close to zero.
D)the standard errors of the regression coefficients for the correlated independent variables will increase and coefficient of determination will assume a value close to zero.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
26
In a multiple regression model, the error variable is assumed to have a mean of:
A)-1.0.
B)0.0.
C)1.0.
D)any value smaller than -1.0.
A)-1.0.
B)0.0.
C)1.0.
D)any value smaller than -1.0.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
27
For a multiple regression model:
A)SST = SSR - SSE.
B)SSE = SSR - SST.
C)SSR = SSE - SST.
D)SST = SSE + SSR.
A)SST = SSR - SSE.
B)SSE = SSR - SST.
C)SSR = SSE - SST.
D)SST = SSE + SSR.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
28
For a multiple regression model with n = 35 and k = 4, the following statistics are given: Total variation in y = SST = 500 and SSE = 100. The coefficient of determination is:
A)0.82.
B)0.80.
C)0.77.
D)0.20.
A)0.82.
B)0.80.
C)0.77.
D)0.20.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
29
For the estimated multiple regression model ŷ = 30 - 4x1 + 5x2 +3 x3, a one unit increase in x3, holding x1 and x2 constant, will result in which of the following changes in y?
A)y will increase by 3 units.
B)y will increase by 2 units, estimated, on average.
C)y will increase by 33 units
D)y will increase by 3 units, estimated, on average.
A)y will increase by 3 units.
B)y will increase by 2 units, estimated, on average.
C)y will increase by 33 units
D)y will increase by 3 units, estimated, on average.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
30
Which of the following measures can be used to assess a multiple regression model's fit?
A)The sum of squares for error.
B)The sum of squares for regression.
C)The standard error of estimate.
D)A single t-test.
A)The sum of squares for error.
B)The sum of squares for regression.
C)The standard error of estimate.
D)A single t-test.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
31
In a multiple regression model, the following statistics are given: SSE = 100, R2 = 0.995, k = 5, n = 15. The coefficient of determination adjusted for degrees of freedom is:
A)0.955.
B)0.992.
C)0.930.
D)None of these choices are correct.
A)0.955.
B)0.992.
C)0.930.
D)None of these choices are correct.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
32
The coefficient of determination is defined as:
A)SSE/SST.
B)MSE/MSR.
C)1 - (SSE/SST).
D)1 - (MSE/MSR).
A)SSE/SST.
B)MSE/MSR.
C)1 - (SSE/SST).
D)1 - (MSE/MSR).

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
33
The graphical depiction of the equation of a multiple regression model with k independent variables (k > 1) is referred to as:
A)a straight line.
B)the response variable.
C)the response surface.
D)a plane only when k = 3.
A)a straight line.
B)the response variable.
C)the response surface.
D)a plane only when k = 3.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
34
In a multiple regression analysis involving 40 observations and 5 independent variables, total variation in y = SST = 350 and SSE = 50. The coefficient of determination is:
A)0.8408.
B)0.8571.
C)0.8469.
D)0.8529.
A)0.8408.
B)0.8571.
C)0.8469.
D)0.8529.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
35
For a multiple regression model, the following statistics are given: Total variation in y = SST = 250, SSE = 50, k = 4, n = 20.
The coefficient of determination adjusted for degrees of freedom is:
A)0.800.
B)0.747.
C)0.840.
D)0.775.
The coefficient of determination adjusted for degrees of freedom is:
A)0.800.
B)0.747.
C)0.840.
D)0.775.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
36
Which of the following best describes the range of the coefficient of determination?
A)0 to 1
B)−1 to 1
C)1 to n, where n is the number of observations in the sample.
D)1 to k, where k is the number of independent variables in the model.
A)0 to 1
B)−1 to 1
C)1 to n, where n is the number of observations in the sample.
D)1 to k, where k is the number of independent variables in the model.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
37
Which of the following is not true when we add an independent variable to a multiple regression model?
A)The adjusted coefficient of determination can assume a negative value.
B)The unadjusted coefficient of determination always increases.
C)The unadjusted coefficient of determination may increase or decrease.
D)The adjusted coefficient of determination may increase.
A)The adjusted coefficient of determination can assume a negative value.
B)The unadjusted coefficient of determination always increases.
C)The unadjusted coefficient of determination may increase or decrease.
D)The adjusted coefficient of determination may increase.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
38
A multiple regression analysis involving 3 independent variables and 25 data points results in a value of 0.769 for the (unadjusted) coefficient of determination. The adjusted coefficient of determination is:
A)0.385.
B)0.877.
C)0.591.
D)0.736.
A)0.385.
B)0.877.
C)0.591.
D)0.736.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
39
In a multiple regression model, the probability distribution of the error variable is assumed to be:
A)normal.
B)non-normal.
C)positively skewed.
D)negatively skewed.
A)normal.
B)non-normal.
C)positively skewed.
D)negatively skewed.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
40
If all the points for a multiple regression model with two independent variables were on the regression plane, then the coefficient of determination would equal:
A) 0.
B) 1.
C) 2, since there are two independent variables.
D) any number between 0 and 2.
A) 0.
B) 1.
C) 2, since there are two independent variables.
D) any number between 0 and 2.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
41
For the multiple regression model = 75 + 25x1 - 15 x2 + 10 x3, if were to increase by 5, holding and constant, the value of y would:
A)increase by 5.
B)increase by 75.
C)decrease on average by 5.
D)decrease on average by 75.
A)increase by 5.
B)increase by 75.
C)decrease on average by 5.
D)decrease on average by 75.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
42
A multiple regression analysis that includes 4 independent variables results in a sum of squares for regression of 1200 and a sum of squares for error of 800. The coefficient of determination will be:
A)0.667.
B)0.600.
C)0.400.
D)0.200.
A)0.667.
B)0.600.
C)0.400.
D)0.200.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
43
In a regression model involving 60 observations, the following estimated regression model was obtained: = 51.4 + 0.70x1 + 0.679 x2 - 0.378 x3. For this model, total variation in y = SST = 119,724 and SSR = 29,029.72. The value of MSE is:
A)1619.541.
B)9676.572.
C)1995.400.
D)5020.235.
A)1619.541.
B)9676.572.
C)1995.400.
D)5020.235.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
44
In testing the validity of a multiple regression model involving 5 independent variables and 30 observations, the numbers of degrees of freedom for the numerator and denominator (respectively) for the critical value of F will be:
A)5 and 25.
B)24 and 5.
C)25 and 5.
D)5 and 24.
A)5 and 25.
B)24 and 5.
C)25 and 5.
D)5 and 24.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
45
For each x term in the multiple regression equation, the corresponding is referred to as a partial regression coefficient or slope of the independent variable.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
46
A multiple regression equation includes 5 independent variables, and the coefficient of determination is 0.64. The percentage of the variation in y that is explained by the regression equation is:
A)8%.
B)12.8%.
C)41%.
D)64%
A)8%.
B)12.8%.
C)41%.
D)64%

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
47
In a regression model involving 50 observations, the following estimated regression model was obtained: ŷ = 10.5 + 3.2x1 + 5.8x2 + 6.5x3. For this model, SSR = 450 and SSE = 175. The value of MSE is:
A)9.783.
B)58.333.
C)150.000.
D)3.804.
A)9.783.
B)58.333.
C)150.000.
D)3.804.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
48
Which of the following best describes first-order autocorrelation?
A)First-order autocorrelation is a condition in which there is no relationship between consecutive residuals.
B)First-order autocorrelation is a condition in which the data is skewed.
C)First-order autocorrelation is a condition in which consecutive residuals differ greatly.
D)First-order autocorrelation is a condition in which a relationship exists between consecutive residuals.
A)First-order autocorrelation is a condition in which there is no relationship between consecutive residuals.
B)First-order autocorrelation is a condition in which the data is skewed.
C)First-order autocorrelation is a condition in which consecutive residuals differ greatly.
D)First-order autocorrelation is a condition in which a relationship exists between consecutive residuals.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
49
A multiple regression analysis that includes 20 data points and 4 independent variables results in total variation in y = SST = 200 and SSR = 160. The multiple standard error of estimate will be:
A)0.80.
B)3.266.
C)3.651.
D)1.633.
A)0.80.
B)3.266.
C)3.651.
D)1.633.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
50
In testing the validity of a multiple regression model in which there are four independent variables, the null hypothesis is:
A) .
B) .
C) .
D) .
A) .
B) .
C) .
D) .

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
51
For a set of 30 data points, Excel has found the estimated multiple regression equation to be = -8.61 + 22x1 + 7x2 + 28x3, and has listed the t statistic for testing the significance of each regression coefficient. Using the 5% significance level for testing whether 3 = 0, the critical region will be that the absolute value of the t statistic for 3 is greater than or equal to:
A)2.056.
B)2.045.
C)1.703.
D)1.706.
A)2.056.
B)2.045.
C)1.703.
D)1.706.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
52
In multiple regression analysis involving 9 independent variables and 110 observations, the critical value of t for testing individual coefficients in the model will have:
A)109 degrees of freedom.
B)8 degrees of freedom.
C)99 degrees of freedom.
D)100 degrees of freedom.
A)109 degrees of freedom.
B)8 degrees of freedom.
C)99 degrees of freedom.
D)100 degrees of freedom.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
53
In a regression model involving 30 observations, the following estimated regression model was obtained: = 60 + 2.8x1 + 1.2 x2 - x3. For this model, total variation in y = SST = 800 and SSE = 200. The value of the F-statistic for testing the validity of this model is:
A)26.00.
B)7.69.
C)3.38.
D)0.039.
A)26.00.
B)7.69.
C)3.38.
D)0.039.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
54
Multicollinearity is a situation in which the independent variables are highly correlated with the dependent variable.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
55
In multiple regression, the descriptor 'multiple' refers to more than one independent variable.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
56
Which of the following best describes the Durbin-Watson test?
A)The Durbin-Watson test is used to determine if there is multicollinearity.
B)The Durbin-Watson test is used to determine if there is heteroscedasticity.
C)The Durbin-Watson test is used to determine if there is first-order autocorrelation.
D)The Durbin-Watson test is used to determine if there is homoscedasticity.
A)The Durbin-Watson test is used to determine if there is multicollinearity.
B)The Durbin-Watson test is used to determine if there is heteroscedasticity.
C)The Durbin-Watson test is used to determine if there is first-order autocorrelation.
D)The Durbin-Watson test is used to determine if there is homoscedasticity.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
57
In a multiple regression analysis, there are 20 data points and 4 independent variables, and the sum of the squared differences between observed and predicted values of y is 180. The multiple standard error of estimate will be:
A)6.708.
B)3.464.
C)9.000.
D)3.000.
A)6.708.
B)3.464.
C)9.000.
D)3.000.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
58
In a multiple regression analysis involving 20 observations and 5 independent variables, total variation in y = SST = 250 and SSE = 35. The coefficient of determination adjusted for degrees of freedom is:
A)0.810.
B)0.860.
C)0.835.
D)0.831.
A)0.810.
B)0.860.
C)0.835.
D)0.831.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
59
Multiple linear regression is used to estimate the linear relationship between one dependent variable and more than one independent variables.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
60
In a multiple regression analysis involving 25 data points and 5 independent variables, the sum of squares terms are calculated as: total variation in y = SST = 500, SSR = 300, and SSE = 200. In testing the validity of the regression model, the F-value of the test statistic will be:
A)5.70.
B)2.50.
C)1.50.
D)0.176.
A)5.70.
B)2.50.
C)1.50.
D)0.176.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
61
A multiple regression analysis that includes 25 data points and 4 independent variables produces SST = 400 and SSR = 300. The multiple standard error of estimate will be 5.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
62
The adjusted coefficient of determination is adjusted for the number of independent variables and the sample size.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
63
In regression analysis, we judge the magnitude of the standard error of estimate relative to the values of the dependent variable, and particularly to the mean of y.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
64
For the multiple regression model , if were to increase by 5 units, holding and constant, the value of would decrease by 50 units, on average.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
65
In reference to the equation = 1.86 - 0.51x1 + 0.60 x2, the value 0.60 is the change in per unit change in , regardless of the value of .

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
66
In order to test the significance of a multiple regression model involving 4 independent variables and 25 observations, the number of degrees of freedom for the numerator and denominator, respectively, for the critical value of F are 4 and 20, respectively.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
67
In a multiple regression analysis involving 4 independent variables and 30 data points, the number of degrees of freedom associated with the sum of squares for error, SSE, is 25.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
68
Given the multiple linear regression equation, the value of is the estimated average increase in y for a one unit increase in x2, whilst holding x1 constant.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
69
In regression analysis, the total variation in the dependent variable y, measured by , can be decomposed into two parts: the explained variation, measured by SSR, and the unexplained variation, measured by SSE.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
70
In a multiple regression problem involving 24 observations and three independent variables, the estimated regression equation is = 72 + 3.2x1 + 1.5 x2 - x3. For this model, SST = 800 and SSE = 245. The value of the F-statistic for testing the significance of this model is 15.102.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
71
In a multiple regression analysis involving 50 observations and 5 independent variables, SST = 475 and SSE = 71.25. The coefficient of determination is 0.85.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
72
In order to test the significance of a multiple regression model involving 4 independent variables and 30 observations, the number of degrees of freedom for the numerator and denominator for the critical value of F are 4 and 26, respectively.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
73
In testing the significance of a multiple regression model in which there are three independent variables, the null hypothesis is Ho: β0 = β1 = β2 = β3.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
74
Given the multiple linear regression equation, = -0.80 + 0.12 x1 + 0.08 x2, the value -0.80 is the intercept.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
75
A multiple regression model has the form 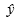 = 24 - 0.001x1 + 3x2.
= 24 - 0.001x1 + 3x2.
As x1 increases by 1 unit, holding constant, the value of y is estimated to decrease by 0.001units, on average.
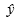 = 24 - 0.001x1 + 3x2.
= 24 - 0.001x1 + 3x2.As x1 increases by 1 unit, holding constant, the value of y is estimated to decrease by 0.001units, on average.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
76
A multiple regression model has the form The coefficient is interpreted as the change in per unit change in x2.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
77
A multiple regression model involves 40 observations and 4 independent variables produces
SST = 100 000 and SSR = 82,500. The value of MSE is 500.
SST = 100 000 and SSR = 82,500. The value of MSE is 500.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
78
In multiple regression, the standard error of estimate is defined by , where n is the sample size and k is the number of independent variables.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
79
Excel prints a second statistic, called the coefficient of determination adjusted for degrees of freedom, which has been adjusted to take into account the sample size and the number of independent variables.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
80
A multiple regression the coefficient of determination is 0.81. The percentage of the variation in that is explained by the regression equation is 81%.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck



