Deck 6: Integer, Goal, and Nonlinear Programming Models
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/55
Play
Full screen (f)
Deck 6: Integer, Goal, and Nonlinear Programming Models
1
An investment strategy restricts the portfolio to a mix of two stocks A and B with the following price/share and annual returns:
Assume that the maximum amount available for investment is $100,000 with the following 2 prioritized investment goals:
Rank R1: Obtain an annual return of at least 6%.
Rank R2: Limit the investment in stock B to no more than 55% of the total investment.
Assume X? = dollar amount allocated to stock A,and X? = dollar amount allocated to stock B.
What is the objective function?
A)Min d?? + d??
B)Min R?(d??)+ R?(d??)
C)Min R?(d??)- R?(d??)
D)Min R?(d??)+ R?(d??)
E)Min R?(d??)+ R?(d??)
Assume that the maximum amount available for investment is $100,000 with the following 2 prioritized investment goals:
Rank R1: Obtain an annual return of at least 6%.
Rank R2: Limit the investment in stock B to no more than 55% of the total investment.
Assume X? = dollar amount allocated to stock A,and X? = dollar amount allocated to stock B.
What is the objective function?
A)Min d?? + d??
B)Min R?(d??)+ R?(d??)
C)Min R?(d??)- R?(d??)
D)Min R?(d??)+ R?(d??)
E)Min R?(d??)+ R?(d??)
Min R?(d??)+ R?(d??)
2
Consider the following constraint and its associated binary variables: XA + XB = 1.
This constraint is an example of an if-then constraint.
This constraint is an example of an if-then constraint.
False
3
Which of the following statements about nonlinear programming is False?
A)Either the objective function or at least one constraint is nonlinear.
B)The optimal solution must be a corner point solution.
C)There can be both local and global optimal solutions.
D)There is no precise way to know where to start the solution search process.
A)Either the objective function or at least one constraint is nonlinear.
B)The optimal solution must be a corner point solution.
C)There can be both local and global optimal solutions.
D)There is no precise way to know where to start the solution search process.
B
4
The "bin" option is used in Solver to specify general integer variables.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
5
A company incurs a cost that is directly proportional to the magnitude of the decision variable.This is an example of a:
A)fixed charge
B)sunk cost
C)variable cost
D)marginal cost
E)inventory cost
A)fixed charge
B)sunk cost
C)variable cost
D)marginal cost
E)inventory cost

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
6
Consider the following constraint and its associated binary decision variables:
XA + XB + XC ≥ 2.
This constraint is an example of a mutually exclusive constraint.
XA + XB + XC ≥ 2.
This constraint is an example of a mutually exclusive constraint.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
7
Which type of cost is independent of the volume of production?
A)inventory cost
B)marginal cost
C)sunk cost
D)variable cost
E)fixed charge
A)inventory cost
B)marginal cost
C)sunk cost
D)variable cost
E)fixed charge

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
8
If a company produces product A then it must also produce either product B or product C.Which of the following constraints enforces this condition?
A)XA - XB - XC ≥ 0
B)XA + XB + XC ≤ 2
C)XA + (XB - XC)≤ 0
D)XA + XB + XC ≥ 2
E)XA - XB - XC ≤ 0
A)XA - XB - XC ≥ 0
B)XA + XB + XC ≤ 2
C)XA + (XB - XC)≤ 0
D)XA + XB + XC ≥ 2
E)XA - XB - XC ≤ 0

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
9
If a company produces Product A,then it must produce at least 200 units of Product A.Which of the following constraints model this condition?
A)X₁Y₁ ≤ 200
B)X₁ ≥ 200 + Y₁
C)X₁ ≤ 200Y₁
D)X₁ - 200Y₁ ≥ 0
E)X1 > 200
A)X₁Y₁ ≤ 200
B)X₁ ≥ 200 + Y₁
C)X₁ ≤ 200Y₁
D)X₁ - 200Y₁ ≥ 0
E)X1 > 200

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
10
Consider a model with 3 ranked goals.Solving this model requires us to solve:
A)a single LP problem
B)3 separate LP problems
C)2 separate LP problems
D)a single quadratic programming problem
E)3 quadratic programming problems
A)a single LP problem
B)3 separate LP problems
C)2 separate LP problems
D)a single quadratic programming problem
E)3 quadratic programming problems

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
11
These are problems in which some,but not all,the decision variables must have integer solutions:
A)mixed IP problems
B)pure IP problems
C)pure binary problems
D)mixed binary IP problems
E)goal programming problems
A)mixed IP problems
B)pure IP problems
C)pure binary problems
D)mixed binary IP problems
E)goal programming problems

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
12
Problems which can be stated as an assortment of desired objectives are known as:
A)quadratic programming
B)integer programming
C)goal programming
D)linear programming
E)binary programming
A)quadratic programming
B)integer programming
C)goal programming
D)linear programming
E)binary programming

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
13
Suppose that XA equals 10.What are the values for d₁⁺ and d₁⁻ in the following constraint?
XA + d₁⁻- d₁⁺ = 7
A)d₁⁻ = 0,d₁⁺ = 3
B)d₁⁻= 3,d₁⁺ = 0
C)d₁⁻ = 7,d₁⁺ = 0
D)d₁⁻ = 0,d₁⁺ = 7
E)d₁⁻ = 10,d₁⁺ = 3
XA + d₁⁻- d₁⁺ = 7
A)d₁⁻ = 0,d₁⁺ = 3
B)d₁⁻= 3,d₁⁺ = 0
C)d₁⁻ = 7,d₁⁺ = 0
D)d₁⁻ = 0,d₁⁺ = 7
E)d₁⁻ = 10,d₁⁺ = 3

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
14
A company wants to select one product from a set of 3 possible products.Which of the following ensures that only one product will be selected?
A)XA + XB + XC = 1
B)XA + XB + XC ≤ 1
C)XA + XB + XC ≥ 1
D)XA + XB + XC ≥ 0
E)XA - XB - XC = 1
A)XA + XB + XC = 1
B)XA + XB + XC ≤ 1
C)XA + XB + XC ≥ 1
D)XA + XB + XC ≥ 0
E)XA - XB - XC = 1

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
15
Rounding off the solution to an LP relaxed problem may yield:
A)an infeasible solution
B)a non-optimal solution
C)a higher objective function value
D)an unbounded solution
E)A or B
A)an infeasible solution
B)a non-optimal solution
C)a higher objective function value
D)an unbounded solution
E)A or B

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
16
Nonlinear programming models can be approximated by linear or almost linear models through a procedure known as ________.
A)separable programming
B)goal programming
C)integer programming
D)quadratic programming
E)mixed integer programming
A)separable programming
B)goal programming
C)integer programming
D)quadratic programming
E)mixed integer programming

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
17
The deviational variable di‾ indicates the amount by which a certain goal is:
A)overachieved
B)over attained
C)binding
D)underachieved
E)non-binding
A)overachieved
B)over attained
C)binding
D)underachieved
E)non-binding

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
18
Consider the following constraint that needs to be expressed as a goal:
3X₁ + 4X₂ ≤ 11.
The correct format is:
A)3X₁ + 4X₂ - d₁⁻ + d₁⁺ = 11
B)3X₁ + 4X₂ + d₁⁻ - d₁⁺ = 11
C)3X₁ + 4X₂ + d₁⁻ - d₁⁺ ≥ 11
D)3X₁ + 4X₂ + d₁⁻ - d₁⁺ ≤ 11
E)3X₁ + 4X₂ + d₁⁻ - d₁⁺ ≠ 11
3X₁ + 4X₂ ≤ 11.
The correct format is:
A)3X₁ + 4X₂ - d₁⁻ + d₁⁺ = 11
B)3X₁ + 4X₂ + d₁⁻ - d₁⁺ = 11
C)3X₁ + 4X₂ + d₁⁻ - d₁⁺ ≥ 11
D)3X₁ + 4X₂ + d₁⁻ - d₁⁺ ≤ 11
E)3X₁ + 4X₂ + d₁⁻ - d₁⁺ ≠ 11

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
19
These are problems in which all decision variables must have integer solutions:
A)mixed binary IP problems
B)pure binary IP problems
C)mixed IP problems
D)pure IP problems
E)goal programming problems
A)mixed binary IP problems
B)pure binary IP problems
C)mixed IP problems
D)pure IP problems
E)goal programming problems

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
20
A company wants to select 2 products from a set of four possible products.Which of the following constraints ensures that no more than 3 will be selected?
A)XA + XB + XC + XD = 3
B)XA + XB + XC + XD ≥ 0
C)XA + XB + XC + XD ≤ 3
D)XA + XB + XC + XD ≥ 3
E)XA + XB + XC + XD ≠ 3
A)XA + XB + XC + XD = 3
B)XA + XB + XC + XD ≥ 0
C)XA + XB + XC + XD ≤ 3
D)XA + XB + XC + XD ≥ 3
E)XA + XB + XC + XD ≠ 3

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
21
The optimal solution of to an NLP model must be a corner point of the feasible region.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
22
In mixed IP problems,some,but not all,of the decision variables have integer solutions.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
23
The deviational variable dᵢ⁻ typically denotes underachievement.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
24
In goal programming,the objective is typically to maximize the sum of the deviational variables.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
25
Use this information to answer the following questions.
A decision maker is provided with 5 different potential projects and must determine which projects to choose.The projects require different amounts of capital and different expected net present values (NPV)over the next three years.
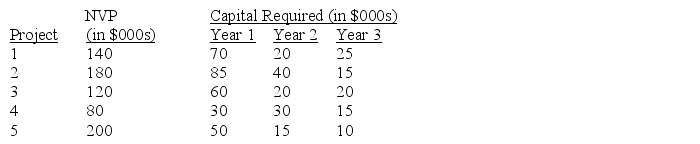
Determine which set of projects should be selected in order to achieve the maximum net present value if the decision maker has $150,000 available for investment each year.
A decision maker is provided with 5 different potential projects and must determine which projects to choose.The projects require different amounts of capital and different expected net present values (NPV)over the next three years.
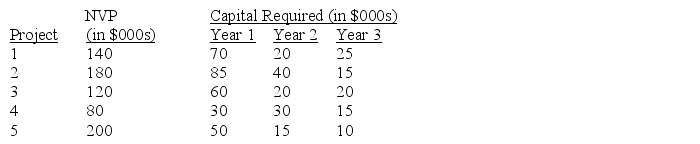
Determine which set of projects should be selected in order to achieve the maximum net present value if the decision maker has $150,000 available for investment each year.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
26
An NLP model can have both local and global optimal solutions.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
27
The IP solution can sometimes produce a better objective function value than its LP relaxed problem.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
28
Use this information for the following questions.
Six products are currently waiting processing on a single machine.The machine is available today for a maximum of 25 hours.The processing time per hour for each product along with the profit contribution per product is show below.

Refer to the information above.Which products should be processed to maximize the total profit contribution?
Six products are currently waiting processing on a single machine.The machine is available today for a maximum of 25 hours.The processing time per hour for each product along with the profit contribution per product is show below.

Refer to the information above.Which products should be processed to maximize the total profit contribution?

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
29
If overachievement is acceptable,the appropriate di⁺ variable can be dropped from the objective function.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
30
An integer programming problem assumes that its objective function and its constraints are linear.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
31
Use this information to answer the following questions.
A decision maker is provided with 5 different potential projects and must determine which projects to choose.The projects require different amounts of capital and different expected net present values (NPV)over the next three years.
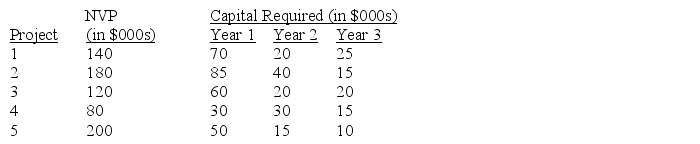
Refer to the information above.Determine which set of projects should be selected in order to achieve the maximum net present value if the following two conditions must also be met:
a)If project 1 is selected,then project 2 must be selected,and vice versa.
b)Since projects 4 and 5 require outsourcing various operations,the decision maker wants at most one of these projects to be included in the solution and not both.
A decision maker is provided with 5 different potential projects and must determine which projects to choose.The projects require different amounts of capital and different expected net present values (NPV)over the next three years.
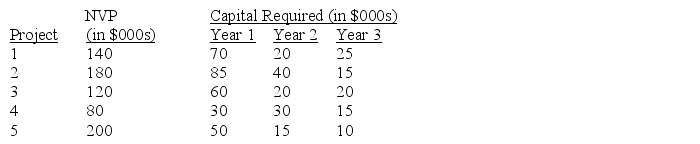
Refer to the information above.Determine which set of projects should be selected in order to achieve the maximum net present value if the following two conditions must also be met:
a)If project 1 is selected,then project 2 must be selected,and vice versa.
b)Since projects 4 and 5 require outsourcing various operations,the decision maker wants at most one of these projects to be included in the solution and not both.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
32
There is no precise way to know where to start the solution search process for an NLP model.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
33
Consider the following objective function with prioritized goals: Min: R₁(d₁⁻)+ R₂(d₁⁻).
This implies that goal 2 is of higher rank than goal 1.
This implies that goal 2 is of higher rank than goal 1.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
34
The optimal solution to an IP model must be at a corner point of the feasible region.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
35
Rounding off the solution to an LP relaxation problem may sometimes yield an infeasible solution to an IP problem.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
36
Use this information for the following questions.
Six products are currently waiting processing on a single machine.The machine is available today for a maximum of 25 hours.The processing time per hour for each product along with the profit contribution per product is show below.

Refer to the information above.Assume that the following conditions must be met:
a)We want to ensure that exactly one of these products: A,B,or C must be processed.
b)We want to ensure that if product E is processed then product F must be processed.
Which products should be processed to maximize the total profit contribution?
Six products are currently waiting processing on a single machine.The machine is available today for a maximum of 25 hours.The processing time per hour for each product along with the profit contribution per product is show below.

Refer to the information above.Assume that the following conditions must be met:
a)We want to ensure that exactly one of these products: A,B,or C must be processed.
b)We want to ensure that if product E is processed then product F must be processed.
Which products should be processed to maximize the total profit contribution?

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
37
A goal programming problem assumes that its objective function and constraints are linear.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
38
When an objective function contains squared terms,and the problem's constraints are linear,it is referred to as a quadratic programming problem.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
39
Mr.Smith,an avid reader,must decide on which books to take with him during a long flight.Mr.Smith will use his carry-on bag,which can hold a maximum of 20 pounds.Mr.Smith has attached a satisfaction index for each book,based on a 5-point scale (1 = low satisfaction,5 =high satisfaction),as shown below.
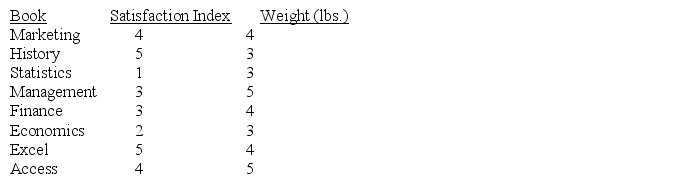
Which books should Mr.Smith take with him on the flight to maximize his satisfaction index?

Which books should Mr.Smith take with him on the flight to maximize his satisfaction index?

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
40
Deviational variables assume the value zero if a goal is completely attained.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
41
Set up and solve the following nonlinear programming problem using Excel:



Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
42
Consider the following linear programming problem:

Reformulate and solve this problem as a goal programming problem if the following prioritized goals must be met.


Reformulate and solve this problem as a goal programming problem if the following prioritized goals must be met.


Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
43
An investor is considering 7 different stocks: A,B,C,D,E,F,and G.The expected annual return for each stock is provided as follows:

The investor has imposed the following restrictions regarding the composition of the portfolio:
•the portfolio must include exactly one of the following stocks: either A or B.
•if stock B is selected,then stock F must also be selected.
•if stock D is selected,then stock E must be excluded.
Which stocks should be included in the portfolio to maximize annual return?

The investor has imposed the following restrictions regarding the composition of the portfolio:
•the portfolio must include exactly one of the following stocks: either A or B.
•if stock B is selected,then stock F must also be selected.
•if stock D is selected,then stock E must be excluded.
Which stocks should be included in the portfolio to maximize annual return?

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
44
A company currently has two factories: F1 and F2,and three retail outlets: R1,R2,and R3.The shipping costs per unit along with the monthly capacity and demand requirements are summarized below:
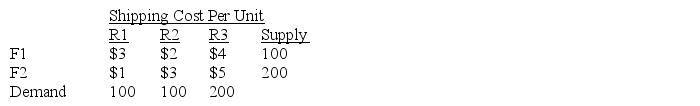
The firm has decided to build a new factory to expand its productive capacity.The two sites being considered are Philadelphia and Pittsburgh.The estimated shipping costs for the new factories along with their estimated fixed cost and production capacity are summarized below:
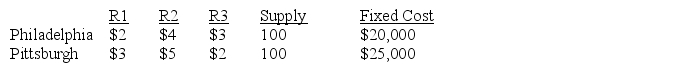
Which of the new locations will yield the lowest cost in combination with the existing factories and retail outlets?
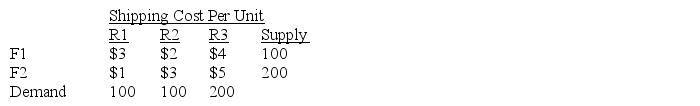
The firm has decided to build a new factory to expand its productive capacity.The two sites being considered are Philadelphia and Pittsburgh.The estimated shipping costs for the new factories along with their estimated fixed cost and production capacity are summarized below:
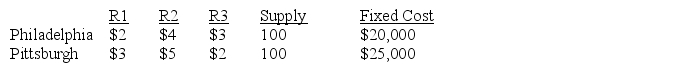
Which of the new locations will yield the lowest cost in combination with the existing factories and retail outlets?

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
45
A marketing manager is considering the following advertising media to promote a new
product.

The marketing manager has established the following goals and weights:
Goal 1: Reach at least 500,000 individuals;Weight 40.
Goal 2: Limit the total spending to $150,000;Weight 60.
Formulate and solve this goal programming problem.
product.

The marketing manager has established the following goals and weights:
Goal 1: Reach at least 500,000 individuals;Weight 40.
Goal 2: Limit the total spending to $150,000;Weight 60.
Formulate and solve this goal programming problem.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
46
A city is reviewing the location of its fire stations.The city is made up of a number of districts,as illustrated below.A fire station can be placed in any district and is able to handle the fires for both its neighborhood and any adjacent neighborhood.The objective is to minimize the number of fire stations used.
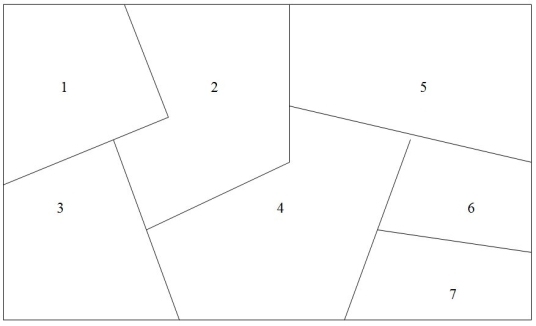


Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
47
Consider the following linear programming problem:

Reformulate and solve this problem as a goal programming problem if the following goals,with their associated weights,must be met.
Goal 1: Produce at least 15 units of product A;Weight 15
Goal 2: Produce at least 15 units of product B;Weight 15
Goal 3: Minimize overtime in assembly department;Weight 25
Goal 4: Minimize overtime in machine department;Weight 25
Goal 5: Achieve at least $100,000 in profit;Weight 20

Reformulate and solve this problem as a goal programming problem if the following goals,with their associated weights,must be met.
Goal 1: Produce at least 15 units of product A;Weight 15
Goal 2: Produce at least 15 units of product B;Weight 15
Goal 3: Minimize overtime in assembly department;Weight 25
Goal 4: Minimize overtime in machine department;Weight 25
Goal 5: Achieve at least $100,000 in profit;Weight 20

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
48
Consider the following linear programming problem:

Reformulate and solve this problem as a goal programming problem if the following goals,stated in no particular order,must be met.
Goal 1: Produce at least 15 units of each product.
Goal 2: Avoid overtime in the assembly and machine departments.
Goal 3: Achieve at least $100,000 in profit.

Reformulate and solve this problem as a goal programming problem if the following goals,stated in no particular order,must be met.
Goal 1: Produce at least 15 units of each product.
Goal 2: Avoid overtime in the assembly and machine departments.
Goal 3: Achieve at least $100,000 in profit.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
49
Consider the following profit expected from each dollar (Xᵢ)spent in the following advertising media:

How should the advertising budget be allocated if the company wants to spend at least $1000 on each medium without exceeding its $10,000 budget?

How should the advertising budget be allocated if the company wants to spend at least $1000 on each medium without exceeding its $10,000 budget?

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
50
A developer wishes to expand an office complex and needs to determine how many small,medium,and large offices to include in the expansion.Each small office requires 400 square feet,each medium office requires 700 square feet,and each large office requires 1200 square feet.The current square footage available for expansion is 35,000 square feet.The developer expects to pay $10,000 for each small office,$20,000 for each medium office,and $35,000 for each large office.The developer wishes to limit his expenditures to $500,000.How many of each type of office should be built if the following goals,stated in no particular order,must be met?
Goal 1: The expansion should include approximately 6 small offices.
Goal 2: The expansion should include approximately 11 medium offices.
Goal 3: The expansion should include approximately 15 large offices.
Goal 4: The expansion should consist of approximately 35,000 square feet.
Goal 5: The expansion should cost approximately $500,000.
Goal 1: The expansion should include approximately 6 small offices.
Goal 2: The expansion should include approximately 11 medium offices.
Goal 3: The expansion should include approximately 15 large offices.
Goal 4: The expansion should consist of approximately 35,000 square feet.
Goal 5: The expansion should cost approximately $500,000.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
51
Set up and solve the following nonlinear programming problem using Excel:



Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
52
Set up and solve the following nonlinear programming problem using Excel:



Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
53
A company produces four products each of which must undergo assembly,inspection,and packaging.The information below summarizes the hours required for each operation by each product,the total hourly capacity for each operation,and the fixed cost incurred by each product if it is produced.

Each unit of product 1 sold will contribute $60 to profit,and each unit of products 2,3,and 4 contributes $65,$70,and $55,respectively.What is the optimal product mix?

Each unit of product 1 sold will contribute $60 to profit,and each unit of products 2,3,and 4 contributes $65,$70,and $55,respectively.What is the optimal product mix?

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
54
A marketing manager is considering the following advertising media to promote a new
product.

The marketing manager has established the following goals,stated in no particular order,for the advertising campaign:
Goal 1: Reach at least 500,000 individuals.
Goal 2: Limit the total spending to $150,000.
Formulate and solve this goal programming problem.
product.

The marketing manager has established the following goals,stated in no particular order,for the advertising campaign:
Goal 1: Reach at least 500,000 individuals.
Goal 2: Limit the total spending to $150,000.
Formulate and solve this goal programming problem.

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck
55
A company is considering producing 8 different products for the upcoming holiday season.The profit contribution per product is illustrated below:

The marketing manager has imposed the following restrictions on the production mix:
•Since products 1,2,and 3 are electronic gadgets,at least two of these products must be in the production mix.
•Since products 4 and 5 are kids-oriented,the production mix must include no more than one of these products.
•If product 7 is included in the mix,then product 8 must be included,and vice versa.
Which products should be included in the mix to maximize profit?

The marketing manager has imposed the following restrictions on the production mix:
•Since products 1,2,and 3 are electronic gadgets,at least two of these products must be in the production mix.
•Since products 4 and 5 are kids-oriented,the production mix must include no more than one of these products.
•If product 7 is included in the mix,then product 8 must be included,and vice versa.
Which products should be included in the mix to maximize profit?

Unlock Deck
Unlock for access to all 55 flashcards in this deck.
Unlock Deck
k this deck



