Deck 13: Testing Hypotheses About Means
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/166
Play
Full screen (f)
Deck 13: Testing Hypotheses About Means
1
Which statement is not true about hypothesis tests?
A) Hypothesis tests are only valid when the sample is representative of the population for the question of interest.
B) Hypotheses are statements about the population represented by the samples.
C) Hypotheses are statements about the sample (or samples) from the population.
D) Conclusions are statements about the population represented by the samples.
A) Hypothesis tests are only valid when the sample is representative of the population for the question of interest.
B) Hypotheses are statements about the population represented by the samples.
C) Hypotheses are statements about the sample (or samples) from the population.
D) Conclusions are statements about the population represented by the samples.
Hypotheses are statements about the sample (or samples) from the population.
2
The primary purpose of a significance test is to
A) estimate the p-value of a sample.
B) estimate the p-value of a population.
C) decide whether there is enough evidence to support a research hypothesis about a sample.
D) decide whether there is enough evidence to support a research hypothesis about a population.
A) estimate the p-value of a sample.
B) estimate the p-value of a population.
C) decide whether there is enough evidence to support a research hypothesis about a sample.
D) decide whether there is enough evidence to support a research hypothesis about a population.
decide whether there is enough evidence to support a research hypothesis about a population.
3
The level of significance associated with a significance test is the probability
A) of rejecting a true null hypothesis.
B) of not rejecting a true null hypothesis.
C) that the null hypothesis is true.
D) that the alternative hypothesis is true.
A) of rejecting a true null hypothesis.
B) of not rejecting a true null hypothesis.
C) that the null hypothesis is true.
D) that the alternative hypothesis is true.
of rejecting a true null hypothesis.
4
A result is called statistically significant whenever
A) the null hypothesis is true.
B) the alternative hypothesis is true.
C) the p-value is less or equal to the significance level.
D) the p-value is larger than the significance level.
A) the null hypothesis is true.
B) the alternative hypothesis is true.
C) the p-value is less or equal to the significance level.
D) the p-value is larger than the significance level.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
5
Which of the following is not one of the steps for hypothesis testing?
A) Determine the null and alternative hypotheses.
B) Verify data conditions and calculate a test statistic.
C) Assuming the null hypothesis is true, find the p-value.
D) Assuming the alternative hypothesis is true, find the p-value.
A) Determine the null and alternative hypotheses.
B) Verify data conditions and calculate a test statistic.
C) Assuming the null hypothesis is true, find the p-value.
D) Assuming the alternative hypothesis is true, find the p-value.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
6
Which of the following is not a correct way to state a null hypothesis?
A) H0:
B) H0: d = 10
C) H0: = 0
D) H0: = 0.5
A) H0:
B) H0: d = 10
C) H0: = 0
D) H0: = 0.5

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
7
In hypothesis testing for one mean, the "null value"is not used in which of the following?
A) The null hypothesis.
B) The alternative hypothesis.
C) The computation of the test statistic.
D) The (null) standard error.
A) The null hypothesis.
B) The alternative hypothesis.
C) The computation of the test statistic.
D) The (null) standard error.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
8
A null hypothesis is that the average pulse rate of adults is 70. For a sample of 64 adults, the average pulse rate is 71.8. A significance test is done and the p-value is 0.02. What is the most appropriate conclusion?
A) Conclude that the population average is 70.
B) Conclude that the population average is 71.8.
C) Reject the hypothesis that the population average is 70.
D) Reject the hypothesis that the sample average is 70.
A) Conclude that the population average is 70.
B) Conclude that the population average is 71.8.
C) Reject the hypothesis that the population average is 70.
D) Reject the hypothesis that the sample average is 70.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
9
The p-value for a one-sided test for a mean was 0.04. The p-value for the corresponding two-sided test would be:
A) 0.02
B) 0.04
C) 0.06
D) 0.08
A) 0.02
B) 0.04
C) 0.06
D) 0.08

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
10
A null hypothesis is that the mean cholesterol level is 200 in a certain age group. The alternative is that the mean is not 200. Which of the following is the most significant evidence against the null and in favor of the alternative?
A) For a sample of n = 25, the sample mean is 220.
B) For a sample of n = 10, the sample mean is 220.
C) For a sample of n = 50, the sample mean is 180.
D) For a sample of n = 20, the sample mean is 180.
A) For a sample of n = 25, the sample mean is 220.
B) For a sample of n = 10, the sample mean is 220.
C) For a sample of n = 50, the sample mean is 180.
D) For a sample of n = 20, the sample mean is 180.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
11
A test of H0: = 0 versus Ha: > 0 is conducted on the same population independently by two different researchers. They both use the same sample size and the same value of = 0.05. Which of the following will be the same for both researchers?
A) The p-value of the test.
B) The power of the test if the true = 6.
C) The value of the test statistic.
D) The decision about whether or not to reject the null hypothesis.
A) The p-value of the test.
B) The power of the test if the true = 6.
C) The value of the test statistic.
D) The decision about whether or not to reject the null hypothesis.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
12
Which of the following is not true about hypothesis testing?
A) The null hypothesis defines a specific value of a population parameter, called the null value.
B) A relevant statistic is calculated from population information and summarized into a "test statistic."
C) A p-value is computed on the basis of the standardized "test statistic."
D) On the basis of the p-value, we either reject or fail to reject the null hypothesis.
A) The null hypothesis defines a specific value of a population parameter, called the null value.
B) A relevant statistic is calculated from population information and summarized into a "test statistic."
C) A p-value is computed on the basis of the standardized "test statistic."
D) On the basis of the p-value, we either reject or fail to reject the null hypothesis.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
13
Use the following information for questions:
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-What are the appropriate null and alternative hypotheses?
A) H0: =185 and Ha: < 185
B) H0: = 185 and Ha: >185.
C) H0: = 185 and Ha: 185.
D) H0: 185 and Ha: = 185.
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-What are the appropriate null and alternative hypotheses?
A) H0: =185 and Ha: < 185
B) H0: = 185 and Ha: >185.
C) H0: = 185 and Ha: 185.
D) H0: 185 and Ha: = 185.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
14
Use the following information for questions:
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-What is the value of the test statistic?
A) t = 1.50
B) t = 1.65
C) t = 1.80
D) None of the above
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-What is the value of the test statistic?
A) t = 1.50
B) t = 1.65
C) t = 1.80
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
15
Use the following information for questions:
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-What is the p-value?
A) p-value = 0.039
B) p-value = 0.053
C) p-value = 0.070
D) None of the above
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-What is the p-value?
A) p-value = 0.039
B) p-value = 0.053
C) p-value = 0.070
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
16
Use the following information for questions:
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-For a significance level of = 0.05, are the results statistically significant?
A) No, the results are not statistically significant because the p-value < 0.05.
B) Yes, the results are statistically significant because the p-value < 0.05.
C) No, the results are not statistically significant because the p-value > 0.05
D) Yes, the results are statistically significant because the p-value > 0.05.
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-For a significance level of = 0.05, are the results statistically significant?
A) No, the results are not statistically significant because the p-value < 0.05.
B) Yes, the results are statistically significant because the p-value < 0.05.
C) No, the results are not statistically significant because the p-value > 0.05
D) Yes, the results are statistically significant because the p-value > 0.05.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
17
Use the following information for questions:
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-Which of the following is an appropriate conclusion?
A) The results are statistically significant so the average total weight of all passengers appears to be greater than 185 pounds.
B) The results are statistically significant so the average total weight of all passengers appears to be less than 185 pounds.
C) The results are not statistically significant so there is not enough evidence to conclude the average total weight of all passengers is greater than 185 pounds.
D) None of the above.
An investigator wants to assess whether the mean = the average weight of passengers flying on small planes exceeds the FAA guideline of average total weight of 185 pounds (passenger weight including shoes, clothes, and carry-on). Suppose that a random sample of 51 passengers showed an average total weight of 200 pounds with a sample standard deviation of 59.5 pounds. Assume that passenger total weights are normally distributed.
-Which of the following is an appropriate conclusion?
A) The results are statistically significant so the average total weight of all passengers appears to be greater than 185 pounds.
B) The results are statistically significant so the average total weight of all passengers appears to be less than 185 pounds.
C) The results are not statistically significant so there is not enough evidence to conclude the average total weight of all passengers is greater than 185 pounds.
D) None of the above.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
18
A random sample of 25 third graders scored an average of 3.2 on a standardized reading test. The standard deviation was 0.95. What is the value of the t-test statistic for determining if the mean score is significantly higher than 3?
A) t = 0.21
B) t = 5.26
C) t = 1.05
D) None of the above
A) t = 0.21
B) t = 5.26
C) t = 1.05
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
19
The WISC scores of a sample of n = 20 5th graders resulted in a mean of 104 with a standard deviation of 9. Is the mean score significantly higher than 100 when = 0.05?
A) No, because t = 1.99 which is greater than the critical value t* = 1.73.
B) Yes, because t = 1.99 which is greater than the critical value t* = 1.73.
C) No, because t = 1.99 which is smaller than the critical value t* = 2.09.
D) Yes, because t = 1.99 which is smaller than the critical value t* = 2.09.
A) No, because t = 1.99 which is greater than the critical value t* = 1.73.
B) Yes, because t = 1.99 which is greater than the critical value t* = 1.73.
C) No, because t = 1.99 which is smaller than the critical value t* = 2.09.
D) Yes, because t = 1.99 which is smaller than the critical value t* = 2.09.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
20
A random sample of 25 third graders scored an average of 3.3 on a standardized spelling test. The standard deviation was 1.06. Is the mean score significantly higher than 3?
A) It is both when = 0.05 and when = 0.10.
B) It is when = 0.05 but it is not when = 0.10.
C) It is not when = 0.05 but it is when = 0.10.
D) It is not when = 0.05 and it is not either when = 0.10.
A) It is both when = 0.05 and when = 0.10.
B) It is when = 0.05 but it is not when = 0.10.
C) It is not when = 0.05 but it is when = 0.10.
D) It is not when = 0.05 and it is not either when = 0.10.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
21
A random sample of 12 bags of barbecue potato chips is collected and the price of each determined. The mean was $1.03 with a standard deviation of 7 cent ($0.07). Using = 0.10, we wish to test H0: = $1.00. The result is significant
A) for both Ha: > $1.00 and for Ha: $1.00.
B) for Ha: > $1.00 but not for Ha: $1.00.
C) for Ha: $1.00 but not for Ha: > $1.00.
D) neither for Ha: > $1.00 nor for Ha: $1.00.
A) for both Ha: > $1.00 and for Ha: $1.00.
B) for Ha: > $1.00 but not for Ha: $1.00.
C) for Ha: $1.00 but not for Ha: > $1.00.
D) neither for Ha: > $1.00 nor for Ha: $1.00.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
22
Spatial perception is measured on a scale from 0 to 10. A group of 9th grade students are tested for spatial perception. SPSS was used to obtain descriptive statistics of the spatial perception scores in the sample. 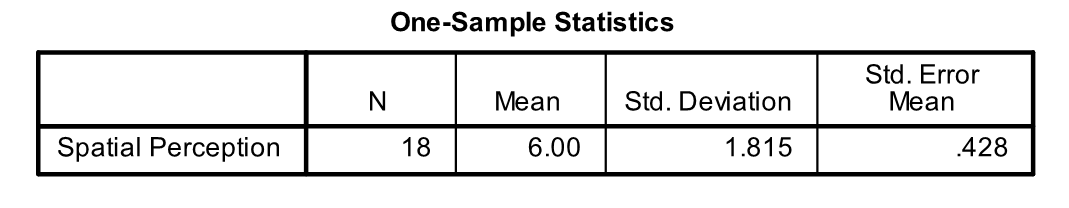
Using = 0.01, is the mean score significantly different from 5?
A) Yes, because t = 2.34 and this is greater than t* = 2.11.
B) No, because t = 2.34 and this is smaller than t* = 3.97.
C) No, because t = 0.55 and this is smaller than t* = 2.11.
D) No, because t = 2.34 and this is smaller than t* = 2.90.

Using = 0.01, is the mean score significantly different from 5?
A) Yes, because t = 2.34 and this is greater than t* = 2.11.
B) No, because t = 2.34 and this is smaller than t* = 3.97.
C) No, because t = 0.55 and this is smaller than t* = 2.11.
D) No, because t = 2.34 and this is smaller than t* = 2.90.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
23
Use the following information for questions:
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-What are the appropriate null and alternative hypotheses?
A) H0: = 25 and Ha: < 25
B) H0: = 25 and Ha: >25.
C) H0: = 25 and Ha: 25.
D) H0: 25 and Ha: = 25.
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-What are the appropriate null and alternative hypotheses?
A) H0: = 25 and Ha: < 25
B) H0: = 25 and Ha: >25.
C) H0: = 25 and Ha: 25.
D) H0: 25 and Ha: = 25.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
24
Use the following information for questions:
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-What is the value of the test statistic?
A) t = -01.80
B) t = -1.70
C) t = -1.50
D) None of the above
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-What is the value of the test statistic?
A) t = -01.80
B) t = -1.70
C) t = -1.50
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
25
Use the following information for questions:
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-What is the p-value?
A) p-value = 0.079
B) p-value = 0.057
C) p-value = 0.048
D) None of the above
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-What is the p-value?
A) p-value = 0.079
B) p-value = 0.057
C) p-value = 0.048
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
26
Use the following information for questions:
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-For a significance level of = 0.05, are the results statistically significant?
A) No, the results are not statistically significant because the p-value < 0.05.
B) Yes, the results are statistically significant because the p-value < 0.05.
C) No, the results are not statistically significant because the p-value > 0.05
D) Yes, the results are statistically significant because the p-value > 0.05.
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-For a significance level of = 0.05, are the results statistically significant?
A) No, the results are not statistically significant because the p-value < 0.05.
B) Yes, the results are statistically significant because the p-value < 0.05.
C) No, the results are not statistically significant because the p-value > 0.05
D) Yes, the results are statistically significant because the p-value > 0.05.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
27
Use the following information for questions:
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-Which of the following is an appropriate conclusion?
A) The results are statistically significant so the average speed appears to be greater than 25 mph.
B) The results are statistically significant so the average speed appears to be less than 25 mph.
C) The results are not statistically significant: there is not enough evidence to conclude the average speed is less than 25 mph.
D) None of the above.
A safety officer wants to prove that = the average speed of cars driven by a school is less than 25 mph. Suppose that a random sample of 14 cars shows an average speed of 24.0 mph, with a sample standard deviation of 2.2 mph. Assume that the speeds of cars are normally distributed.
-Which of the following is an appropriate conclusion?
A) The results are statistically significant so the average speed appears to be greater than 25 mph.
B) The results are statistically significant so the average speed appears to be less than 25 mph.
C) The results are not statistically significant: there is not enough evidence to conclude the average speed is less than 25 mph.
D) None of the above.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
28
Use the following information for questions:
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-What are the appropriate null and alternative hypotheses?
A) H0: = 21 and Ha: < 21
B) H0: = 21 and Ha: >21.
C) H0: = 21 and Ha: 21.
D) H0: 21 and Ha: = 21.
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-What are the appropriate null and alternative hypotheses?
A) H0: = 21 and Ha: < 21
B) H0: = 21 and Ha: >21.
C) H0: = 21 and Ha: 21.
D) H0: 21 and Ha: = 21.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
29
Use the following information for questions:
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-What is the value of the test statistic?
A) t =1.80
B) t =2.00
C) t =2.33
D) None of the above
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-What is the value of the test statistic?
A) t =1.80
B) t =2.00
C) t =2.33
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
30
Use the following information for questions:
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-What is the p-value?
A) p-value = 0.022
B) p-value = 0.053
C) p-value = 0.076
D) None of the above
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-What is the p-value?
A) p-value = 0.022
B) p-value = 0.053
C) p-value = 0.076
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
31
Use the following information for questions:
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-For a significance level of = 0.05, are the results statistically significant?
A) No, the results are not statistically significant because the p-value < 0.05.
B) Yes, the results are statistically significant because the p-value < 0.05.
C) No, the results are not statistically significant because the p-value > 0.05
D) Yes, the results are statistically significant because the p-value > 0.05.
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-For a significance level of = 0.05, are the results statistically significant?
A) No, the results are not statistically significant because the p-value < 0.05.
B) Yes, the results are statistically significant because the p-value < 0.05.
C) No, the results are not statistically significant because the p-value > 0.05
D) Yes, the results are statistically significant because the p-value > 0.05.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
32
Use the following information for questions:
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-Which of the following is an appropriate conclusion?
A) The results are statistically significant so the average age appears to be greater than 21.
B) The results are statistically significant so the average age appears to be less than 21.
C) The results are not statistically significant so there is not enough evidence to conclude average age is different from 21.
D) None of the above.
A counselor wants to show that for men who are married by the time they are 30, = average age when the men are married is not 21 years old. A random sample of 10 men who were married by age 30 showed an average age at marriage of 22.2, with a sample standard deviation of 1.9 years. Assume that the age at which this population of men get married for the first time is normally distributed.
-Which of the following is an appropriate conclusion?
A) The results are statistically significant so the average age appears to be greater than 21.
B) The results are statistically significant so the average age appears to be less than 21.
C) The results are not statistically significant so there is not enough evidence to conclude average age is different from 21.
D) None of the above.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
33
Explain the difference between the null and alternative hypotheses in hypothesis testing. Give an example.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
34
Use the following information for questions:
A sample of n = 9 men are asked. "What's the fastest you've ever driven a car?"The sample mean is 120 mph and the standard deviation is 30.
-What is the value of the t-test statistic for testing the null hypothesis that the population mean response is 100 mph?
A sample of n = 9 men are asked. "What's the fastest you've ever driven a car?"The sample mean is 120 mph and the standard deviation is 30.
-What is the value of the t-test statistic for testing the null hypothesis that the population mean response is 100 mph?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
35
Use the following information for questions:
A sample of n = 9 men are asked. "What's the fastest you've ever driven a car?"The sample mean is 120 mph and the standard deviation is 30.
-What are the degrees of freedom for conducting this t-test?
A sample of n = 9 men are asked. "What's the fastest you've ever driven a car?"The sample mean is 120 mph and the standard deviation is 30.
-What are the degrees of freedom for conducting this t-test?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
36
Use the following information for questions:
A sample of n = 9 men are asked. "What's the fastest you've ever driven a car?"The sample mean is 120 mph and the standard deviation is 30.
-Find the p-value for testing whether the population mean response is more than 100 mph?
A sample of n = 9 men are asked. "What's the fastest you've ever driven a car?"The sample mean is 120 mph and the standard deviation is 30.
-Find the p-value for testing whether the population mean response is more than 100 mph?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
37
Use the following information for questions:
A sample of n = 9 men are asked. "What's the fastest you've ever driven a car?"The sample mean is 120 mph and the standard deviation is 30.
-Give an appropriate conclusion using a 1% level of significance.
A sample of n = 9 men are asked. "What's the fastest you've ever driven a car?"The sample mean is 120 mph and the standard deviation is 30.
-Give an appropriate conclusion using a 1% level of significance.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
38
Use the following information for questions:
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the beginning of the course the women are given a questionnaire to determine how afraid they are. Fear scores range between 100 and 400. SPSS is used to obtain descriptive statistics. Output is shown below.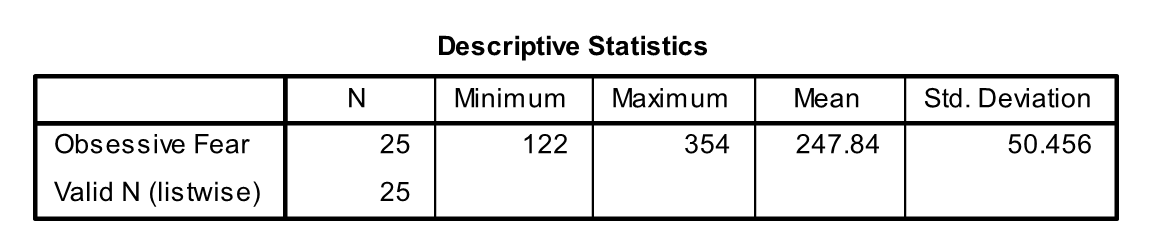 The course coordinator wishes to test if the women score significantly lower than 250.
The course coordinator wishes to test if the women score significantly lower than 250.
-What is the value of the t-test statistic?
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the beginning of the course the women are given a questionnaire to determine how afraid they are. Fear scores range between 100 and 400. SPSS is used to obtain descriptive statistics. Output is shown below.
 The course coordinator wishes to test if the women score significantly lower than 250.
The course coordinator wishes to test if the women score significantly lower than 250.-What is the value of the t-test statistic?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
39
Use the following information for questions:
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the beginning of the course the women are given a questionnaire to determine how afraid they are. Fear scores range between 100 and 400. SPSS is used to obtain descriptive statistics. Output is shown below. The course coordinator wishes to test if the women score significantly lower than 250.
The course coordinator wishes to test if the women score significantly lower than 250.
-What do we know about the p-value for this test?
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the beginning of the course the women are given a questionnaire to determine how afraid they are. Fear scores range between 100 and 400. SPSS is used to obtain descriptive statistics. Output is shown below.
 The course coordinator wishes to test if the women score significantly lower than 250.
The course coordinator wishes to test if the women score significantly lower than 250.-What do we know about the p-value for this test?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
40
Use the following information for questions:
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the beginning of the course the women are given a questionnaire to determine how afraid they are. Fear scores range between 100 and 400. SPSS is used to obtain descriptive statistics. Output is shown below. The course coordinator wishes to test if the women score significantly lower than 250.
The course coordinator wishes to test if the women score significantly lower than 250.
-Give an appropriate conclusion using a 10% level of significance.
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the beginning of the course the women are given a questionnaire to determine how afraid they are. Fear scores range between 100 and 400. SPSS is used to obtain descriptive statistics. Output is shown below.
 The course coordinator wishes to test if the women score significantly lower than 250.
The course coordinator wishes to test if the women score significantly lower than 250.-Give an appropriate conclusion using a 10% level of significance.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
41
The amount of time the husband and the wife spend on house work is measured for 15 women and their 15 husbands. For the wives the mean was 7 hours/week and for the husbands the mean was 4.5 hours/week. The standard deviation of the differences in time spent on house work was 2.85. What is the value of the test statistic for testing the difference in mean time spent on housework between husbands and wives?
A) 0.88
B) 2.40
C) 3.40
D) 4.80
A) 0.88
B) 2.40
C) 3.40
D) 4.80

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
42
The head circumference is measured for 25 girls and their younger twin sisters. The mean of the older twin girls was 50.23 cm and the mean of the younger twins was 49.96 cm. The standard deviation of the differences was 1 cm. Is this difference significant at a significance level of 5%?
A) Yes, because t = 1.35 and the p-value < 0.10.
B) Yes, because t = 6.25 and the p-value < 0.10.
C) No, because t = 1.35 and the p-value > 0.10.
D) No, because t = 0.27 and the p-value > 0.10.
A) Yes, because t = 1.35 and the p-value < 0.10.
B) Yes, because t = 6.25 and the p-value < 0.10.
C) No, because t = 1.35 and the p-value > 0.10.
D) No, because t = 0.27 and the p-value > 0.10.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
43
An experiment is conducted with 15 seniors who are taking Spanish at Oak View High School. A randomly selected group of eight students is first tested with a written test and a day later with an oral exam. To avoid order effects, the other seven students are tested in reverse order. The instructor is interested in the difference in grades between the two testing methods. SPSS is used to obtain descriptive statistics for the grades of the two tests. 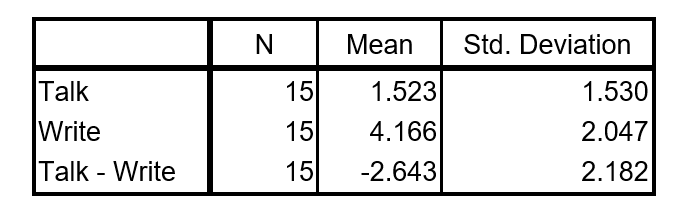
Is there a significant difference between the mean grades using the two different testing methods? Use = 0.05.
A) Yes, because t = -4.69 and the p-value < 0.05.
B) No, because t = -1.21 and the p-value > 0.05.
C) Yes, because t = -6.63 and the p-value < 0.05.
D) No, because t =-1.48 and the p-value > 0.05.

Is there a significant difference between the mean grades using the two different testing methods? Use = 0.05.
A) Yes, because t = -4.69 and the p-value < 0.05.
B) No, because t = -1.21 and the p-value > 0.05.
C) Yes, because t = -6.63 and the p-value < 0.05.
D) No, because t =-1.48 and the p-value > 0.05.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
44
Use the following information for questions:
It is known that for right-handed people, the dominant (right) hand tends to be stronger. For left-handed people who live in a world designed for right-handed people, the same may not be true. To test this, muscle strength was measured on the right and left hands of a random sample of 15 left-handed men and the difference (left - right) was found. The alternative hypothesis is one-sided (left hand stronger). The resultingt-statistic was 1.80.
-This is an example of
A) a two-sample t-test.
B) a paired t-test.
C) a pooled t-test.
D) an unpooled t-test.
It is known that for right-handed people, the dominant (right) hand tends to be stronger. For left-handed people who live in a world designed for right-handed people, the same may not be true. To test this, muscle strength was measured on the right and left hands of a random sample of 15 left-handed men and the difference (left - right) was found. The alternative hypothesis is one-sided (left hand stronger). The resultingt-statistic was 1.80.
-This is an example of
A) a two-sample t-test.
B) a paired t-test.
C) a pooled t-test.
D) an unpooled t-test.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
45
Use the following information for questions:
It is known that for right-handed people, the dominant (right) hand tends to be stronger. For left-handed people who live in a world designed for right-handed people, the same may not be true. To test this, muscle strength was measured on the right and left hands of a random sample of 15 left-handed men and the difference (left - right) was found. The alternative hypothesis is one-sided (left hand stronger). The resultingt-statistic was 1.80.
-Which of the following is true about the conditions necessary to carry out the t-test in this situation?
A) Because the sample size is small (15), the population of differences must be assumed to be approximately normal, but no assumption about the variances is required.
B) Because the sample size is not small (15 + 15 = 30), the population of differences need not be assumed to be approximately normal, and no assumption about the variances is required.
C) Because the sample size is small (15), the population of differences must be assumed to be approximately normal, and the variances of the right and left hand strengths must be assumed to be equal.
D) Because the sample size is not small (15 + 15 = 30), the population of differences need not be assumed to be approximately normal, but the variances of the right and left hand strengths must be assumed to be equal.
It is known that for right-handed people, the dominant (right) hand tends to be stronger. For left-handed people who live in a world designed for right-handed people, the same may not be true. To test this, muscle strength was measured on the right and left hands of a random sample of 15 left-handed men and the difference (left - right) was found. The alternative hypothesis is one-sided (left hand stronger). The resultingt-statistic was 1.80.
-Which of the following is true about the conditions necessary to carry out the t-test in this situation?
A) Because the sample size is small (15), the population of differences must be assumed to be approximately normal, but no assumption about the variances is required.
B) Because the sample size is not small (15 + 15 = 30), the population of differences need not be assumed to be approximately normal, and no assumption about the variances is required.
C) Because the sample size is small (15), the population of differences must be assumed to be approximately normal, and the variances of the right and left hand strengths must be assumed to be equal.
D) Because the sample size is not small (15 + 15 = 30), the population of differences need not be assumed to be approximately normal, but the variances of the right and left hand strengths must be assumed to be equal.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
46
Use the following information for questions:
It is known that for right-handed people, the dominant (right) hand tends to be stronger. For left-handed people who live in a world designed for right-handed people, the same may not be true. To test this, muscle strength was measured on the right and left hands of a random sample of 15 left-handed men and the difference (left - right) was found. The alternative hypothesis is one-sided (left hand stronger). The resultingt-statistic was 1.80.
-Assuming the conditions are met, based on the t-statistic of 1.80 the appropriate decision for this test using = 0.05 is:
A) df = 14, so p-value < 0.05 and the null hypothesis can be rejected.
B) df = 14, so p-value > 0.05 and the null hypothesis cannot be rejected.
C) df = 28, so p-value < 0.05 and the null hypothesis can be rejected.
D) df = 28, so p-value > 0.05 and the null hypothesis cannot be rejected.
It is known that for right-handed people, the dominant (right) hand tends to be stronger. For left-handed people who live in a world designed for right-handed people, the same may not be true. To test this, muscle strength was measured on the right and left hands of a random sample of 15 left-handed men and the difference (left - right) was found. The alternative hypothesis is one-sided (left hand stronger). The resultingt-statistic was 1.80.
-Assuming the conditions are met, based on the t-statistic of 1.80 the appropriate decision for this test using = 0.05 is:
A) df = 14, so p-value < 0.05 and the null hypothesis can be rejected.
B) df = 14, so p-value > 0.05 and the null hypothesis cannot be rejected.
C) df = 28, so p-value < 0.05 and the null hypothesis can be rejected.
D) df = 28, so p-value > 0.05 and the null hypothesis cannot be rejected.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
47
Use the following information for questions:
It is known that for right-handed people, the dominant (right) hand tends to be stronger. For left-handed people who live in a world designed for right-handed people, the same may not be true. To test this, muscle strength was measured on the right and left hands of a random sample of 15 left-handed men and the difference (left - right) was found. The alternative hypothesis is one-sided (left hand stronger). The resultingt-statistic was 1.80.
-Which of the following is an appropriate conclusion?
A) The results are statistically significant so the left hand appears to be stronger.
B) The results are statistically significant so the left hand does not appear to be stronger.
C) The results are not statistically significant so there is not enough evidence to conclude the left hand appears to be stronger.
D) None of the above.
It is known that for right-handed people, the dominant (right) hand tends to be stronger. For left-handed people who live in a world designed for right-handed people, the same may not be true. To test this, muscle strength was measured on the right and left hands of a random sample of 15 left-handed men and the difference (left - right) was found. The alternative hypothesis is one-sided (left hand stronger). The resultingt-statistic was 1.80.
-Which of the following is an appropriate conclusion?
A) The results are statistically significant so the left hand appears to be stronger.
B) The results are statistically significant so the left hand does not appear to be stronger.
C) The results are not statistically significant so there is not enough evidence to conclude the left hand appears to be stronger.
D) None of the above.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
48
Use the following information for questions:
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the end of the course the women are given a questionnaire to determine how afraid they are. Six months after the course the women answer the questionnaire again as a follow-up measure. The fear-scores are typed into SPSS. Output is shown below.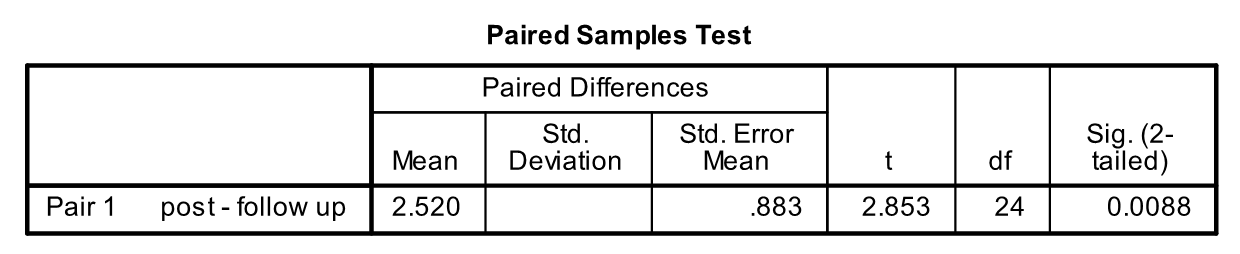
-How much lower, on average, did the women score on the fear questionnaire six months after the course than directly at the end of the course?
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the end of the course the women are given a questionnaire to determine how afraid they are. Six months after the course the women answer the questionnaire again as a follow-up measure. The fear-scores are typed into SPSS. Output is shown below.

-How much lower, on average, did the women score on the fear questionnaire six months after the course than directly at the end of the course?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
49
Use the following information for questions:
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the end of the course the women are given a questionnaire to determine how afraid they are. Six months after the course the women answer the questionnaire again as a follow-up measure. The fear-scores are typed into SPSS. Output is shown below.
-What is the value of the standard deviation of the differences that is missing from the output?
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the end of the course the women are given a questionnaire to determine how afraid they are. Six months after the course the women answer the questionnaire again as a follow-up measure. The fear-scores are typed into SPSS. Output is shown below.

-What is the value of the standard deviation of the differences that is missing from the output?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
50
Use the following information for questions:
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the end of the course the women are given a questionnaire to determine how afraid they are. Six months after the course the women answer the questionnaire again as a follow-up measure. The fear-scores are typed into SPSS. Output is shown below.
-What is the p-value for testing whether women score significantly lower on fear 6 months after the course than directly at the end of the course?
A group of women suffering from various types of obsessive fear is given a therapeutic course to learn how to handle their fear. At the end of the course the women are given a questionnaire to determine how afraid they are. Six months after the course the women answer the questionnaire again as a follow-up measure. The fear-scores are typed into SPSS. Output is shown below.

-What is the p-value for testing whether women score significantly lower on fear 6 months after the course than directly at the end of the course?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
51
Use the following information for questions:
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-What is the value of the t-test statistic?
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-What is the value of the t-test statistic?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
52
Use the following information for questions:
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-What are the degrees of freedom for conducting this t-test?
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-What are the degrees of freedom for conducting this t-test?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
53
Use the following information for questions:
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-Find the p-value for testing whether using the non-dominant hand takes longer on average than using the dominant hand?
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-Find the p-value for testing whether using the non-dominant hand takes longer on average than using the dominant hand?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
54
Use the following information for questions:
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-Given an appropriate conclusion using a 5% level of significance.
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-Given an appropriate conclusion using a 5% level of significance.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
55
Use the following information for questions:
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-What is the value of the t-test statistic?
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-What is the value of the t-test statistic?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
56
Use the following information for questions:
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-What are the degrees of freedom for conducting this t-test?
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-What are the degrees of freedom for conducting this t-test?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
57
Use the following information for questions:
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-Find the p-value for testing whether using the non-dominant hand takes longer on average than using the dominant hand?
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-Find the p-value for testing whether using the non-dominant hand takes longer on average than using the dominant hand?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
58
Use the following information for questions:
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-Given an appropriate conclusion using a 5% level of significance.
An instructor wanted to prove that writing with the non-dominant hand takes longer on average than writing with the dominant hand. A significance level of 5% will be used. Ten students randomly sampled from a large class each wrote the word "Statistics" twice; once with their dominant hand and once with their non-dominant hand, in random order. The average time to write using their dominant hands was 5 seconds, while the average time to write with their non-dominant hand was 10 seconds. The standard deviation of the difference was 7.9 seconds, and the standard error was 2.498. Assume that writing times with each hand are normally distributed.
-Given an appropriate conclusion using a 5% level of significance.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
59
Suppose that a difference between two groups is examined. In the language of statistics, the alternative hypothesis is a statement that there is __________
A) no difference between the sample means.
B) a difference between the sample means.
C) no difference between the population means.
D) a difference between the population means.
A) no difference between the sample means.
B) a difference between the sample means.
C) no difference between the population means.
D) a difference between the population means.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
60
The maximum distance at which a highway sign can be read is determined for a sample of young people and a sample of older people. The mean distance is computed for each age group. What's the most appropriate null hypothesis about the means of the two groups?
A) The population means are different.
B) The sample means are different.
C) The population means are the same.
D) The sample means are the same.
A) The population means are different.
B) The sample means are different.
C) The population means are the same.
D) The sample means are the same.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
61
Researchers want to see if men have a higher blood pressure than women do. A study is planned in which the blood pressures of 50 men and 50 women will be measured. What's the most appropriate alternative hypothesis about the means of the men and women?
A) The sample means are the same.
B) The sample mean will be higher for men.
C) The population means are the same.
D) The population mean is higher for men than for women.
A) The sample means are the same.
B) The sample mean will be higher for men.
C) The population means are the same.
D) The population mean is higher for men than for women.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
62
When comparing two means, the situation most likely to lead to a result that is statistically significant but of little practical importance is
A) when the actual difference is large and the sample sizes are large.
B) when the actual difference is large and the sample sizes are small.
C) when the actual difference is small and the sample sizes are large.
D) when the actual difference is small and the sample sizes are small.
A) when the actual difference is large and the sample sizes are large.
B) when the actual difference is large and the sample sizes are small.
C) when the actual difference is small and the sample sizes are large.
D) when the actual difference is small and the sample sizes are small.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
63
When comparing two means, which situation is most likely to lead to a result that is statistically significant? (Consider all other factors equal, such as significance level and standard deviations.)
A) = 10, = 20 and the sample sizes are = 25 and = 25
B) = 10, = 25 and the sample sizes are = 25 and = 25
C) = 10, = 20 and the sample sizes are = 15 and = 20
D) = 10, = 25 and the sample sizes are = 25 and = 35
A) = 10, = 20 and the sample sizes are = 25 and = 25
B) = 10, = 25 and the sample sizes are = 25 and = 25
C) = 10, = 20 and the sample sizes are = 15 and = 20
D) = 10, = 25 and the sample sizes are = 25 and = 35

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
64
Use the following information for questions:
A null hypothesis is that the mean nose lengths of men and women are the same. The alternative hypothesis is that men have a longer mean nose length than women.
-Which of the following is the correct way to state the null hypothesis?
A)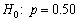
B)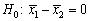
C)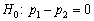
D)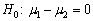
A null hypothesis is that the mean nose lengths of men and women are the same. The alternative hypothesis is that men have a longer mean nose length than women.
-Which of the following is the correct way to state the null hypothesis?
A)
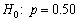
B)
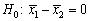
C)
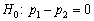
D)
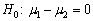

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
65
Use the following information for questions:
A null hypothesis is that the mean nose lengths of men and women are the same. The alternative hypothesis is that men have a longer mean nose length than women.
-A statistical test is performed for assessing if men have a longer mean nose length than women. The p-value is 0.225. Which of the following is the most appropriate way to state the conclusion?
A) The mean nose lengths of the populations of men and women are identical.
B) There is not enough evidence to say that that the populations of men and women have different mean nose lengths.
C) Men have a greater mean nose length than women.
D) The probability is 0.225 that men and women have the same mean nose length.
A null hypothesis is that the mean nose lengths of men and women are the same. The alternative hypothesis is that men have a longer mean nose length than women.
-A statistical test is performed for assessing if men have a longer mean nose length than women. The p-value is 0.225. Which of the following is the most appropriate way to state the conclusion?
A) The mean nose lengths of the populations of men and women are identical.
B) There is not enough evidence to say that that the populations of men and women have different mean nose lengths.
C) Men have a greater mean nose length than women.
D) The probability is 0.225 that men and women have the same mean nose length.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
66
Use the following information for questions:
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What are the appropriate null and alternative hypotheses?
A) H0: - = 0 and Ha: - 0
B) H0: - 0 and Ha: - = 0
C) H0: - = 0 and Ha: - < 0
D) H0: - = 0 and Ha: - > 0
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What are the appropriate null and alternative hypotheses?
A) H0: - = 0 and Ha: - 0
B) H0: - 0 and Ha: - = 0
C) H0: - = 0 and Ha: - < 0
D) H0: - = 0 and Ha: - > 0

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
67
Use the following information for questions:
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the value of the test statistic?
A) t =-1.80
B) t =-102.00
C) t =-2.33
D) None of the above
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the value of the test statistic?
A) t =-1.80
B) t =-102.00
C) t =-2.33
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
68
Use the following information for questions:
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the p-value?
A) p-value = 0.021
B) p-value = 0.022
C) p-value = 0.038
D) None of the above
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the p-value?
A) p-value = 0.021
B) p-value = 0.022
C) p-value = 0.038
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
69
Use the following information for questions:
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-For a significance level of = 0.05, are the results statistically significant?
A) No, results are not statistically significant because the p-value < 0.05.
B) Yes, results are statistically significant because the p-value < 0.05.
C) No, results are not statistically significant because the p-value > 0.05
D) Yes, results are statistically significant because the p-value > 0.05.
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-For a significance level of = 0.05, are the results statistically significant?
A) No, results are not statistically significant because the p-value < 0.05.
B) Yes, results are statistically significant because the p-value < 0.05.
C) No, results are not statistically significant because the p-value > 0.05
D) Yes, results are statistically significant because the p-value > 0.05.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
70
Use the following information for questions:
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-Which of the following is an appropriate conclusion?
A) The average delay for Airline 1 does appear to be less than the average delay for Airline 2.
B) The results are not statistically significant so there is not enough evidence to conclude the average delay for Airline 1 is less than the average delay for Airline 2.
C) The average delay for Airline 1 is at least 3 minutes less than Airline 2.
D) None of the above.
An airport official wants to assess if the flights from one airline (Airline 1) are less delayed than flights from another airline (Airline 2). Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 9.5 minutes delay with a standard deviation of 3 minutes. A random sample of 10 flights for Airline 2 shows an average of 12.63 minutes delay with a standard deviation of 3 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-Which of the following is an appropriate conclusion?
A) The average delay for Airline 1 does appear to be less than the average delay for Airline 2.
B) The results are not statistically significant so there is not enough evidence to conclude the average delay for Airline 1 is less than the average delay for Airline 2.
C) The average delay for Airline 1 is at least 3 minutes less than Airline 2.
D) None of the above.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
71
Use the following information for questions:
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What are the appropriate null and alternative hypotheses?
A) H0: - = 0 and Ha: - 0
B) H0: - 0 and Ha: - = 0
C) H0: - = 0 and Ha: - < 0
D) H0: - = 0 and Ha: - > 0
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What are the appropriate null and alternative hypotheses?
A) H0: - = 0 and Ha: - 0
B) H0: - 0 and Ha: - = 0
C) H0: - = 0 and Ha: - < 0
D) H0: - = 0 and Ha: - > 0

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
72
Use the following information for questions:
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the value of the test statistic?
A) t = -2.68
B) t = -1.50
C) t = 1.50
D) t = 2.68
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the value of the test statistic?
A) t = -2.68
B) t = -1.50
C) t = 1.50
D) t = 2.68

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
73
Use the following information for questions:
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-For a significance level of 0.05, what is the critical value?
A) The critical value = 1.83.
B) The critical value = 2.10.
C) The critical value = 2.26.
D) None of the above.
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-For a significance level of 0.05, what is the critical value?
A) The critical value = 1.83.
B) The critical value = 2.10.
C) The critical value = 2.26.
D) None of the above.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
74
Use the following information for questions:
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-Are the results statistically significant?
A) No, results are not statistically significant because | t | < 1.83.
B) Yes, results are statistically significant because | t | > 2.26.
C) No, results are not statistically significant because | t | > 2.26.
D) None of the above.
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-Are the results statistically significant?
A) No, results are not statistically significant because | t | < 1.83.
B) Yes, results are statistically significant because | t | > 2.26.
C) No, results are not statistically significant because | t | > 2.26.
D) None of the above.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
75
Use the following information for questions:
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-Which of the following is an appropriate conclusion?
A) The average delay for Airline 1 does appear to be different than the average delay for Airline 2.
B) The results are not statistically significant so there is not enough evidence to conclude that the average delays are different.
C) The average delay for Airline 1 is at least 3 minutes less than for Airline 2.
D) None of the above.
A bank official wants to assess if the average delays for two airlines are different.Let = average delay for Airline 1 and = average delay for Airline 2. A random sample of 10 flights for Airline 1 shows an average of 6 minutes delay with a standard deviation of 5 minutes. A random sample of 10 flights for Airline 2 shows an average of 12 minutes delay with a standard deviation of 5 minutes. Assume delay times are normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-Which of the following is an appropriate conclusion?
A) The average delay for Airline 1 does appear to be different than the average delay for Airline 2.
B) The results are not statistically significant so there is not enough evidence to conclude that the average delays are different.
C) The average delay for Airline 1 is at least 3 minutes less than for Airline 2.
D) None of the above.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
76
Use the following information for questions:
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What are the appropriate null and alternative hypotheses?
A) H0: - = 0 and Ha: - 0
B) H0: - 0 and Ha: - = 0
C) H0: - = 0 and Ha: - < 0
D) H0: - = 0 and Ha: - > 0
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What are the appropriate null and alternative hypotheses?
A) H0: - = 0 and Ha: - 0
B) H0: - 0 and Ha: - = 0
C) H0: - = 0 and Ha: - < 0
D) H0: - = 0 and Ha: - > 0

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
77
Use the following information for questions:
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the value of the test statistic?
A) t = 1.80
B) t = 2.00
C) t = 2.33
D) t = 2.58
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the value of the test statistic?
A) t = 1.80
B) t = 2.00
C) t = 2.33
D) t = 2.58

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
78
Use the following information for questions:
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the p-value?
A) p-value = 0.015
B) p-value = 0.022
C) p-value = 0.038
D) None of the above
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-What is the p-value?
A) p-value = 0.015
B) p-value = 0.022
C) p-value = 0.038
D) None of the above

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
79
Use the following information for questions:
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-For a significance level of = 0.05, are the results statistically significant?
A) No, results are not statistically significant because the p-value < 0.05.
B) Yes, results are statistically significant because the p-value < 0.05.
C) No, results are not statistically significant because the p-value > 0.05
D) Yes, results are statistically significant because the p-value > 0.05.
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-For a significance level of = 0.05, are the results statistically significant?
A) No, results are not statistically significant because the p-value < 0.05.
B) Yes, results are statistically significant because the p-value < 0.05.
C) No, results are not statistically significant because the p-value > 0.05
D) Yes, results are statistically significant because the p-value > 0.05.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
80
Use the following information for questions:
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-Which of the following is an appropriate conclusion?
A) The average age of first marriage for women in 1990 does appear to be greater than the average age in 1960.
B) The results are not statistically significant so there is not enough evidence to conclude that the average age in 1990 is greater than the average age in 1960.
C) The average age of marriage is at least 23 years old for both 1960 and 1990.
D) None of the above.
A researcher wants to assess if the average age when women first marry has increased from 1960 to 1990. Let = average age of first marriage for women in 1990 and = average age of first marriage for women in 1960. A random sample of 10 women married in 1990 showed an average age at marriage of 24.95 years, with a sample standard deviation of 2 years. A random sample of 20 women married in 1960 showed an average age at marriage of 23.1 years, with a sample standard deviation of 1.5 years. Assume that age of first marriage for women is normally distributed, but do not assume the population variances are equal. Use the conservative "by hand" estimate for the degrees of freedom.
-Which of the following is an appropriate conclusion?
A) The average age of first marriage for women in 1990 does appear to be greater than the average age in 1960.
B) The results are not statistically significant so there is not enough evidence to conclude that the average age in 1990 is greater than the average age in 1960.
C) The average age of marriage is at least 23 years old for both 1960 and 1990.
D) None of the above.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck



