Deck 10: Statistical Inference Concerning Two Populations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/132
Play
Full screen (f)
Deck 10: Statistical Inference Concerning Two Populations
1
Two random samples are considered independent if the observations in the first sample are related to the observations in the second sample.
False
2
The statistical inference concerning the difference between two population proportions is used for qualitative data.
True
3
We use the difference between the sample proportions 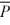 1 -
1 - 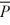 2 as the point estimator of the difference between two population proportions p1 - p2.
2 as the point estimator of the difference between two population proportions p1 - p2.
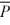 1 -
1 - 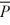 2 as the point estimator of the difference between two population proportions p1 - p2.
2 as the point estimator of the difference between two population proportions p1 - p2.True
4
The hypothesis test H0: p1 - p2 ≤ d0; HA: p1 - p2 > d0 is a left-tailed test.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
5
Statistical inference about the differences between two population means based on ________ random samples.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
6
For a statistical inference regarding μ1 - μ2, it is imperative that the sampling distribution of 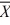 1 -
1 - 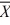 2 is normally distributed.
2 is normally distributed.
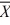 1 -
1 - 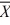 2 is normally distributed.
2 is normally distributed.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
7
To use R for solving hypothesis tests for the difference between two means, use the function ________.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
8
The t statistic is used to estimate the difference between two population proportions.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
9
For a hypothesis test about the difference of two proportions, the standard error can be improved on when the hypothesized difference does not equal zero.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
10
In the case when 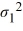 and
and 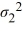 are unknown and can be assumed equal, we can calculate a pooled estimate of the population variance.
are unknown and can be assumed equal, we can calculate a pooled estimate of the population variance.
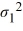 and
and 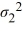 are unknown and can be assumed equal, we can calculate a pooled estimate of the population variance.
are unknown and can be assumed equal, we can calculate a pooled estimate of the population variance.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
11
We convert the estimate  1 -
1 -  2 into the corresponding value of the z or t test statistic by dividing the difference between
2 into the corresponding value of the z or t test statistic by dividing the difference between  1 -
1 -  2 and the hypothesized difference d0 by the standard error of the estimator
2 and the hypothesized difference d0 by the standard error of the estimator 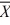 1 -
1 - 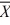 2.
2.
 1 -
1 -  2 into the corresponding value of the z or t test statistic by dividing the difference between
2 into the corresponding value of the z or t test statistic by dividing the difference between  1 -
1 -  2 and the hypothesized difference d0 by the standard error of the estimator
2 and the hypothesized difference d0 by the standard error of the estimator 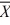 1 -
1 - 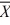 2.
2.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
12
2) multiplied by either zα/2 or tα/2,df depending on whether or not the population variances are known.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
13
A common case of dependent sampling is usually referred to as ________ sampling.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
14
The difference between the two sample means 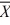 1 -
1 - 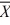 2 is an interval estimator of the difference between two population means μ1 - μ2.
2 is an interval estimator of the difference between two population means μ1 - μ2.
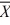 1 -
1 - 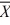 2 is an interval estimator of the difference between two population means μ1 - μ2.
2 is an interval estimator of the difference between two population means μ1 - μ2.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
15
A left-tailed test determines whether μ1 is ________ then μ2.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
16
The confidence interval for the difference μ1 - μ2 is based on the same approach used in the case of one sample: Point Estimate ± Standard Error.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
17
If the underlying populations cannot be assumed to be normal, then by the central limit theorem, the sampling distribution of 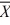 1 -
1 - 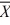 2 is approximately normal only if the sum of the sample observations is sufficiently large-that is, when n1 + n2 ≥ 30.
2 is approximately normal only if the sum of the sample observations is sufficiently large-that is, when n1 + n2 ≥ 30.
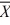 1 -
1 - 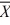 2 is approximately normal only if the sum of the sample observations is sufficiently large-that is, when n1 + n2 ≥ 30.
2 is approximately normal only if the sum of the sample observations is sufficiently large-that is, when n1 + n2 ≥ 30.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
18
There is only one type of matched-pairs sampling.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
19
We always deal with matched-pairs sampling if two samples have the same number of observations.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
20
The margin of error in the confidence interval for the difference μ1 - μ2 equals the standard error se( 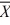 1 -
1 - 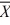
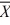 1 -
1 - 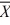

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
21
Assume the competing hypotheses take the following form H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0, where µ1 is the population mean for population 1 and µ2 is the population mean for population 2. Also assume that the populations are normally distributed, the variances are known, and independent sampling is used. Which of the following expressions is the appropriate test statistic?
A) tdf =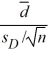
B) tdf =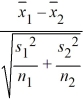
C) z =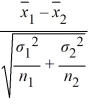
D) tdf =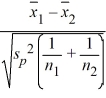
A) tdf =
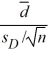
B) tdf =
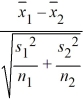
C) z =
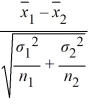
D) tdf =
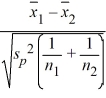

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
22
If the hypothesized difference d0 is ________, then the value of the test statistic is computed as z = 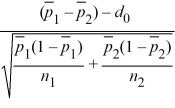 .
.
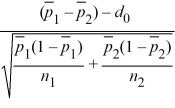 .
.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
23
Suppose you want to perform a test to compare the mean GPA of all freshmen with the mean GPA of all sophomores in a college? What type of sampling is required for this test?
A) Independent sampling with qualitative data
B) Independent sampling with quantitative data
C) Matched-pairs sampling with qualitative data
D) Matched-pairs sampling with quantitative data
A) Independent sampling with qualitative data
B) Independent sampling with quantitative data
C) Matched-pairs sampling with qualitative data
D) Matched-pairs sampling with quantitative data

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
24
2).

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
24
To use Excel for solving hypothesis tests for the mean difference you should choose:
Data >________> t-Test: Paired Two Sample for Means options.
Data >________> t-Test: Paired Two Sample for Means options.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
25
When constructing a confidence interval for the mean difference μD, we follow the same general format of point estimate ± ________.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
26
Two or more random samples are considered independent if ________.
A) the process that generates one sample is the same as the process that generates the other sample
B) the process that generates one sample is related to the process that generates the other sample
C) the process that generates one sample is influenced by the process that generates the other sample
D) the process that generates one sample is completely separate from the process that generates the other sample
A) the process that generates one sample is the same as the process that generates the other sample
B) the process that generates one sample is related to the process that generates the other sample
C) the process that generates one sample is influenced by the process that generates the other sample
D) the process that generates one sample is completely separate from the process that generates the other sample

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
27
Which of the following pairs of hypotheses are used to test if the mean of the first population is smaller than the mean of the second population, using independent random sampling?
A) H0: µD ≤ 0, HA: µD > 0
B) H0: µD ≥ 0, HA: µD < 0
C) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
D) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
A) H0: µD ≤ 0, HA: µD > 0
B) H0: µD ≥ 0, HA: µD < 0
C) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
D) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
28
We estimate the unknown standard deviation of 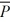 1 -
1 - 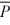 2 by the ________.
2 by the ________.
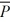 1 -
1 - 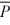 2 by the ________.
2 by the ________.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
29
When testing the difference between two population means and the population variances are unknown and unequal, the degrees of freedom is calculated as 34.7. What degrees of freedom should be used to find the p-value of the test?
A) 34
B) 34.7
C) 35
D) None of these choices is correct.
A) 34
B) 34.7
C) 35
D) None of these choices is correct.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
30
When testing the difference between two population means under independent sampling, we use the z distribution if ________.
A) the population variances are known
B) the population variances are unknown, but assumed to be equal
C) the population variances are unknown and cannot be assumed equal
D) Both the population variances are known and the population variances are unknown, but assumed to be equal
A) the population variances are known
B) the population variances are unknown, but assumed to be equal
C) the population variances are unknown and cannot be assumed equal
D) Both the population variances are known and the population variances are unknown, but assumed to be equal

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
31
Assume the competing hypotheses take the following form: H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0, where µ1 is the population mean for population 1 and µ2 is the population mean for population 2. Also assume that the populations are normally distributed and that the observations in the two samples are independent. The population variances are not known but are assumed equal. Which of the following expressions is appropriate test statistic?
A) tdf =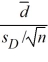
B) tdf =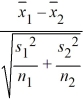
C) z =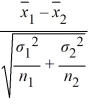
D) tdf =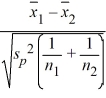
A) tdf =
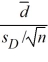
B) tdf =
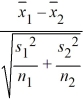
C) z =
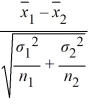
D) tdf =
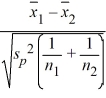

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
32
A demographer wants to measure life expectancy in countries 1 and 2. Let µ1 and µ2 denote the mean life expectancy in countries 1 and 2, respectively. Specify the hypothesis to determine if life expectancy in country 1 is more than 10 years lower than in country 2.
A) H0: µ1 - µ2 ≤ 10, HA: µ1 - µ2 > 10
B) H0: µ1 - µ2 ≥ 10, HA: µ1 - µ2 < 10
C) H0: µ1 - µ2 ≤ -10, HA: µ1 - µ2 > −10
D) H0: µ1 - µ2 ≥ -10, HA: µ1 - µ2 < −10
A) H0: µ1 - µ2 ≤ 10, HA: µ1 - µ2 > 10
B) H0: µ1 - µ2 ≥ 10, HA: µ1 - µ2 < 10
C) H0: µ1 - µ2 ≤ -10, HA: µ1 - µ2 > −10
D) H0: µ1 - µ2 ≥ -10, HA: µ1 - µ2 < −10

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
33
When calculating the standard error of 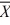 1 -
1 - 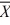 2, under what assumption do you pool the sample variances
2, under what assumption do you pool the sample variances 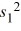 and
and 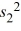 ?
?
A) Known population variances.
B) Unknown population variances that are assumed equal.
C) Unknown population variances that are assumed unequal.
D) All of these choices are correct.
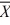 1 -
1 - 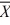 2, under what assumption do you pool the sample variances
2, under what assumption do you pool the sample variances 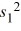 and
and 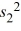 ?
?A) Known population variances.
B) Unknown population variances that are assumed equal.
C) Unknown population variances that are assumed unequal.
D) All of these choices are correct.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
34
If the underlying populations cannot be assumed to be normal, then by the central limit theorem, the sampling distribution of 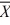 1 -
1 - 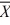 2 is approximately normal only if both sample sizes are sufficiently large-that is, when ________.
2 is approximately normal only if both sample sizes are sufficiently large-that is, when ________.
A) n1 + n2 = 30
B) n1 + n2 ≥ 30
C) n1 = 30 and n2 = 30
D) n1 ≥ 30 and n2 ≥ 30
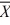 1 -
1 - 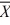 2 is approximately normal only if both sample sizes are sufficiently large-that is, when ________.
2 is approximately normal only if both sample sizes are sufficiently large-that is, when ________.A) n1 + n2 = 30
B) n1 + n2 ≥ 30
C) n1 = 30 and n2 = 30
D) n1 ≥ 30 and n2 ≥ 30

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
35
When comparing two population means, their hypothesized difference ________.
A) must be negative
B) must be positive
C) must be zero
D) may assume any value
A) must be negative
B) must be positive
C) must be zero
D) may assume any value

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
36
Assume the competing hypotheses take the following form: H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0, where µ1 is the population mean for population 1 and µ2 is the population mean for population 2. Also assume that the populations are normally distributed and we use independent sampling. The population variances are not known and assumed to be unequal. Which of the following expressions is the appropriate test statistic?
A) tdf =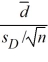
B) tdf =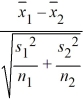
C) z =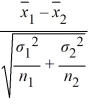
D) tdf =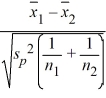
A) tdf =
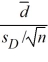
B) tdf =
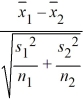
C) z =
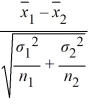
D) tdf =
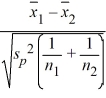

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
37
If the sampling distribution of 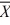 1 -
1 - 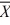 2 cannot be assumed normal, we ________.
2 cannot be assumed normal, we ________.
A) are unable to compute a confidence interval
B) are still able to use the z distribution to compute a confidence interval
C) must use the tdf distribution with n1 + n2 - 2 degrees of freedom to compute a confidence interval
D) must use the tdf distribution and calculate the degrees of freedom using n1, n2, s1, and s2 to compute a confidence interval
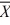 1 -
1 - 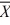 2 cannot be assumed normal, we ________.
2 cannot be assumed normal, we ________.A) are unable to compute a confidence interval
B) are still able to use the z distribution to compute a confidence interval
C) must use the tdf distribution with n1 + n2 - 2 degrees of freedom to compute a confidence interval
D) must use the tdf distribution and calculate the degrees of freedom using n1, n2, s1, and s2 to compute a confidence interval

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
38
The choice of an appropriate test for comparing two population means depends on whether we deal with ________.
A) qualitative or quantitative data
B) independent or matched-pairs sampling
C) the equality or lack of equality of population variances
D) All of these choices are correct
A) qualitative or quantitative data
B) independent or matched-pairs sampling
C) the equality or lack of equality of population variances
D) All of these choices are correct

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
39
What formula is used to calculate the degrees of freedom for the t test for comparing two population means when the population variances are unknown and unequal?
A) df = n - 1
B) df = n1 + n2 - 2
C) df = n1 + n2 - 1
D) df =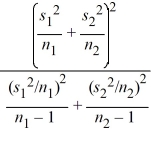
A) df = n - 1
B) df = n1 + n2 - 2
C) df = n1 + n2 - 1
D) df =
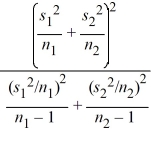

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
40
Which of the following is not a restriction for comparing two population means?
A) A normally distributed sampling distribution of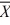 1 -
1 - 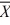 2
2
B) Matched-pairs sampling
C) Independent random samples
D) Equal or unequal variances
A) A normally distributed sampling distribution of
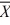 1 -
1 - 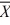 2
2 B) Matched-pairs sampling
C) Independent random samples
D) Equal or unequal variances

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
41
A farmer uses a lot of fertilizer to grow his crops. The farmer's manager thinks fertilizer products from distributor A contain more of the nitrogen that his plants need than distributor B's fertilizer does. He takes two independent samples of four batches of fertilizer from each distributor and measures the amount of nitrogen in each batch. Fertilizer from distributor A contained 23 pounds per batch and fertilizer from distributor B contained 18 pounds per batch. Suppose the population standard deviation for distributor A and distributor B is four pounds per batch and five pounds per batch, respectively. Assume the distribution of nitrogen in fertilizer is normally distributed. Let µ1 and µ2 represent the average amount of nitrogen per batch for fertilizer's A and B, respectively. Which of the following is an appropriate p-value?
A) 0.0592
B) 0.0847
C) 0.0896
D) 0.1184
A) 0.0592
B) 0.0847
C) 0.0896
D) 0.1184

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
42
A restaurant chain has two locations in a medium-sized town and, believing that it has oversaturated the market for its food, is considering closing one of the restaurants. The manager of the restaurant with a downtown location claims that his restaurant generates more revenue than the sister restaurant by the freeway. The CEO of this company, wishing to test this claim, randomly selects 36 monthly revenue totals for each restaurant. The revenue data from the downtown restaurant have a mean of $360,000 and a standard deviation of $50,000, while the data from the restaurant by the freeway have a mean of $340,000 and a standard deviation of $40,000. Assume there is no reason to believe the population standard deviations are equal, and let μ1 and μ2 denote the mean monthly revenue of the downtown restaurant and the restaurant by the freeway, respectively. Which of the following hypotheses should be used to test the manager's claim?
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0
D) H0: µ1 - µ2 > 0, HA: µ1 - µ2 ≤ 0
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0
D) H0: µ1 - µ2 > 0, HA: µ1 - µ2 ≤ 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
43
A farmer uses a lot of fertilizer to grow his crops. The farmer's manager thinks fertilizer products from distributor A contain more of the nitrogen that his plants need than distributor B's fertilizer does. He takes two independent samples of four batches of fertilizer from each distributor and measures the amount of nitrogen in each batch. Fertilizer from distributor A contained 23 pounds per batch and fertilizer from distributor B contained 18 pounds per batch. Suppose the population standard deviation for distributor A and distributor B is four pounds per batch and five pounds per batch, respectively. Assume the distribution of nitrogen in fertilizer is normally distributed. Let µ1 and µ2 represent the average amount of nitrogen per batch for fertilizer's A and B, respectively. Which of the following is the appropriate conclusion at the 5% significance level?
A) Reject H0; we can conclude that the mean amount of fertilizer per batch for distributor A is greater than the amount of fertilizer per batch for distributor B.
B) Reject H0; we cannot conclude that the mean amount of fertilizer per batch for distributor A is greater than the amount of fertilizer per batch for distributor B.
C) Fail to reject H0; we can conclude that the mean amount of fertilizer per batch for distributor A is greater than the amount of fertilizer per batch for distributor B.
D) Fail to reject H0; we cannot conclude that the mean amount of fertilizer per batch for distributor A is greater than the amount of fertilizer per batch for distributor B.
A) Reject H0; we can conclude that the mean amount of fertilizer per batch for distributor A is greater than the amount of fertilizer per batch for distributor B.
B) Reject H0; we cannot conclude that the mean amount of fertilizer per batch for distributor A is greater than the amount of fertilizer per batch for distributor B.
C) Fail to reject H0; we can conclude that the mean amount of fertilizer per batch for distributor A is greater than the amount of fertilizer per batch for distributor B.
D) Fail to reject H0; we cannot conclude that the mean amount of fertilizer per batch for distributor A is greater than the amount of fertilizer per batch for distributor B.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
44
What type of data is required to compare prices of the same textbooks sold by two different vendors?
A) Dependent random samples with qualitative data.
B) Dependent random samples with quantitative data.
C) Independent random samples with qualitative data.
D) Independent random samples with quantitative data.
A) Dependent random samples with qualitative data.
B) Dependent random samples with quantitative data.
C) Independent random samples with qualitative data.
D) Independent random samples with quantitative data.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
45
A restaurant chain has two locations in a medium-sized town and, believing that it has oversaturated the market for its food, is considering closing one of the restaurants. The manager of the restaurant with a downtown location claims that his restaurant generates more revenue than the sister restaurant by the freeway. The CEO of this company, wishing to test this claim, randomly selects 36 monthly revenue totals for each restaurant. The revenue data from the downtown restaurant have a mean of $360,000 and a standard deviation of $50,000, while the data from the restaurant by the freeway have a mean of $340,000 and a standard deviation of $40,000. Assume there is no reason to believe the population standard deviations are equal, and let μ1 and μ2 denote the mean monthly revenue of the downtown restaurant and the restaurant by the freeway, respectively. Which of the following is the correct value of the test statistic to analyze the claim?
A) t66 = 1.848
B) t67 = 1.848
C) t66 = 1.874
D) t67 = 1.874
A) t66 = 1.848
B) t67 = 1.848
C) t66 = 1.874
D) t67 = 1.874

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
46
What type of test for population means should be performed when employees are first tested, trained, and then retested?
A) A z test under independent sampling with known population variances.
B) A t test under independent sampling with unknown but equal population variances.
C) A t test under dependent sampling.
D) A t test under independent sampling with unknown and unequal population variances.
A) A z test under independent sampling with known population variances.
B) A t test under independent sampling with unknown but equal population variances.
C) A t test under dependent sampling.
D) A t test under independent sampling with unknown and unequal population variances.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
47
What type of data should be collected to compare the likelihoods of two candidates winning their elections when the candidates are running in different elections?
A) Matched-pairs sampling with qualitative data.
B) Matched-pairs sampling with quantitative data.
C) Independent sampling with qualitative data.
D) Independent sampling with quantitative data.
A) Matched-pairs sampling with qualitative data.
B) Matched-pairs sampling with quantitative data.
C) Independent sampling with qualitative data.
D) Independent sampling with quantitative data.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
48
Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling.
A) In every case.
B) Only if n1 ≥ 30 and n2 ≥ 30.
C) Only if n1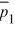 ≥ 5, n1(1 -
≥ 5, n1(1 - 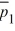 ) ≥ 5, n2
) ≥ 5, n2
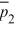 ≥ 5, and n2(1 -
≥ 5, and n2(1 - 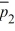 ) ≥ 5.
) ≥ 5.
D) Only if n1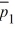 ≥ 30, n1(1 -
≥ 30, n1(1 - 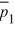 ) ≥ 30, n2
) ≥ 30, n2
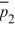 ≥ 30, and n2(1 -
≥ 30, and n2(1 - 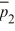 ) ≥ 30.
) ≥ 30.
A) In every case.
B) Only if n1 ≥ 30 and n2 ≥ 30.
C) Only if n1
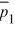 ≥ 5, n1(1 -
≥ 5, n1(1 - 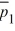 ) ≥ 5, n2
) ≥ 5, n2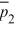 ≥ 5, and n2(1 -
≥ 5, and n2(1 - 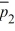 ) ≥ 5.
) ≥ 5.D) Only if n1
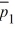 ≥ 30, n1(1 -
≥ 30, n1(1 - 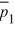 ) ≥ 30, n2
) ≥ 30, n2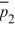 ≥ 30, and n2(1 -
≥ 30, and n2(1 - 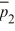 ) ≥ 30.
) ≥ 30.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
49
You would like to determine if there is a higher incidence of smoking among women than among men in a neighborhood. Let men and women be represented by populations 1 and 2, respectively. Which of the following hypotheses is relevant to this claim?
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: p1 - p2 ≤ 0, HA: p1 - p2 > 0
D) H0: p1 - p2 ≥ 0, HA: p1 - p2 < 0
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: p1 - p2 ≤ 0, HA: p1 - p2 > 0
D) H0: p1 - p2 ≥ 0, HA: p1 - p2 < 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
50
When the hypothesized difference of the population proportions is equal to 0, we ________.
A) use a matched-pairs approach
B) are able to estimate the standard error of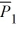 -
- 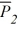 using the pooled
using the pooled 
C) can use the confidence interval to implement the test if the difference of the population proportions is equal to 0
D) Both estimate the standard error of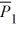 -
- 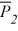 using the pooled
using the pooled  and use the confidence interval to implement the test if the difference of the population proportions is equal to 0
and use the confidence interval to implement the test if the difference of the population proportions is equal to 0
A) use a matched-pairs approach
B) are able to estimate the standard error of
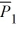 -
- 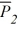 using the pooled
using the pooled 
C) can use the confidence interval to implement the test if the difference of the population proportions is equal to 0
D) Both estimate the standard error of
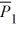 -
- 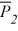 using the pooled
using the pooled  and use the confidence interval to implement the test if the difference of the population proportions is equal to 0
and use the confidence interval to implement the test if the difference of the population proportions is equal to 0
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
51
You would like to determine if there is a higher incidence of smoking among women than among men in a neighborhood. Let women and men be represented by populations 1 and 2, respectively. Which of the following hypotheses is relevant to this claim?
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: p1 - p2 ≤ 0, HA: p1 - p2 > 0
D) H0: p1 - p2 ≥ 0, HA: p1 - p2 < 0
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: p1 - p2 ≤ 0, HA: p1 - p2 > 0
D) H0: p1 - p2 ≥ 0, HA: p1 - p2 < 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
52
A particular personal trainer works primarily with track and field athletes. She believes that her clients run faster after going through her program for six weeks. How might she test that claim?
A) A hypothesis test for p1 - p2.
B) A hypothesis test for μ1 - μ2.
C) A matched-pairs hypothesis test for μD.
D) We are unable to conduct a hypothesis test because the samples would not be independent.
A) A hypothesis test for p1 - p2.
B) A hypothesis test for μ1 - μ2.
C) A matched-pairs hypothesis test for μD.
D) We are unable to conduct a hypothesis test because the samples would not be independent.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
53
Calcium is an essential nutrient for strong bones and for controlling blood pressure and heart beat. Because most of the body's calcium is stored in bones and teeth, the body withdraws the calcium it needs from the bones. Over time, if more calcium is taken out of the bones than is put in, the result may be thin, weak bones. This is especially important for women who are often recommended a calcium supplement. A consumer group activist assumes that calcium content in two popular supplements are normally distributed with the same unknown population variance, and uses the following information obtained under independent sampling:  Let μ1 and μ2 denote the corresponding population means. Can we conclude that the average calcium content of the two supplements differs at the 95% confidence level?
Let μ1 and μ2 denote the corresponding population means. Can we conclude that the average calcium content of the two supplements differs at the 95% confidence level?
A) No, because the 95% confidence interval contains the hypothesized value of zero.
B) Yes, because the 95% confidence interval contains the hypothesized value of zero.
C) No, because the 95% confidence interval does not contain the hypothesized value of zero.
D) Yes, because the 95% confidence interval does not contain the hypothesized value of zero.
 Let μ1 and μ2 denote the corresponding population means. Can we conclude that the average calcium content of the two supplements differs at the 95% confidence level?
Let μ1 and μ2 denote the corresponding population means. Can we conclude that the average calcium content of the two supplements differs at the 95% confidence level?A) No, because the 95% confidence interval contains the hypothesized value of zero.
B) Yes, because the 95% confidence interval contains the hypothesized value of zero.
C) No, because the 95% confidence interval does not contain the hypothesized value of zero.
D) Yes, because the 95% confidence interval does not contain the hypothesized value of zero.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
54
A farmer uses a lot of fertilizer to grow his crops. The farmer's manager thinks fertilizer products from distributor A contain more of the nitrogen that his plants need than distributor B's fertilizer does. He takes two independent samples of four batches of fertilizer from each distributor and measures the amount of nitrogen in each batch. Fertilizer from distributor A contained 23 pounds per batch and fertilizer from distributor B contained 18 pounds per batch. Suppose the population standard deviation for distributor A and distributor B is four pounds per batch and five pounds per batch, respectively. Assume the distribution of nitrogen in fertilizer is normally distributed. Let µ1 and µ2 represent the average amount of nitrogen per batch for fertilizer's A and B, respectively. Which of the following is the correct value of the test statistic?
A) t6 = −1.5617
B) t6 = 1.5617
C) z = −1.5617
D) z = 1.5617
A) t6 = −1.5617
B) t6 = 1.5617
C) z = −1.5617
D) z = 1.5617

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
55
A particular bank has two loan modification programs for distressed borrowers: Home Affordable Modification Program (HAMP) modifications, where the federal government pays the bank $1,000 for each successful modification, and non-HAMP modifications, where the bank does not receive a bonus from the federal government. To qualify for a HAMP modification, borrowers must meet a set of financial suitability criteria. Define the null and alternative hypotheses to test whether borrowers who receive HAMP modifications default less than borrowers who receive non-HAMP modifications. Let p1 and p2 represent the proportion of borrowers who received HAMP and non-HAMP modifications that did not re-default, respectively.
A) H0: p1 - p2 ≤ 0, HA: p1 - p2 > 0
B) H0: p1 - p2 ≥ 0, HA: p1 - p2 < 0
C) H0: p1 - p2 = 0, HA: p1 - p2 ≠ 0
D) H0: p1 - p2 > 0, HA: p1 - p2 ≤ 0
A) H0: p1 - p2 ≤ 0, HA: p1 - p2 > 0
B) H0: p1 - p2 ≥ 0, HA: p1 - p2 < 0
C) H0: p1 - p2 = 0, HA: p1 - p2 ≠ 0
D) H0: p1 - p2 > 0, HA: p1 - p2 ≤ 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
56
A particular bank has two loan modification programs for distressed borrowers: Home Affordable Modification Program (HAMP) modifications, where the federal government pays the bank $1,000 for each successful modification, and non-HAMP modifications, where the bank does not receive a bonus from the federal government. To qualify for a HAMP modification, borrowers must meet a set of financial suitability criteria. What type of hypothesis test should we use to test whether borrowers from this particular bank who receive HAMP modifications are more likely to re-default than those who receive non-HAMP modifications?
A) A hypothesis test for p1 - p2.
B) A hypothesis test for µ1 - µ2.
C) A matched-pairs hypothesis test.
D) We are unable to conduct a hypothesis test because independent random samples of each group could not be collected.
A) A hypothesis test for p1 - p2.
B) A hypothesis test for µ1 - µ2.
C) A matched-pairs hypothesis test.
D) We are unable to conduct a hypothesis test because independent random samples of each group could not be collected.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
57
Which of the following set of hypotheses is used to test if the mean of the first population is smaller than the mean of the second population, using matched-paired sampling?
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: µD ≤ 0, HA: µD > 0
D) H0: µD ≥ 0, HA: µD < 0
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: µD ≤ 0, HA: µD > 0
D) H0: µD ≥ 0, HA: µD < 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
58
A farmer uses a lot of fertilizer to grow his crops. The farmer's manager thinks fertilizer products from distributor A contain more of the nitrogen that his plants need than distributor B's fertilizer does. He takes two independent samples of four batches of fertilizer from each distributor and measures the amount of nitrogen in each batch. Fertilizer from distributor A contained 23 pounds per batch and fertilizer from distributor B contained 18 pounds per batch. Suppose the population standard deviation for distributor A and distributor B is four pounds per batch and five pounds per batch, respectively. Assume the distribution of nitrogen in fertilizer is normally distributed. Let µ1 and µ2 represent the average amount of nitrogen per batch for fertilizer's A and B, respectively. Specify the competing hypotheses to determine if fertilizer A contains more nitrogen per batch than fertilizer B.
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: p1 - p2 ≤ 0, HA: p1 - p2 > 0
D) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: p1 - p2 ≤ 0, HA: p1 - p2 > 0
D) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
59
Calcium is an essential nutrient for strong bones and for controlling blood pressure and heart beat. Because most of the body's calcium is stored in bones and teeth, the body withdraws the calcium it needs from the bones. Over time, if more calcium is taken out of the bones than is put in, the result may be thin, weak bones. This is especially important for women who are often recommended a calcium supplement. A consumer group activist assumes that calcium content in two popular supplements are normally distributed with the same unknown population variance, and uses the following information obtained under independent sampling: ![<strong>Calcium is an essential nutrient for strong bones and for controlling blood pressure and heart beat. Because most of the body's calcium is stored in bones and teeth, the body withdraws the calcium it needs from the bones. Over time, if more calcium is taken out of the bones than is put in, the result may be thin, weak bones. This is especially important for women who are often recommended a calcium supplement. A consumer group activist assumes that calcium content in two popular supplements are normally distributed with the same unknown population variance, and uses the following information obtained under independent sampling: Let μ<sub>1</sub> and μ<sub>2</sub> denote the corresponding population means. Construct a 95% confidence interval for the difference μ<sub>1</sub> - μ<sub>2</sub>.</strong> A) [-30.9386, 1.0614] B) [-31.5886, -0.4114] C) [-33.8007, 1.8007] D) [-34.8012, 2.8012]](https://d2lvgg3v3hfg70.cloudfront.net/TB6618/11ea8309_a8c8_60ef_b223_ade8382cb9ec_TB6618_00.jpg) Let μ1 and μ2 denote the corresponding population means. Construct a 95% confidence interval for the difference μ1 - μ2.
Let μ1 and μ2 denote the corresponding population means. Construct a 95% confidence interval for the difference μ1 - μ2.
A) [-30.9386, 1.0614]
B) [-31.5886, -0.4114]
C) [-33.8007, 1.8007]
D) [-34.8012, 2.8012]
![<strong>Calcium is an essential nutrient for strong bones and for controlling blood pressure and heart beat. Because most of the body's calcium is stored in bones and teeth, the body withdraws the calcium it needs from the bones. Over time, if more calcium is taken out of the bones than is put in, the result may be thin, weak bones. This is especially important for women who are often recommended a calcium supplement. A consumer group activist assumes that calcium content in two popular supplements are normally distributed with the same unknown population variance, and uses the following information obtained under independent sampling: Let μ<sub>1</sub> and μ<sub>2</sub> denote the corresponding population means. Construct a 95% confidence interval for the difference μ<sub>1</sub> - μ<sub>2</sub>.</strong> A) [-30.9386, 1.0614] B) [-31.5886, -0.4114] C) [-33.8007, 1.8007] D) [-34.8012, 2.8012]](https://d2lvgg3v3hfg70.cloudfront.net/TB6618/11ea8309_a8c8_60ef_b223_ade8382cb9ec_TB6618_00.jpg) Let μ1 and μ2 denote the corresponding population means. Construct a 95% confidence interval for the difference μ1 - μ2.
Let μ1 and μ2 denote the corresponding population means. Construct a 95% confidence interval for the difference μ1 - μ2.A) [-30.9386, 1.0614]
B) [-31.5886, -0.4114]
C) [-33.8007, 1.8007]
D) [-34.8012, 2.8012]

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
60
A restaurant chain has two locations in a medium-sized town and, believing that it has oversaturated the market for its food, is considering closing one of the restaurants. The manager of the restaurant with a downtown location claims that his restaurant generates more revenue than the sister restaurant by the freeway. The CEO of this company, wishing to test this claim, randomly selects 36 monthly revenue totals for each restaurant. The revenue data from the downtown restaurant have a mean of $360,000 and a standard deviation of $50,000, while the data from the restaurant by the freeway have a mean of $340,000 and a standard deviation of $40,000. Assume there is no reason to believe the population standard deviations are equal, and let μ1 and μ2 denote the mean monthly revenue of the downtown restaurant and the restaurant by the freeway, respectively. Which of the following is the appropriate critical value(s) to test the manager's claim at the 5% significance level?
A) 1.645
B) 1.668
C) 1.960
D) 1.997
A) 1.645
B) 1.668
C) 1.960
D) 1.997

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
61
A university wants to compare out-of-state applicants' mean SAT math scores (μ1) to in-state applicants' mean SAT math scores (μ2). The university looks at 35 in-state applicants and 35 out-of-state applicants. The mean SAT math score for in-state applicants was 540, with a standard deviation of 20. The mean SAT math score for out-of-state applicants was 555, with a standard deviation of 25. It is reasonable to assume the corresponding population standard deviations are equal. At the 5% significance level, can the university conclude that the mean SAT math score for in-state students and out-of-state students differ?
A) No, because the confidence interval contains zero.
B) Yes, because the confidence interval contains zero.
C) No, because the confidence interval does not contain zero.
D) Yes, because the confidence interval does not contain zero.
A) No, because the confidence interval contains zero.
B) Yes, because the confidence interval contains zero.
C) No, because the confidence interval does not contain zero.
D) Yes, because the confidence interval does not contain zero.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
62
A tutor promises to improve GMAT scores of students by more than 50 points after three lessons. To see if this is true, the tutor takes a sample of 49 students' test scores after and before they received tutoring. The mean difference was 53 points better after tutoring, with a standard deviation of the difference equal to 12 points. Let µD denote the mean of the difference: score after tutoring minus score before tutoring. Which of the following is the correct value of the test statistic?
A) z = 1.7143
B) z = 1.7500
C) t48 = 1.7143
D) t48 = 1.7500
A) z = 1.7143
B) z = 1.7500
C) t48 = 1.7143
D) t48 = 1.7500

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
63
A producer of fine chocolates believes that the sales of two varieties of truffles differ significantly during the holiday season. The first variety is milk chocolate while the second is milk chocolate filled with mint. It is reasonable to assume that truffle sales are normally distributed with unknown but equal population variances. Two independent samples of 18 observations each are collected for the holiday period. A sample mean of 12 million milk chocolate truffles sold with a sample standard deviation of 2.5 million. A sample mean of 13.5 million truffles filled with mint sold with a sample standard deviation of 2.3 million. Use milk chocolate as population 1 and mint chocolate as population 2. Which of the following are the appropriate hypotheses to determine if the average sales of the two varieties of truffles differ significantly during the holiday season?
A) H0: µD ≥ 0, HA: µD < 0
B) H0: µD = 0, HA: µD ≠ 0
C) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
D) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0
A) H0: µD ≥ 0, HA: µD < 0
B) H0: µD = 0, HA: µD ≠ 0
C) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
D) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
64
A 7,000-seat theater is interested in determining whether there is a difference in attendance between shows on Tuesday evening and those on Wednesday evening. Two independent samples of 25 weeks are collected for Tuesday and Wednesday. The mean attendance on Tuesday evening is calculated as 5,500, while the mean attendance on Wednesday evening is calculated as 5,850. The known population standard deviation for attendance on Tuesday evening is 550 and the known population standard deviation for attendance on Wednesday evening is 445. Which of the following is the appropriate decision given a 5% level of significance?
A) Conclude that the mean attendance differs because the p-value = 0.0067 < 0.05.
B) Conclude that the mean attendance differs because the p-value = 0.0134 < 0.05.
C) Do not conclude that the mean attendance differs because the p-value = 0.0067 < 0.05.
D) Do not conclude that the mean attendance differs because the p-value = 0.0134 < 0.05.
A) Conclude that the mean attendance differs because the p-value = 0.0067 < 0.05.
B) Conclude that the mean attendance differs because the p-value = 0.0134 < 0.05.
C) Do not conclude that the mean attendance differs because the p-value = 0.0067 < 0.05.
D) Do not conclude that the mean attendance differs because the p-value = 0.0134 < 0.05.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
65
A new sales training program has been instituted at a rent-to-own company. Prior to the training, 10 employees were tested on their knowledge of products offered by the company. Once the training was completed, the employees were tested again in an effort to determine whether the training program was effective. Scores are known to be normally distributed. The sample scores on the tests are listed next. Use pre-test score as µ1 for population 1 and post-test score as µ2 for population 2, or µD as the difference calculated as pre-test score minus post-test score. 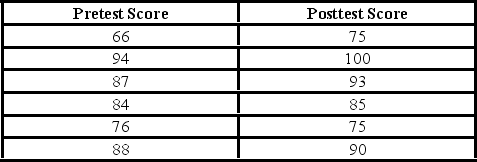 Which of the following is the correct conclusion for the test at α = 0.05?
Which of the following is the correct conclusion for the test at α = 0.05?
A) Given the critical value 1.645, we conclude that training increases scores on average.
B) Given the critical value 2.015, we conclude that training increases scores on average.
C) Given the critical value −2.015, we conclude that training increases scores on average.
D) Given the critical value −1.645, we conclude that training increases scores on average.
 Which of the following is the correct conclusion for the test at α = 0.05?
Which of the following is the correct conclusion for the test at α = 0.05?A) Given the critical value 1.645, we conclude that training increases scores on average.
B) Given the critical value 2.015, we conclude that training increases scores on average.
C) Given the critical value −2.015, we conclude that training increases scores on average.
D) Given the critical value −1.645, we conclude that training increases scores on average.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
66
A producer of fine chocolates believes that the sales of two varieties of truffles differ significantly during the holiday season. The first variety is milk chocolate while the second is milk chocolate filled with mint. It is reasonable to assume that truffle sales are normally distributed with unknown but equal population variances. Two independent samples of 18 observations each are collected for the holiday period. A sample mean of 12 million milk chocolate truffles sold with a sample standard deviation of 2.5 million. A sample mean of 13.5 million truffles filled with mint sold with a sample standard deviation of 2.3 million. Use milk chocolate as population 1 and mint chocolate as population 2. Which of the following is the appropriate decision given a 5% level of significance?
A) Conclude that the average milk chocolate and mint chocolate sales differ because the p-value is greater than 0.05.
B) Conclude that the average milk chocolate and mint chocolate sales do not differ because the p-value is less than 0.05.
C) Do not conclude that the average milk chocolate and mint chocolate sales differ because the p-value is greater than 0.05.
D) Do not conclude that the average milk chocolate and mint chocolate sales do not differ because the p-value is less than 0.05.
A) Conclude that the average milk chocolate and mint chocolate sales differ because the p-value is greater than 0.05.
B) Conclude that the average milk chocolate and mint chocolate sales do not differ because the p-value is less than 0.05.
C) Do not conclude that the average milk chocolate and mint chocolate sales differ because the p-value is greater than 0.05.
D) Do not conclude that the average milk chocolate and mint chocolate sales do not differ because the p-value is less than 0.05.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
67
A 7,000-seat theater is interested in determining whether there is a difference in attendance between shows on Tuesday evening and those on Wednesday evening. Two independent samples of 25 weeks are collected for Tuesday and Wednesday. The mean attendance on Tuesday evening is calculated as 5,500, while the mean attendance on Wednesday evening is calculated as 5,850. The known population standard deviation for attendance on Tuesday evening is 550 and the known population standard deviation for attendance on Wednesday evening is 445. Which of the following is the value of the appropriate test statistic?
A) z = -2.4736
B) z = 2.4736
C) tdf = -2.4736
D) tdf = 2.4736
A) z = -2.4736
B) z = 2.4736
C) tdf = -2.4736
D) tdf = 2.4736

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
68
A 7,000-seat theater is interested in determining whether there is a difference in attendance between shows on Tuesday evening and those on Wednesday evening. A random sample of 25 weeks is collected for Tuesday a different sample of 25 weeks is collected for Wednesday. The mean attendance on Tuesday evening is calculated as 5,500, while the mean attendance on Wednesday evening is calculated as 5,850. The known population standard deviation for attendance on Tuesday evening is 550 and the known population standard deviation for attendance on Wednesday evening is 445. Let μ1 be the population mean of Tuesday, μ2 be the population mean of Wednesday, and μD be the mean difference for a matched-pairs sampling. What are the appropriate hypotheses to determine whether there is a difference, on average, in attendance between shows on Tuesday evening and Wednesday evening?
A) H0: µD ≥ 0, HA: µD < 0
B) H0: µD = 0, HA: µD ≠ 0
C) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0
D) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
A) H0: µD ≥ 0, HA: µD < 0
B) H0: µD = 0, HA: µD ≠ 0
C) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0
D) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
69
A tutor promises to improve GMAT scores of students by more than 50 points after three lessons. To see if this is true, the tutor takes a sample of 49 students' test scores after and before they received tutoring. The mean difference was 53 points better after tutoring, with a standard deviation of the difference equal to 12 points. Let µD denote the mean of the difference: score after tutoring minus score before tutoring. Which of the following hypotheses will determine if the students improved their test scores by more than 50 points after being tutored?
A) H0: µD ≤ 0, HA: µD > 0
B) H0: µD ≥ 0, HA: µD < 0
C) H0: µD ≤ 50, HA: µD > 50
D) H0: µD ≥ 50, HA: µD < 50
A) H0: µD ≤ 0, HA: µD > 0
B) H0: µD ≥ 0, HA: µD < 0
C) H0: µD ≤ 50, HA: µD > 50
D) H0: µD ≥ 50, HA: µD < 50

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
70
A statistics professor at a large university hypothesizes that students who take statistics in the morning typically do better than those who take it in the afternoon. He takes a random sample of 36 students who took a morning class and, independently, another random sample of 36 students who took an afternoon class. He finds that the morning group scored an average of 74 with a standard deviation of 8, while the evening group scored an average of 68 with a standard deviation of 10. The population standard deviation of scores is unknown but is assumed to be equal for morning and evening classes. Let µ1 and µ2 represent the population mean final exam scores of statistics' courses offered in the morning and the afternoon, respectively. Compute the appropriate test statistic to analyze the claim at the 1% significance level.
A) t70 = −2.811
B) t70 = 2.811
C) z = −2.811
D) z = 2.811
A) t70 = −2.811
B) t70 = 2.811
C) z = −2.811
D) z = 2.811

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
71
A restaurant chain has two locations in a medium-sized town and, believing that it has oversaturated the market for its food, is considering closing one of the restaurants. The manager of the restaurant with a downtown location claims that his restaurant generates more revenue than the sister restaurant by the freeway. The CEO of this company, wishing to test this claim, randomly selects 36 monthly revenue totals for each restaurant. The revenue data from the downtown restaurant have a mean of $360,000 and a standard deviation of $50,000, while the data from the restaurant by the freeway have a mean of $340,000 and a standard deviation of $40,000. Assume there is no reason to believe the population standard deviations are equal, and let μ1 and μ2 denote the mean monthly revenue of the downtown restaurant and the restaurant by the freeway, respectively. At the 5% significance level, does the evidence support the manager's claim?
A) No, because the test statistic value is less than the critical value.
B) Yes, because the test statistic value is less than the critical value.
C) No, because the test statistic value is greater than the critical value.
D) Yes, because the test statistic value is greater than the critical value.
A) No, because the test statistic value is less than the critical value.
B) Yes, because the test statistic value is less than the critical value.
C) No, because the test statistic value is greater than the critical value.
D) Yes, because the test statistic value is greater than the critical value.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
72
A new sales training program has been instituted at a rent-to-own company. Prior to the training, 10 employees were tested on their knowledge of products offered by the company. Once the training was completed, the employees were tested again in an effort to determine whether the training program was effective. Scores are known to be normally distributed. The sample scores on the tests are listed next. Use pretest score as µ1 for population 1 and post-test score as µ2 for population 2, or µD as the mean of the difference calculated as pre-test score minus post-test score. 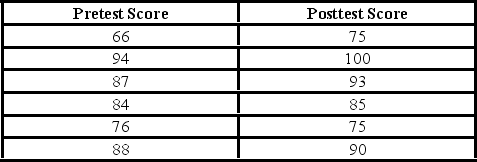 Which of the following are the appropriate hypotheses to determine if the training increases scores?
Which of the following are the appropriate hypotheses to determine if the training increases scores?
A) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
B) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
C) H0: µD ≥ 0, HA: µD < 0
D) H0: µD ≤ 0, HA: µD > 0
 Which of the following are the appropriate hypotheses to determine if the training increases scores?
Which of the following are the appropriate hypotheses to determine if the training increases scores?A) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
B) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
C) H0: µD ≥ 0, HA: µD < 0
D) H0: µD ≤ 0, HA: µD > 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
73
A statistics professor at a large university hypothesizes that students who take statistics in the morning typically do better than those who take it in the afternoon. He takes a random sample of 36 students who took a morning class and, independently, another random sample of 36 students who took an afternoon class. He finds that the morning group scored an average of 74 with a standard deviation of 8, while the evening group scored an average of 68 with a standard deviation of 10. The population standard deviation of scores is unknown but is assumed to be equal for morning and evening classes. Let µ1 and µ2 represent the population mean final exam scores of statistics' courses offered in the morning and the afternoon, respectively. Which of the following is(are) the appropriate critical value(s) to test the professor's claim at the 1% significance level?
A) -2.381 and 2.381
B) -2.326 and 2.326
C) 2.326
D) 2.381
A) -2.381 and 2.381
B) -2.326 and 2.326
C) 2.326
D) 2.381

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
74
A university wants to compare out-of-state applicants' mean SAT math scores (μ1) to in-state applicants; mean SAT math scores (μ2). The university looks at 35 in-state applicants and 35 out-of-state applicants. The mean SAT math score for in-state applicants was 540, with a standard deviation of 20. The mean SAT math score for out-of-state applicants was 555, with a standard deviation of 25. It is reasonable to assume the corresponding population standard deviations are equal. To calculate the confidence interval for the difference μ1 - μ2, what is the number of degrees of freedom of the appropriate probability distribution?
A) 64
B) 64.87
C) 68
D) 69
A) 64
B) 64.87
C) 68
D) 69

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
75
A new sales training program has been instituted at a rent-to-own company. Prior to the training, 10 employees were tested on their knowledge of products offered by the company. Once the training was completed, the employees were tested again in an effort to determine whether the training program was effective. Scores are known to be normally distributed. The sample scores on the tests are listed next. Use pre-test score as µ1 for population 1 and post-test score as µ2 for population 2, or µD as the difference calculated as pre-test score minus post-test score. 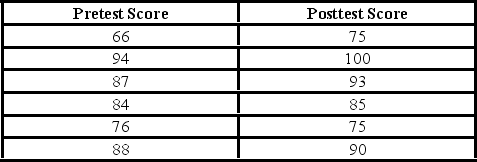 Which of the following is the value of the appropriate test statistic?
Which of the following is the value of the appropriate test statistic?
A) t5 = −2.4947
B) t5 = 2.4947
C) z = −2.4947
D) z = 2.4947
 Which of the following is the value of the appropriate test statistic?
Which of the following is the value of the appropriate test statistic?A) t5 = −2.4947
B) t5 = 2.4947
C) z = −2.4947
D) z = 2.4947

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
76
A university wants to compare out-of-state applicants' mean SAT math scores (μ1) to in-state applicants' mean SAT math scores (μ2). The university looks at 35 in-state applicants and 35 out-of-state applicants. The mean SAT math score for in-state applicants was 540, with a standard deviation of 20. The mean SAT math score for out-of-state applicants was 555, with a standard deviation of 25. It is reasonable to assume the corresponding population standard deviations are equal. Calculate a 95% confidence interval for the difference μ1 - μ2.
A) [-25.6067, -4.3933]
B) [-25.7961, -4.2039]
C) [-25.8124, -4.1876]
D) [-33.6105, 3.6105]
A) [-25.6067, -4.3933]
B) [-25.7961, -4.2039]
C) [-25.8124, -4.1876]
D) [-33.6105, 3.6105]

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
77
A producer of fine chocolates believes that the sales of two varieties of truffles differ significantly during the holiday season. The first variety is milk chocolate while the second is milk chocolate filled with mint. It is reasonable to assume that truffle sales are normally distributed with unknown but equal population variances. Two independent samples of 18 observations each are collected for the holiday period. A sample mean of 12 million milk chocolate truffles sold with a sample standard deviation of 2.5 million. A sample mean of 13.5 million truffles filled with mint sold with a sample standard deviation of 2.3 million. Use milk chocolate as population 1 and mint chocolate as population 2. Assuming the population variances are equal, which of the following is the value of the appropriate test statistic?
A) z = −1.8734
B) z = 1.8734
C) t34 = −1.8734
D) t34 = 1.8734
A) z = −1.8734
B) z = 1.8734
C) t34 = −1.8734
D) t34 = 1.8734

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
78
A statistics professor at a large university hypothesizes that students who take statistics in the morning typically do better than those who take it in the afternoon. He takes a random sample of 36 students who took a morning class and, independently, another random sample of 36 students who took an afternoon class. He finds that the morning group scored an average of 74 with a standard deviation of 8, while the evening group scored an average of 68 with a standard deviation of 10. The population standard deviation of scores is unknown but is assumed to be equal for morning and evening classes. Let µ1 and µ2 represent the population mean final exam scores of statistics' courses offered in the morning and the afternoon, respectively. At the 1% significance level, does the evidence support the professor's claim?
A) No, because the test statistic is less than the critical value.
B) Yes, because the test statistic is less than the critical value.
C) No, because the test statistic is greater than the critical value.
D) Yes, because the test statistic is greater than the critical value.
A) No, because the test statistic is less than the critical value.
B) Yes, because the test statistic is less than the critical value.
C) No, because the test statistic is greater than the critical value.
D) Yes, because the test statistic is greater than the critical value.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
79
A statistics professor at a large university hypothesizes that students who take statistics in the morning typically do better than those who take it in the afternoon. He takes a random sample of 36 students who took a morning class and, independently, another random sample of 36 students who took an afternoon class. He finds that the morning group scored an average of 74 with a standard deviation of 8, while the evening group scored an average of 68 with a standard deviation of 10. The population standard deviation of scores is unknown but is assumed to be equal for morning and evening classes. Let µ1 and µ2 represent the population mean final exam scores of statistics' courses offered in the morning and the afternoon, respectively. Which of the following hypotheses will test the professor's claim?
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: µ1 - µ2 > 0, HA: µ1 - µ2 ≤ 0
D) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0
A) H0: µ1 - µ2 ≤ 0, HA: µ1 - µ2 > 0
B) H0: µ1 - µ2 ≥ 0, HA: µ1 - µ2 < 0
C) H0: µ1 - µ2 > 0, HA: µ1 - µ2 ≤ 0
D) H0: µ1 - µ2 = 0, HA: µ1 - µ2 ≠ 0

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck



