Robert Hope Received a Welcome Surprise in This Management Science $25 \%$

Robert Hope received a welcome surprise in this management science class; the instructor has decided to let each person define the percentage contribution to their grade for each of the graded instruments used in the class. These instruments were: homework, an individual project, a mid-term exam, and a final exam. Robert's grades on these instruments were 75, 94, 85, and 92, respectively. However, the instructor complicated Robert's task somewhat by adding the following stipulations:
-homewark can account far up to $25 \%$ of the grade, but must be at least $5 \%$ af the grade;
- the praject can account for up to $25 \%$ of the grade, but must be at least $5 \%$ af the grade;
- the mid-term and final must each accaunt far betwen $10 \%$ and $40 \%$ of the grade but cannot accaunt far mare than $7 \%$ of the grade when the percentages are cambined; and
- the project and final exam grades may not collectively constitute more than $50 \%$ of the Iratade. The following LP model allows Robert to maximize his numerical grade.
$\begin{array} { l } \text { Let } \quad W _ { 1 } = \text { weight assigned to hamewark } \\ \quad \quad \quad W _ { \mathbf { 2 } } = \text { waight assigned to the praject } \\\quad \quad \quad W _ { 3 } = \text { weight assigned to the midi-term } \\ \quad \quad \quad W _ { 4 } = \text { waight assigned to the final } \\\\\text { MAX: } \quad 75 \mathrm {~W} _ { 1 } + 94 \mathrm {~W} _ { 2 } + 85 \mathrm {~W} _ { 3 } + 92 \mathrm {~W} _ { 4 } \\\text { Subject to: } \quad W _ { 1 } + W _ { 2 } + W _ { 3 } + W _ { 4 } = 1 \\ \quad \quad \quad \quad \quad \quad W _ { 3 } + W _ { 4 } \leq0 .70 \\ \quad \quad \quad \quad \quad \quad W _ { 3 } + W _ { 4 } \geq 0.50 \\ \quad \quad \quad \quad \quad \quad\text { 0. } 05 \leq W _ { 1 } \leq 0.25 \\ \quad \quad \quad \quad \quad \quad\text { 0. 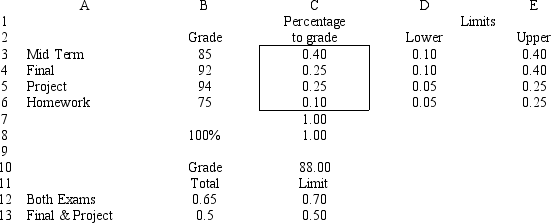} 05 \leq W _ { 2 } \leq 0.25 \\ \quad \quad \quad \quad \quad \quad\text { 0.10 } \leq W _ { 3 } \leq 0 .4 0 \\ \quad \quad \quad \quad \quad \quad0 .10 \leq W _ { 4 } \leq 0.40 \\\end{array}$ What values would you enter in the Risk Solver Platform (RSP) task pane for the cells in this Excel spreadsheet implementation of this problem?
Objective Cell:
Variables Cells:
Constraints Cells: