An investor is developing a portfolio of stocks. She has identified 3 stocks in which to invest. She wants to earn at least 11% return but with minimum risk.
Let: Pi = proportion of total funds invested in i, i = A, B, C
The NLP for this problem is:
MIN:
Subject to:
What formulas should go in cells G4:J14 of the spreadsheet for this problem? NOTE: Formulas are not required in all of these cells.
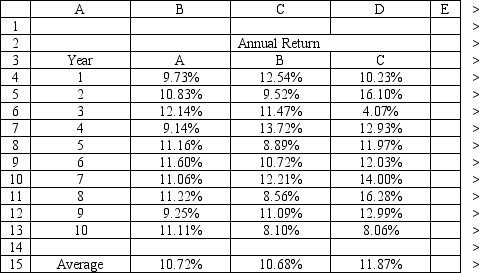
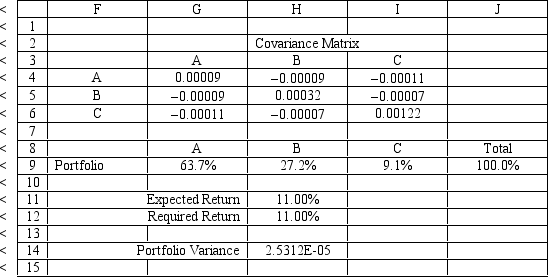
Correct Answer:
Verified
Q49: An office supply company is attempting
Q50: How much must the objective function
Q51: An investor is developing a portfolio
Q52: An investor wants to determine how much
Q53: A company makes products A and
Q55: How much are additional units of
Q56: A company wants to locate a
Q57: An investor wants to determine how much
Q58: How many local minimum solutions are there
Q59: How many local maximum solutions are there
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents