An Industrial Psychologist Is Investigating the Effects of Work Environment $H _ { 0 } : \mu _ { \text {green } } = \mu _ { \text {blue } } = \mu _ { \text {gray } } = \mu _ { \text {red, } }$

An industrial psychologist is investigating the effects of work environment on employee attitudes. A group of 20 recently hired sales trainees were randomly assigned to one of four different ʺhome
Roomsʺ- five trainees per room. Each room is identical except for wall color. The four colors used
Were light green, light blue, gray, and red. The psychologist wants to know whether room color
Has an effect on attitude, and, if so, wants to compare the mean attitudes of the trainees assigned to
The four room colors. At the end of the training program, the attitude of each trainee was measured
On a 60-pt. scale (the lower the score, the poorer the attitude) . The data was subjected to a
One-way analysis of variance.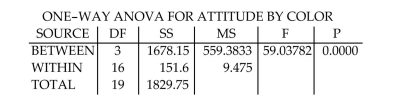


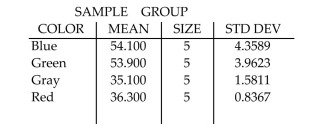
Give the null hypothesis for the ANOVA F-test shown on the printout.

A) $H _ { 0 } : \mu _ { \text {green } } = \mu _ { \text {blue } } = \mu _ { \text {gray } } = \mu _ { \text {red, } }$ , where the $\mu _ { \text {'s represent mean attitudes for the four rooms } }$
B) $H _ { 0 } : \mu _ { 1 } = \mu _ { 2 } = \mu _ { 3 } = \mu _ { 4 } = \mu _ { 5 }$ , where the $\mu _ { i }$ represent attitude means for the $i$ th person in each room
C) $H _ { 0 } : p _ { \text {green } } = p _ { \text {blue } } = p _ { \text {gray } } = p _ { \text {red, } }$ , where the $p$ 's represent the proportion with the corresponding attitude
D) $H _ { 0 } : x _ { 1 } = x _ { 2 } = x _ { 3 } = x _ { 4 }$ , where the $x ^ { \prime }$ 's represent the room colors