THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION:
An economist is in the process of developing a model to predict the price of gold.She believes that the two most important variables are the price of a barrel of oil (x1)and the interest rate (x2).She proposes the model y = β0 + β1x1 + β2x2 + β3x1x3 + ε.A random sample of 20 daily observations was taken.The computer output is shown below.
THE REGRESSION EQUATION IS
y = 115.6 + 22.3x1 + 14.7x2 - 1.36x1x2
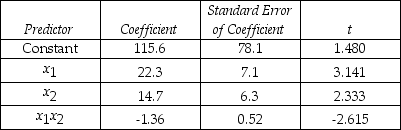
S = 20.9 R-Sq = 55.4%
ANALYSIS OF VARIANCE
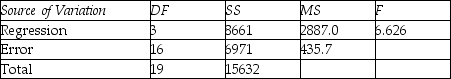
-Suppose that a regression relationship is given by Y = β0 + β1X1 + β2X2 + ε.If the simple linear regression of Y on X1 is estimated from a sample of n observations,the resulting slope estimate will generally be biased for β1.But if the sample correlation between X1 and X2 is 0,the slope will not be biased for β1.
How does X2 affect β1 if the sample correlation between X1 and X2 is zero?
Correct Answer:
Verified
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Q150: Test the hypotheses H0: There is no
Q151: THE NEXT QUESTIONS ARE BASED ON THE
Q152: THE NEXT QUESTIONS ARE BASED ON THE
Q154: THE NEXT QUESTIONS ARE BASED ON THE
Q155: THE NEXT QUESTIONS ARE BASED ON THE
Q157: THE NEXT QUESTIONS ARE BASED ON THE
Q158: THE NEXT QUESTIONS ARE BASED ON THE
Q159: THE NEXT QUESTIONS ARE BASED ON THE
Q160: THE NEXT QUESTIONS ARE BASED ON THE
Q161: THE NEXT QUESTIONS ARE BASED ON THE
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents