The amount of time it takes to serve each customer in a bank is a random variable,X,with a mean of μ minutes and a standard deviation of σ minutes.When you arrive at the bank there are three customers in front of you.If the times for the three customers are independent of one another,which of the following shows the correct method for calculating the variance of your wait time?
A) Var(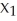
+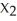
+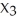
) =
Var(X) = 9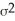
B) Var(3X) = 3Var(X) = 3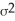
C) Var(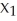
+
+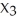
) =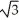
Var(X) =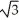
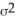
D) Var(3X) =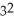
Var(X) = 9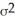
E) Var(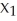
+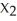
+
) = Var(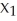
) + Var(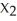
) + Var(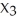
) = 3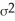
Correct Answer:
Verified
Q102: The amount of time it takes to
Q103: The amount of money that Jon can
Q104: Janet is planning to rent a booth
Q105: If X and Y are independent random
Q106: The amount of money that Maria earns
Q107: Janet is planning to rent a booth
Q108: The amount of money that Maria earns
Q110: An insurance company estimates that it should
Q118: The amount of money that Jon can
Q193: Miguel buys a large bottle and a
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents