A rocket is moving in outer space where no external forces exist.Suppose its mass is mR and its velocity is vR at a particular time.When a mass mF of fuel is ejected,the mass of the rocket becomes (mR - mF) and its velocity increases to (vR+ 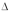 vR) .Suppose the fuel is always ejected at a constant speed vF with respect to the rocket.Derive an expression for vR as a function of mR given the rocket's initial mass and speed are m0 and v0,respectively.
vR) .Suppose the fuel is always ejected at a constant speed vF with respect to the rocket.Derive an expression for vR as a function of mR given the rocket's initial mass and speed are m0 and v0,respectively.
A) vR = v0 + vF ln(mR/m0)
B) vR = v0 - vF ln(mR/m0)
C) vR = v0 - vF exp(m0/mR)
D) vR = v0 - vF ln(m0/mR )
E) vR = v0 + vF exp(mR/m0)
Correct Answer:
Verified
Q24: A 25-kg boy jumps off of a
Q25: A ping pong ball has a mass
Q26: Two equal masses are connected by an
Q27: Find the y-coordinate of the center of
Q28: A 25-kg boy jumps off of a
Q30: A 25-kg boy jumps off of a
Q31: The center of mass of a uniform,symmetric
Q32: The center of mass of an irregular
Q33: The coordinates for the center of mass
Q34: A rod has a linear density
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents