If the underlying populations cannot be assumed to be normal, then by the central limit theorem, the sampling distribution of 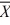 1 -
1 - 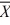 2 is approximately normal only if both sample sizes are sufficiently large-that is, when ________.
2 is approximately normal only if both sample sizes are sufficiently large-that is, when ________.
A) n1 + n2 = 30
B) n1 + n2 ≥ 30
C) n1 = 30 and n2 = 30
D) n1 ≥ 30 and n2 ≥ 30
Correct Answer:
Verified
Q29: When testing the difference between two population
Q30: When testing the difference between two population
Q31: Assume the competing hypotheses take the following
Q32: A demographer wants to measure life expectancy
Q33: When calculating the standard error of
Q35: When comparing two population means, their hypothesized
Q36: Assume the competing hypotheses take the following
Q37: If the sampling distribution of 
Q38: The choice of an appropriate test for
Q39: What formula is used to calculate the
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents