Solved

The Following Data Show the Demand for an Airline Ticket

Question 96

Multiple Choice

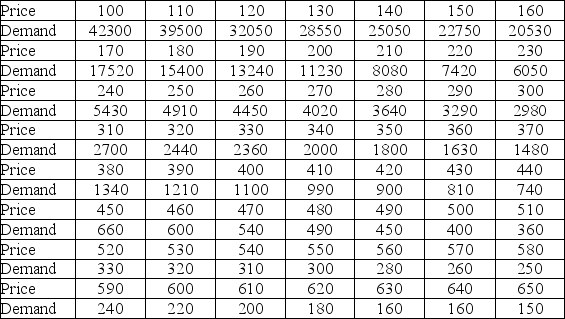The following data show the demand for an airline ticket dependent on the price of this ticket. The following data show the demand for an airline ticket dependent on the price of this ticket. For the assumed cubic and log-log regression models, Demand = β<sub>0</sub> + β<sub>1</sub>Price + β<sub>2</sub>Price<sup>2 </sup>+ β<sub>3</sub>Price<sup>3 </sup>+ ε and ln(Demand) = β<sub>0</sub> + β<sub>1</sub>ln(Price) + ε, the following regression results are available. Assuming that the sample correlation coefficient between Demand and = exp(26.3660 - 3.2577 ln(Price) + (0.2071) <sup>2</sup>/2) is 0.956, what is the predicted demand for a price of $250 found by the model with better fit? A) 4,447.88 B) 3,914.38 C) 4,029.38 D) 5,175.09 For the assumed cubic and log-log regression models, Demand = β0 + β1Price + β2Price2 + β3Price3 + ε and ln(Demand) = β0 + β1ln(Price) + ε, the following regression results are available. 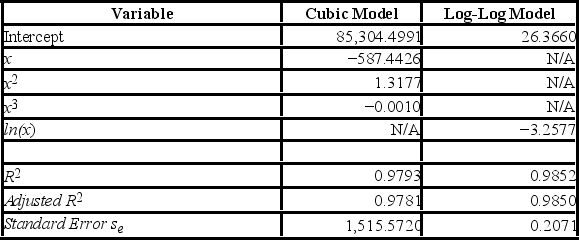The following data show the demand for an airline ticket dependent on the price of this ticket. For the assumed cubic and log-log regression models, Demand = β<sub>0</sub> + β<sub>1</sub>Price + β<sub>2</sub>Price<sup>2 </sup>+ β<sub>3</sub>Price<sup>3 </sup>+ ε and ln(Demand) = β<sub>0</sub> + β<sub>1</sub>ln(Price) + ε, the following regression results are available. Assuming that the sample correlation coefficient between Demand and = exp(26.3660 - 3.2577 ln(Price) + (0.2071) <sup>2</sup>/2) is 0.956, what is the predicted demand for a price of $250 found by the model with better fit? 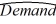A) 4,447.88 B) 3,914.38 C) 4,029.38 D) 5,175.09 Assuming that the sample correlation coefficient between Demand and The following data show the demand for an airline ticket dependent on the price of this ticket. For the assumed cubic and log-log regression models, Demand = β<sub>0</sub> + β<sub>1</sub>Price + β<sub>2</sub>Price<sup>2 </sup>+ β<sub>3</sub>Price<sup>3 </sup>+ ε and ln(Demand) = β<sub>0</sub> + β<sub>1</sub>ln(Price) + ε, the following regression results are available. Assuming that the sample correlation coefficient between Demand and = exp(26.3660 - 3.2577 ln(Price) + (0.2071) <sup>2</sup>/2) is 0.956, what is the predicted demand for a price of $250 found by the model with better fit? A) 4,447.88 B) 3,914.38 C) 4,029.38 D) 5,175.09 = exp(26.3660 - 3.2577 ln(Price) + (0.2071) 2/2) is 0.956, what is the predicted demand for a price of $250 found by the model with better fit?


A) 4,447.88
B) 3,914.38
C) 4,029.38
D) 5,175.09

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q91: The following data show the demand for

Q92: In which of the following models does

Q93: In which of the following models does

Q94: The following data show the demand for

Q95: The scatterplot shown below represents a typical

Q97: Thirty employed single individuals were randomly selected

Q98: Which of the following is a typical

Q99: In which of the following models does

Q100: Which of the following is not a

Q101: Thirty employed single individuals were randomly selected

Unlock this Answer For Free Now!

View this answer and more for free by performing one of the following actions

qr-code

Scan the QR code to install the App and get 2 free unlocks

upload documents

Unlock quizzes for free by uploading documents