If 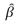_j,an Unbiased Estimator of 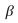_j,is Consistent,then The

If _j,an unbiased estimator of _j,is consistent,then the:

A) distribution of 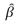_jbecomes more and more loosely distributed around
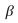_jas the sample size grows.
B) distribution of 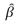_jbecomes more and more tightly distributed around
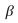_jas the sample size grows.
C) distribution of 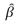_jtends toward a standard normal distribution as the sample size grows.
D) distribution of 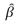_jremains unaffected as the sample size grows.