(Requires Appendix Material)The Long-Run,stationary State Solution of an AD(p,q)model,which Can
(Requires Appendix material)The long-run,stationary state solution of an AD(p,q)model,which can be written as A(L)Yt = β0 + c(L)Xt-1 + ut,where 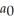 = 1,and
= 1,and 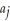 = -βj,cj = δj,can be found by setting L=1 in the two lag polynomials.Explain.Derive the long-run solution for the estimated ADL(4,4)of the change in the inflation rate on unemployment:
= -βj,cj = δj,can be found by setting L=1 in the two lag polynomials.Explain.Derive the long-run solution for the estimated ADL(4,4)of the change in the inflation rate on unemployment: 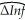 t = 1.32 - .36 ΔInft-1 - 0.34vInft-2 + 0.7ΔInft-3 - 0.3ΔInft-4
t = 1.32 - .36 ΔInft-1 - 0.34vInft-2 + 0.7ΔInft-3 - 0.3ΔInft-4
-2.68Unempt-1 + 3.43Unempt-2 - 1.04Unempt-3 + .07Unempt-4
Assume that the inflation rate is constant in the long-run and calculate the resulting unemployment rate.What does the solution represent? Is it reasonable to assume that this long-run solution is constant over the estimation period 1962-1999? If not,how could you detect the instability?
Correct Answer:
Verified
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Q32: The BIC is a statistic
A)commonly used to
Q41: You have collected data for real GDP
Q43: Find data for real GDP (Yt)for the
Q44: (Requires Appendix material): Show that the AR(1)process
Q45: Consider the AR(1)model Yt = β0 +
Q46: Consider the following model
Yt = α0 +
Q46: You want to determine whether or not
Q47: The following two graphs give you a
Q49: You have decided to use the Dickey
Q50: Consider the standard AR(1)Yt = β0 +
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents